Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

274
The
Orbits
Method
or
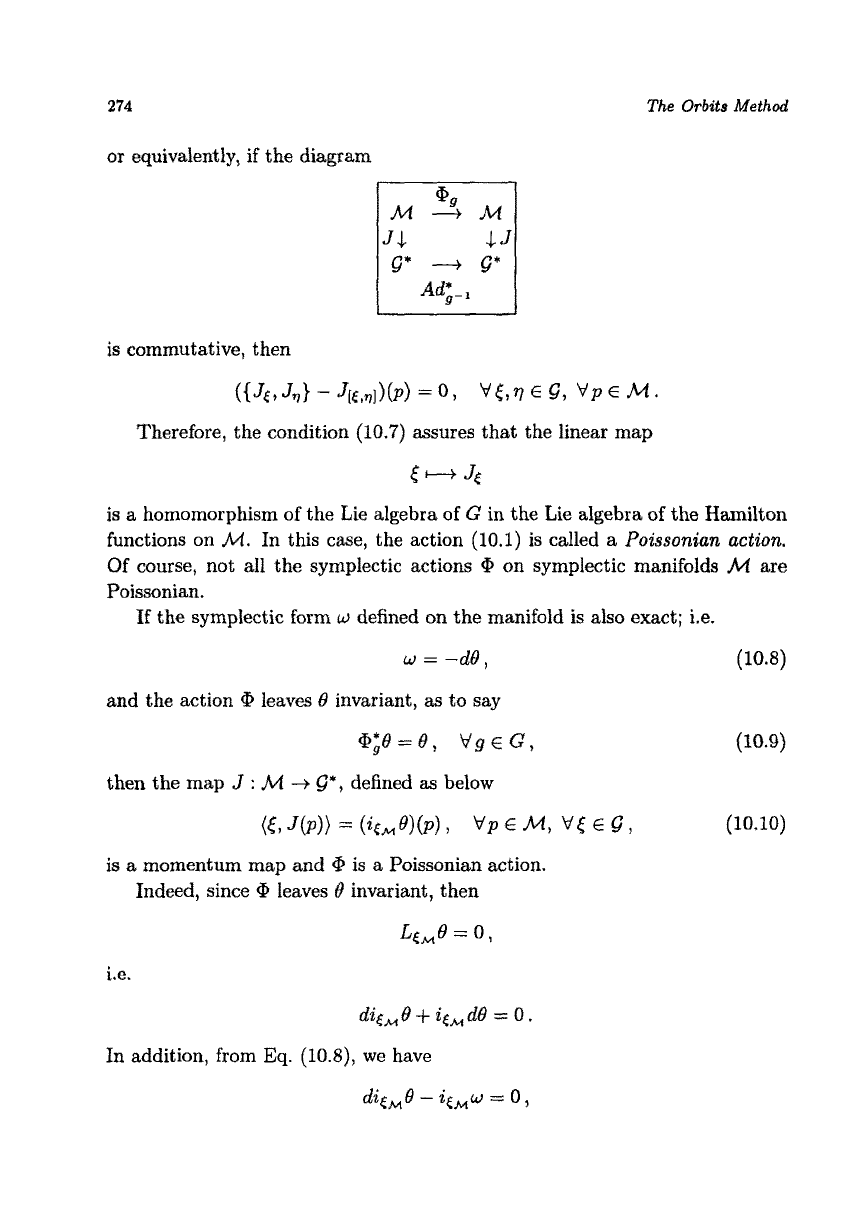
equivaiently, if the diagram
is
commutative, then
({J€,Jv)-J[€,q])(P)
=o,
VE,rlEG,
VPEM.
‘5
-
JE
Therefore, the condition (10.7) assures that the linear map
is
a homomorphism of the Lie algebra
of
G
in the Lie algebra of the Hamilton
functions on
M.
In this case, the action (10.1)
is
called a
Poissonian
action.
Of course, not
all
the symplectic actions
@
on symplectic manifolds
M
are
Poissonian.
If
the symplectic form
w
defined on the manifold
is
also exact;
i.e.
w
=
-d6,
(10.8)
and the action
@
leaves
B
invariant,
its
to
say
@;6=6,
VgEG,
(10.9)
(t>
J@))
=
(i<M6)(p)
9
’P
M,
‘t
9
9
(10.10)
then
the map
J
:
M
-+
9*,
defined
as
below
is
a
momentum map
and
cf,
is
a
Poissonian action.
Indeed, since
@
leaves
B
invariant, then
L,cM6
=
0,
i.e.
dicM
6
-+
icM
d6
=
0
I
In addition, from
Eq.
(10.8), we have
di<,B
-
itMw
=
0,

Reduced
Phase
Space
275
as
to say
dJ6
=
icMw,
that
is,
J
is
a momentum map. Now, because (see Appendix
D)
(Adg-lOM
(PI
=
(@g-1)wDS(p)
(<M
(@g(P)))
1
we also have
i.e.
J(@g(P))
=
Adi-1
(J(P))
t
VP
E
M,
Vg
E
G*
If
p
is
an
element
of
G*,
the
set
Gp
=
{g
E
G
:
Adi-ip
=
p}
is
a subgroup
of
G,
acting on
M.
The group
G
usually permutes the sets
of
the type
J-'(p)
=
{p
E
M
:
J(p)
=
p}
.
In fact,
if
p
E
J-l(p),
then
J(p)
=
p
but
Qg(p),
with
g
E
G,
may not belong
to
J-'(p).
Let
p
E
J-l(p)
and
g
be an element of
G,,
then
Ad;-i(J(p)) =Ad;-ip=
p,
which, by using
Eq.
(10.7),
can be written
as
Adi-1
(J(P))
=
J(Qg(P))
9

276
The
Orbits
Method
so
that
J(@.,(P))
=
P,
vg
E
G,,
VP
E
J-YP)
7
that is,
G,
leaves
J-'(p)
fixed.
Then, by
Eq.
(lO.l),
we
can define an action
@
of
G,
on
J-l(p),
@
:
(SlP)
E
G,
x
J-'(p)
-+
@(g,P)
E
J-'(p)
*
(10.12)
The orbit of the point
p
E
J-'(p)
under the action of the group
G,
is
given
bY
G,*P=
{@g(P)
:
9
E
G,}.
(10.13)
We can introduce an
equivalence relation
on
J-'(p)
by defining two points
The set of equivalence classes, denoted as usual by
J-l(p)/G,,
is the set
The point
p
E
G*
is
said to be a
regular value
of
J
if,
for
every
p
E
J-'
(p),
of
J-'(p)
to be
equivalent
if
they belong to the same orbit (Eq. (10.13)).
of the orbits of the points of
J-'(p)
under the action of
G,.
the derivative
JaP
:
7,M
+
7,B*
is a surjective map; in such case it can be proven' that the set
J-'(,u)
is a
differential manifold.
The action (Eq. (10.12)),
@
:
(SlP)
E
G,
x
J-Yd
+
@(g)P)
E
J-'(p)
1
is called a
proper action
if
it satisfies the following condition:
If
(pn)nE~
and
(Qgn(p,)),E~
are sequences
of
points
of
J-'(p))
which con-
verge
in
J-'(p),
then (gn)nEN admits a subsequence which converges
in
G,.
The hypotheses that
p
E
B'
is a regular value of
J
and that the action
(10.12)
is a proper action are sufficient conditions to assure that
Jdl(p)/G,
is a differential manifold and that the map
flp
:
J-W
--t
J-l(P)/Gp
)
which associates with every point of
J-'(p))
the orbit to which it belongs, and
its derivative
(flp)*p
:
%J-'(p)
+
TG,,T(J-'(~)/G~)
I
(1
0.14)
are surjective maps.

Reduced
Phdae
Space
277
The ~~nifoId
J-l(~~/Gp
is
called the
~d~ce~ phuse space
and can be
endowed with
a
natural symplectic structure.
Indeed, let
us
consider two vectors and
@
tangent to
J-l(p)/G,
at the
point
y,
which
is
the orbit of
a
point
P1(p)
under the action
of
G,.
Let us
choose
and
@
tangent to the orbit at
y
can be obtained from some vectors
V
and
W,
tangent to
P1(p)
at
the point
p,
by
wing
the map
(10.14).
We
can
thus define on
~-l(~)/Gp
a
bilinear form
$2,
expressible
in
terms
of
the symplectic form
u
on
M
point
p
in this orbit: the vectors
that
is,
0
The bilinear
form
a,
does not depend
by
the choice
of
the point p in
the
orbit and
of
the vectors
V
and
W
of
TJ-l(p).
Indeed, let
?(Gp
*
=
{SM(P)
:
E
gp)
2
where
&@)
is
defined
in
Eq.
(10.3)
and
gp
is
the Lie algebra of
Gp.
We
can
now prove that
7p(GP
*
P)
=
7,@
'
P)
n
7,J--'(P)
*
(10.16)

As
a
matter of fact
if
<~(p)
belongs to
&(G*p),
then
Eq.
(10.16)
can
be
seen to be equivalent
to
saying that
(~(p)
E
7pJ-l(p)
if and only if
(
E
Gp.
Therefore, we may write
since
J
satisfies
Eq.
(10.7).
Because
J(p)
=
p
for every
p
E
J-I(p),
~J-'((CL)
s
ker
J*~,
Then,
(~(p)
E
7pJ-'(p)
=
ker
JSp
if and only
if
that
is,
if
(
E
Gp.
Let
V
be
a
vector
in
7,M;
for every
E
9,
from Eq.
(10.4),
we
have
~p(<M(p),
v,
==
(~€~w)~(v)
=
dJ€fp(V)
*
If
a(t)
is
the integral curve
of
V,
then
so
that,
V
E
7pJ-l(p)
=
ker
Jwp
if
and only
if
or equivalently,
if
and
only
if
Wp(<M~~,V~
0,
v<
*
(10.17)
Therefore,
the two tangent spaces
%(J-I(pL))
and
7,(G
.
p)
are
one
the
Now, because
orthogonal complement (with respect to
w)
of the other.

Reduced
Phase
Space
279
we have
and
(Zg)*p(<M(p))
=
0
1
v<
E
Gp.
(10.18)
In
this
way the vectors
V
and
W,
which correspond to the vectors
and
@
in
Eq.
(10.15), are defined up to
a
vector of
G(G,
+
p);
but the addition
of
a vector of this space to
V
and
W
does not modify the right-hand side of
Eq.
(10.15),
because the spaces
7pJ-'l(p)
and
7pG.
p
are "orthogonal".
Concerning the independence
of
Eq.
(10.15) from the choice
of
the point
p
on
the
orbit, this depends on the fact that the action
CP
is symplectic and on
the invariance
of
J-'(,u).
In
fact,
(~~Rp~~~(p)(v/,
W')
=
#~g(p)(v/,
W')
5
V'I
W'
E
Xis(p)J-'(ct)
?
V'
=
(W*P(V)
I
W'
=
(@g)*pW)
,
v,
W
E
TJ-l(I.4
I
(~~~~~~~(P~(V',
W')
=
#~g(p)((@~)*P(v),
(~g)*P(W))
so
that, by
using
the relations
we finaliy obtain
=
#p(V,
W>
=
(~~~p)p(~
W)
*
0
The bilinear
form
R,
is
not
degenerate.
Indeed,
if
Qp(P,
W)
=
0
for every
l8',
the representative vector
V
should be orthogonal to all the vectors
of
GP1(p),
so
that it should belong to
G(G
*
p)
and,
by
Eq.
(10.16), to
T(G,
p).
Therefore, by
Eq.
(10.18), we have
v
=
(~p)*p(v~
=
0.
Q
The daflerential2-fom
Clp
is
closed.
280
The
Orbits
Method
In
fact, because
dw
=
0,
we have
Thus, from
we also have
dfl,
=
0,
since
(A,)*
is
a
surjective application.
For
more details on reduction processes, see Refs.
106, 41, 153,
128
and
107;
last reference also containing an example of noncommutative reduction in
the context of noncommutive geometry.
12133
10.2
Orbits
of
a Lie Group in the Coadjoint Representation
In the previous section we have seen how, given
a
symplectic manifold and
a
symplectic action of
a
Lie group on this manifold, which admits
a
momentum
map, under appropriate conditions, we can define
a
symplectic structure on
the reduced phase space. In this section we are going to see how,
for
the
cotangent bundle
7'G
of
a
Lie group
G,
we can define
a
symplectic action and
a
momentum map, such that the reduced phase space coincides with the orbit
of the group in the coadjoint repre~entation.~~
Let
G
be a Lie group and consider the action of
G
on itself given by the
left translations
L,
@
:
(9,h)
E
G
x
G
+
@(g,h)
=
gh
E
G,
(10.19)
that
is,
by
setting
Qg
=
L,,
Yg
E
G.
By using
Eq.
(10.19),
we can introduce an action
$,
of
G
on
T*G

Orbita
of
a
Lie
Group
in
the
Coadjoint
Representation
281
where
ah
is
an arbitrary point of
TG,
that
is
a
differential l-form on the
tangent space to
G
at
the point
h,
and
(Lg-,)f;h
:
TG
-+
T;G
(10.21)
is
the transposed operator of the derivative of
L,-I
at the point
gh,
(L,-I)*~~
:
GhG
-+
ThG
(.
Therefore,
Eq.
(10.20) gives
$'(el
ah)
=
LZ(Qlt)
=
ah
3
$J(fl$J($,
ah))
=
$(f,
Llf-i(Qh))
=
(L;-i
Llf-I)(@h)
=
L;-lj-l(uh)
=
qfg)-lQh
=
~(~g,Qh).
By
Eq.
(10.21), we can
see
that
$J
maps the differential l-form
ah
on
ThG
to
a
differential l-form on
7ghG.
The diffeomorphisms (10.21) preserve the
canonical differential l-form
8
on the cotangent bundle.
Moreover, because
LE-~u
=
-Llf-id8
=
-dLi-,O
=
-d8
=
u
,
Vg
E
G,
where
u
is
the canonical symplectic form, the action
(10.20)
on the cotangent
bundle
is
a
symplectic action, This allows us to define
a
map
J
for
$'
as
in
Eq.
(10.10).
Let
<
be
an element
of
9
and consider the action (10.19)
of
G
into
itself.
The map
QPE
:
(t,g)
E
R
x
G
-+
@c(t,g)
=
@(efPE,g)
E
G
defines an action of
R
on
G.
The vector field
is
a
right invariant vector field, because
@(ett,g)
=
etEg
=
Rg(etE),
and

282
The
Orbits
Method
From
Eq.
(lO.lO),
we deduce that the momentum
J
is
the
map
J
:
a,
E
T*G
-+
J(a,)
E
8*,
(10.22)
defined by (Appendix
E)
(c,
J(ag))
=
ag(cG(g))
ag((%)*e(C)>
=
(Riag)([)
T
Vt
E
9
that is
J(ag)
=
Ria,.
(10.23)
Every point
p
in
E*
is a regular value for the momentum (10.23); that is,
for every
ag
E
J-'(p),
the map
J*a,
:
Z,(TG)
-+
7,E*
is
surjective. Indeed
if
Y
E
T,G*
and
p(t)
is
the integral curve
of
Y
with
P(0)
=
I.1,
by applying to
1.1(~~
the operator
Ri-l,
we obtain
a
curve in
PG
throug~
ag
at
t
=
0.
This
is
so
because
Rp,
=
/.L,
va,
E
J-'(I.1)
and
which says that,
for
every
Y
E
7,4*,
there exists a vector
X
E
T,,(TG)
such
that
J*a,(Xf
=
Y.
If
for every
g
f
G,
to the element
p
in
r?*
we apply the right translation
R;-l,
we obtain a right invariant differential 1-form on
G,
a,(g)
=
R;-ip*
(10.24)
By letting
g
vary in
G,
Eq. (10.24) defines
all
and only
the
points of
J-l(p),
because
of
Eq. (10.23). From
Eq.
(10.24), it
is
evident that the action of
L:

Orbits
of
a
Lie
Group
in
the
Coadjoint
Representation
283
on the cotangent bundle maps points
of
J-l(p)
to points
of
J-'(p)
for all
g
be~ong~~g to
the
subgroup
G,
defined by
Gp
=
(9
E
G:
Adi-Ip
=
p}.
(10.25)
From the relation
(10.26)
it
follows
that
G,
can be also expressed in the form
G,
=
(9
E
G
:
L;-1ap
=
a,}.
(10.27)
fiom
Eq,
(10.26),
we
can
define an action of
GP
on
J-'(p),
which coincides
with the action
(10.20),
when it
is
restricted to
G,
x
J-'(p).
This action is a
proper action: in fact, if
aP(hn)
is
a
sequence of points in
P1(pL)
converging
to a point
of
J-'(p),
we have
lim
a,(hn)
=
a,@).
n4+m
By
the continuity
of
the map
(10.24),
we have
so
that
lim
h,
=h
n++w
with
h
E
G,,
since
G,
is closed.
for
n
+
+m.
We
can
thus
write
Let
us
suppose that the sequence
L*-la,(hn)
converges to a point
of
J-I(p)
Sn
n++m
lim
Li;lap(hn)
=
n++w
lim
a
pgn
(
h
)=a,
where
obviously,
gn
E
G,,
Vn E
N.
SO
the sequence
(9nhn)nE.N
converges to
a
point
f
of
G,,
because
G,
is
closed.
Therefore,
Iim
h,=h,
lim
g
h
-f,
n++m n++w
n-
