Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


244
Symplectic Manifolds and Hamiltonian Systems
0
A
symplectic transformation,
as
defined in Part
I,
is just
a
map between
two Darboux chart
(U,
cp
3
(p/q))
and
(V,
$J
f
(r/x));
that is, it is
a
map such that
dpi
A
dqi
=
dri
A
dXi
-
0
The above relation is the exterior derivative of
which is just the Lie condition
for
a
transformation to be symplectic.
0
A
completely canonical transformation is
a
map between two
almost
Darbow charts, in the sense that
0
The Lagrange bracket
,
dxh
axh
arb
axh
%,q3
-
--
-
--
b
I-
api
aqj
aqj
api
I
in which the inversion of the position of covariant and contravariant
indices is just caused by the old notations, can be then obtained much
more easily by expanding the previous equality to the form
which gives the familiar conditions for
a
transformation to be com-
pletely canonical,
c[Pa,qj]
=
6;.
0
The Poisson bracket
{rh,xk}
as
defined in Part
1
is,
vice versa,
ob-
tained expanding the inverse equality
ad da
-
A-=c-A-
8.h
aXh
api
aqi
in the inverted direction, to obtain
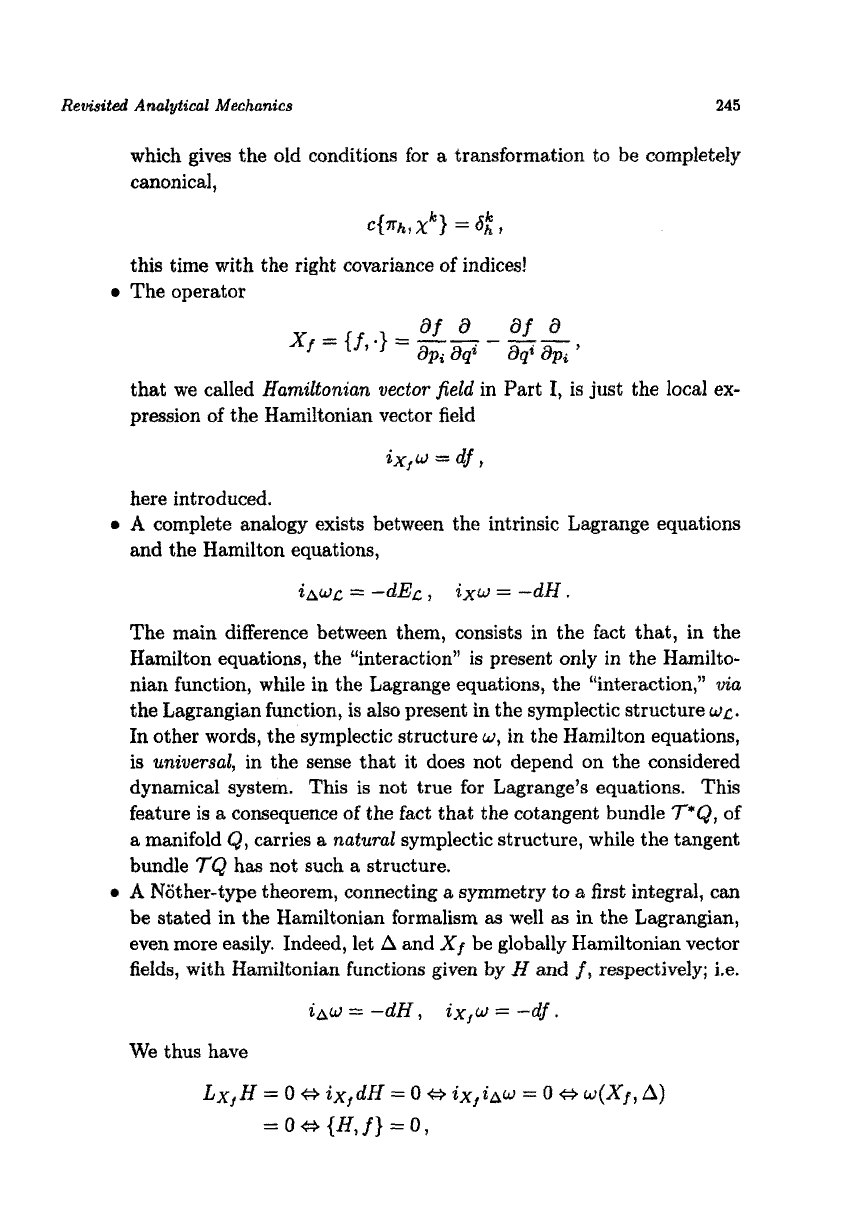
Revisited
Analytical
Mechanics
245
which gives the ofd conditions for
a
transformation to be completely
canonical,
C(rhThr
Xk)
=
@
3
this time with the right covariance of indices!
0
The operator
that we called
Harniltonian vector
field
in Part
I,
is
just the local ex-
pression
of
the
H~iltoni~ vector field
ix,~
=
df
,
here introduced.
and the Hamilton equations,
0
A
compfete andogy exists between the intrinsic Lagrange equations
i~w~
=
-dE&,
ixw
=
-dH
.
The main difference between them, consists in the fact that, in the
Hamilton equations, the “interaction”
is
present only in the Hamilto-
nian function, while in the Lagrange equations, the “interaction,”
via
the Lagrangian function,
is
also present in the symplectic structure
WE.
In other words, the symplectic structure
w,
in the Hamilton equations,
is
universal,
in the sense that it does not depend on the considered
dynamical system. This
is
not true for Lagrange’s equations. This
feature is
a
consequence
of
the fact that the cotangent bundle
T*Q,
of
a manifold
Q,
carries a
natural
symplectic structure, while the tangent
bundle
‘T&
has not such
a
structure.
0
A
Nother-type theorem, connecting
a
symmetry to
a
first integral, cm
be stated in the Hamiltonian formalism
M
well
as
in the Lagrangian,
even more easily. Indeed, let
A
and
Xj
be globally Hamiltonian vector
fields,
with ~amiIto~ian functions given
by
H
and
f,
respectively; i.e.
iAw
=
-dH,
ix,w
=
-df
.
We thus have
Lx,
H
=
0
es
ix,
dH
=
0
~3
ix,iAw
=
0
H
w(Xf,
A)
=
0
es
{HJ}
=
0,

246
Symplectic
Manifolds and Hamiltonian
Systems
so
that
Lx,H
=
0
H
LAf
=
0,
that
is,
to any symmetry of the Hamiltonian corresponds
a
constant
of
the motion and,
vice versa,
any constant of the motion
is
the
in-
finitesimal generator
of
a symmetry transformation.
In other words,
A
first znteg~ul,
for
a
~u~zltoniu~ dynamics, generates a one-parameter
group
of
symplectomorphisms, which leaves the Hamiltonian function
H
invariant
and,
vice versa, with
any
one-parameter
group
of
symplec-
to-mo~~zsms, ~e~~ng
H
in~~~a~~, we
can
associate
a
first ~nte~~
The Sec.
(4.1),
on the
Integral invariants,
can be revisited
as
follows.
Let
us
observe that the Lie derivative, with respect to the vector field
X,
of
the differential n-form
CY
=
p(~)d~'
Adz2
A***AdXn,
on an n-dimensional manifold
M,
is
given by
Lxa
=
dixa
=
div(~~)dX1
A
dx2
A
-.
A
dxn
,
so
that the relation
(4.5),
for
a function
p
not depending explicitly on
time,
simply
says
that
It
follows that a necessary and sufficient condition for
fu
a
to be in-
variant
is
Lxa
=
0.
What has been said can be generalized
as
follows.
A
differential k-form
a
E
hk((M),
on an n-dimensional manifold
M,
is
said
to be
an
ubso~~~e i~te~a~ 2~~u~ant
of
the complete vector field
X,
if
Lxa=O.
The latter is equivalent to
cp:(4CpT(P)))
=
4P)
t
where
(p7
denote the
flow
of the vector field
X,
(9.25)

Revisited Analytical
Mechanics
247
If
U
is
a
k-dimens~o~al submanifold of
M
and
i
the immersion map
i:Ut,M,
(p7
o
i)
(U)
is
a
new k-dimensional submanifold of
M
,
and
J
a
=
~(~~
o
i)*a
=
~(i*
o
V;)Ly.
(Vp+Oi)(W
It
follows, from
Eq.
(9.25),
that if
a
is invariant, then
Vice
versa,
if the relation
holds, for any choice of
U,
i
and
T,
then
(i*
o
&)a
=
i*a
or, equiva-
lently,
cp:a
=
a.
We
can conclude that a necessary and sufficient condition for a differ-
ential Ic-form to be an absolute integral invariant is that
for any choice of
U,
i
and
r.
A
differential (k-1)-form
j3
E
A"-'(M),
on an n-dimensional manifold
M,
is
said to be
a
~~~t~ve
int~~~ invu~~~t
of the complete vector field
X,
if
dj3
is an absolute integral invariant; that
is,
if
0
A
revisiting
of
the ~~i~ton-~aco~i theory can be found
in
Ref,
149.
Another difficult task is to globalize the Liouville theorem. Undertaking
this task would also be useless, since
as
we shall
see
in the next section, it
has
already been acc~mplished,~~
lS5,
t3.

248
Symplectic
Manafolds
and
Hamiltonian Systems
9.6
The
Liouville
Theorem
Let
(M,
w)
be
a
2n-dimensional symplectic manifold on which
n
differentiable
functions are defined
fi:M+FR,
Vi=l,
...,
n,
Let
us suppose that the functions
fl,
.
.
.
,
fR
are in involut~on;
i.e.
{fi,fj}=O,
Vi,j=l,
...,
n, (9.26)
and that the
n
differential
1-forms
dfl,
.
.
.
,
dfm
are linearly independent at every
point
p
of the
level
set
Mf(%j
defined by
Mf(,,)
={p~M:fa(p)=ni,
i=l,
...,
n}.
&om the implicit functions theorem, the level set
Mf(=)
is
an
n-
Because
of
the isomorphism
(9.2),
with each differential l-form
dfd,
we can
dimensional submanifold of
M,
which
is
called the
level
~anifo~~
associate
a
vector field
Xf,
on
M
such that
ix,,
w
=
dfi
.
These vector fields
Xf,
,
which are supposed to be complete, are linearly inde-
pendent at every point of
Mf(z)
since the differentials
dfi,
. .
.
,
dfR
are linearly
independent and the symplectic form
w
is
not degenerate.
In
addition, by
Eq.
(9.26), the vector fields
Xfi
commute each other,
fXfi,Xfj]
=
0,
vi,j
=
1,.
.
*,
n.
Moreover, since
(Lx,,(p)fi)(~)
=
(ixfjdfii(p)
=
dfilp(Xfg(p))
=
{fi,fj)(P)
0,
the fields
Xf,,
. .
.
,
Xf,,
are tangent to
Mf(+
Thus, there exist n commuting tangent vector fields on
Mf(T)
that are
linearly independent at every point.
These vector fields form a local basis of an involutive distribution which,
by F'robenius' theorem, is completely integrable.
Moreover,
Mf(T)
is invariant with respect to each one of the
n
commuting
flows
ct
associated with the functions
fi.
It
can be proven that the differential manifold
Mf(=),
if compact and con-
nected, is diffeomorphic to an n-dimensional torus
T",
which admits the angles

The
Liouville
Theorem
249
'pl,.
.
.
,
cpn,
as
local coordinates, being
Tn
the product of
n
circles. Indeed, let
us observe that, by hypothesis, on
Mj(m)
there exist n functions
fi,
which
define an n-dimensional Abelian Lie algebra with the Poisson bracket
as
a Lie
bracket. They generate, at each point,
n
independent flows under which
Mf(?,)
is invariant. It follows that,
a priori,
Mf(T)
N
x
P,
but if
Mj(m)
is
compact, we can only have
k
=
n.
Under the action of the Hamiltonian flow, generated by
H
=
fl,
the angular
coordinates
'pa
will change according to
dpi
--=wi,
Vi=1,
...,
n,
dt
where
wi
=
wa(f1,.
.
.
,
fn),
so
that the motion on
Mf(m)
p*(t>
=
'pi(0)
+
wit,
Vi
=
I,.
.
.
,
n
(9.27)
is
almost
periodic.
Let
ua
consider a neighborhood
U
G
M
of
Mf(=).
If
we use the functions
fl,
.
. .
,
fn
as
coordinates in
U,
we can find a neighborhood
U'
C
U
c
M
of
Mf(*),
which is diffeomorphic to the direct product
T"
x
S",
where
S"
is a
sphere
of
an n-dimensional Euclidean space; i.e.
a
neighborhood
of
T
in
8".
The Hamiltonian flow, generated by
H
=
fl,
expressed in terms
of
coordi-
nates
(cp',
.
.
.
,
'p",
f1,.
.
.
,
fn)
becomes
(9.28)
The system
(9.28)
can be directly integrated to
fi(t)
=
f,(o),
(pi(t)
=
'pi(0)
+-
wi(fl(o),
.
. .
,
fn(0))t,
Vi
=
1,.
.
.
,n.
The integration
of
the original canonical system is, then, equivalent to
finding the angular variables
'p',
.
.
.
,
cpn.
This can be done by only using
quadratures.
What has been previously said concerning the compact case, can be sum-
marized by the following theorem.2
Theorem
31
(Liouville)
If
on the Bn-dimensional symplectic manifold
M
are
defined
n
functions
f1,.
.
.
,
fn
in
involution
{fi,fj}=O,
Vi,j=l,
...,
n,

250
Sympleetic
Manifolds and
Hamiltonian
Systems
and the
n
differential l-forms
dfl,
.
. .
,
df,& are linearly independent at every
point in the level manifold
Mf(?,)
=
{p
E.
M
:
fifp)
=
TIT^,
i
=
1,.
.
.
,n},
then
(a)
Mf(n)
is
an n-dimensional submanifold
of
M,
invariant
with
respect
(b)
if
compact and connected,
Mf(,)
is
d~ffeomo~hic to the n-di~~ns~o~al
(c)
the motion on
Mf(,l,
determined
by
the Hamiltonian
flow
generated
to the ~a~~ltonian flow g~nerated by
H
=
fl;
torus
T”,
with angular coordinates
(pl,
.
.
.
,
p”);
by
H,
is almost-periodic
(d)
the canonical equations with Hamilton function
H
can be integrated
by
pure quadratures.
Let us now observe that,
in
general, the coordinates
(fit..
.
,fa,
cpl,,
.
.
,
p”)
do
not form
a
symplectic coordinates system. However, there exist functions
Jh
=
Jh(f1,.
,.
,
fn),
\di
=
1,.
.
.
,n,
(9.29)
such that the coordinates
(J1,
. .
.
,
.In,
PI,.
.
.
,p”)
are symplectic; that
is,
such
that the original symplectic
form
w
can be expressed
as
w=dJhAdph.
The variables
(9.29),
which conjugate with the angles, are called
action vari-
ables;
they are first integrals of the Hamiitonian flow generated by
H.
In terms
of
these coordinates, the system
(9.28)
takes the form
d
Ji
dpi
-=O,
-=d(J1,...,Jn),
Vi=1,
...,
n.
(9.30)
dt
dt
9.6.1
An analysis for the construction of global action coordinates can be found in
Refs.
19
and
13
and a general analysis on the possibility
to
introduce “action-
angle type” coordinates can be found in
Refs.
158
and
41.
The
construction
of
the action-angle coordinates

The
Lioudle
Theorem
251
Let us consider the case in which the manifold
M
is a cotangent bundle,
Let
us
then consider the immersion
so
that
w
=
d$,
=
d(phdqh).
i
:
MI(,)
+
M
of the level manifold
Mf(,)
into
M
and the pull-back
i*w
to
Mf(,)
of
the
symplectic structure, Since
di'
=
i*d,
we
have
di'w
=
i'du
=
0.
Thus,
i'w
is a closed differential 2-form on the torus. It is not an exact differ-
ential form since the torus
is
not simply connected; that
is,
there exist curves
on the torus which cannot be contracted to a point. We have
i'w
=
i'dd,
=
di'19,.
On the other hand, the vector fields
efi
=
Xfi
are a basis for vector field,
which are tangent to
Mf(,),
so
that, for any two such fields
X
and
Y,
we may
write
X
=
Xiefi,
Y
=
Yiefi
I
It
thus follows that
(i*w)(X,
Y)
=
XiYj(i*w)(ef,,efi)
=
xiyj{ji,
fj}
=
0.
Therefore, over any bidimensional region
C
on the torus, we have
Since two homotopic curves
y1
and
72
on the torus
will
be the boundary of
a
two dimensional region
C,
we obtain
o=p=
J
i*?J,,
71U{-72)
by Stokes' theorem.
As
a consequence, we have

252
Symplectic
Manz-folds and Hamiltonian Systems
The curves on the torus
T"
can be divided in
n
equivalence classes
['yh],
each class containing noncontractible homotopic curves. The action variables
are then defined as follows:
where
h
=
1,2,.
.
.
,
n.
The construction can be finally completed
as
in Sec.
4.6.4.
9.7
A
New Characterization
of
Complete Integrability
In general, the peculiarities of
a
given dynamics
A
can be characterized by the
invariance of some geometric structure.
For
instance, the symplectic character
of
a
dynamics is characterized by the invariance of a symplectic structure. This
is the case
of
both Lagrangian and Hamiltonian dynamics.
It is then interesting to note the question whether the integrability prop-
erties of
a
dynamics can be characterized from this point
of
view.
As
we shall
see, this can be done.
Let
us
start with the following considerations.
Meaning
of
the vanishing Nijenhuis torsion
of
a
mixed tensor jield.
A
consequence of the vanishing Nijenhuis torsion
h/~,
of
a
mixed tensor field
T,
is that, given
a
vector field
Al,
the vector fields
of
the sequence
close on an Abelian Lie algebra
and that the transposed endomorphism
T
generates sequences
of
exact differ-
ential l-f~rms,~~ in the sense that
(da=O, dTa=O,
N;.=.O)+d(Fna)=O,
n>l,
a~7,*M.
Moreover, the invariance of
T,
under the flow generated by
a
vector field
A,
implies the invariance
of
the vector fields
TAn
and
of
the differential 1-forms
Pa.

A
New
Chamcterimtiora
of
Complete
Integrability
253
Propertiee
of
eigenvectors.
It
is
also interesting to analyze the properties
of
vector fields
which
are e~genvectors of
a
torsionless diagonal~zable mixed
tensor field.
Let
M
be
a
differen~iable
ani if old
and
T
a
d~a~onalizable mixed tensor
field; i.e.
pek
=
&ek,
rfgk
,
where
{ek)
is
fi
generic basis
of
7,M,
k
[ei,
ej]
=
cijek
3
and
{t9*}
its dual basis
of
TM,
&9&
=
--crs6
lk
T
A$"
*
2
We
recall that the N~jen~uis torsion
of
T
is
defined by
NT(%
xi
Y)
=
(a,
%T(X,
Y)>
t
with
?t!T(X,
Y)
=
(LrffxT)"Y
-
rf.(&XT)"Y
=
[PX,
W]
+
!P[X,
Y]
-
P[PX,
Y]
-
qx,
PY]
t
Let
us
evaluate
%T
on the
basis
{ek}:
'Hr(ei,
ej)
=
[Pq,
f'ej]
+
f"[ei,
e,]
-
?[f'ei,
ej]
-
p[ei,
Pej]
.
(9.31)
Since,
for any two differentiable functions
f
and
g,
and any
two
vector fields
X
and
Y
on
M,
we
may write
[fX,
9Y1
=
f$[X,
I"]
+
S(LYf)X
-
f(Lxg>Y
1
[Pei,fej]
=
[~iei,~jej]
=
~~,[ea,ej]
i-
Xj(L,Xi)ei
-
Ai(L,,Xj)ej,
II;[Pei,
ejl
=
P(Xd[ei,
ejl
+
(Leg
X,)ei)
=
XiP[ei,
ej]
i-
Xi(L,,Xi)ei,
II;[ei,fejl
=
P(Aj[ei,ej]
-
(~,*X~)e~)
=
XjQeiiejl-
~j(L~,Xj~e~.
and have
Thus, the relation
(9.31)
becomes
NT(ei,ej)
=
(P
-
~i)(f
-
~j)[ei,ej]
+
(Xj
-
Xi)[(Le,Xjjej
i-
(~~Ai)ei]
