Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.

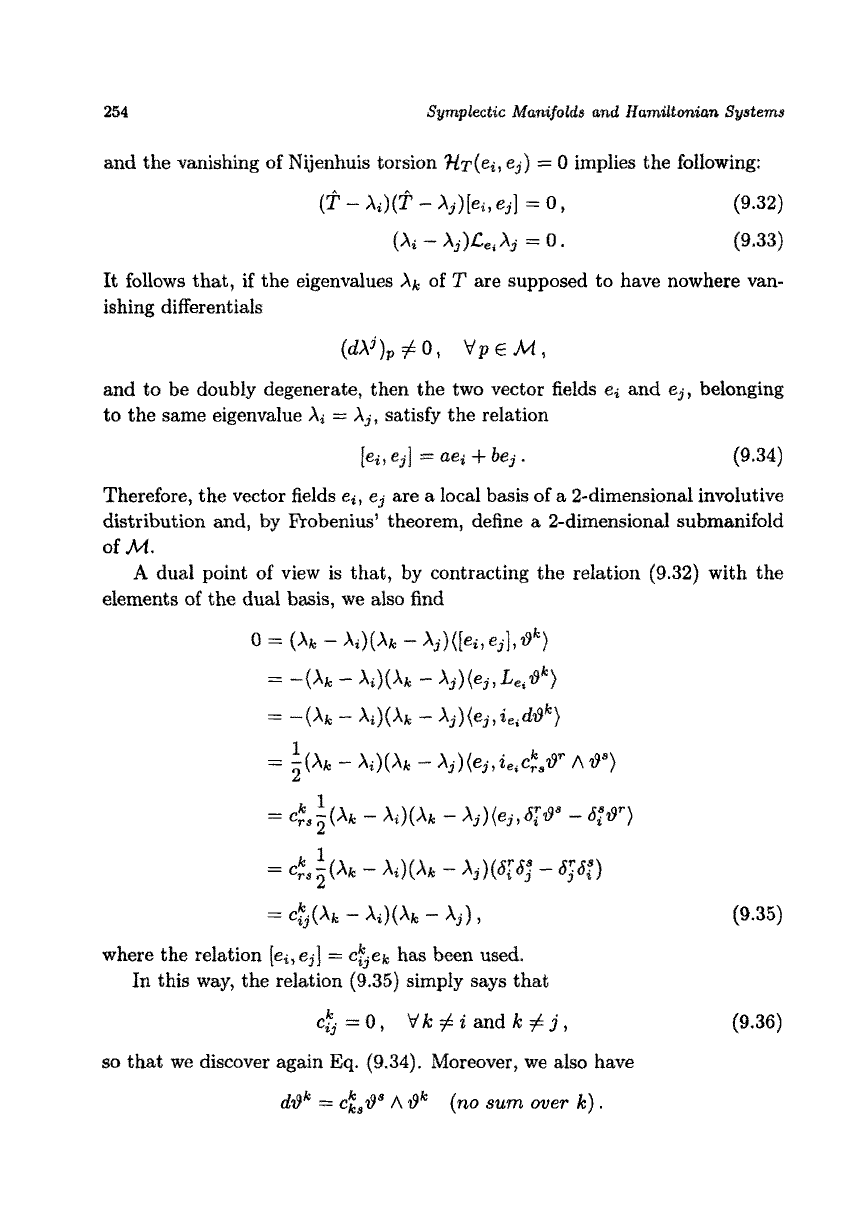
and the vanishing
of
Nijenhuis torsion
~~~e~,
ej)
=
0
implies the following:
(F
-
A,)(?
-
Xj)[ei,
ejl
=
0,
(9.32)
(A,
-
Aj)C,,Aj
=
0
*
(9.33)
It
follows that, if the eigenvalues
Xk
of
T
are supposed to have nowhere van-
ishing differentials
(dAj)p#O,
VPEM,
and to be doubly degenerate, then the two vector
fields
ei
and
ej,
belonging
to the same eigenvalue
Xi
=
Xj,
satisfy the relation
lei,
ej]
=
aei
+
bej
.
(9.34)
Therefore, the vector fields
ei,
ej
are a local basis
of
a
2-dimensional involutive
distributio~ and,
by
Frobenius' theorem, define
a
2-dime~sional sub~anifold
of
M.
A
dual point of view
is
that, by contracting the relation (9.32) with the
elements of the dual basis, we also find
1
=
C:~-(X~
-
X%)(X~
-
Xj)(ej,S[S'
-
S~V)
2
2
=
C,.,-((Xk
k1
-
Xi)(&
-
Aj)(S~S~
-
6;di)
=
C:j(Xk
-
&)(Xk
-
Xj)
,
where the relation
lei,
ej]
=
ckjeh
has been used.
In
this way, the relation (9.35) simply says that
cij=O,
k
Qk#iandk#j,
so
that we discover again
Eq.
(9.34). Moreover, we also have
dt?k
=
cEs@'
A
19k
(no
sum
over
k)
.
(9.35)
(9.36)

A
New Chamcterization
of
Complete Integrability
255
The last relation implies that
dk Addk
=0,
which again, by F'robenius' theorem (in the dual form), ensures the holonomic-
ity of the basis.
In conclusion, the relations
(9.32)
or
(9.35),
which directly follows from the
Nijenhuis condition, ensure the holonomicity
of
the basis
{ek},
in which the
tensor
field
T
is
diagonal,
Invariance
of
the eigenvalues
of
an invariant mixed tensor field.
It
is easy to check that the invariance
of
T,
under the flow generated by a vector
field A, implies the invariance of its eigenvalues
A.
Indeed, let
V
E
7,M
and
a
E
TM
be eigenvectors
of
T
and
Tl
respec-
t ivel
y,
PV=XV,
Tff=Xff,
belonging to the same eigenvalue
A,
such that
iva
#
0.
If
T
is supposed to be A-invariant, we have
La(TV)
=
(LaT)*V
+
T(LAV)
=
T(LaV)
V
E
7,M,
(9.38)
so
that
!fv
=
Xv
P(LAv)
=
LA(PV)
=
(LAX)V
+
X(LAv),
and
Then, from
we
finally have
(LaX)(V,
a)
=
0
+
LAX
=
0
;
that is, we obtain the invariance of
X
under the flow generated by A.
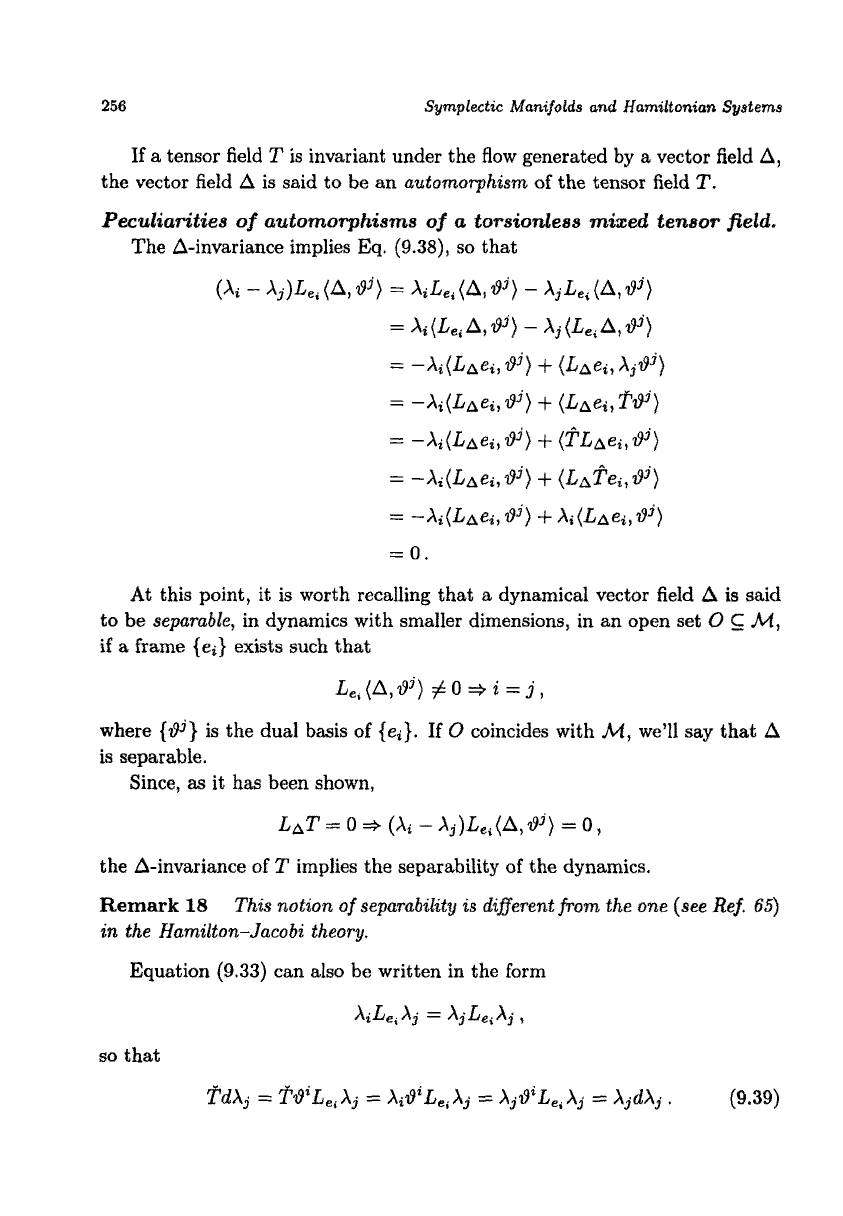
256
Symplectic Manijotds and Hamiltoprian
Systems
If
a tensor field
T
is
invariant under the flow generated by a vector
field
A,
the vector field
A
is
said to be an
autornorphism
of
the tensor fieid
T.
P~~l~a~t~e~
of
~~torn~~h~s~s
of
a
~o~s~a~ess
mixed
teraeor
field.
The A-invariance implies
Eq.
(9.38),
so
that
(Xi
-
X.j)Lei(A,d’)
=
XiLe,(A,d)
-
XjLei(A18j)
=
Xi(LeiA’dj)
-
Xj(LqA+t9’)
=
-Xi(LAei,
?!q
+
(LAei,
Xjt9’>
=
-Ai(Laei,
I9j)
+
(LAei,
TIP)
=
-Xi(Laei,
8’)
+
(PLAei,
d)
=
-Xi(LAei’
@’}
+
(LaPeg,
d’}
=
-&(LAe$,
8’)
+
,&(LAe$,
83)
=
0.
At this point, it
is
worth recalling that
a
dynamical vector field
A
is
said
to be
se~Tu~le’
in dynamics with smaller dimensions, in an
open
set
0
I:
M,
if
a
frame
{ei}
exists such that
Lei (A,
19’)
#
0
=t
i
=
j
,
where
{&}
is
the dual basis
of
(ei).
If
0
coincides with
M,
we’ll say that
A
is
separable.
Since,
~1s
it has been shown,
LAT
=
0
+
(Xi
-
Xj)L,,(A,8’)
=
0,
the A-invariance
of
T
implies the separability
of
the dynamics.
Remark
18
in
the Hamilton-Jacobi theory.
This not~on
of
sepaTu~~~~~~
is
~i~e~e~~
from
the one (see Ref.
65)
Equation
(9.33)
can also be written in the form
XILe,Xj
=
XjL,,Xj,
TdXj
=
PdiLeiXj
=
XiSiLeiXj
=
XjdiLeiXj
=
XjdXj,
so
that
(9.39)
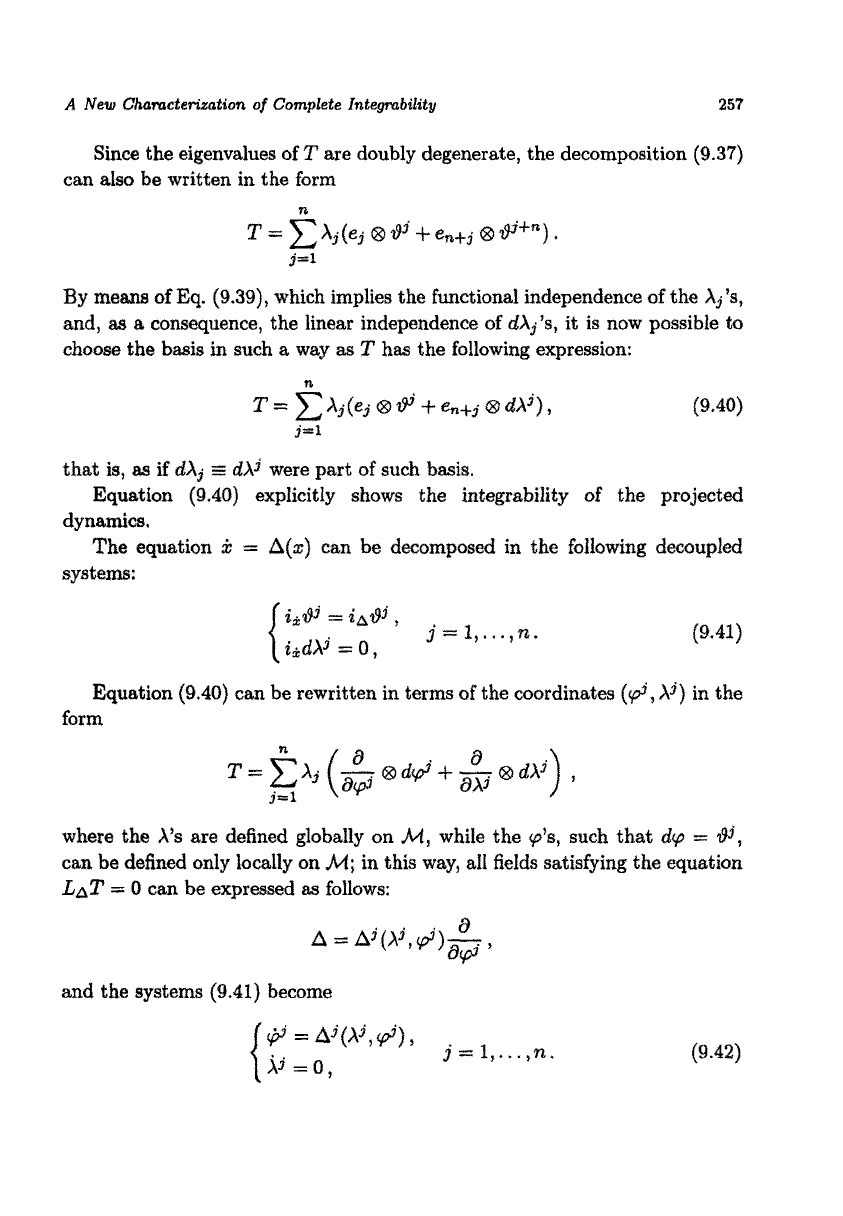
A
New Chamcterization
of
Complete Integmbility
257
Since the eigenvalues of
T
are
doubly degenerate, the decomposition
(9.37)
can
also
be written in the form
n
T
CAj(ej
Q@
+
e,+j
Q
&+”).
j=1
By meam
of
Eq.
(9,39),
which implies the functional independence of the
Aj’s,
and,
as
a
consequence, the Iinear independence
of
dAj’s,
it
is
now possible to
choose the basis
in
such
a
way
as
T
has the following expression:
n
T
=
C
~j
(ej
QP
4
+
ea+j
t~3
d~j)
,
(9.40)
j=1
that
is,
as
if
dAj
dynamics,
systems:
dAj
were part of
such
basis.
Equation
~9.40~
expIicit~y shows the ~tegr~bi~ity of the projected
The
equation
&
=
A(s)
can be decomposed in the foIiow~ng
d~~u~Ied
(9.41)
Equation
(9.40)
can be rewritten in terms
of
the coordinates
((pi,
Aj)
in the
form
where the
A’s
are defined globally on
M,
while the
p’s,
such that
dp
=
@,
can be defined only locally on
M;
in this way, all fields satisfying the equation
LAT
=
O
can be expressed
as
follows:
a
and the systems
(9.41)
become
(9.42)

258
Sgmplectac
Mun~~o~ds
and
H~m~~~on~an
Systems
It is easy to check that the separable and integrable vector field
A
is also
In fact, given
A,
we can build many invariant symplectic structures
w
a
H~iltonian vector field.
w
=
fk(Ak,
~k)d~k
A
dXk
,
(9.43)
k
where
fk
are arbitrary functions required to ensure the invariance
of
the
differ-
ential 2-form
(Eq.
(9.43)).
If
we suppose that the field
A
has not got singular
points, the generic symplectic structure
w
will have the form
Choosing,
as
a
basis,
the one associated to the action-angle variables
(Jk,
qk),
the tensor field
T
becomes
and
w
takes the following form:
k
What has been said in the present section can be summarized
as
follow^.^^^^^
Theorem
32
(DMSV)
Let
A
be a dynamical vector field on a manifold
M
which admits a diagonalizable mixed tensor field
T
which
is inuariant
LaT=0,
has a vanishing Nijenhuis torsion
N-=0,
a
has doubly degenerate eigenvalues
Xj
with nowhere vanishing difleren-
tials
deg
X3
=
2
~
(dXj),
f
0,
Vp
E
M
.
Then, the vector
field
A
is
separable, completely antegrable and Hamiltmian.

A
New
Chamcterization
of
Complete Integmbihty
259
Remark
19
The conditions
LAT
=
0
and
NT
=
0
and the bidimension-
a~ity
of
the eigenspaces
of
T
was extracted
jkm
the e~tence
of
dynamics
with infinitely many degrees
of
freedom, admitting a
Lax
representation (see
Part
IV).
The fact that nonlinear fi~ld theories, inte~ble
~th
the inverse
scattering method show an endomorphism, invariant under the dynamics, with
van~h~~g ~~e~hu~s
tor~~on
and b~dime~iona~ inv~~ant eigenspa~s, s~ggested
that the analysis
of
the integrability
of
dynamical systems could be realized,
instead that
in
terms
of
a mixed tensor field
T,
rather than s~p~ectic struc-
ture
w.
The integrabi$i~y conditions
in
terms
of
symplectic structures
w
strictly de-
pend on the finite dimensionality
of
the space and cannot easily be extended to
the infinite-dimensional case. On the contrary, the integrability
in
terms
of
T
is expressed by conditions which do not depend on the finite number
of
degrees
of fieedom
of
the
dynamical system
A.
Remark
20
It
is worth remarking that the vector field
A
is
not taken to be
a
priori
a
~~mi~tonian vector field.
As
we shall see
in
Part IV, integ~bi~ity
of
dissipative dynamics can be put
in
the same setting
by
assuming diflerent
spectral hy~thesis for the tensor field
T,
9.7.1
EFom
the
L~o~v~~le
~~t~~rn~~~~~~
to
~n~~~~nt
mixed
tensor
fields
Let
us
now study the problem
of
constructing invariant mixed tensor fields,
with the appropriate properties (also called a
recursion operator),
for a given
Liouville’s
integrable Hamiltonian dynamics
A.
If
H
is
the Hamiltonian func-
tion
and
{a,
.}
is the Poisson bracket, we have
Let
u8
introduce in some neighborhood
of
a Liouville’s torus
Tn
action-
We
have
angle variables
(J1,
.
.
.
,
Jn,
p’,
.
.
.
,
cp”).
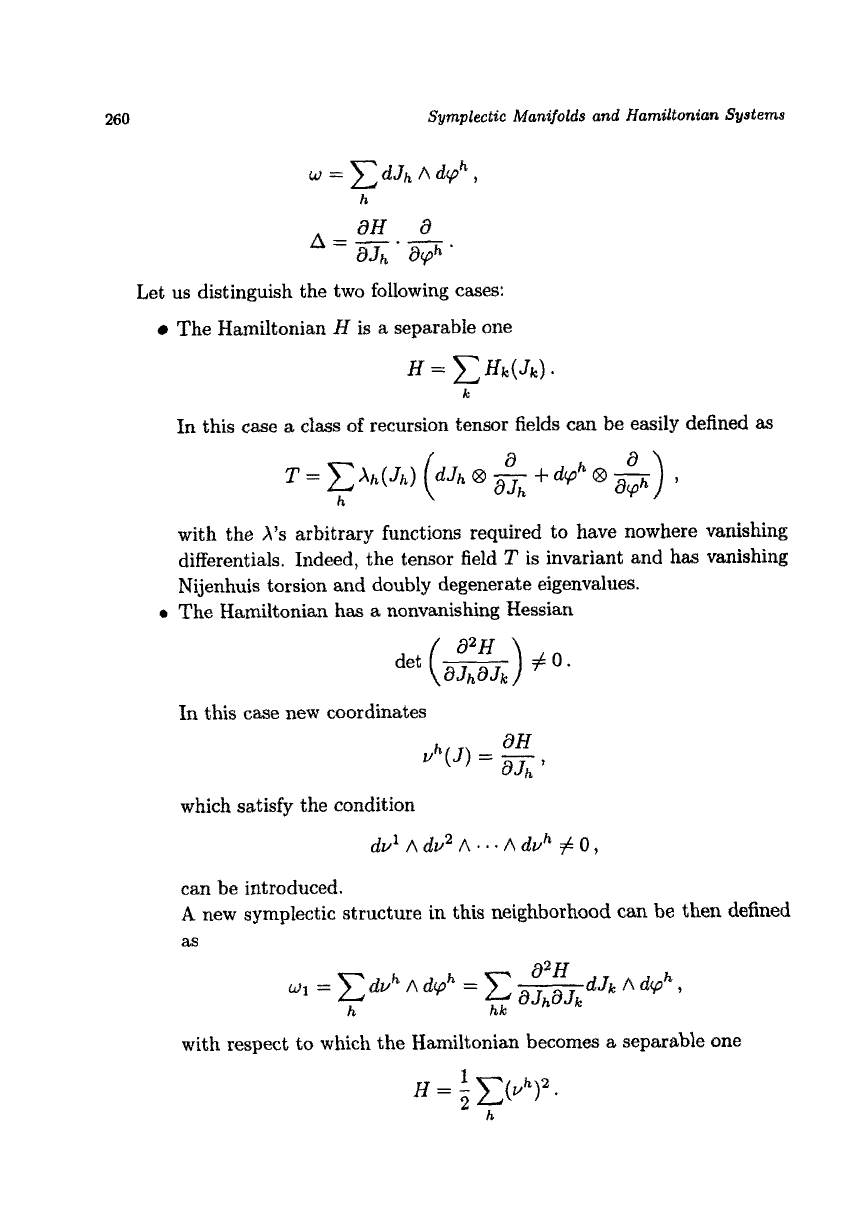
260
Symplectic Manifolds and Hamiltonian Systems
h
aH
a
A=-.-
aJh
8vh'
Let us distinguish the two following cases:
The ~~iltonian
H
is
a separabIe one
k
In
this case
a
class
of
recursion tensor fields can
be
easily defined
as
with the
A's
arbitrary functions required to have nowhere vanishing
differentials. Indeed, the tensor field
IT
is
invariant and
hap;
vanishing
Nijenhuis torsion and doubly degenerate eigenvalues.
0
The Ha~i~tonian has
a
nonvanishing Hessian
In this
case
new
coordinates
which satisfy the condition
du'
A
du2
A
+
Aduh
#
0,
can be introduced.
A
new sympbctic structure
in
this neighborhood can be then defined
as
with respect
to
which the Hamiltonian becomes
a
separable one
Applicntiona
261
The class of recursion tensor fields is then given by
By means of this construction, it is possible to find the second symplectic
structure
for
a completely integrable Hamiltonian system.
9.8
Applications
9.8.1
An invariant mixed tensor field, with vanishing Nijenhuis tensor and dou-
bly degenerate eigenvalues, can be easily constructed,86 for the Lagrange-
Poisson gyroscope dynamics, without gravity for the sake of simplicity, by
using the constants of the motion found by Mishenko, Dikii, Manakov, and
A
Recursion
operator
for
the
rigid
body dynamics
&tiu.
154,84,141,165
The Hamiltonian function for the rigid body is locally given by
+%)
,
1
(pfi
cos
cp
+
c
sin
(P)~
(pa
sin
cp
-
c
cos
cp)'
l3
+
H=-(
2
A
where
29,
cp
and
II,
are the Euler angles (of the body principal axes frame
Oxyz
with respect to a generic fixed frame
Otqr),
pol
p,
and
p+
their conjugate
variables, and
A,
l3
and
C
the components of the inertial tensor with respect
to
Oxcya,
and
p$
-
p,
sin
29
sin
29
U=
When
A
=
B
the Hamiltonian
H
reduces to
and the rigid body is said to possess
a
gyroscopic structure
(Lagrange-Poisson
gyroscope).
Its complete integrability is obviously granted by the Liouville
theorem in the open submanifold where
H,
p,
and
p+
are independent.
262
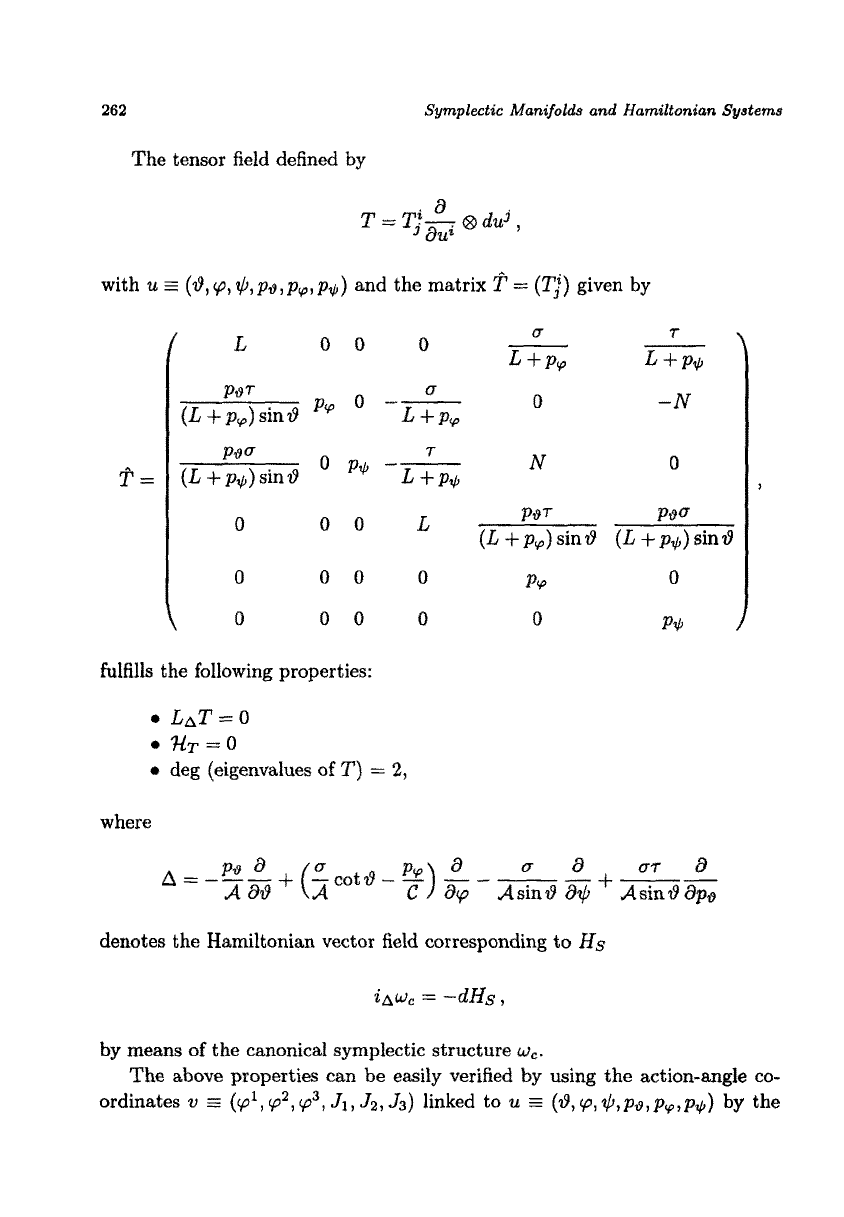
The tensor field defined by
Symplectic
Manifolds and Hamiltonian
Systems
with
u
(d,y?,$,p8,p,,p*)
and the matrix
f'=
(Tj)
given by
T=
LT
7-
L
00
0
L
+P,
L+P$
-N
0
00
0
00
0
00
PV
0
0
P@
,
fulfills
the following properties:
b
deg (eigenvalues of
T)
=
2,
where
denotes the Hamiltonian vector field corresponding to
Hs
~AW~
=
-dHs,
by means
of
the canonical symplectic structure
wC.
The above properties can be easily verified by using the action-angle co-
ordinates
ZI
=
('p','p2,'p3,
J1,
Jz,.Js)
linked to
u
=
(d,yr,$,pe,p,,p+)
by
the
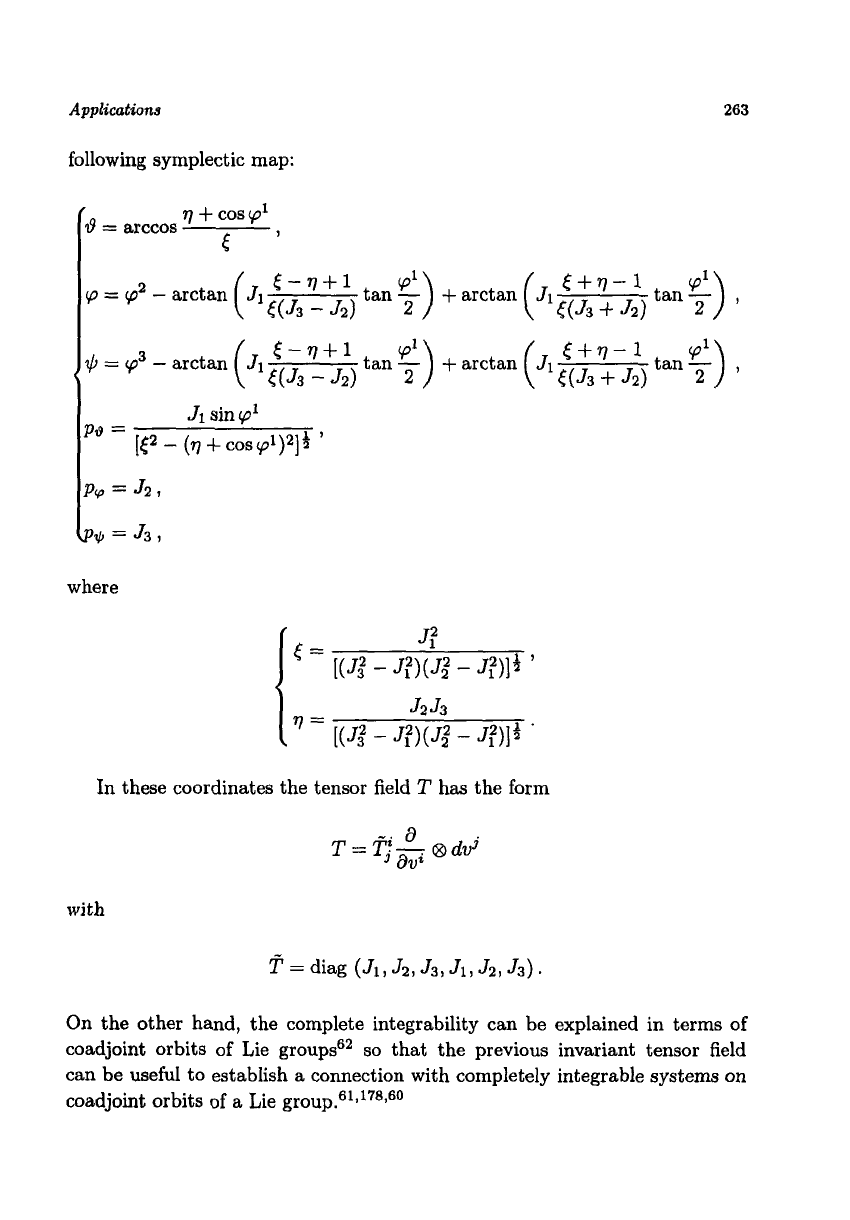
Applications
/
q
+
cos 'PI
E'
19
=
arccos
cp
=
p2
-
arctan
+
=
p3
-
arctan
J+Q-~
tan:),
J(
J3
+
J2)
J1
sin
cp'
Pe
=
[p
-
(7)
+
COSyJ1)2]*
'
Pv
=
J27
$11
=
J3
263
following symplectic map:
where
J?
[(J:
-
J?)(Ji
-
J,")]i
'
[(J:
-
J?)(Ji
-
J?)]i
{
1:
J2J3
In these coordinates the tensor field
T
has
the form
with
=
diag
(JI,
52,
J3,
J1,
J2,
J3).
On
the other hand, the complete integrability can be explained in terms
of
coadjoint orbits
of
Lie groups62
so
that the previous invariant tensor field
can be useful to establish a connection with completely integrable systems on
coadjoint orbits of a Lie gro~p.~~1~~~~~~
