Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


284
The
Orbit8
Method
and the sequence
(gn)nf~
converges to a point
of
G,.
The orbit of the point
a,(la)
of
J-I(p)
under the action of the group
G,
is
the set
G,
1
a,(h)
=
(Lg-,a,(h)
:
g
€
G,}
.
(10.28)
Thus, we have shown that
(a)
p
is
a regular value of
J;
(b)
G,
acts properly on
J-'(p).
From what
has
been said in the previous section, conditions
(a)
and (b)
are
suffici~nt to affirm that the set
J-I(p)/G,,
that
is
the set of the orbits of
the points of
J-'(p)
under the action
of
G,,
is
a symplectic manifold. This
manifold
is
the reduced phase space and,
of
course, can be identified with the
orbit
of
fi
under the coadjoint action of the
group
G,
that
is
G*p
=Z
{Adi-ip:
g
E
G).
Indeed, from
Eq.
(10.26),
the action
of
G,
on the points
of
J-I{p)
reduces
to the left translation
of
the points on the base. Therefore, with every orbit
G,
a,(h)
of
a
point in
J-I{p)
under the action
of
the group
G,,
we can
associate the orbit
G,
.
h
of
the point
h
in
G
under the action of
G,,
G,*a,(h)
4Gp.h.
In this
way
the reduced phase space
is
diffeomorphic to
GIG,,
so
that
(10.29)
To
every orbit
G,
.
h
of
GIG,
we can associate
a
point
of
the orbit of
p
in
g8
under the
coadjoint
representation
G,*h+Ad;l-ip,
so
that
(10.30)
and then

Orbita
of
a
Lie
Gmup
an the Coadjoint Representation
285
Thus, by
Eqs.
(10.29)
and
(10.30),
the reduced phases space J-l(p)/Gp
can be identified with the orbit G
-
p
under the coadjoint action. Hence, the
orbit
of
p
under the coadjoint representation is a symplectic manifold.
Now
let
us
find the expression of the symplectic form
0,
on the orbit
G.
p;
for
this purpose let
us
introduce the map
C*0,
=
W.
Since
where
ap(etEg)
is a curve in
J-'(p)
through
ap(g),
so
that
(ap)*g((Rg)*e(t))
E
Zp(g)J-l(pL)
i
Vt
E
-
Vice
versa,
if
V
E
7a,(g)J-1(p),
its integral curve
is
a,(a(t))
with
a(0)
=
g.
Furthermore
,
a(t)
=
a(t)g-'g
=
7(t)g
=
Rg7(t)
,
where
~(t)
is
a
curve in
G
passing on
e
at
t
=
0,
so
that
(
10.3
1)
and where

286
The
Orbits
Method
we have
where
Ad*
is the coadjoint action; i.e.
Ad*(g,p)
=
Adi-,p.
Orbits
of
a
Lie
Gmup
in
the
Goadjoint
Representation
287
d d
=
--Ad*(etcg, dt
p)
=
-Ad;-,Ad;Ad*(ettg, dt
p)
d
dt
=
-Adl-lAd*(g-letfg,
p)l
t=O
d
<p(p)
=
-Ad*(etc,p
dt
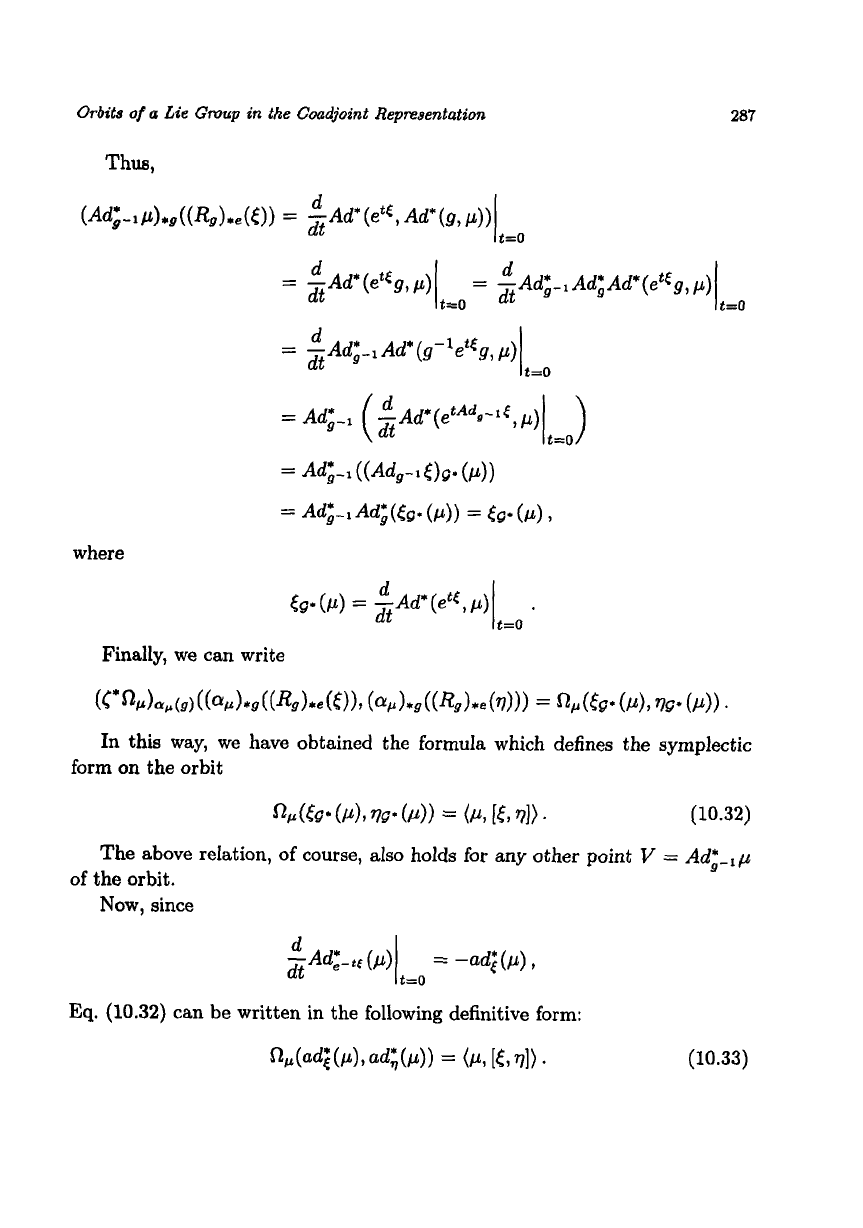
Finally, we can write
In this way, we have obtained the formula which
defines
the
symplectic
form
on
the orbit
The
above relation,
of
course, also holds for
any
other point
V
=
Ad,*-,p
Now, since
of the orbit.
Eq.
(10.32)
can be written in the following definitive form:
Qcl(ad&4, ad;(P))
=
(P,
[&
111)
*
(10.33)

288
The
Orbits
Method
10.3
The
Rigid
Body
In this section, we analyze the rigid body motion about a fixed point, in the ab-
sence of external forces. The rigid body represents a simple example
of
Hamil-
tonirtn system, whose configurations space
is
a
Lie group. We shall see how, on
every orbit of the coadjoint representation, the Euler equation is Hamiltonian,
the Hamilton function being given by the kinetic energy.
A
rigid body
is
a
system
of
particles subject to the holonomic constraint
defined by the condition that the distance between any two points of the system
is
constant. The configuration
space
of
a
rigid body
is
the six-dimensional
manifold
R3
x
S0(3),
where
SO(3)
is
the group
of
the orthogonal matrixes
3
x
3,
if
in the considered rigid body there are at
least
three not-aligned points.
Let us consider the problem of determining the motion
of
a free rigid body.
This system is invariant under translations and thus there exist three first
integrals which are the three components of the total
moment^.
Therefore
the motion of the centre of mass
is
a free motion and we can thus choose
an
inertial system in which the centre of mass is at rest. In this frame a free rigid
body rotates about its inertial centre
as
if
it
were bound
to
a
fixed point. Thus
the problem of the free motion of a rigid body is equivalent to the problem
of the rigid body motion about
a
fixed point with three degrees
of
freedom.
The configurations space is simply
SO(3)
and the position and the velocity of
the body are defined by
a
point of the tangent bundle
TSO(3).
The system
is
invariant under rotations about the fixed point and, by Noether’s theorem,
there exist three corresponding first integrals which are the three components
J,,
Jar
and
J,
of the angular momentum. Besides these three integrals there
is
the total energy of the system,
E,
which has onfy the kinetic part.
The
four
first integrals,
J,,
.Iv,
J,
and
E,
are functions defined on the tangent bundle
rsop).
We can define an action
of
SO(3)
on itself with the left translations
L,
:
h
E
SO(3)
+
L,(h)
=
gh
E
S0(3),
where
gh
denotes the matrix product.
The tangent bundle
TSO(3)
is isomorphic to
SO(3)
x
7,S0(3),
xSO(3)
denoting the tangent space to
SO(3)
at
the identity
e;
i.e, the space
of
3
x
3
antisymmetric matrices.
The
Rigid
Body
289
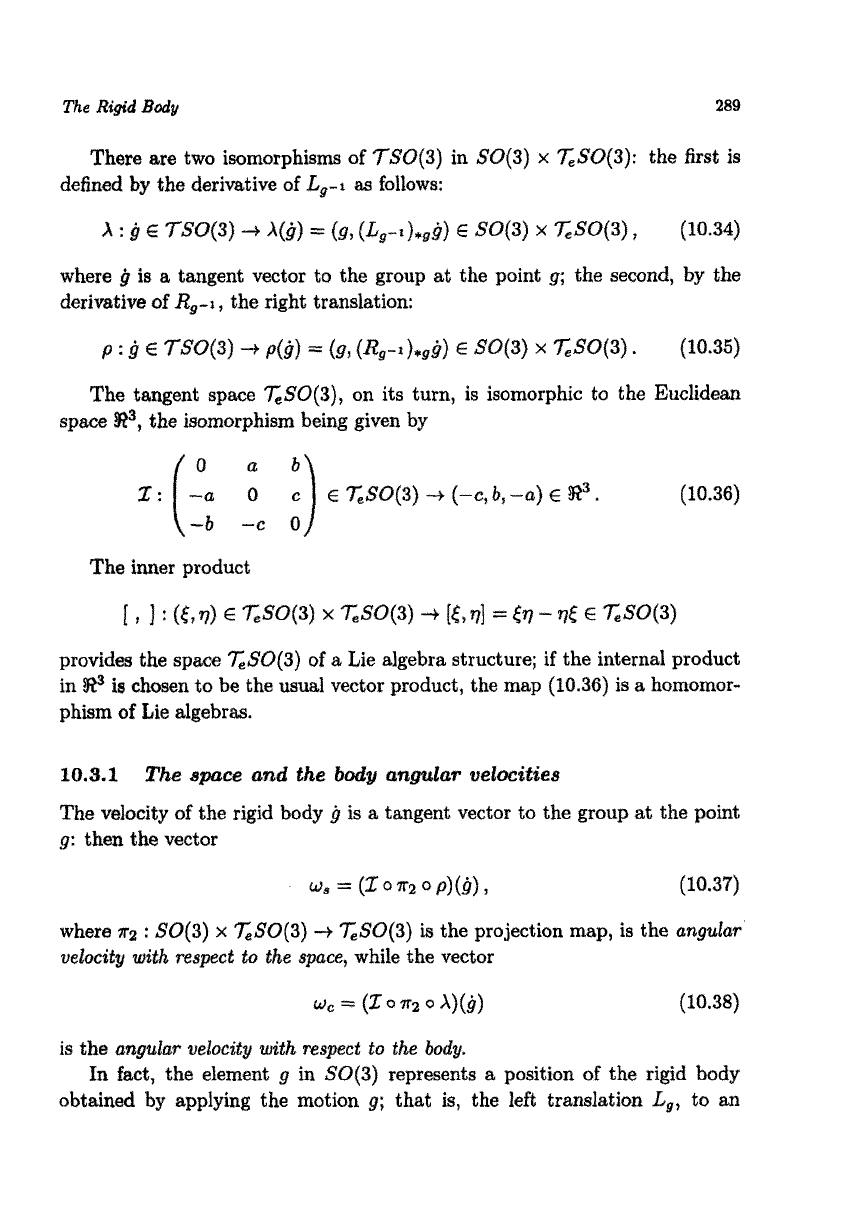
There are two isomorphisms
of
TSO(3)
in
SU(3)
x
730(3):
the
first
is
defined by the derivative
of
L,-i
as
foflows:
X
:
9
E
TSO(3)
-+
A(&)
=
(g,
(L,--i)*,&)
E
SU(3)
x
7,S0(3),
(10.34)
where
g
is
a
tangent vector to the group at the point
g;
the second, by the
deri~tjve
of
Rg-l,
the right trans~ation:
The tangent space
7',S0(3),
on its turn, is isomorphic to the Euclidean
space
r#3,
the jsom~rphism being given by
z:
(-",
:
")
E
TeSU(3)
t
(-c,b,-a)
E
R3*
(10.36)
-b
-c
0
The inner product
provides the space
XSO(3)
of
a
Lie algebra structure; if the internal product
in
is
chosen to be the
usual
vector product, the map
(10.36)
is
a
homomor-
phism of Lie algebras.
10.3.1
The
velocity
of
the rigid body
g
is
a
tangent vector to the group
at
the point
g:
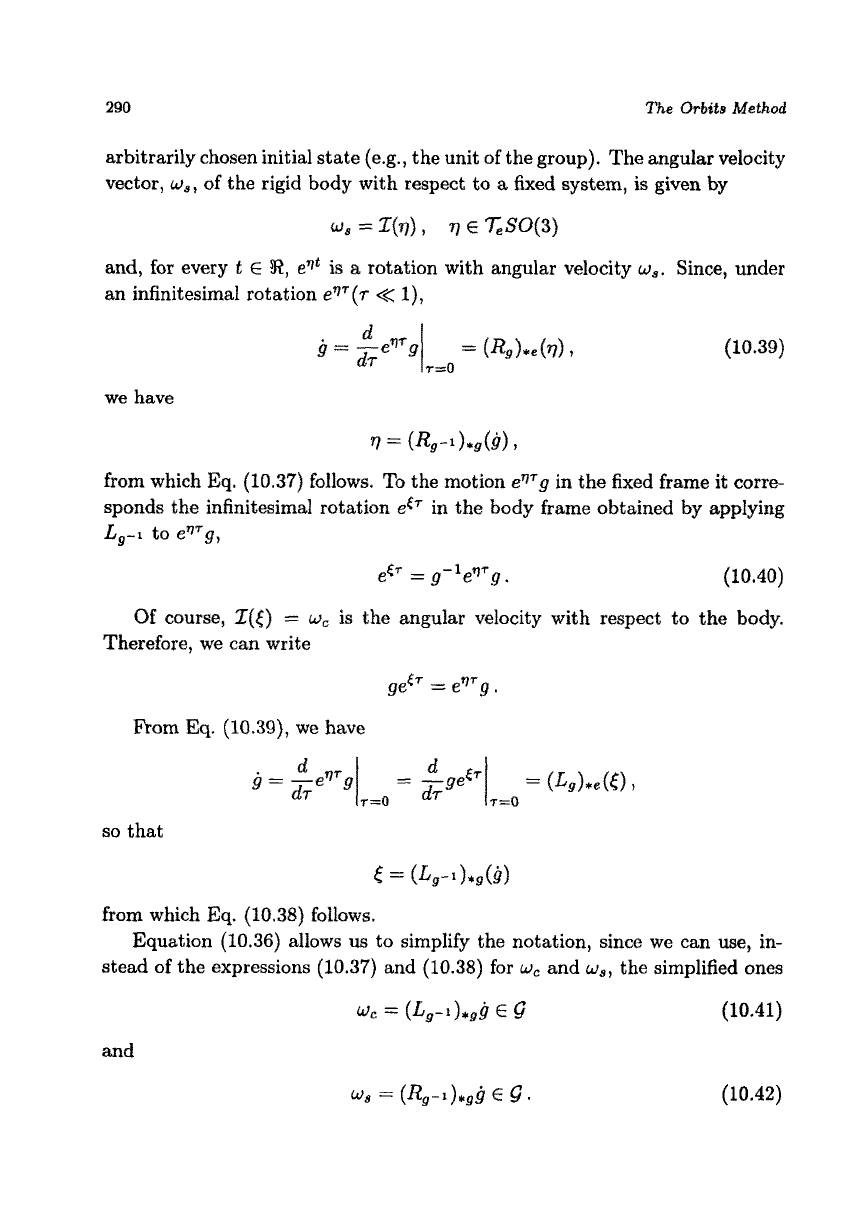
then the vector
The
spce
and
the
bad@
aPtgular
welocities
Ws
Z=
(207b
QP>(&),
(10.33)
where
7r2
:
SO(3)
x
ZSO(3)
-+
ZSO(3)
is the projection map, is the
angular
v~~oci~~ with respect to
the
spuce,
while the vector
is
the
apbgzllar
velocitg with respect
to
the
body.
In
fact, the e~ement
g
in
SU(3)
represents
a
position
of
the rigid body
obtained
by
applying the motion
g;
that
is,
the left translation
L,,
to an
290
The
Orbits
Method
arbitrarily chosen initial state (e.g., the unit of the group). The angular velocity
vector,
w3,
of the rigid body with respect to
a
fixed system,
is
given by
w3
=
qrl),
9
E
ZSO(3)
and,
for
every
t
E
3,
eqt
is a rotation with angular velocity
w3.
Since, under
an infinitesimal rotation
eq7(T
<<
l),
(10.39)
we
have
77
=
(Rg-l)*g(i)
1
from which Eq.
(10.37)
foilows. To the motion
eq7g
in Le fixed frame it corre-
sponds the infinitesimal rotation
efT
in the body frame obtained by applying
L,-I
to
eq7g,
,ET
=
9
-1
e
r1T
9.
(10.40)
Of course,
X(()
=
w,
is
the angular velocity with respect
to
the body.
Therefore, we can write
gecT
=
eqTg.
From
Eq.
(10.39),
we
have
so
that
t
=
Gg-1
)*g(Q)
from which
Eq.
(10.38)
follows.
Equation
(10.36)
allows us to simplify the notation, since we can use, in-
stead
of
the expressions
(10.37)
and
(10.38)
for
wc
and
w3,
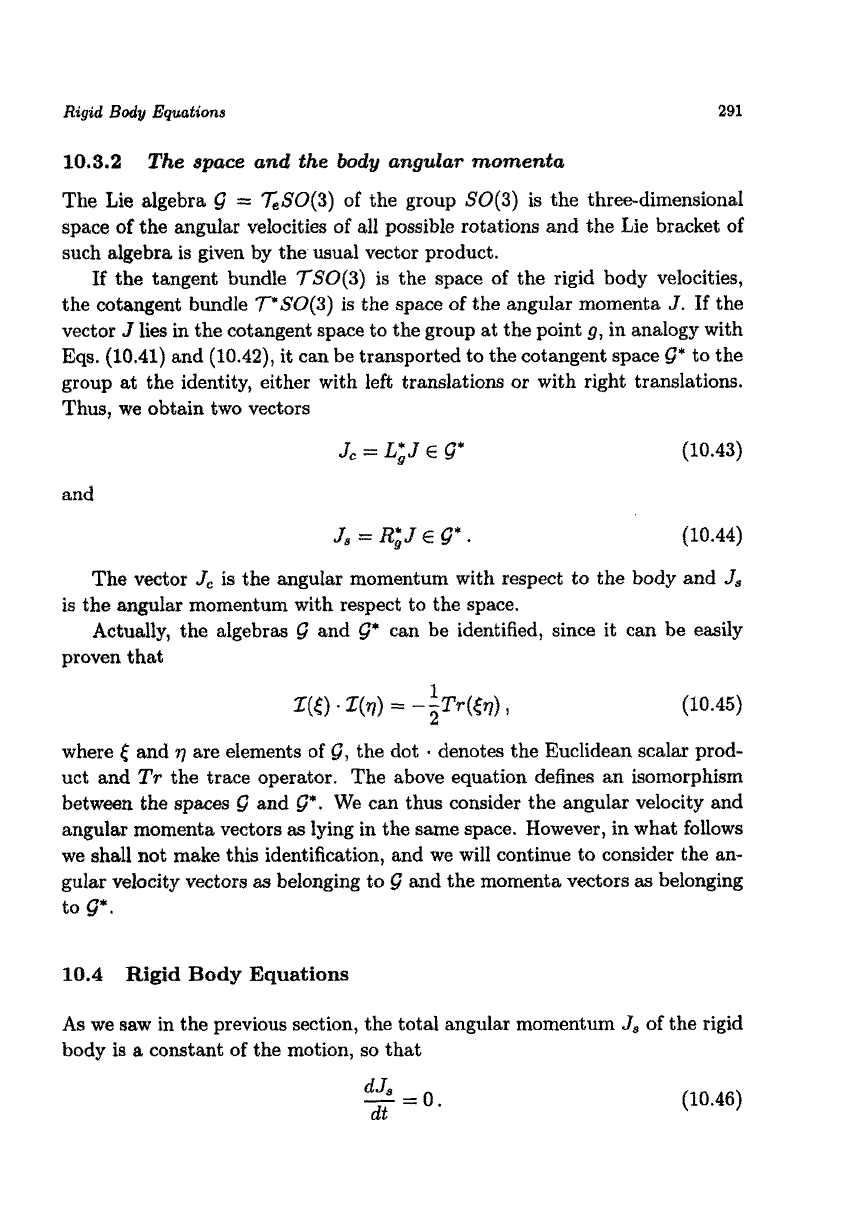
the simplified ones
wc
=
(Lg-1)*g9
E
6
(10.41)
and
w*
=
(Rg-l)*gb
E
0'
(10.42)
Rigid
Body
Equations
291
10.3.2
The Lie algebra
B
=
7eS0(3)
of the group
SO(3)
is the three-dimensional
space
of
the angular vebcities of
a11
possible rotations and the Lie bracket of
such algebra
is
given by the usual vector product.
If
the tangent bundle
7S0(3)
is
the space
of
the rigid body velocities,
the cotangent bundle
'PSO(3)
is
the space
of
the angular momenta
J.
If the
vector
J
lies
in
the cotangent space to the group at the point
9,
in analogy with
Eqs.
(10.41)
and
(10.42),
it can be transported to the cotangent space
G*
to the
group at the identity, either with left translations
or
with right translations.
Thus, we obtain two vectors
The
space
and
the
bod@
an~~~a~ ~~~ent~
J,
=
L:J
E
Q*
(10.43)
and
J,
=
RiJ
E
g*
-
(10.44)
The vector
J,
is
the angular momentum with respect to the body and
J,
Actually, the algebras
9
and
I?'
can be identified, since it can be easily
is
the angular momentum with respect to the space.
proven that
where
6
and
rl
are elements
of
B,
the dot denotes the Euclidean scalar prod-
uct
and
Tr
the trace operator. The above equation defines an isomorphism
between the spaces
Q
and
Q*.
We can thus consider the angular velocity and
angulas momenta vectors
as
lying in the same space. However, in what follows
we
shall not make
this
identification, and we will continue to consider the an-
gutar velocity vectors
as
belo~ging to
B
and
the momenta vectors
as
belonging
to
g*.
10.4
Rigid Body Equations
As
we
saw
in the previous section, the total angular momentum
J,
of the rigid
body
is
a
constant of the motion,
so
that
d
Js
-
=o.
dt
(10.46)
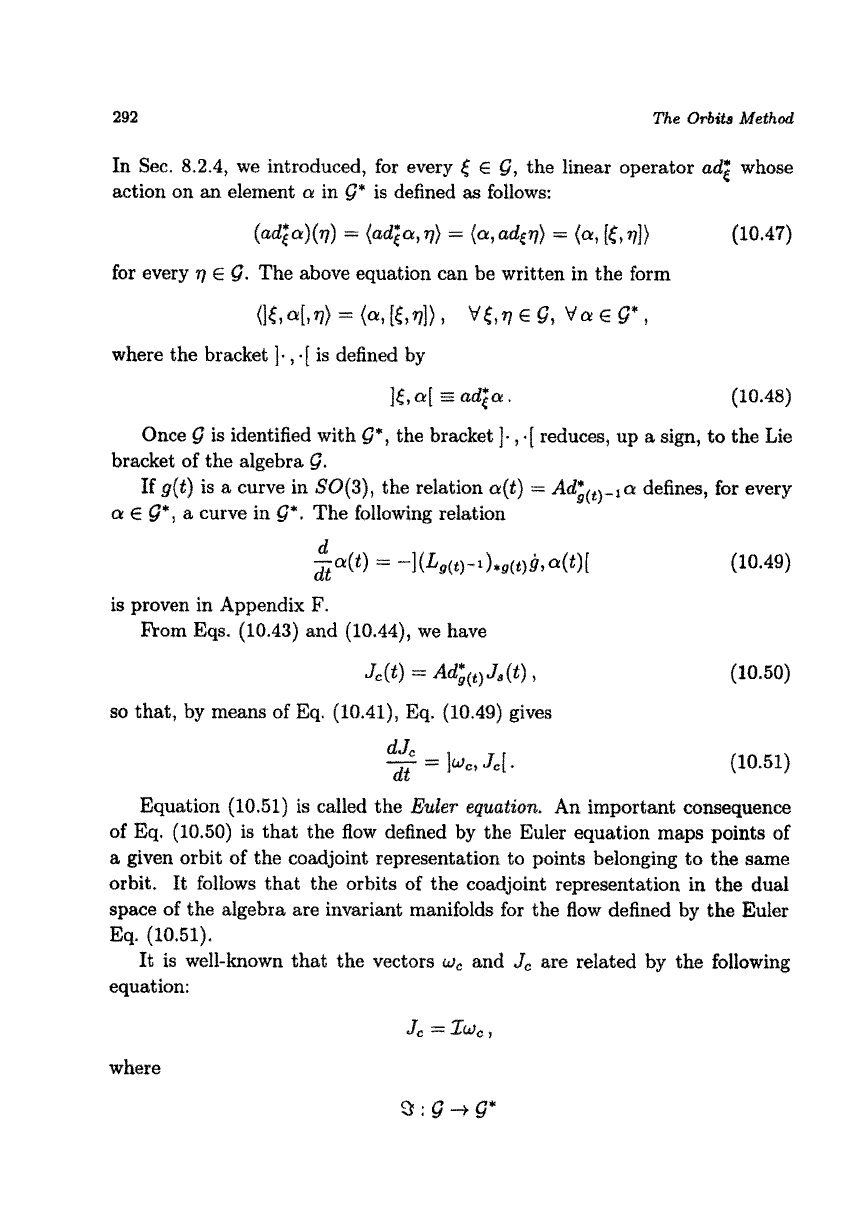
292
The
Orbits
Method
In Sec,
8.2.4,
we introduced, for every
6
E
Q,
the linear operator
adz
whose
action on
an
element
a!
in
Q*
is defined
as
follows:
(ud~a)(77)
=
(ad+,
rt)
=
(a,
Ud~77)
=
(a,
ft)
rtl)
(10.47)
for every
71
E
Q.
The above equation can be written in the form
(It1
41
77)
=
(a,
!&
rtl)
I
vt,
77
6,
v
E
8*
I
where the bracket
1.
,
.[
is defined by
14,
a[
=
adza.
(10.48)
Once
8
is identified with
Q*,
the bracket
f-
,
a[
reduces, up
a
sign, to the Lie
If
g(t)
is
a
curve in
S0(3),
the relation
a(t)
=
Adift)-la
defines, for every
bracket
of
the algebra
8.
a
E
Q*,
a
curve in
G*.
The following relation
(10.49)
d
is
proven in Appendix
F.
-4t)
dt
=
-l(~~(t)-l)*~(~)g,a(t~[
&om Eqs.
(10.43)
and
(10.44))
we have
Jc(t)
=
Adi~t~J~(t)
so
that, by means of Eq.
~10.41~,
Eq.
(10.49)
gives
dJ,
-
=
1WC)
JCI
dt
(1 0.50)
(10.51)
Equation
(10.51)
is
called the
EuEer equation.
An important ~~q~en~
of
Eq,
(10.50)
is
that the flow defined by the Euler equation maps points of
a
given orbit of the coadjoint representation to points belonging to the same
orbit. It follows that the orbits
of
the coadjoint representation in the dual
space
of
the algebra are invariant manifolds for the flow defined by the Euler
Eq.
(10.51).
It is well-known that the vectors
wc
and
Jc
are related by the following
equation:
Jc
=
zw,
}
where

Rigid
Body
Equations
293
is the inertial operator. Since
5
is
linear and symmetric operator, we can
define
a
~ema~ian metrics on
SO(3).
Indeed, by setting
where
which
defines
a
metric tensor field on
SO(3).
The kinetic energy
T
is
Since
we
=
S-'
Jc,
the kinetic energy can be expressed
as
a
function
of
the
angular momentum
(10.52)
Thus,
the kinetic energy can be considered to be
a
function defined on the
Every tangent vector to the orbit
V
at the point
J
can be written
as
follows:
1 1
1
T
=
~(wC,Qwc)
=
Z(W~,W~)
=
5(W1Jc,
Jc)
.
dual space
9'
of the algebra.
It,
JL
with
6
E
9.
On
the other hand, since
dT
=wc,
the right hand side
of
the Eder equation can be written in the form
IdT,
JL
where the differential
1-form
dT,
being the exterior derivative of
a
function
defined on
G*,
belongs to the dual space
of
8*,
that is
S.
