Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


214
hie
Groups
and
Lie
Algebras
relevance; they are
Abelian Lie algebras, simple Lie
algebras,
and
se~~-$imp~e
Lie alge~~as.
Vector spaces endowed with
an
identically vanishing co~mutator co~titute
the so-called commutative or Abelian Lie algebras. The definitions of simple
and semi-simple Lie algebra need the introduction of a further concept, that
of
ideal
in a Lie algebra.
A
subspace
Z
of
a
Lie algebra
A
is said to be an
ideal
if
that is
z
E
Z,
if
[y,x]
E
Z
for every
y
E
A.
Of course, since
[y,
4
=
-[z,
3)
and
Z
is
a vector spacet if
[z,
yf
E
Z
for
every
3
E
A,
then
x
E
Z.
Notice that this implies
Z
is
a
subalgebra.
~~~za~
ideals
in
A
are
(0)
and
A.
A
Lie atgebra. coIitaining just trivial
ideals is called
simple.
A
Lie algebra containing nontrivial ideals, but none
of
them Abelian,
is
called a
semi-simple Lie algebra.
There exist different methods
to
build
a
Lie algebra from a Lie group. Here
we are going to give account of the two most significant methods.
The first of them is based on the use of differential operators
on
the group.
8.2.2
&eft
inwariant
vector
Pelds
Let
G
be
a
finite dimensional Lie group. For every
g
E
G,
the left translation
L,
:
h
E
G
-+
L,(h)
=
gh
E
G
is
a
diffeomorphism from
G
to itself. Any neighborhood of
e
is mapped by
left translation along
a
particular
g
onto a neighborhood
of
g,
so
that
the
map carries curves through
e
into curves through
g,
and
curves through
h
into
curves through
gh.
Then, the derivative
of
the map
at
point
h,
namely
(A@)&,
is
a
linear map from the tangent space
ThG
to the tangent space
qhG,
If
v
is
a vector field,
its
value
V(h)
at
point
h
belongs to
ThG.
Its
image
by
(&)+h,
which belongs to
7&G,
will be denoted by
~gV)(g~);
i.e.

Building
of
a
Lie
Algebra
from
a
Lie
Group
215
so
that we have
(gV)(h)
=
(~g)*g-dWW
'
(8.5)
(SV)(h)
=
V(h)
)
vg,
h
E
G7
A
vector field
V
on
a
Lie group
G
is
said to be
left inva~ant
if
or equivalently,
if
V(gh)
=
(Lg)*hV(h)
*
The addition of vector fields
on
G
and their product with real numbers can
be
naturally defined
as
follows:
(V
+
W(g)
=
V(g>
+
wl)
9
t/g
G,
(.lV>(g)
=
.lV(g)
7
so
that,
by
the linearity
of
the operator
(Lg)*gr
it follows that
The set
of
left invariant vector fields on
G
is a vector space over
!I?.
Moreover,
e
A
left invariant vector field on
G
is
uniquely determined
by
its value
at
the identity element e
of
the group
G.
Indeed, if
V(h)
and
W(h)
are left invariant vector fields on
G
The vector space
of
the left invariant vector fields is isomorphic
to
ZG,
the
t~~~e~t space to
G
at e,
Indeed, with every vector
V,
E
7,G,
we can associate a vector field
V(h)
on
G
by
means
of
the operator
(Lh)*e
V(h)
=
(Lh)*e(Ve)
1
Vh
E
G,
(8.6)

216
Lie
Groups
and
Lie
Algebras
the left invariance of
V(g)
following from
Let
us
consider now the set of differential l-forms
a
on
G
that consti-
tutes a vector space on
!R
if
the sum and the product with real number
T
are
defined
as
A
useful notation for the operator
(Lg)*
is
given by the symbol
dL,.
Then,
relations
(8.5)
and
(8.6)
can be rewritten
as
follows:
(9Wh)
=
dW%J-lh))
,
(8.7)
V(h)
=
dLh(V,)
1
(8.8)
Let
us
introduce the transposed operator
dLi
of
dL,,
which acts on the
differential l-forms
a,
by
v,
dL;(a))
=
(dLg(V)
I4
1
(8.9)
where
V
and
a
are
a
vector field and
a
differential 1-form
on
G,
respectiveIy,
and the brackets
(.,
a}
denotes,
as
it
is
usual,
the interior product.
Thus,
dL,
and
dL:
are the following operators:
dLg
:
GG
-+
GhG,
dLz
:
Tj,G
+
7zG.
(8.10)
A
differential l-form a on
G,
is transformed by means of the translation
dL:
in a differential
l-form
ga
on
G,
according to the relation
(9ff)(h)
=
d~~(~~gh)).
A
differential l-form
a!
is
said to be
left
invariant
if
(9~)(h)
=
ff(W
f
v9,
h
E
G.
Since the linearity of
dL,
implies the linearity of
dL:,
we
have that

Building
of
a
Lie
Algebm
from
a
Lie
Group
217
The
set
of
left invariant d~~e~ent~~l
I-forms
on
G
is a vector space on
92.
Moreover
e
A
left invariant diflerential
1-form
is uniquely determined
by
its value
in
e.
Indeed,
if
a
and
a‘
are two left invariant differential 1-forms, such that
a(e>
=
d(e),
we have
a(g)
=
(9-’a)(g)
=
dL;-l(a(g-lg))
=
dLi-l(a(e))
=
dL~-~(a’(e))
=
dL;-~(~’~g-’g))
=
a’(g)
.
As
the
vector space of the left invariant vector fields
is
isomorphic to
T,G,
so
the vector space
of
left invariant differential
1-forms
is
isomorphic to
TG.
If
a,
denotes
a
covector on
7,Gl
the differential 1-form
a(g),
defined by
a(g)
=
dLrf-i
(~le)
Vg
E
G,
is
a
left
invariant differential 1-form.
Indeed,
since
dL&
=
dLS;
o
dLi,
we
have
(ga)(h)
=
dLS;(a(gh))
=
*
dL&h)-l)(@e)
=
(dL:
o
dL;-l9-1)(ae)
=
dL;-l(ae)
=
a(h)
Vgh
E
G.
Thus,
with
every covector on
7,G
we can associate,
in
a
unique way,
a
left
An
interesting and useful result is the following:
invariant differential form on
G.
I)
The
contraction
(a,V),
between a left invariant differential
1-form
a
and
a
Zeft
invaria~~ vector field
V,
is
constant on
G.
Indeed,
(a1
V)(g)
=
(ff(d1
Vb))
=
(d-q-l(a(e)), dLg(V(e)))

218
Lie
Groups
and
Lie
Algebras
=
Wg-1
0
dLg)((ff(e),
V(~)))
=
(44,
V(e))
7
vg
E
G.
There
is
a converse to this, namely
a
A
vector field
V
on
G,
for
which
(a,
V)
is
constant on
G
for
every left
invariant differential
1-form,
is
a
left
invariant vector field.
Indeed,
{~(h),
9V(h))
=
(4h),
~~~(V(g-lh)~~
=
{~~~(~(~)),
v(g-'h))
=
(49-
lh),
V(9-'h))
=
{ff(h~,
V(h)}
*
Since the left invariant form
a
is arbitrary, then
(gV)(h)
=
V(h)
,
Qg,
h
E
G.
These two properties, together with the useful relation*
d4X,
Y)
=
Lx((ff,
y>>
-
LY((&,
X))
+
(a,
[X,
'YI)
,
(8.11)
allow us to prove the following statement:
*
If
X
and
Y
are two
left
invuria~t uec~o~~elds on a Lie
group
G,
their
Lie ~ra~~ets
[X,
Yj
is
a
left
invaria~~ vector field.
To this purpose, we just have to prove that
{a,
IX,
Y])
is
constant on
G
for every left invariant differential 1-form
a(g).
Indeed,
if
a
is
left invariant,
then
(a,
X)
and
(a,
Y)
are constant on
G,
because
X(g)
and
Y(g)
are, by
hypothesis, left invariant vector fields,
so
that the Lie derivatives
Lx((a,
Y))
and
Ly((a,
X))
vanish identically.
Therefore, we have
WX,
Y)
=
(a,
[X,
yi>
*
(8.12)
Since
da
is
an exact %form, for which
dda
=
0,
and the right hand
of
Eq.
(8.12)
is
a O-form; that is,
a
function on
G,
then
(a,
[X,
Y])
=
constant.
*In
this chapter the Lie derivative, with respect to a vector field
X,
has been denoted
with the
symbol
Lx,
instead
of
Lx,
to
avoid confusion with the left translation
Lx.

Building
of
a
Lie Algebnz
from
a
Lie
Group
219
Thus, by using the isomorphism between
7,G
and the vector space of the
left invariant vector fields, it is possible to introduce, in the tangent space
ZG,
a
commutation relation which, being bilinear, antisymmetric, and satisfying
the Jacobi identity, endows it with
a
Lie algebra structure.
To be specific, if
Xe
and
Ye
denote two vectors belonging to
7,G,
the Lie
brackets
of
the two left invariant vector field on
G
corresponding to them, still
is
a left invariant vector field which also is uniquely determined by its value at
the identity element of the group.
Thus, given
Xe
and
Ye
belonging to
7,G,
we define the
Lie commutator
of
X
and
Y
as
the value, at the identity element
e
of the group
G,
of
the Lie
bracket of the corresponding left invariant vector fields
This Lie algebra is called the Lie algebra
of
the Lie group
G.
8.2.3
There exists a second method,
&s
well, which allows
us
to introduce a Lie
algebra structure in the tangent space
7,G.
The
adjoint representation
of
a Lie
group
Let
us
observe that the map
A,
:
h
E
G
-+
A,(h)
=
ghg-'
E
G
,
composed of the left translation
by
g
and the right translation by
9-'
A,
=
Rg-i
L,
:
h
E
G
+
(R,-i
L,)(h)
=
ghg-'
E
G,
is
a
onsto-one, differentiable map. Actually, since
A;'
=
A,-I,
the map is a
diffeomorphism of
G
into itself.
Since
A,
is
a
homomorphism of
G
into itself. Actually,
A,
is
an isomorphism
of
G
into itself,
as
to say an
inner automorphism
of
G,
since
A,-I
=
A;'.
Notice that each
A,
maps the identity element
e
into itself,
so
that ev-
ery curve through
e
is
mapped into a, possibly different, curve through
e.

220
Lie
Groups
and
Lie
Algebras
Therefore, the derivative
at the unit
e,
usually denoted with
Ad,,
Adg
:
ZG
-+
7,G,
is an invertible linear map
of
any tangent vector of
7,G
to another one
in
7,G.
For
the automorphism
A,,
we have
and for derivatives
so
that
Adfg
=
Ad$
o
Ad,
.
The set of all invertible linear maps of
ZG
into itself is a group whose
internal composition law is the usual composition
of
maps. This
group
is
denoted by Aut
7,G.
Thus, the map
Ad
:
g
E
G
3
Ad(g)
=
Adg
E
Aut
ZG
(8.13)
is
a
homomorphism
of
G
into the group
Aut7,G
of the invertibIe linear maps
of the vector space
7,G.
Once
a
basis
in
7,G
is
chosen, the map
Ad
becomes
a
homomorphism
of
G
into the group
GL(n,
S),
where
n
is
the dimension
of
7,G.
The group
GL(n,
8)
is the group of nonsingular real matrices
n
x
n
and can be endowed with
a
differential manifold structure.
The compatibility of group and differential manifold structures promotes
the group
GL(n,
92)
to a Lie group. Obviously, the dimension of
G~(~,
!I?)
is
n2.
Thus, the map
Ad
is
a representation of
G
on
7,G
and
is
called the
adjoint
representation
of the Lie group
G.
The tangent space to
GL(n,R)
at
the identity
I
(the unit matrix)
is
the
space, denoted with
~~t~~8),
of
the not necessarily invertible real matrixes
n
x
n.
The map
Ad
is
differentiable and its derivative
(Ad)*,
at the unit
e
is
a
linear map
of
ZG
in
EndKG,
the vector space of the (not necessarily

Building
of
a
Lie
Algebra
from
a
Lie
Group
221
invertible) linear maps
of
7,G into itself, that are endomorph~sms
of
7,G.
In
other terms,
End7,G
=
T(Aut 7,G)
.
The derivative
(Ad),,
is
denoted with the symbol
ad,
ad
:
V
E
7,G
4
ad(V)
=
adv
f
End7,G.
A
one parameter
subgroup
of
a Lie group G
is
a
representation of
R
in G,
as
to say
a
homeomorphism
of
!I?
in
G;
that is, a differentiable map
p
:
t
E
R
-+
p(t)
E
G,
such that
p(0)
=
e
,
p(t
i-
t')
=
p(t)p(t'),
Vt,
t'
E
R.
Let
Ve
be an element in
7,G
and let
pv,
:
t
E
R
+
pv,(t)
=
etve
E
G
(8.14)
be the integral curve
of
the left invariant vector field
(Lg)*e(K)
on
G.
Let
us
also
fix
s
E
R
and define the map
Xl
:
t
f
92
-k
xft)
=
PV.
(S)PV*
(t)
=
L,ve
(o)Pv,
(t)
E
G
9
where
Lpve
(dl
is the left translation
by
pv,
(9).
Since the vector field
(La)*e(V)
on G
is
left invariant, we have
SO
that
XI($)
is
an
integral curve
of
V
=
(LQ)*e(Ve) through
pv,(~)
at
t
=
0.
On the other hand, the map
xz
:
t
E
!R
-+
~2(t)
=
pv.
(s-tt)
E
G
is
also
an
integral curve
of
V
=
(Lg)*e(Ve)
through
pv,
(3)
at
t
=
0.
pv,(s)
at
t
=
0
is unique.
As
a
consequence, we have
Thus,
xl(t)
=
~2(t),
since the integral curve of
V
=
(LQ)*e(&)
through
Pv,
(8
4-
t)
=
PV.
(S)PV,
(t)
(8.15)
222
and
Lie
Gmups
and
Lie
Algebras
&om
Eq.
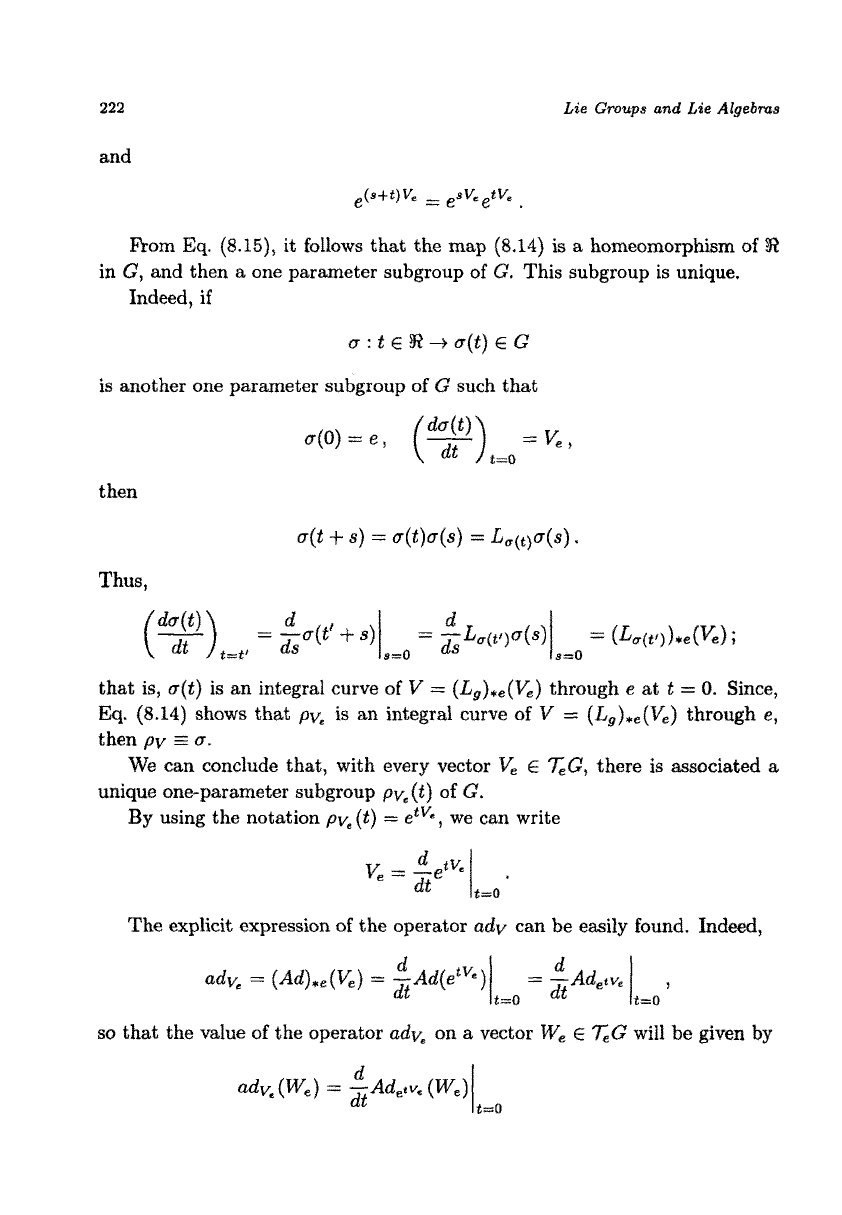
(8.15), it follows that the map (8.14)
is
a homeomorphism
of
R
Indeed,
if
in
G,
and then a one parameter subgroup
of
G.
This subgroup
is
unique,
u
:
t
E
92
+
6(t)
E
G
is
another one parameter subgroup
of
G
such that
then
a(t
f
s)
=
a(t)a(s)
=
LV(t)6(S).
Thus
,
that
is,
G(t)
is an integral curve
of
V
=
(L~)*e(~)
through
e
at
t
=
0.
Since,
Eq.
(8.14) shows that
pv,
is
an integral curve of
V
=
(Lg)*e(Ve)
through
e,
then
pv
=
0.
We can conclude that, with every vector
V,
E
7,G,
there
is
associated
a
unique one-parameter subgroup
pv,
(t)
of
G.
By
using the notation
pv,
(t)
=
etv-
,
we can write
The explicit expression
of
the operator
adv
can be easily found. Indeed,
so
that the value
of
the operator
adv,
on
a
vector
We
E
T,G
will be given
by

Building
of
a
Lie
Algebm
from
a
Lie
Group
223
On the other hand,
etVe
is just the value
at
e
of
the flow of the vector field
V(g)
=
(Lg)*e(K),
as
it
is easily followed by
&$)
=
getv=
=
Retveg,
so
that
etv
=
&(e).
Thus,
we have
(8.16)
By
Eq.
(8.16) and by
the
well-known properties
of the
Lie derivative, we
can define the following bracket in
7,G:
[.
1
*]
=L:
(Ve,
We)
E
'ZG
x
T",G
-+
fVe,
We]
=
adv,(We)
E
7,G
(8.17)
which
can
be
easily seen to be
bilinear because
a~c,xl+caXa(y)
=
cladx,
(Y)
+
czadx,(Y),
adx(c1YI
+
c2Yz)
=
c1adxfY1)
4-
aadx(Y2),
whatever
XI,
XZ,
V,
YI,
Y2,
Y
E
7,G
and
CI,
c2
E
8
are chosen;
e
antisymmetric because
adx(Yf
=
-udy(X), VX,Y
E
7,G;
and
satisfying the Jacobi identity
Udx(udy(2))
f
dY(adz(X))
-t-
~dz(u~~~Y))
=
0.
