Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


Chapter
7
Integration
Theory
7.1
Orientable
Manifolds
A
differential manifold
M
is said to be
orientable
if a nowhere vanishing con-
tinuous differential n-form
R
exists on
it.
Such a differential n-form is said to
be a
volume
n-form.
At each point
p
E
M,
the n-form
R
will define an n-covector,
0,
E
A"('&M),
whose value
QP(el,e2,.
.
.,en)
on a basis
(el,e2,.
.
.,en}
in
7,M
will be different from zero.
So
all the basis in
7,M
will be divided in two
classes according to the sign
of
Rp(el,
e2,.
. .
,
en).
The two classes are indepen-
dent from
0,
because every nowhere vanishing n-form
0'
will
be proportional
to
52
by a factor
f
#
0,
and then it will take the same sign (depending
on
the
sign of
f)
on the elements of each class. The two classes will be called
left-
handed
and
right-handed.*
Thus, it will be possible to choose, continuously
Vp
E
M,
a basis
{el,
e2,.
,
.
,
en},
belonging to the same class.
If
the basis are
coordinate basis, the Jacobian determinant in the transition from one basis to
another will have, in the neighborhood of each point
p
E
M,
the same sign.
The Mobius band is a not an orientable manifold.
'Which class has which name
is
a convention, since the sign
of
R'
will depend on
f,
which
is
at
our
disposal.
195
196
Integmtion Theory
7.2
Integration
on
Orientable
Manifolds
Let
M
be an n-dimensional orientable manifold and
w
be
a
differentia1 n-form
on it. In
a
given chart
(U,
cp),
w
will
be
locally
re~r~~nt~
as
w
=
f(~',
.
, ,
)
sn)dx'
A
* *
A
d~*
I
The integral of
w
over
U
C_
M
is
defined by
s,
w
=
i(u,
f(x',
. .
.
,
xn)dx'
.
.
.
dz"
,
(7.1)
where
p(U)
C
Rn
is
the
~~uge
of
U
and the
symbof
dx'
*
*
dxn
denotes the
measure for the usual integral of differential calculus.
In
order to show that the integral
so
defined does not depend on the coor-
dinates, let us choose a different coordinate basis
{a/ayi}
in which
w
=
f(y1,.
. .
,y*)dyl
.
. .
dy"
.
The equality
~(y)dyi
A
*.
*
A
dyn
=
f(x)dzl
A
-
*
*
A
dxn
,
evaluated on the
basis
{B/ayi}
gives
or
where
J
is the Jacobian determinant.
Thus,
we
have

p
-
Vectors
and
Dual
Tensors
197
Then,
our
definition
of
the integral
of
w
will not depend on the coordinates
if
f(.’,
.
,
.
,
sn)ds’
’
*
*
0kn
=
Leu,
Jf(Y’,’*’,yn)&’
*’*&J”.
J,,,
As
we know from differential calculus, the above equality holds only when
It
follows that in the definition
(7.1),
an orientation
for
2.4
must be chosen;
that is,
a
requirement on the handedness
of
the basis must be added. This
ex-
plains why, from the very beginning,
M
has been supposed to be an orientable
manifold; that
is,
one for which it
is
possible to choose, continuously at every
point
p
E
M,
a
coordinate basis
{6/axk}
with the same h~dedness.
However, the integration theory
of
differential forms has been extended, by
de
%am,
to
nonorientable manifolds4 by introducing forms
of
odd
parity,
and
this
can
have interesting physical applications.173
On
the historical side we
shall mention that they were introduced by Hermann WeylS6 and developed
by S~houten,*~ and called
Weyl tensors.
Synge and Schild refer to them
as
oriented tensors,
while de Rham called them
tensors
of
odd kind.7
Under
a
change
of
coordinates
(x
#
x’),
a
~~~sted ~~~e~nt~~~
fo~
transforms
as
folIows
J
>
0.
J
8xP
6x9
axr
w;b...c
=
---
*.
.
-
I
JI
axfa
ax’b
&lc
wpq”’r
where
J
is the Jacobian determinant and
I
JI
its absolute value.
7.3
p-Vectors
and
Dual
Tensors
Completely antisymmetric tensors of type (p,
0),
on a n-dimensional vector
space
E,
are called p-vectors.
A
Grassmann algebra, can be,
of
course, con-
structed on them in complete analogy with that
of
p-covectors. The vector
space
of
p-vectors is denoted with Vp(E).
Its
dimension
is
dimVF(E~
=
(r
)
=
(
)
=
dimVn-p(~)
,
n-p
and
a
basis
is given by

198
Integration
Theory
Thus,
dimVp(E~
=
dimVn-"(E)
=
dimAp(~~
=
dimA"(E).
If
{&)
is
the dual basis
of
{ei),
the n-covector
1
n!
f),
=
6'
A
6'
A
..
.
A
6"
z
-&iliz,.qi,~il
A
@
A
.
,.
A
din
is
a
basis
for the vector space
A",
and will be called
a
vol~~e covec~~r.
By
using the volume covector, we can associate, with any p-vector
1
X
=
-Xab''cea
A
eb
A
. .
A
e,
,
P!
the
(n
-
p)-covector defined by
1
...
fz(X)
ixfz
=
-X'1'2'%E
i,az...i,@b+l
.
A
&+z
A
.
.
.
A
dim
.
P!
The above
(n
-
p)-covector is called the
f),-duaZ
of
X
or
also
the
Poincare'
dual
of
x.
A
basis independent definition
is
given
by
ixfl(Y"fi,.
.
.
,yn)
=
fz(x
AYP+~
A,.
.
A
Y-)
vyp3-1,.
.
.
,yn
E
E.
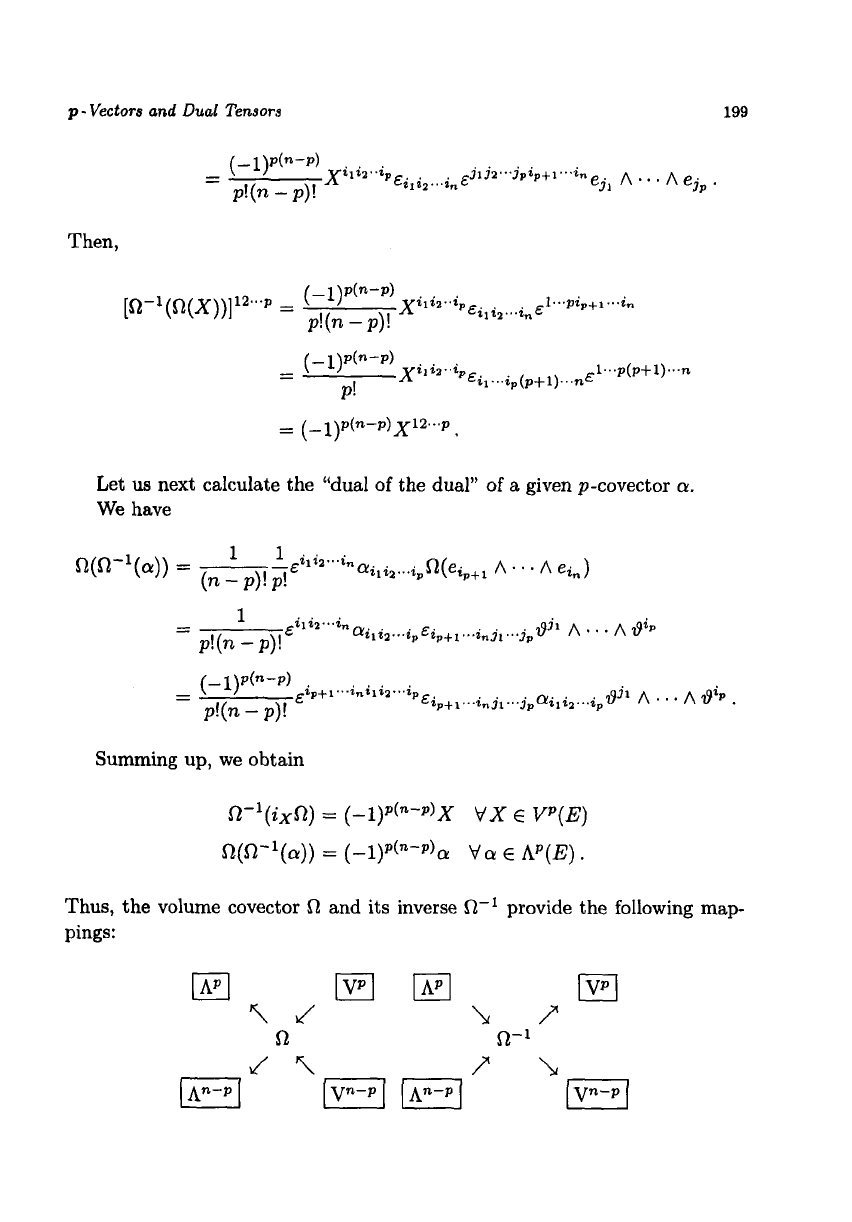
It
is
also possible to make the inverse; that
is,
to associate, with
any
p-
covector
a,
the
(n
-
p)-vector
where
p
-
Vectors
and
Dual
Tensors
199
Then,
Let
us
next calculate the "dual
of
the dual"
of
a given p-covector
a.
We
have
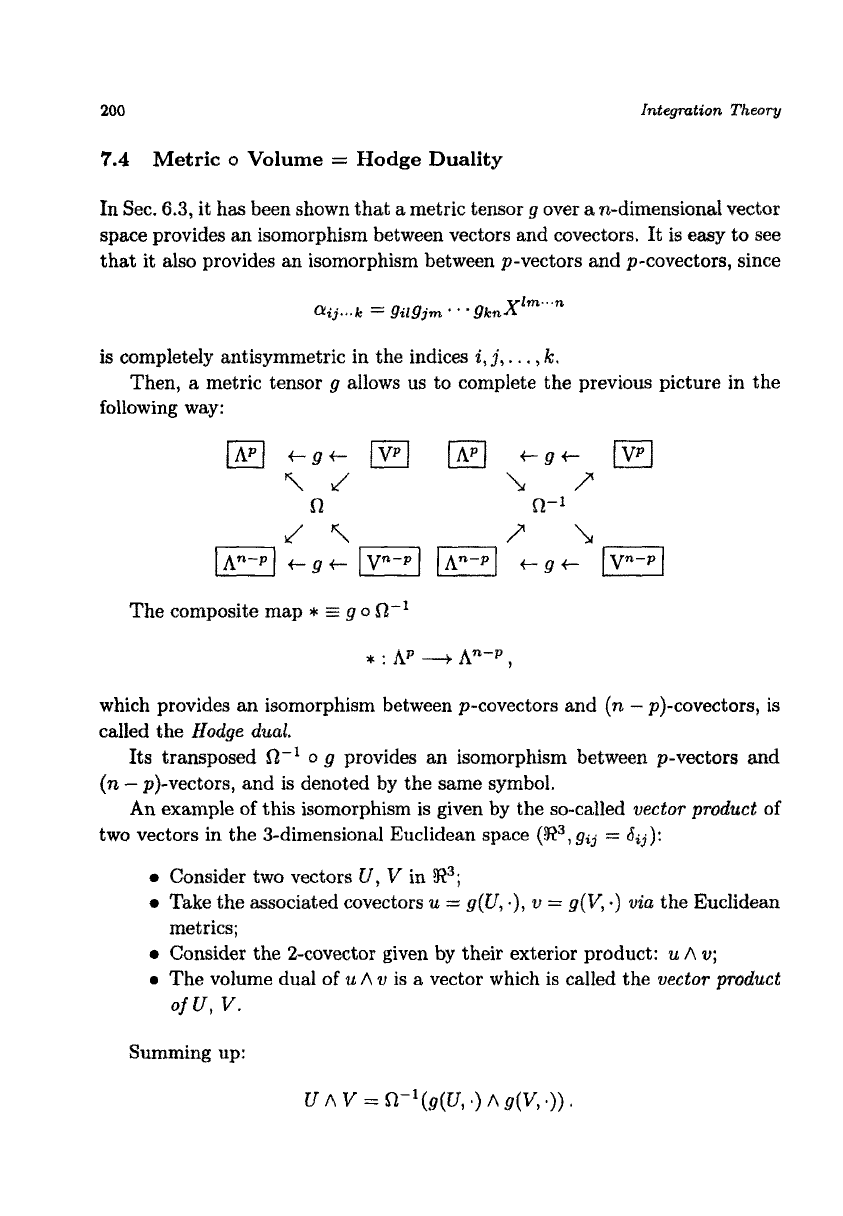
Summing
up, we obtain
R-l(ixR)
=
(-1)P(*-P)X
vx
E
VP(E)
R(R-'(a))
=
(-l)p(n-p)~
Va
E
hp(E).
Thus, the volume covector
R
and its inverse
O-'
provide the following map-
pings:
200
Integration
Theory
7.4
Metric
o
Volume
=
Hodge
Duality
In See.
6.3,
it has been shown that
a
metric tensor
g
over
a
~-d~mensiona~ vector
space provides an isomorphism between vectors and covectors.
It
is easy to see
that it also provides
an
isomorphism between p-vectors and p-covectors, since
is
completely antisymmetric in the indices
e',
j,
.
.
.
,
k.
following way:
Then,
a
metric tensor
g
allows us to complete the previous picture in the
cgc-
eg4-
\d
LP
sz
$2-'
2\
pl<g>F]pJ
tgt
[w
The composite map
*
=
g
o
51-I
which provides an isomorphism between p-covectors and
(n
-
p)-covectors,
is
called the
Hodge dual.
Its
transposed
W'
o
g
provides an isomorphism between p-vectors and
(n
-
p)-vectors, and is denoted by the same symbol.
An example
of
this
isomorphism is given by the so-caHed
vector
product
of
two vectors in the %dimensional Euclidean space
(R',
gij
=
Sij):
Consider two vectors
U,
V
in
R3;
Take the associated covectors
u
=
g(U,
a),
v
=
g(V,
*)
via
the Euclidean
Consider the 2-covector given by their exterior product:
u
A
u;
0
The volume dual
of
u
A
v
is
a
vector which is called the
vector product
metrics;
of
u,
v.
Summing up:

Metric
o
Volume
=
Hodge
Duality
201
Remark
14
for
diflerential 1-forms, and
fl
be the volume-form
If
a manifold has a metric g, let
{di}
be an orthonormal basis
fl=
d1
A
d2
A
*
*
'
A
8".
If
{xk}
is
an arbitrary coordinate, and
A
is
the transformation matrix from
{dxk} to
(19~);
i.e.
di
=
,
we have
fl
=
A:
.
A?.
. .
A
dxj
A
.
.
.
A
dxk
3
=
.
A;.
.
.
Az&"'kdxl
A
dx2
A
. .
.
A
dx"
=
(det A)dxl
A
dx2
A*.
.
A
dxn
.
On the other hand, we also have
gij
=
@A$g(eh, ek)
9
where
gij
are the components
of
the metric tensor g
in
the coordinate basis
{8/axi}, and g(eh, ek)
=
dhk,
since the original basis was orthonormal.
Therefore,
and
g
E
det(gij)
=
(det
A)2
fl
=
mdx' Adx2
A
A
dxn
.
&om
Eq,
(7.3)
it
follows
that the components
Rij'..k
of
fl-'
are given by
1
--
1
~12s.-
-
-
n12...n
*
However,
in
our metric manifold the inverse
of
R
could also be defined by
fiW..k
=
,pgjq
.
. .
gk~~Rpq...,
,
so
that

202
Integration
Theory
If
g
is negative,
f1212".n
and
flf12"'n
differ
by
a sign. In special
or
general
relativity, it is conventional to use
fl"''.n
in
the dual relations.
7.5
Stokes
Theorem
Let
M
be an orientable n-dimensional differential manifold and
U
be
a
region
of
M.
We call
boundary
of
U
an orientable n
-
1
dimensional submanifold of
M,
namely
aU,
which divides
M
-
dU
in two disjoint parts,
U
and
CU,
in
such
a
way that any continuous curve joining a point of
U
with
a
point in
CU
contains
a
point of
dU.
Let
us
consider the integral
of
an arbitrary n-form
w
over
U
Let
X
be a vector field and
U(T)
the images of
U
under the flow
cp
generated
by
x:
u(T)
=
(pT(u)
'
From the analysis already performed in Sec.
4.1,
it
follows that
Lx
L(7)
=
k(T)
Lxw
'
or
since the form
w
is obviously closed.
obtain
On the other hand, by applying directly the definition of Lie derivative, we
1
=
lim
-
T-+o
with
U(0)
=
U
and
W(T)
=
U(T)
-
U(0).

Stokea
Theorem
203
Let us consider
a
part
aV(0)
E.
aU(O),
to which the vector field
X
is not
tangent, and a part
6V
(T)
of
~U(T),
representing a region between
OU(0)
and
aU(.r)
locally given by
6v(T)
=
av(0)
X]o,
T[
.
Then
if
(22,
x3,.
.
.
,x,)
denote the coordinates
for
aV(O),
we can introduce,
with
$1
=
T,
coordinates
(x~,xz,.
. .
,z,)
for
~V(T).
In these coordinates the
differential n-form
w
can be expressed
as
follows:
W=
f(x1,~z
,...,zn)d~lAdx2A."'Ad~,
and
=
lim
1
J
[T
f
(O,X~,.
.
.
,
xCn)]dxz
A
* *
-
A
dx,
7-tO-r
BV(0)
where the symbol
denotes the restriction to
dU.
The final equation
is independent from the constructed coordinates, but it requires that
X
should
not be tangent to
aU
in
V.
For the whole boundary
NJ,
two cases can
occur:
X
is tangent
to
isolated points forming
a
submanifold of lower dimen-
sionality.
