Vilasi G. Hamiltonian Dynamics

324

KdV

Equation

1

2

B

=

-.laxxx

-

U(Z,

t)a,

-

-u~(z,

t)

.

Let

us

consider the eigenvalue problem

L$

=

k2$

,

which will have

as

solutions

fie states,

corresponding to

k2

>

0,

represented by waves

+O(x,

k,

t),

whose asymptotic behavior is given by

where

are the unknown

tra~sm~ss~on coeficient

and the

reflection coefficient,

respect ivefy

;

bound states,

corresponding

to

k2

<

0,

represented by eigenfunctions

$:(x,kn),

with

ki

=

-x$

(or

better,

kn

=

iXn,

xn

>

0),

whose

asymptotic behavior

is

given by

N

$n(Z,

ixn,t)

x4--00

exPlxn4

1

$3.1

ixn,

t)

zf;bo

Cn(Xn7

t>

.XP[-Xn.I

1

where the coefficients

ci(xn,

t)

are the unknown

normalization con-

stants.

The set

s

=

{xn, cn(xn,

t.),

R(k,

t)

1

Vk

E

Ill}

is

the

set

of

scattering data

which can be determined by the asymptotic be-

havior

of

the operator

B

Conservation

Laws

325

Indeed, the equation

$=B$,

400

=

B,&J

9

given in

Eq.

(12.19),

will be true also ~ymptotically

so

that we

can

write

e

for

bound

states

&(xnrt)

@XP[-Xn4

=

4X%n(Xn,

t)

exP[-Xn4

r

4Xn,

t)

=

&xn>

exp[4x3ntI

;

which

is

trivially integrated to

for

fTee

states

C(k,

t)e-+kc

=

-4ik3c(k,

t)e-ik”,

C-

(k,

t)e-ik”

+

&+{kt

t)eikx

=

-4ik3fC- (k,

t)e-ikx

-

C+

(k,

t)e’ik”2]

,

which gives

C(k,

t)

=

-4ik3C(k,

t),

C-(k,

t)

=

-4ik3C-(k,

t)

,

C+(k,

t)

=

4ik3C+(k,

t)

,

and then,

T(k,

t)

=

TO(k),

R(k,

t)

=

Ro(k)

=p(8ik3t]

.

At this point, we know the time evolution of scattering data when the

“potential” changes according to the KdV equation. We also know that the

number of bound

states

does not change

in

time and

is

determined

by

the initial condition

UO(Z).

In

our

time-dependent case, the

GLM

formula (see Appendix

G)

must be

written in the form

CQ

A(z,

y,

t)

+

F(z

+

y,

t)

+

1

A(z,

z,

t)F(z

+

y,

t)dz

=

0,

X

326

Kd

V

Equation

and the solution

of

KdV

will

be given by

d

dx

U(Z,

t)

=

-~-A(x,

Z,

t)

.

The

o~e-so~~~o~

so~~~~o~~

Let us consider

a

tr~n~p~~nt ~otent~u~

i.e. an

initial condition

UO(Z),

such

that the direct scattering problem gives

Ro(k)

=

0.

Suppose, moreover, that there exists

only

one bound state, with eigenvalue

-x2

and normalization constant

CO.

The kernel

F

of

GLM will be given by

~(2,

t)

=

c;e4x3t-xz

,

so

that

GLM

gives

or

Since

this kernel

is

separable, we can try to

find

a

solution

of the following

form:

so

that the equation simplifies to

and algebraically,

we

obtain

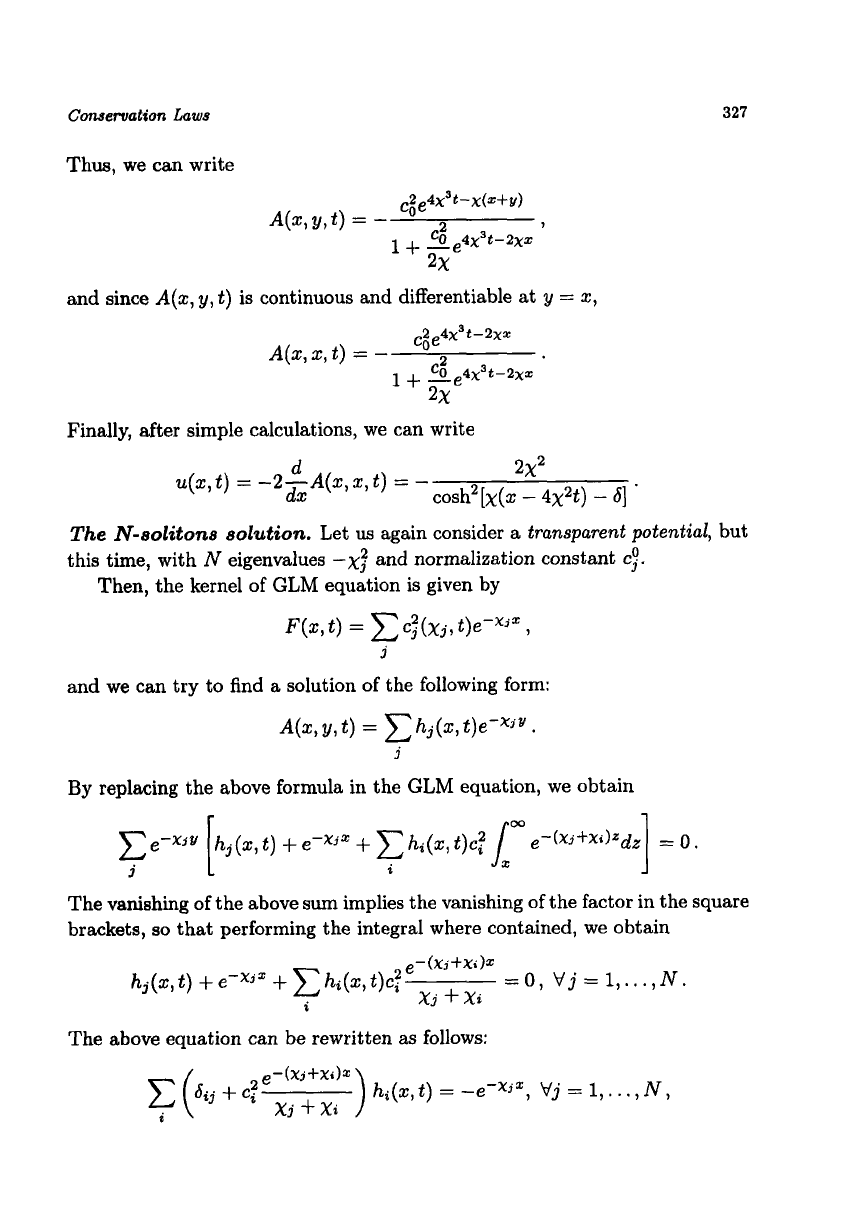
Conseruation

Laws

Thus, we can write

2

4x%-x(xSy)

COe

A(x,y,t)

=

-

2

7

co

4x3t-2xx

2%

1+-e

and

since

A(%,

y,

t)

is continuous and differentiable at

y

=

z,

C$e4X9t

-2xx

1

+

Cge4x3t-2xx

A(z,~,t)

=

-

2

2X

Finally, after simple calculations, we can write

327

d

2X2

U(Z,

t)

=

-2--A(z,

Z,

t)

=

-

dx

cosh2[x(x

-

4x2t)

-

61

'

The

N-solitons

solution.

Let

us

again consider a

transparent potential,

but

this time, with

N

eigenvalues

-$

and normalization constant

c;.

Then, the kernel

of

GLM equation is given by

F(Z,

t)

=

C

ci(xjl

t)e-xjz

,

j

and we can try to find a solution of the following form:

A(z,

y,

t)

=

hj(x,

t)e-xjY

.

j

By

replacing the above formula in the GLM equation, we obtain

The vanishing

of

the above

sum

implies the vanishing

of

the factor in the square

brackets,

so

that performing the integral where contained, we obtain

The above equation can be rewritten as follows:

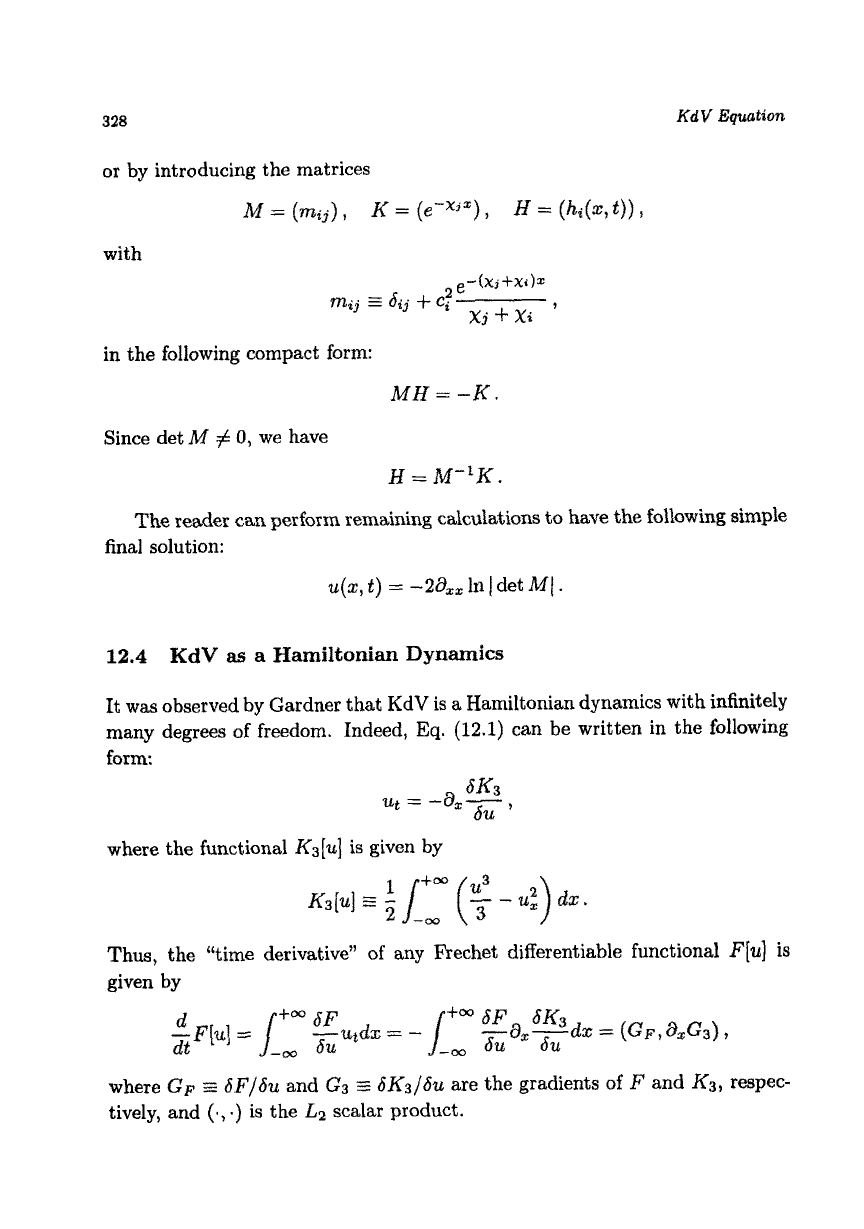
328

Kd

V

Equation

or

by

introducing the matrices

M

=

(mi$)

,

K

=

(e-xj"),

H

=

(~~(~,t)),

with

in the following compact form:

MH=--K.

Since det

M

#

0,

we have

H=M-lK.

The

reader

can

perform

remaining calculations

to

have the

following

simple

final solution:

u(x, t)

=

-2aX.

In

1

det

MI.

12.4

KdV

as

a

Hamiltonian

Dynamics

It

was

observed by Gardner that KdV is a Hamiltonian dynamics with infinitely

many degrees of freedom. Indeed,

Eq.

(12.1)

can be written in the following

form.

6K3

ut

=

-ax-

du

'

where the functional

K3[21]

is

given

by

Thus,

the "time derivative"

of

any Fkechet differentiable functional

F[u]

is

given by

where

GF

I=

SF/Gu

and

G3

3

6K3/6u

are the gradients

of

F

and

KJ,

respec-

tively, and

(0,

.)

is the

Lz

scalar product.

KdV

ap

a

Hamiltonian

Dynamics

329

It

is

easy to check that, for any two Frechet differentiable functional

F

and

F',

the bracket

on the chosen class of functions, is antisymmetric. Moreover, it satisfies the

Jacobi identity and is a derivation on the associative product

of

functionals.

Thus, we

can

conclude that KdV is

a

Poisson dynamics whose Poisson bivector

field

A

has components, in the formal basis

e(z)

=

6/6u(z),

given by the

operator

8,.

The operator

ax

has

a

kernel given by constants

c

E

IR,

so

that it does not

have

an

inverse, and KdV

is

not strictly

a

Hamiltonian dynamics.

However, on the quotient manifold, namely

M,

KdV is

a

Hamiltonian

dynamics; indeed, the manifold

M

can be endowed the symplectic structure

qu]

=

s'"

dx

[;

dY[6+)

A

WY)]

9

-"

which having constant coefficients, is trivially closed.

structed by means

of

Lenard's sequence

(12.11),

a

-Gn+1

=

EkGn

1

dX

Moreover, it

is

easy to show that functionals

Kn,

whose gradients are con-

n

=

1,2,3,

are pairwise in involution. Indeed,

Thus,

if

n

<

m,

an index

k

can be found such that

with

A,

one

of

the two antisymmetric operators

8,

or

Ek,

so

that

A

further important step on the study of KdV,

is

represented by the fol-

lowing result by Faddeev and Zakharo~.'~

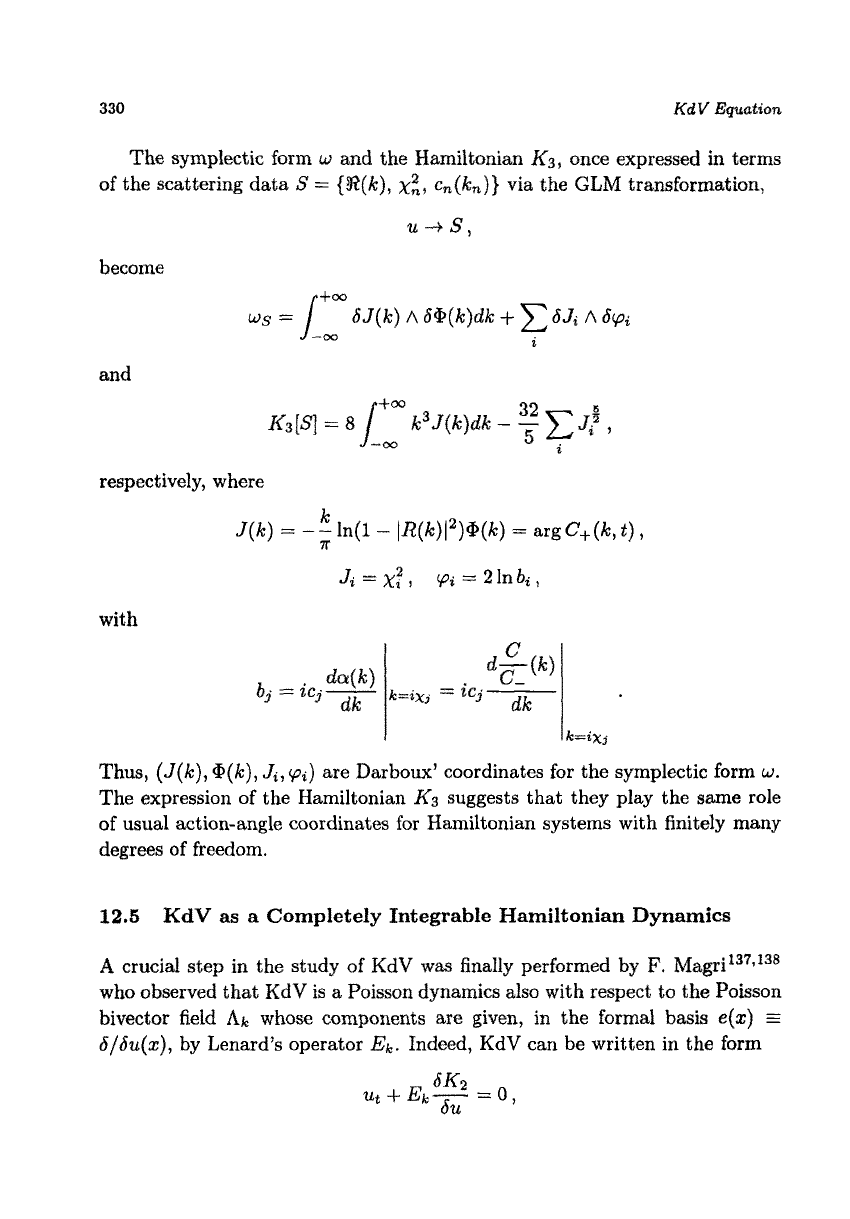
330

Kd

V

EquatQopt,

The sympIe~tic form

w

and the ~~miltonian

&,

once expressed in terms

of the scattering data

S

=

{R(k),

xz,

cn(k,))

via the GLM transformation,

U3S,

become

+w

GJ(k)

A

S@(k)dk

+

C

6Ja

A

6~;

i

and

respectively, where

k

J(k)

=

--ln(1

-

lR(h)(2)@(k)

=

argC+(k,t),

7r

Ji

=

xf

,

pi

=

Zlnbi,

with

I

Ik=iXj

Thus,

(J(k),

@(k),

Ji,

cpi)

are Darboux’ coordinates for the symplectic form

w.

The expression

of

the Hamiltonian

K3

suggests that they play the

same

role

of usual action-angle coordinates for Hamiltonian systems with finitely many

degrees of freedom.

12.5

KdV

as

a Completely Integrable Hamiltonian Dynamics

A

crucial step in the study

of

KdV was finaliy performed by

F.

Magri137~138

who observed that KdV

is

a

Poisson dynamics also with respect to the Poisson

bivector field

Ak

whose

components

are

given, in the formal

basis

e(z)

=

b/Su(z),

by Lenard’s operator

&.

Indeed, KdV can be written

in

the

form

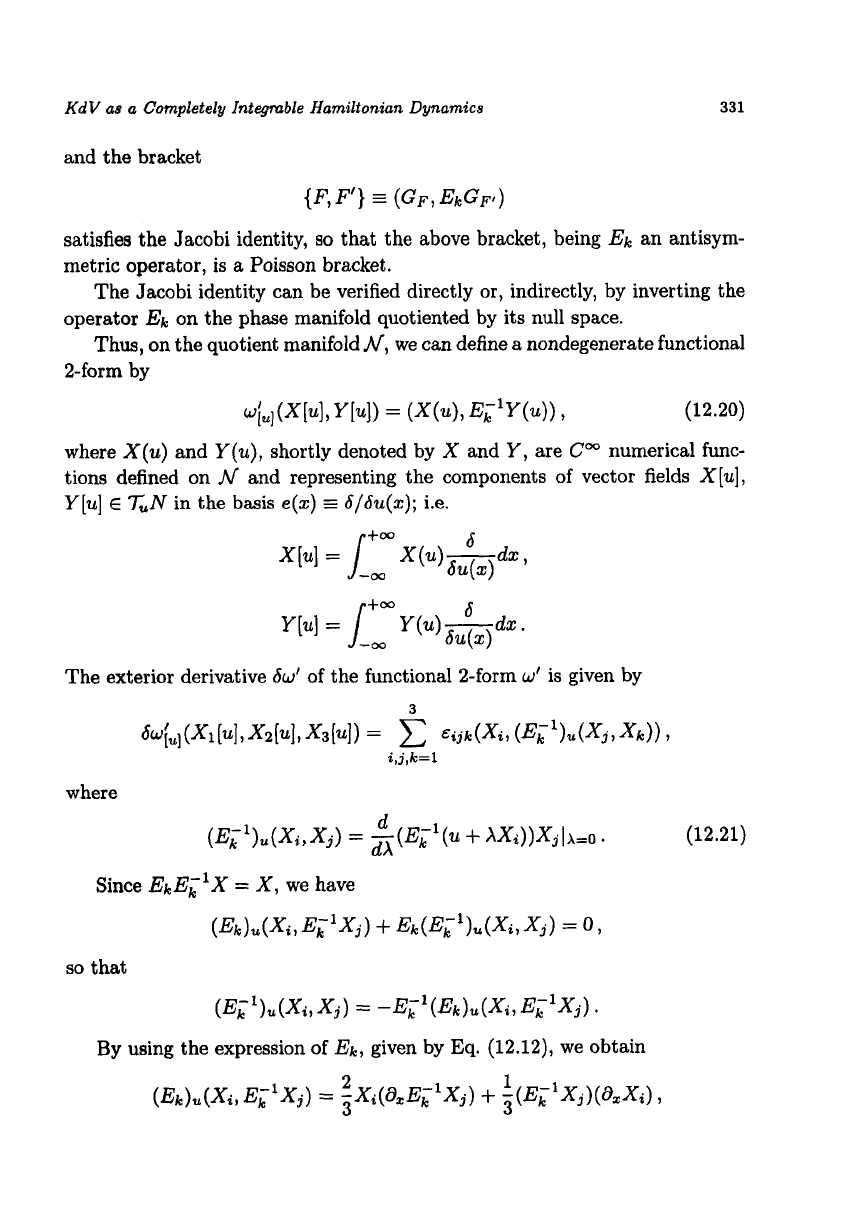
KdV

as

a

Completely Integrable Hamiltonian Dynamics

331

and the bracket

{F,

F'}

3

(GF,

E~GF~)

satisfies the Jacobi identity,

so

that the above bracket, being

Ek

an antisym-

metric operator,

is

a

Poisson bracket.

The Jacobi identity can be verified directly or, indirectly, by inverting the

operator

Ek

on the phase manifold quotiented by its null space.

Thus, on the quotient manifold

N,

we can define

a

nondegenerate functional

2-form by

wju](X[UI,

YI.1)

=

(X('LL),

Jw(4)

1

(12.20)

where

X(u)

and

Y(u),

shortly denoted by

X

and

Y,

are

C""

numerical func-

tions defined on

N

and representing the components

of

vector fields

X[U],

Y[u]

E

TUN

in the

basis

e(z)

=

6/6u(z);

i.e.

d

X(U)-dz,

+m

WX)

s

+m

Y(u)--dx.

Y[UI

=

s_,

a+>

The exterior derivative

6w'

of

the functional 2-form

w'

is given by

3

awjUi(Xi[~],Xz[~],X3[~])

=

C

~ijrc(Xi,

(Eil)u(Xj,Xk)),

i,

j,

k=l

where

d-

dX

(E~l)u(X~,Xj)

=

-(Ek

'(u

+

XXi))Xjl~=o.

Since

EkEi'X

=

X,

we have

(Ek)u(xi)

EF'Xj)

+

Ek(Zr')u(Xai

xj)

=

0

7

so

that

(E;')u(xi,Xj)

=

-E;l(E&(Xi,

EL'Xj).

(Ek)u(xi, E,-'Xj)

=

-Xi(&E;lXj)

+

-(E;lXj)(azxi),

By

using the expression of

Ek,

given by Eq.

(12.12),

we obtain

2

1

3 3

(12.21)

332

KdV

Equation

and then

so

that

swj,,

=

0.

Thus, the

wiU1

is

a

symplectic structure on

N

and the interior product

~AW'

is

given

by

that is

so

that

KdV

is,

on

N,

a Hamiltonian dynamics also with respect to

w'

We

can

define an operator

i"

such that

gk

=Z

&p,

which allows us

to

write the Lenard recursion of gradients

of

conserved func-

tionals

K,(u]

in the

following

form:

On

the quotient manifold

M,

the operator

ax

can

be

inverted

to

give

D-'P(x)

=

([L

cp(z:)dx

-

1'"

~(x)dx)

,

Vtp

E

M

,

X

and we have

KdV

as

a

Completely

Integrable

Hamiltonian Rpamics

333

Between the operator

rf'

and the

Lax

operator

L

=

ax,

+

(1/6)u,

there

exists the following remarkabte reiation:

L$

=

A$

===$-

T$2

=

4Xzf12,

(12.24)

that

is,

if

eigenvalue

A,

then

$a

is

an

eigenstate

of

i"

belonging to the eigenvalue

4X.

is an eigenstate

of

the Schrodinger operator belonging to the

Indeed, if

L$

=

A$,

we have

1

$xx

+

-gu$

=

A$

and

1

=

29:

+

2X$2

-

-u$J'

+

p$2

+

4X

$$)ydy

-

4

Jx

$v$l.'%ratdy

3

L:

--oo

=

2$Z

+

4X$2

-

2$:

=

4Xzf12.

Let

us

observe that the relation, expressed by Eq.

(12.24),

does not depend

on the fact that

u

satisfies the KdV equation. Moreover, by supposing that

the

@'s

are normalized, we have

so

that

and this implies that

Vilasi G. Hamiltonian Dynamics

Подождите немного. Документ загружается.