Vilasi G. Hamiltonian Dynamics
Подождите немного. Документ загружается.


344
where
Geneml
Strztctures
Indeed,
E,
sin
Y
=
D-'
sin
Y
,
so
that the sineGordon equation can also be written in the fo~~~ing
form:'38
vt
+
E,
sin
v
=
0.
Thus,
a
Lenard's type recursion
of
gradients of conserved functionals
can be written
as
follows:
Many of the previous systems, including the Burgers' equation, admit,
in
conclusion, an operator; i.e. an endomorphism on the module
of
vector
fields, namely
rr*,
which
is
invariant under the dynamics and responsible
for
the construction
of
(infinitely many) Abelian symmetries {vector fields)
or,
for
the Hamiltonian ones,
of
infinitely many conservation laws.
Thus, the endomorphism
p,
or its associated tensor fieid
T(a,X)
=
(a,rfX)
appears to be the most interesting object
in
the analysis
of
integrability
of
field
theories. In fact,
as
it
has been shown in Part
111,
it
is
possible to characterize
the complete integrability
of
systems with finitely many degrees
of
freedom
(Liouville integrability)
in
terms
of
mixed tensor field
T
satisfying suitable
conditions.
Example
37
The sine-Gordon
equation
v,t
+
sinv
=
0,

Invariant Endomorphism
345
with
v
=
3(.\/--iD2
-
ux,
-
3v,D)
and where the tilde indicates that the transformation
3
2
u
=
-(u;
+
&uxx)
(13.14)
has been performed.
Then,
T,
and
Tk
are the same tensor field referred to two diflerent
coordinate systems and KdV equation corresponds,
in
the same reading,
to
the Harniltonian dynamics generated by the second conserved functional
of
sine-Gordon eq~ation.'~'
It
follows that the conserved functionals of the sine-
Gordon equation can be obtained from the ones
of
KdV equation simply by
using the transformation
(13.14).
For
instance,
and
so
on.
Example
38
The Liouville equation
a,t
+
exp
a
=
0
admits the invariant endomorphism
TL
=
D2
-
Da,D-la,
+
a2
,
a
=
lim
a,,
which is related to the one
pk
of KdV equation by the similarity transformation
X++CO
TL
=
JfkJ-'
with
J
3
3(-D2
+
azx
+
uxD)
,
and where the tilde indicates that the following transformation
3
2
21
=
--(a;
+
2a
21
-
a2)
has been performed.
346
GenemE
Stmctures
Then
TL
and
Tk
are the same tensor
field
referred to two different co-
ordinate systems and KdV equation corresponds,
in
the same reading,
to
the
Hami$ton~an dynam~cs generated
by
the second conserved funct~onal
of
Laou-
vilk’s
equation.’80
Example
39
The Burgers’ equation
‘1Lt
=
2uu,
4-
u,,
admits the invariant endomorphism
PB
=
D
i-
DUD-1,
w~ich generates an Abelian sequence
of
sy~metries
of
the dynamics.
The
next sections will be devoted to analyze
the
properties
of
our
phe-
nomenological tensor fields.
13.3.1
~~nfl~~cal
~n~fl~~ance
Because
of
the Lenard’s sequence and
of
the bi-Hamiltonian structure
of
(some)
evolution equations, the first relevant property
of
the tensor field
T
is
given
by
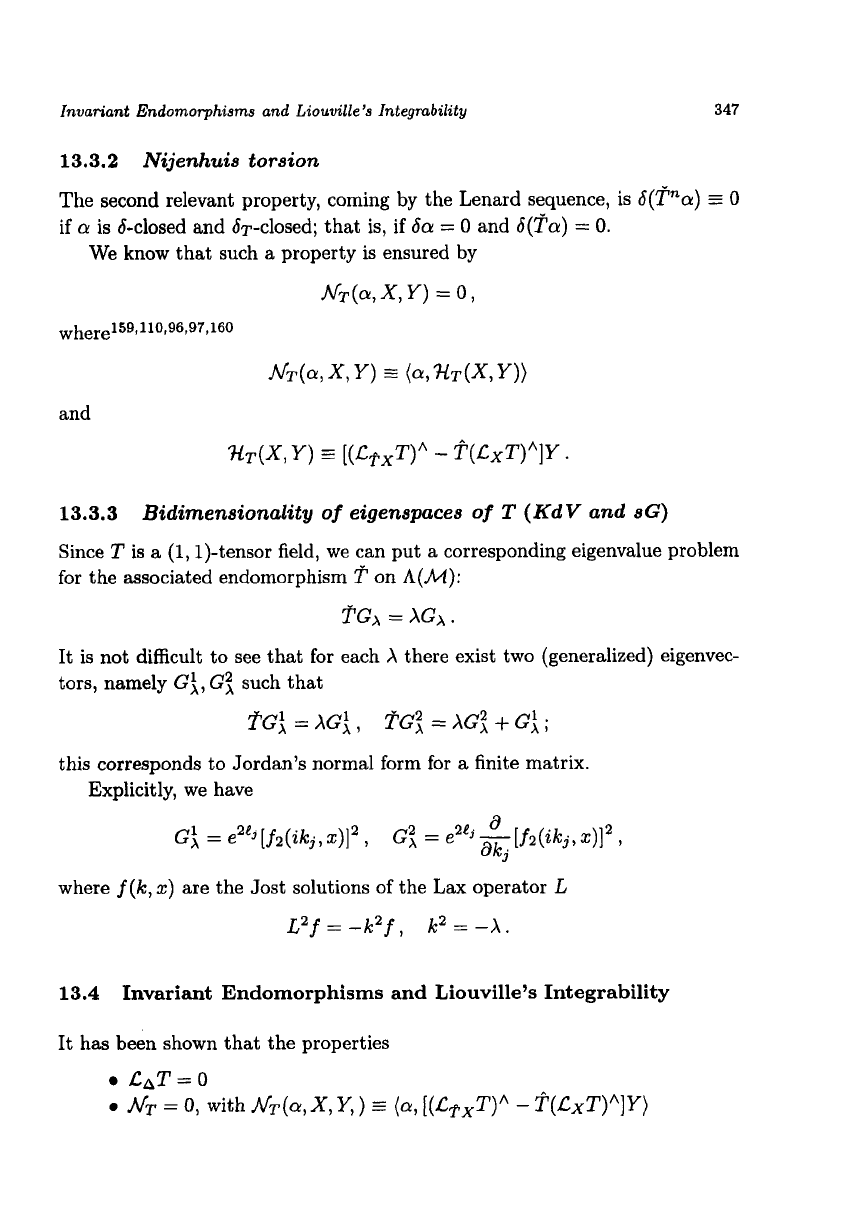
This characterization
of
the dynamics is very suggestive because
of
the
simili-
tude
Dynamics
Invariant
structure
Symplectic
w
a
not degenerate, ske~symmetric, closed
(3
tensor field
Geodesical
I’
a connection
2-
form
Killing
g
a
symmetric, not degenerate
(i)
tensor
field
HamiEtonian
A
a
skewsymmetric
(20)
tensor field,
fu~lling Jacobi a~e~tit~
Liouville
fl
a
volume form
Lax
T
a
(:)
tensor fieEd with vanishing torsion
Invariant Endomorphisms and Liouville
's
Integrability
347
13.3.2
Nijenhuis torsion
The second relevant property, coming by the Lenard sequence, is
6(p"a)
=
0
if
CY
is &closed and dpclosed; that is, if
6a
=
0
and
6(Ta)
=
0.
We know that such
a
property is ensured by
NT(%
x,
Y)
=
0
1
where159,110,96,97,
160
NT(%
x,
Y)
(a,
'flT(X,
Y))
and
'fl~(X,
Y)
5
[(Lq+xT)"
-
f'(LxT)"]Y.
13.3.3
Bidimensionality
of
eigenspaces
of
T
(KdV and
sG)
Since
T
is
a
(1,
1)-tensor field, we can put
a
corresponding eigenvalue problem
for the associated endomorphism
T
on
A(&):
TGx
=
XGx
.
It is not difficult
to
see that for each
X
there exist two (generalized) eigenvec-
tors, namely
G:, G:
such that
TG;
=
XG;
,
TG:
=
AG:
t-
G;
;
this corresponds to Jordan's normal form for
a
finite matrix.
Explicitly, we have
d
G:
=
e2eJ[f2(ikj,z)]2,
G:
=
e2ej-
dkj
[f2(ikj,~)I2
7
where
f(k,
z)
are the Jost solutions
of
the Lax operator
L
L2f
=
-k2f
,
k2
=
-A.
13.4
Invariant Endomorphisms and Liouville's Integrability
It has been shown that the properties
CAT=O
0
N-
=
0,
with
N~(cr,
X,
Y,
)
-=
(a,
[(LpxT)"
-
f'(LxT)"]Y)

348
*
d
=
dim (eigenspaces of
T)
=
2
seem to be verified by all evolution equations integrable by the Inverse Scat-
tering Method.
We recall that in Part
111,
by using the first two properties but assuming
diagonalizability instead of the third pr~perty,~~~~~
a
geometrical integrability
scheme was constructed according to which it was stated that:
A
dynamicat vector field
A
which admits an invariant
(,CAT
=
0)
mixed,
d~agonali~ab~e tensor
field
T,
with vanishing Nijenhuis tensor field
(NT
=
0)
and dou&Z~ degenerat~ eigen~alu~s
X
witho~t stat~ona~ points
(6.4
#
0),
is
sep~ra~le~ integrable and H~m~ltonian~
i.
e.
a
sep~ra~Le completely ~n~~grab~~
~~miltonian s~stem.'O
The proof was performed by showing that
NT
=
0
implies the F'robenius integrability of the eigenspaces
of
T.
0
CAT
=
0
implies the separability of
A
along the eigenmanifolds in
dynamics with
1
degree
of
freedom, each of them with a first integral.
The construction of a symplectic form (actually infinitely many), with re-
spect to which
A
is
a
Hamiltonian vector field, was then easily accomplished.
In spite of the relevance of the diagonalizable case, the third property
is
a
characteristic feature of soliton theories. We want to state here an
a
priori
separability criterion, based on this new spectral hypothesis and worth using
for
soliton equations.
As
far
as
solitonic dynamics is concerned, integrability is
proven without further hypotheses, while for background-radiation dynamics,
a
compact
a
priori
integrability criterion is, to date, lacking.
The present results should naturally lead to the corresponding ones in terms
of
Lax
pairs (these are considered in the context of bundles just based on phase
manifold),a1 once the relationship between them and the above operator, now
only analyticaily understood, will be translated into clear geometrical terms.
We can prove the following integrability criterionE3:
A
dynamical vector field
A
which adm~ts an in~ariant mded tensor field
T,
wit^
vanishing ~z~enh~is tensor
Ni.
and b~dime~siona~ eigenspaces, com~lete~y
separates
in
1
degree of ~ee~o~ d~namics. The ones as~oci~~ed to those degrees
of
freedom, whose corresponding eigenvalues
X
are not stationary, are integrable
and Hamiltonian.
Indeed, denote by
Xi
the generic discrete eigenvalue of
T
and assume that
the continuous spectrum of
T
consists of the real semiaxis
R+.
Then the

Invariant
Endomorphbms
and
Liouvrille
's
Integnrbility
349
vanishing
of
the Nijenhuis torsion
N;.,
associated to
T,
means that for all
cy
E
h(')(M)
and
X,
Y
E
TM,
Ni.(~r,
Y,
X)
(0,
[(LTxT)
-
T(CxT)]Y)
=
0.
(13.15)
According to our assumptions, a basis
of
TM
exists, such that
Now introduce the corresponding dual
basis
of
A1(~)
that
is
a
basis, for which
(13.16)
where
i,
j
==
1,.
,
.
,
n,
6:,:;
=
6;6(k
-
h).
read
The relations corresponding to
Eqs.
(13.16) in terms of differential
1-forms
26,
=
Xi6i
+
72,
21-i
=
xiri,
2~~i(k)
=
kyW)
,
i
=
I,
2,.
.
.
,
n
,
2
=
1,2,
k
E
R+
,
where denotes the transposed
of
9.
As
it will be shown, no more ingredients
are needed to prove the separability in
1
degree of freedom dynamics, and
(except
for
nowhere stationarity
of
the
Xi's)
integrability of the discrete part
of
it. The analysis starts by observing that an explicit transcription of condition

350
General
Structures
(13.15)
is the following:
(13.17)
As
a
matter of fact, it
is
easily seen that Eq.
(13.17)
are equivalent to7
,-a
p,
6~'
=
T~
f,
&$i
A
6.9'
=
y'~(~f
p,
r2,fk)
6~~,(k)
=
0
(13.18)
this implying, by the F'robenius theorem, that without
loss
of generality, the
T'S,
0's
and
y's
can be considered to be closed differential forms,
or
equivalently,
the basis
{ei,
sir
fl,(k),
f2,(k)
1
i
=
172,
*
'
-
9%
,
f
R+)
can be chosen to be
a
holonomic frame.
(LEiXi)ri,
this implying that
On the other hand, the first line of Eq.
(13.17)
is
equivalent to
6Xi
=
P'SX'
=
Xi&Xif
(13.19)
It
particularly means that the
7%
can be chosen
as
equal to
the
GXa's
if,
as
will be assumed, the
Xi's
are nowhere stationary. Furthermore, holonomicity
implies that the set
of
functions
A',
X2,.
.
,,A"
can be completed to form
a
coordinate system!!
(A',
A',
.
.
.
,
An5
cp',
p2,.
.
.
rpn,
$JlYk,
$J21k,
k
E
%+)
in such a
way
that
7Here and in
the
following,
6
denotes
the exterior
derivative and
A
the
usual
wedge
IISome
of
them
may
not
be
global
but
only periodic
ones.
product.

Invariant Endomorphisms and
Liouwille
's
Integrability
351
Just to fix our ideas, the tensor operator
T
acquires the following
canonical
form:
It
is
now easily proved that for such a
TI
the
A
invariance, namely
LAT
=
0,
gives
(a)
(6Ai,
A)
=
0,
6
(b)
-(6cpi,A)
=0,
6$d
6
(&pi,
A)
=
0,
(Xi
-
A')z(6pi)
A)
=
0,
(c)
@m
6
(d)
(13.20)
6
(e)
-(6$'1('),
A)
=
0
,
6pi
from which separability and integrability follow. More specifically,
Eq.
(13.20(a)) means the vanishing of
"A
components" of
A;
Eq.
(13.20(b))
the independence
of
the
'p
components on the
p's;
Eq.
(13.20(c)) the indepen-
dence of the continuous coordinates; and
Eq.
(13.20(d)) just means that each
cp
component can only depend on the corresponding
A.
On the other hand,
Eq.
(13.20(e)) shows that the continuous components cannot depend on dis-
crete variables; and
Eq.
(13.20(f)) that each continuous component can only
be a function of the continuous variables with the same continuous index. The
most general form of
A
is then

352
Geneml
Structures
The dynamical equation then decouples in the following second-order systems
for the continuous degrees
of
freedom
(background radiation dynamics):
dl(k)
=
Al,k($1s(k), $z!(k))),
q)2,(k)
=
A2rk($l,(k), $2>(k))
,
and the following trivially integrable ones:
@
=
Ai(Xi)
,
..
=
0,
for the discrete part (soliton dynamics). Incidentally, the discrete part of the
dynamics is Hamiltonian with respect to
all
symplectic forms
i
for the discrete part
of
the spectrum,
f’s
being arbitrary nonvanishing func-
tions.
Remark
23
The vector field
A
is not supposed to define a Hamiltonian
dynamics.
Its
Hamiltonian structure arises from the supposed bidimensionality
of
eigenspaces
of
T
and the requirement
bX
#
0.
13.5
Recursion
Operators
in Dissipative Dynamics
We have seen that a nonlinear evolution equation
ut
=
A[.];
i.e. the equation
defining integral curves
of
the vector field
A,
is integrable once that
a
mixed
tensor field
T
on
M
exists satisfying the following conditions:
a
T
is
A
invariant; i.e.
LaT
=
0,
a
T
satisfies Nijenhuis condition; i.e.
[LTxT
-
TCxTJY
=
0,
for any two
a
T
is diagonalizable with doubly degenerate eigenvalues
X
without sta-
vector fields
X
and
Y,
tionary points.
These assumptions on
T
not only ensure generic integrability, but also the
existence of symplectic forms with respect to which dynamics is Hamiltonian
and integrability is the usual one in terms of action-angle variables. On the
other hand, there are many physically relevant cases in which the dynamics
is not Hamiltonian, and nevertheless
a
suitable generalization of the above

Recursion
Opemtors
in
Dissipative
Dynamics
353
geometrical scheme could still be useful. The aim of the present example
is to explore the possibility of using invariant mixed tensor fields to analyze
dissipative dynamics. In order to do that,
it
is
natural to begin by removing
only the last condition on
T,
as
it
is the one leading to the existence of constants
of motion, An instance of
a
dynamics which admits an invariant mixed tensor
field
T
which satisfies Nijenhuis condition, but which
is
not diagonalizable
without complexification and whose eigenvalues are trivially constant, is given
by Burgers’ equation.
This equation
is
just
the simplest one combining both nonlinear propaga-
tion and diffusive effects, and it can be used
as
the working example for our
anaIysis,
13.5.1
The
Buqers’
h~~~rc~y
It
is
well-kno~n~~~*~~ that the Burgers’ equation can
be
linearized through the
transformation
(13.21)
vx
u=-
V
where
v
satisfies the heat equation
vt
=
v,,
.
It can easily be shown7’ that the Burgers’ equation
is
a
member
of
a whole
hierarchy of nonlinear evolution equations which linearize, by using the same
transformation
(13.21),
to equations
of
the type
vt
=
D”v,
n
=
1,2
,...
,
(13.22)
with
D
denoting
2
derivative. The even elements of Eq.
(13.22)
obviously
define dissipative dynamics, while the odd ones are integrab~e Hamiltonian
evolution equations with respect to the following symplectic form:
(13.23)
where
