Vij D.R. Handbook of Applied Solid State Spectroscopy

Подождите немного. Документ загружается.

1. Nuclear Magnetic Resonance Spectroscopy

equation (1.30), but it also depends on the time scale and geometry of the

molecular motion [50]. In the limit where many frequency changes occur

during the signal acquisition, W

<< 2S/Z, (the fast exchange limit) [49] a fully

averaged powder pattern with the shape defined by the characteristics of the

molecular motion is observed. The characteristic shape of the spectrum

obtained in the fast exchange regime is dictated by the amplitude and

geometry of the motion. In general, the NMR line shape in the fast exchange

limit can be obtained simply by calculating the effective precession frequency

(average frequency) under the anisotropic spin interaction [7, 49, 51]. This

effective precession frequency is calculated according to an average tensor

that depends on the motion geometry. Because the average tensor is also a

second rank tensor, its anisotropy can also be characterized by two

parameters, namely

and į .

The dependence of

and į on the geometry of the motion is

demonstrated in Figures 1.6a and b, for the cases of chemical shift anisotropy

(I = 1/2 nuclei) and first order quadrupolar (I = 1 nuclei) interactions,

respectively.

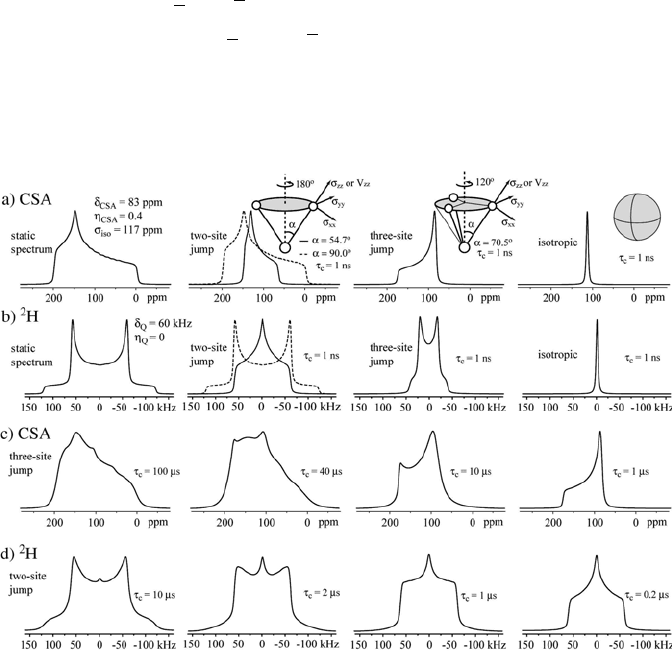
Figure 1.6 Series of powder pattern line shapes simulated for different kinds of molecular

motions: a) I = ½ (CSA) and b) I = 1 (

H quadrupolar). c) Chemical shift anisotropy powder

pattern for three-site jump motion with D = 70.5q (methyl rotations) and different correlation

different correlation

times.

times. d) H powder pattern for two-site jump motion with D = 54.7q and

1.7 Molecular Dynamics and Local Molecular Conformation in Solid Materials

Different spectra are observed according to the motional geometry and

amplitude. Two general features are: i) for any uniaxial motion, such as the

three-site jump shown in Figure 1.6, the resulting average tensor will be

axially symmetric because the rotationally averaged x and y principal values

are equal; ii) for isotropic motions all the three principal values are averaged

out and a single isotropic line is observed in the fast limit [7].

For spin 1/2 systems under the influence of the Zeeman and chemical shift

interactions, the density matrix of the spin system behaves exactly like

macroscopic magnetizations. Hence, if many molecular orientations can exist

simultaneously, the concept of magnetization isochromats can be used for

describing the set of different nuclear spins with the same precession

frequency, i.e., under identical chemical shielding. If we consider the same

kind of chemical species, the spin system in an amorphous or polycrystalline

material can be described by a set of magnetization isochromats, M

, each one

corresponding to a given molecular orientation. Under the influence of

molecular motion during the precession of the transverse magnetization, the

molecular orientations, and consequently the precession frequencies, change

stochastically, producing significant modifications in the resulting NMR

spectrum.

Thus, the time evolution of the transverse magnetization isochromats

depends not only on their individual precession frequencies but also on the

probability of exchange between different isochromats. Assuming a stationary

Markov process, the time evolution of each transverse magnetization

isochromat can be calculated with the following equation [7]:



3



(1.74)



1exp Ȧ 0Mt i t

ªº

 3

¬¼



(1.75)

where

()tm

is a vector where each component is a complex number

representing the isochromat magnetization vector in the xy-plane, M

(t). Ȧ



is a

diagonal matrix, which is composed of the elements

ȦįȦ

jl jl j

= , where Z

the precession frequency of the jth magnetization isochromat and depends on

the molecular orientation according to equation (1.30). The matrix



is the

so-called exchange matrix, composed of off-diagonal elements 3

that

represent the transition rate k from the state l (one given orientation) to the

state j (another orientation), and diagonal elements 3

that represent the total

transition rate from a state j to any other state with a negative sign. Note that

the transition rates are directly associated with the correlation time of the

motion (for example, for a two-site jump k = 1/(2W

)). To obtain the total

magnetization it is necessary to sum over all isochromats. This is done by

multiplying the complex magnetization vector

()tm

by the row vector

1 (1, 1, 1, 1, . .., 1)=

. Using such procedure, from the formal solution of equation

(1.74), the total magnetization vector can be written as [7]

1. Nuclear Magnetic Resonance Spectroscopy

Thus, the resulting magnetization depends on the number of accessible

frequencies and on the exchange rates (correlation times) through the

exchange matrix. To include the initial distribution of magnetization

isochromats, the NMR signal under the influence of the motion is calculated

as the powder averaging of equation (1.75), i.e. [7, 50],









1exp Ȧ 0St i t

ªº

 3

¬¼



(1.76)

Figure 1.6c shows a set of simulated CSA powder patterns as a function of

the correlation time, considering jumps between three molecular sites. For

motional rates, k = 1/3W

, of the order of the chemical shift anisotropy

parameter, G

CSA

, the powder spectrum changes considerably with W

, making it

possible to estimate this parameter from the line shapes. In the fast exchange

regime, W

<< 2S/G

CSA

, the spectrum also has a powder pattern, but with

distinct CSA parameters (

CSA CSA

Ș and į ). Nevertheless, there are some

intrinsic difficulties in obtaining such information from CSA line shapes: i) a

small deviation in the size and orientation of the CSA tensor can produce

significant changes in the average spectrum; ii) different types of motion can

produce identical line shapes; iii) for the case of the

C NMR, which is the

most common nucleus for studying organic systems, line shape analysis can

only be done for systems with one or few different chemical sites, or requires

C isotopic labeling at selected molecular sites. This can actually be

overcome by special 2D NMR techniques that produce 2D spectra containing

high resolution MAS spectra in the first dimension and the corresponding

powder line shapes in the second dimension [52, 53]; and iv) the use of high

power broadband

H decoupling may also be problematic because the

molecular motion can also interfere with the proton decoupling, inducing

spectral line broadening and, sometimes, even precluding the acquisition of

CSA powder line shapes in the intermediate motional regime.

Other NMR interactions, such as quadrupolar or dipolar, can also be used

for probing molecular motions. In fact, in the case of the dipolar interaction,

more traditional NMR methods, e.g., second moment calculations [6, 49] and

studies of the line width as a function of temperature, allow one to obtain

information about the rates and activation energies of molecular motions.

However, site-specific information such as the reorientation angles and

geometry of the motion cannot be obtained by these methods.

This can be done using deuterium (

H) NMR.

H has nuclear spin I = 1, a

gyromagnetic ratio. Because

these reasons, line shape and relaxation

properties of

H resonances are

determined almost exclusively by the first

order quadrupolar interaction.

Besides, X-

H bonds can be arranged to replace

X-

H bonds (X is a low J

nucleus), and

H is a good spin label because its molecular properties are

similar

H. For

H in a X-

H bond the electric field

gradient tensor

almost axially symmetric, K

= 0, and the z-axis of its PAS

roximately along the direction of the X-

H bond. Thus, the spectral

large quadrupolar moment, and a relatively small

points app-

shape

1.7 Molecular Dynamics and Local Molecular Conformation in Solid Materials

produced by single transition between two adjacent

H energy levels is

the same as for the case of spin ½ nuclei with axially symmetric chemical

shift tensor. As a result, the full quadrupolar powder pattern can be calculated

by superimposing two mirror-symmetric powder patterns (one for each

transition) calculated in the same way as for the chemical shift interaction.

However, the effects of the NMR interaction during the rf pulses, which may

be significant for

H NMR [50], have been disregarded in equation (1.76), and

some care must be taken with finite pulse effects when using this

approximation. Figure 1.6d shows a set of simulated powder patterns as a

function of the motional rates for two-site jump motions. In the fast exchange

limit, W

<< 2S/G

, an averaged powder pattern characterized by

Ș and į is

observed. Note that the axial symmetry of the

H quadrupolar interaction was

broken and a motionally averaged spectrum characteristic of a non-axial

interaction was observed.

An experimental problem in the acquisition of

H line shapes is that the

lines are very broad and, consequently, the decay time of the NMR signal is

very short (~ Ps). This makes it very difficult to acquire the first points of the

FID, because it is necessary to wait a few Ps to start the acquisition after the

application of an intense rf pulse (dead-time problem). Thus, to acquire the

signal, it is necessary to use a special pulse sequence, known as solid- or

quadrupolar-echo sequence [50, 54]. The solid-echo pulse sequence is shown

in Figure 1.7a. It produces an echo with maximum intensity at 2W, making it

possible to displace the acquisition to the echo maximum, and thus avoiding

the dead time problem. Typical W values are of the order of 10s of Ps, and

molecular motion occurring with correlation times within this time scale

interferes with the refocusing of the echo, producing a decrease in the signal

intensity. Hence, motions in the intermediate exchange regime can be studied

by accompanying the W dependence of the echo intensity. This is shown in

Figure 1.7b, where the echo intensity, normalized by its rigid limit value

(reduction factor), is plotted as a function of the jump frequency (1/

) for

two-site jump motions occurring with several jump angles. Besides the

reduction in the signal intensity, molecular motions in the intermediate

exchange regime also affect the observed line shapes, and it is crucial to take

these effects into account when performing line shape analysis. This can be

done by adding two extra evolution periods W in equation (1.76):







ȦĲ ȦĲ Ȧ

solid-echo

iiit

St e e e

3r 3 3

½



®¾

¯¿

 



  

(1.77)

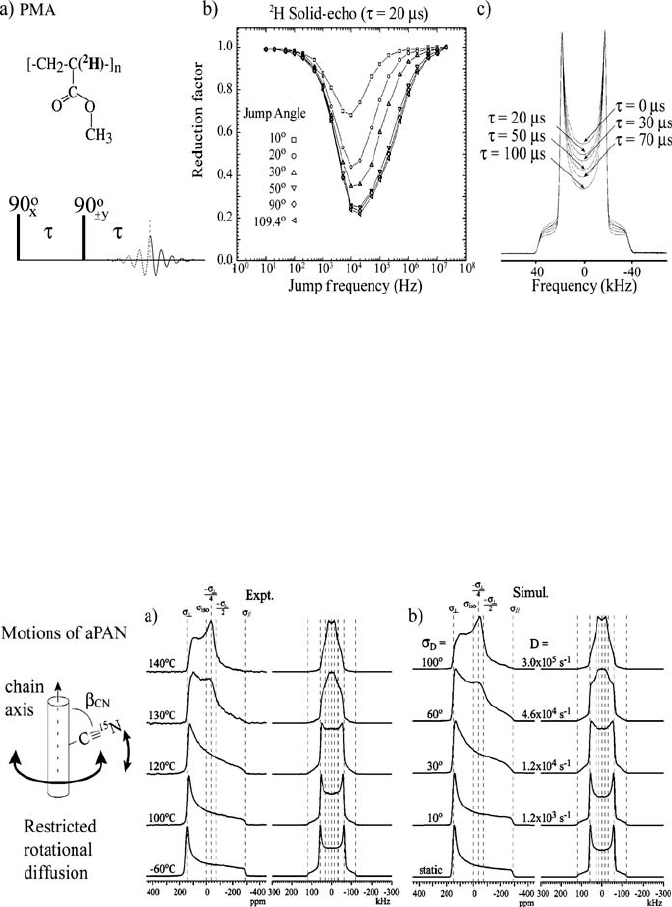
Figure 1.7c presents a set of experimental

H spectra for methyl deuterated

poly(methyl acrylate) (PMA) acquired with the solid-echo pulse sequence

using distinct W values. The effect of the molecular motion in the line shape is

clearly observed.

1. Nuclear Magnetic Resonance Spectroscopy

Figure 1.7 a) Molecular structure of PMA and solid-echo pulse sequence. b) Theoretical

reduction factor of the solid-echo amplitude for a two-site jump between equally populated

sites as a function of the jump frequency and for different jump angles. c) Effect of the solid-

echo delay W in the spectrum of deutered PMA. (Adapted with permission from references [55]

Figure 1.8 shows an experimental example of the use of

H and CSA line

shapes for obtaining information on the molecular motions in solids.

H and

N static spectra were acquired as a function of temperature to elucidate the

molecular mechanism of the relaxation processes observed by dielectric

relaxation in atactic poly(acrylonitrile) ([C

-aPAN) [57].

Figure 1.8 a) Experimental 1D

N CSA (left) and

H (right) spectra of aPAN as a function of

temperature. b) Best-fit simulations of the experimental spectra based on the model of uniaxial

rotational diffusion with distributed restricted amplitudes (see left). The width V

of the

amplitude distribution and the diffusion coefficients D are shown for each case. (Adapted with

The experimental spectra are well reproduced by simulations considering

C, the standard deviation V of the

110°

uniaxial rotation around the main chain with a restricted distribution

of amplitudes. Below

Gaussian

1.7 Molecular Dynamics and Local Molecular Conformation in Solid Materials

distribution of motional amplitudes increases with temperature up to

~30 °C. The small-angle fluctuations rapidly change to larger-amplitude

motions above this temperature; V

increases from 30 to 100° in the

temperature range of 120–140 °C, which correlates with the intensity increase

observed in dielectric measurements.

Experiments involving line shape analysis under magic-angle spinning

have also been performed for

H. These experiments explore the dependence

of the spinning sidebands on the molecular motions. In these cases, the effects

of both molecular and mechanical rotation must be put together in the

simulation of the spectra, which can be done by using appropriated theories

[58]. Similar approaches have also been used for spin ½ systems under the

effect of the chemical shift interaction [32]. However, molecular motions can

also be studied in sideband-free MAS NMR spectra, which is particularly

useful for

C NMR, where multiline spectra are generally observed. This is

so because molecular rotations also interfere with the MAS averaging,

introducing characteristic line broadenings in the MAS line shapes [59, 60].

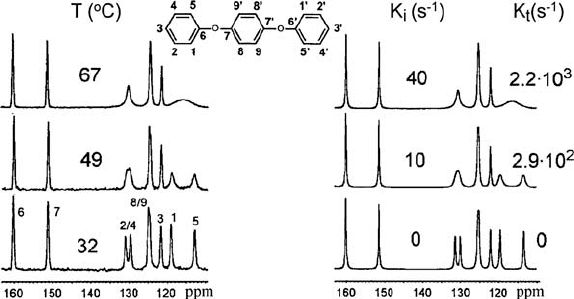
Figure 1.9 shows high resolution

C NMR spectra of 1,4-diphenoxy

benzene (DPB) obtained at different temperatures. A clear broadening of the

2,4,1,5 lines (see assignments in the inset) as a function of temperature is

observed.

Figure 1.9 Experimental (left) and simulated (right)

C MAS spectra of DPB (see molecular

structure in the inset) as a function of temperature at Q

= 5.2 kHz. The simulated spectra were

calculated considering S ring flips with the indicated rate. (Adapted from reference [60].

Since carbons 2,4,1,5 belong to the terminal rings of DPB, this broadening

can be associated to 180q rotations S-flips around the 1,4 axis of the rings. A

slight broadening is also observed for the line attributed to carbons 8 and 9,

showing that a fraction of the inner rings also executes

-flips. The

temperature dependence of the experimental MAS NMR spectra is nicely

reproduced considering S-flips occurring with appropriate motional rates

1. Nuclear Magnetic Resonance Spectroscopy

k = 1/W

, k

for inner rings and k

for terminal rings. From these data the

temperature dependence of the motional rates was obtained and the S-flips

activation energies of the inner and terminal rings of DPB were estimated to

be 90 and 73 kJ/mol, respectively.

The use of

H line shapes is also one of the most traditional methods for

studying molecular dynamics. The

H NMR spectrum of rigid materials is

characterized by broad lines, usually with Gaussian or Lorentzian shapes due

to the strong coupling between the

H nuclei. As in the case of any other

anisotropic interaction, molecular motion can induce the averaging of the

H-

H dipolar interaction, reducing the line widths. Thus, monitoring the line

width as a function of temperature and using appropriate models, information

about correlation time and activation energy of molecular motions can be

obtained [61, 62]. These studies, which can also be performed for other

nuclei, are useful for the characterization of materials like solid polymer

electrolytes, where the line widths and T

relaxation of the charge carriers

(usually Li, Be, Ag, or Cs), provide information about their mobilities [37,

38]. However, due to the lack of spectral resolution in the static or regular

MAS

H spectra, these studies have been limited to cases where no local

NMR methods that are generally called separated-local-field (SFL)

experiments overcomes this limitation.

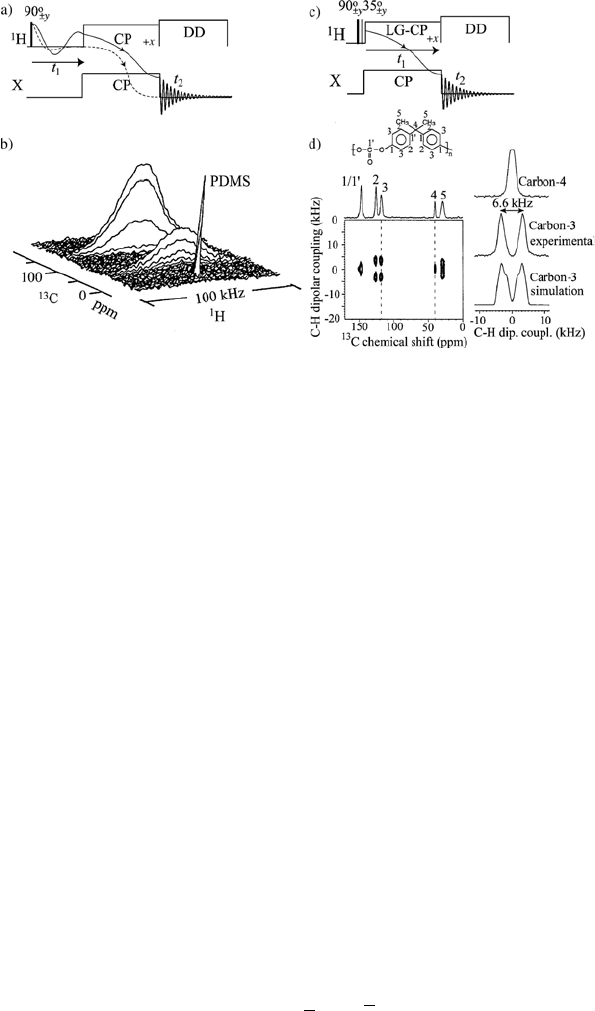
The simplest version of SLF experiments, the so-called wideline

separation (WISE) [63], is derived from the conventional CP-MAS method

by adding an extra time period t

in the CP pulse sequence, as shown in

Figure 1.10a. After the

H S/2 pulse, the

H magnetization evolves under the

action of the chemical shift and homonuclear dipolar interactions and then is

transferred to a low natural abundance X nucleus (usually a spin 1/2 nucleus

such as

Si, or

N). Then, after the CP transfer, the magnetization of the

X nucleus depends upon the evolution of the

H magnetization during t

Incrementing t

according to the usual rules of signal sampling, a 2D time

matrix, S(t

), can be obtained and its 2D Fourier transform provides a 2D

spectrum, S(Z

), that contains the high resolution MAS spectra of the X

nuclei along Z

, and the corresponding

H wideline spectra along Z

. This

experiment is very convenient for distinguishing regions of the material with

different mobilities. Figure 1.10b shows an example where the WISE

experiment was applied to study the phase separation in the block copolymer

polystyrene (PS)-b-poly(methyl siloxane) (PDMS). It is possible to observe

that while the PS phase of the material is rigid (broad lines) the PDMS phase

is mobile (narrow line), despite the high chemical connectivity and spatial

proximity (~20 nm) between the phases. The WISE experiment was also

modified to obtain information about the domain sizes of each phase [63].

information about molecular motions were necessary. A class of two-dimensional

1.7 Molecular Dynamics and Local Molecular Conformation in Solid Materials

Figure 1.10 a) Pulse sequence for 2D WISE experiments. b) Application of the WISE method

for identifying rigid and mobile phases in the block copolymer PS-b-PDMS. c) Pulse sequence

for 2D LG-CP experiments. The extra 35q pulse is applied because the precession plane of the

H magnetization under LG irradiation is 35q tilted relative to the transverse plane. (Adapted

The main problem of using WISE experiments for probing localized

motions is related to the so-called spin-diffusion phenomenom [6, 49]. Strong

homonuclear dipolar couplings induce transfer of z magnetization between the

coupled spins (spin-diffusion or spin-exchange) with or without rf irradiation

[6, 7, 49]. Hence, due to the high natural abundance and the strong

H-

dipolar coupling, the

H magnetization diffusion (

H spin-diffusion) during

the cross-polarization period is very effective [63, 64]. Some approaches were

used to avoid this problem for the WISE experiments [65]. However, a similar

experiment, but much less prone to spin-diffusion, was also proposed [43]. In

this experiment, the effect of spin-diffusion is minimized by replacing the CW

spin-lock pulse by the Lee-Goldburg homonuclear decoupling sequence. In

addition, instead of using an extra evolution period t

in the pulse sequence,

the CP period is incremented to produce the 2D signal (2D LG-CP

experiment); see Figure 1.10c. The result is a 2D spectrum that contains the

correlation between the X nucleus chemical shift, and the X-

H dipolar

interaction scaled by a factor of 0.577 due to the LG irradiation [66, 67].

Specifically for

C, the Z

spectral dimension contains the high resolution

NMR spectrum, and the corresponding scaled

C-

H dipolar powder

spectrum appears in the Z

dimension. As in the case of

H line shape, the

molecular motion breaks the axial symmetry of the dipolar interaction and in

the fast exchange limit the average

Ș and į reflect the geometry of the

motion [43]. An example of local motion studied by 2D LG-CP is the S ring-

flip of polycarbonate. The two phenylene rings in each repeat unit undergo

S-flips superimposed with wobbling motions with a distribution of amplitudes

1. Nuclear Magnetic Resonance Spectroscopy

around the equilibrium positions. Figure 1.10d shows the 2D

C-

H LG-CP

spectrum of polycarbonate and several cross sections along the Z

axis. A

1D LG-CP MAS spectrum is shown at the top of the 2D spectrum, where the

five resolved

C signals are assigned. The C-H cross-section of the Carbon 4

signal, which results from the aliphatic quaternary carbon, does not exhibit

any resolved splitting, as expected for a carbon site that is not directly bonded

to any protons. In contrast, the aromatic Carbon-3 exhibits a splitting of 6.6

kHz and a smooth line shape devoid of sharp features. The spectral simulation

for this group is also shown [43]. The experimental spectrum is reproduced

using a model where both aromatic rings undergo S-ringflips and wobble

around the equilibrium ring positions with an average amplitude of ~ 20q.

Exchange NMR [7, 68] has become one of the premier tools for

characterizing segmental motions in organic solids. In these experiments

molecular reorientations on the milliseconds-to-seconds time scale (that do

not change the powder spectrum) are observed in terms of changes of

orientation-dependent NMR frequencies. They permit obtaining correlation

times and their distributions [69, 70], reorientation angle distributions [69,71],

orienta-tional memory and rate memory [72], verifying the existence of

dynamic heterogeneities [73], and determining their sizes [74]. Due to the

variety of exchange experiments that can be performed [75–82], some general

ideas and applications of standard 2D-exchange experiments will be discussed

first and then some extensions will be addressed together with the

corresponding experimental examples. For the sake of simplicity, the simpler

case of spin I = ½ will be discussed first and then extended for the case of

spin I = 1.

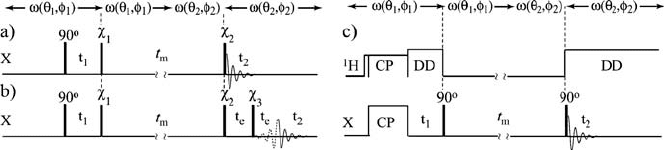
Figure 1.11a shows a general pulse sequence for the static 2D-exchange

experiment. The first S/2 pulse (or cross-polarization) excites the transverse

magnetization, which evolves under the chemical shift interaction during the

first period t

. After t

, a second S/2 pulse stores one component of the

magnetization along the z direction, where it remains during the long mixing

time (t

Figure 1.11 Pulse sequence used for 2D-exchange NMR experiment. a) Basic scheme using a

single 90q excitation pulse. b) With single 90q excitation pulse and echo acquisition. c) Using a

cross-polarization and

H decoupling.

and

represent rf pulses with general flip angles.

1.7.2 Two-Dimensional Exchange NMR Experiments

1.7 Molecular Dynamics and Local Molecular Conformation in Solid Materials

The remaining transverse magnetization during t

is eliminated by cycling

the phases of the S/2 pulses. A second S/2 (read-out) pulse is applied after t

and then the magnetization evolves under the influence of the chemical shift

interaction during t

. Hence, the detected signal intensity S(t

) depends on

both evolution times, t

and t

, and on the mixing time t

. The 2D Fourier

transformation [28, 29] of the data produces a 2D spectrum that contains the

intensity distribution as a function of the NMR frequencies experienced by the

magnetization during t

) and t

), and also on the molecular motion that

occurred during t

, S(Z

If no motions have occurred during t

, the orientation-dependent

frequencies are equal before and after the mixing time and the resulting 2D

spectrum is purely diagonal. In contrast, if segmental reorientation occurs

during t

, the orientation-dependent frequencies change and off-diagonal

intensities are observed. Moreover, the change in the NMR frequency for each

molecular site is dictated by the molecular motion occurring during t

and the

spectral intensity S(Z

) depends on the time scale and geometry of the

molecular reorientations. While small-angle motions induce little

modifications in the NMR frequencies and only near diagonal intensity is

observed, high angle rotations produce large frequency changes and the

corresponding 2D spectrum contains intensities in a broad spectral range.

Actually, if the correlation time of the motion is much longer than the

evolution times t

and t

(slow motion regime), the magnetization that gives

rise to a certain intensity at a frequency point (Z

) depends on the fractional

population of spins that precess with Z

during t

, P

(0), and on the conditional

probability that it changes its orientation in such a way that it precesses with

during t

, P

) [7, 69, 83]:











ȦȦ

12m m

,, 0

it it

ij ij j

Mttt PtP e e .

(1.78)

Transverse relaxation during t

and longitudinal relaxation during t

were

disregarded. Because molecular motion can be generally considered a

stationary Markov process, P

(0) and P

) can be properly calculated from a

master equation [7, 69, 83], resulting in a 2D NMR signal given by







ȦȦ

12m

detect mix evolve

,, 1 01

it it

St t t e e e P







(1.79)

The elements of





represent the a priori probabilities P

of finding a

segment in a given orientation, i.e.,





is another representation of the

initial magnetization vector

(0)m

, which accounts for the initial distribution of

magnetization isochromats. The exchange matrix



and the frequency matrix



have the same definitions as before.

The 2D spectrum can be interpreted as a map of reorientation probabilities,

i.e., it represents the probability density of finding a segment with frequency

before t

and Z

after t

. Thus, because these probabilities are dictated by

the correlation time and the geometry of the motions, the 2D-exchange pattern