Vij D.R. Handbook of Applied Solid State Spectroscopy
Подождите немного. Документ загружается.

1. Nuclear Magnetic Resonance Spectroscopy
4
The dipolar Hamiltonian can also be represented by
D
11
NN
jk
jk
HIDI
¦¦
GG
,
(1.11)
where
D
is defined by
2
12
3
3
ȖȖ
m n m jk jk n jk jk
mn
jk
ee er r er r
D
r
GG GG G GG G
=
(1.12)
and
1
e
G
,
2
e
G
, and
3
e
G
are the basis vectors of the coordinate system in which the
tensor
D
is represented.
Taking only two spins
1
I and
2
I and expressing
1
x
I ,
2
x
I ,
1y
I , and
2 y
I in
terms of the raising and lowering operators
1
I
,
1
I
,
2
I
, and
2
I
, and
expressing the Cartesian coordinates in terms of spherical coordinates, we can
write the dipolar Hamiltonian in a form that is more convenient:
2
12
3
ȖȖ
D
H ABCDEF
r
=
,
(1.13)
where
2
12
2
12 12
12 12
13cosș
1
13cosș
4
3
sinșcosș
2
zz
i
zz
AII
BIIII
CIIII e
I
12 12
22
12
22
12
3
sinșcosș
2
3
sin ș
4
3
sin ș
4
i
zz
i
i
DIIII e
EII e
FII e
I
I
I
(1.14)
and T
is the angle between the distance vector r
G
and
0
B
G
.
While the Zeeman interaction corresponds to the interaction of a nuclear
the first two elements A and B:
2
12
D
3
ȖȖ
HAB
r
=
.
(1.15)
If the dipolar interaction occurs between unlike spins (heteronuclear di-
polar interaction), only the term A is necessary for the first order perturbation
calculations:
2
2
12
D12
3
ȖȖ
3cos ș 1
zz
HII
r
=
.
(1.16)
2
D12
35
11
3
ȖȖ
NN
jjk kjk
jk
jk
jk jk
kj
Ir Ir
II
H
rr
z
ªº
«»
«»
¬¼
¦¦
GG
GG
GG
=
.
(1.10)
G
G
moment with magnetic fields of the order 1–10 teslas, the dipolar interaction
corresponds to an interaction with a field of about 1 gauss. Therefore, the dipolar
the Zeeman interaction and the effective dipolar Hamiltonian can be truncated to
interaction can be also treated as a first order perturbation with respect to

1.3 Nuclear Spin Interactions in Solids
5
DE
are, respectively, the electric quadrupule moment, the
electrical potential at the site of the nucleus.
1.3.1 General Structure of the Internal Hamiltonians
The internal Hamiltonians have a common structure [1]. They can be
expressed as:
33
Į Įȕ ȕ Įȕ ȕĮ
Į,ȕ 1 Į,ȕ 1
HC IRA C RT
¦¦
,
(1.18)
where
C is a constant equal to Ȗ= ,
2
12
ȖȖ= , and
621eQ I I = , respectively,
for chemical shift, dipolar, and quadrupolar interactions. As already observed,
Įȕ
R is a second rank Cartesian tensor whose components depend on the
specific characteristics of each interaction. Finally,
ȕĮ
T are dyadic products
constructed from two vectors, one of which is always a nuclear spin vector
and another that can be the same nuclear spin vector (homonuclear dipolar or
quadrupolar interactions), another nuclear spin vector (heteronuclear dipolar
interaction), or the external magnetic field (chemical shift).
The second rank Cartesian tensor
R
can be decomposed into three
components [1]:
012
RR R R
.
(1.19)
The first term is the trace of the tensor
R
:
0
1
Tr 1
3
RR
(1.20)
and represents the isotropic contribution of each interaction, where
1
is the
identity tensor. In the case of internal Hamiltonians, it is equal to zero for
dipolar and quadrupolar interactions and equal to
iso
V
for the isotropic
chemical shift.
The components of the traceless antisymmetric
1
R
and symmetric
2
R
constituents are given by
1
Įȕ Įȕ ȕĮ
1
2
RRR
and
2
Įȕ Įȕ ȕĮ ĮĮ
11
23
RRR R
¦
.
(1.21)
Quadrupolar Interaction. When the nuclei have spin > ½, they possess also
the electric quadrupole moment that interact with the electric field gradients
present in the spin molecular surroundings [1, 7]. This Hamiltonian can be
written in the following form:
3
2
Q Įȕ Į ȕ ȕ Į Įȕ
Į,ȕ 1
3
621 2 621
eQ eQ
HVIIIIIIVI
II II
ªº
«»
¬¼
¦
GG
==
G
,
(1.17)
where eQ, I, and V
nuclear spin quantum number, and the second spatial (D,E) derivative of the
1. Nuclear Magnetic Resonance Spectroscopy
6
1
IJ
3
x
xyyzz
RRR (isotropic term – tensor trace),
(1.22)
įIJ
zz
R (anisotropy factor),
(1.23)
Ș
į
yy xx
RR
(asymmetry parameter).
(1.24)
The tensor trace
IJ is different from zero only for the chemical shift
interaction and in this case is named isotropic chemical shift. For the dipolar
interaction the anisotropy factor is
3
į 1 r and the asymmetry parameter is
Ș 0
.
In terms of
ĮĮ
R and the new parameters IJ , į , and Ș , we have in the PAS:
00 100 1Ș 200
00IJ 010 į 01Ș 20
00 001 0 0 1
xx
yy
zz
R
R PAS R
R
ª
º
ªº
ªº
«
»
«»
«»
«
»
«»
«»
«
»
«»
«»
¬¼
¬¼
¬
¼
.
(1.25)
1.3.2 Behavior of Internal Hamiltonians under Rotations
All the techniques used for high resolution solid state NMR to average out
nuclear spin interactions rely on rotations of the internal Hamiltonians [1].
Due to this, it is worthwhile to represent the Hamiltonians using irreducible
spherical tensor operators, denoted by
,lm
T and
,lm
R , which can be obtained
from the respective Cartesian tensors
Įȕ
R and
Įȕ
T . Using these operators we
can rewrite the general Hamiltonian in the following way:
,,
1
l
m
lm lm
lm l
HC R T
¦¦
.
(1.26)
Because we are dealing with second rank Cartesian tensors
R
, only the
,lm
R with 0, 2l are different from zero. If we consider that the
R
tensors are
represented in their principal axes systems, only components with
0, 2m r
are non-zero. Denoting the components of the irreducible spherical tensor
operators
,lm
,lm
, we obtain the following
relations:
0,0
ȡIJ ,
2,0
ȡ 32į , and
2, 2
ȡȘį2
r
. Normally, the
,lm
T elements
are expressed in the laboratory frame. To express the
,lm
R elements also in the
laboratory frame we have to make use of the Wigner rotation matrices
',
0,ș,
l
mm
I:
only the isotropic and traceless symmetric parts are measurable by NMR.
Therefore, we will disregard any eventual antisymmetric constituents and
treat the second rank Cartesian tensor
R
as symmetric.
For each interaction there exists a principal axes system (PAS), where their
respective tensors
R
become diagonal. The diagonal elements in the PAS are
called principal components:
x
x
R ,
yy
R , and
zz
R . It is often convenient to
introduce instead of the three parameters
ĮĮ
R , three new parameters:
Some interactions contain, in principle, all the three components. However,
R in their respective PAS s by ȡ
’
1.3 Nuclear Spin Interactions in Solids
7
was chosen equal to zero because the Hamiltonian is invariant under rotations
around
0
B
G
.
Using this procedure and getting only the first order perturbation terms
(
0m ) of the general secular Hamiltonian, we obtain [12]:
2
sec 2
0,0 2,0
33cosș 11
3 IJį Șsin școs 2
222
HCTCT
ªº
I
«»
¬¼
.
(1.28)
The
2,0
T spherical tensor components are summarized in Table 1.1 for each
spin interaction. Because dipolar and quadrupolar tensor traces are equal to
zero, the only important
0,0
T component is that of the chemical shift
interaction. For this interaction,
0,0 0
3
z
TIB .
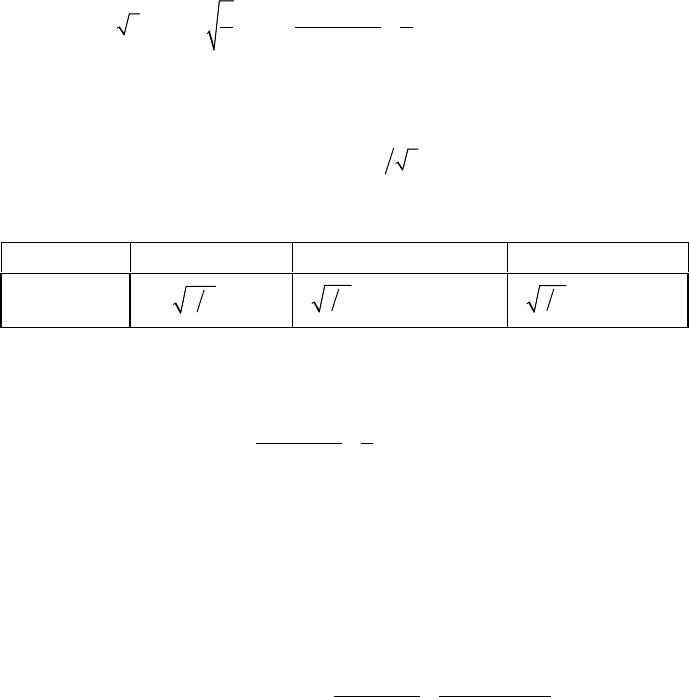
Table 1.1 Irreducible tensor components
2,0
T .
Interaction Chemical Shift Dipolar Quadrupolar
2,0
T
0
23
z
I
B
12 1 2
16 3
zz
I
III
GG
2
16 3
z
I
II
GG
From Table 1.1 and equation (1.28) we obtain the secular chemical shift
Hamiltonian:
2
sec 2
cs 0
3cos ș 11
Ȧıį Șsin ș cos2
22
z
HI
½
ªº
°°
I
®¾
«»
°
¬¼
¯¿
=
.
(1.29)
Similar Hamiltonians can be obtained for the dipolar and quadrupolar
interactions.
One common property of the Hamiltonians shown in equations (1.28) and
(1.29) is that all of them are diagonal in the I
z
basis and have the same angular
dependence. Thus, the transition energies and, consequently, the anisotropic
part of the NMR frequencies, can be written in a general form for all spin
interactions as
22
iso aniso iso
3cos ș 1 Șsin școs 2
Ȧș, ȦȦș, Ȧį
22
§·
I
I I
¨¸
©¹
.
(1.30)
The anisotropy of the internal interaction in the solid state makes NMR a
unique tool for probing molecular orientation, local structure, relative
conformation between sites, or molecular motion. Many of the solid state
NMR techniques used for studying these properties are based on the
anisotropy of the internal interactions, making this issue crucial for
understanding solid state NMR.
,',,
'
0,ș, ȡ
l
l
lm m m lm
ml
R
I
¦
,
(1.27)
where
ș and
I
are the Euler angles by which the laboratory frame can be
brought into coincidence with the principal axes system. The first Euler angle
'

1. Nuclear Magnetic Resonance Spectroscopy
8
1.4 QUANTUM MECHANICAL CALCULATIONS
In elementary quantum mechanics, it is customary to study systems whose
state is perfectly known (pure state) in terms of wave functions. For nuclear
spins in magnetic fields, where the state of the system is not perfectly known
terms of the density operator
ȡ t . Due to this, the density operator
description of spin systems, which combines quantum mechanics and
statistical mechanics, will be employed, and it will be shown how the
quantum mechanical time evolutions can be calculated for both simple and
advanced NMR experiments [7, 10].
The density operator describes the state of the system, while the
Hamiltonian represents the interactions, which act to change the state of the
system [7]. They are related through the von Neumann equation:
1
ȡ ,ȡ
d
tHtt
dt i
ª º
¬¼
=
.
(1.31)
If
A
is an observable component, the mean value of
A
at the instant t is
obtained from the expression
^`
Tr ȡ
A
ttA .
(1.32)
If
Ht and
ȡ t commute, the system characterized by ȡ does not
change with time. If they do not commute and
H is time-independent
(
0Htww ), a formal solution for the von Neumann equation is given by:
ȡȡ0
iH t iH t
te e
==
.
(1.33)
The operator
iH t
Ut e
=
,
(1.34)
which compels the density operator to change in time, is often termed
propagator.
In the case of NMR experiments, the density operator evolves under
different time-independent Hamiltonians during distinct intervals of time, and
we can easily calculate the time evolution of
ȡ as follows:
33 33
2 2 11 11 2 2
1
2
3
ȡ ... ȡ 0 ...
nn nn
iH t iH t iH t iH t
iH t iH t iH t iH t
event
event
event
event n
te e e e e e e e
½
°°
ªº
°°
«»
§·
°°
°°
«»
¨¸
®¾
«»
¨¸
°°
©¹
«»
°°
«»
¬¼
°°
°°
¯¿
== ==
== ==
.
(1.35)
This equation will be used below to introduce the NMR concepts in a fully
quantum mechanical way, allowing the connection to several valid classical
pictures of the precessing magnets, as used in several introductory NMR texts.
(statistical mixture of states), it is more convenient to describe it in

1.4 Quantum Mechanical Calculations
9
1.4.1 Quantum Mechanical Description of NMR
1.4.1.1 System in Thermodynamic Equilibrium
The first example to be analyzed is that of a system in thermodynamic
equilibrium with a reservoir at absolute temperature
T . It can be shown that
its density operator, under these conditions, is given by [10]:
ȡ
H
kT
e
Z
,
(1.36)
where
H is the Hamiltonian operator of the system, k is the Boltzmann
constant, and
Z
is the partition function, which makes the trace of ȡ equal
to 1:
^`
Tr
kT
Ze
+
.
(1.37)
In NMR, the dominant interaction for the Hamiltonian is the Zeeman
interaction [7]
z0 0z
Ȗ I Ȧ I
z
HB ==.
(1.38)
For temperatures above 1 K and under magnetic fields currently available,
we always have
0
Ȧ 1kT =
(1.39)
so that we obtain the high temperature approximation:
0z
Ȧ I
ȡ 1
z
HkT
eZ
kT
§·
|
¨¸
©¹
=
.
(1.40)
The identity operator
1
commutes with any Hamiltonian H and is time-
independent. Due to this, only the partial density operator
0z
z
Ȧ I
ȡĮI
kT
'
=
(1.41)
evolves under equation (1.31). Therefore, for our subsequent discussions we
will consider it as the density operator (
ȡȡ{'
).
In order to further simplify our next calculations, we are also going to
consider
Į 1, so that the thermodynamic equilibrium density operator will
be represented only by
z
I . For all the NMR applications, we will have as the
equilibrium state
eq
ȡ
z
I| .
(1.42)
We are now equipped with the necessary physical and mathematical
background to start discussing NMR in quantum mechanical terms [4].
1.4.1.2 Zeeman Interaction
As already discussed, the Hamiltonian for the Zeeman interaction is
expressed by
z0 0 0
µ ȖȦ
zz
HB BII
G
G
==
.
(1.43)
1. Nuclear Magnetic Resonance Spectroscopy
10
When the magnetic field
0
B
G
is static, the Hamiltonian is time-independent,
and we can analyze the spin motion according to equations (1.33) and (1.34).
Considering that in equilibrium
ȡ 0
z
I and that the propagator is given by:
00
ȖȦ
z
zz
iH t
iBIt i It
Ut e e e
=
,
(1.44)
the matrix density at the instant
t is
0000
ȦȦȦȦ
z
ȡȡ0I
zzzz
iIt iIt iIt iIt
te e e e
.
(1.45)
Since we are interested in the evolution of the magnetization, which is
proportional to the angular momentum
I , we are going to calculate the
expectation values for
z
I ,
x
I , and
y
I as a function of time using the
expression
^`
^`
00
ȦȦ
ĮĮ
Tr ȡ 0Tr ȡ 0
zz
iIt iIt
It It e Ie
D
, where D = x, y, z.
z
It is given by
^`
^`
^`
^`
0
00
ȦȦ
z
ȦȦ
Tr ȡ 0Tr I ȡ 0
Tr ȡ 0Trȡ 00,
zz
zz
iIt iIt
zz
iIt iIt
zzz
It It e e
ee I I I
(1.46)
i.e., the z-component of the angular momentum is time-independent.
Instead of calculating separately the expectation values for
x
I and
y
I , we
will compute the expectation values for
+x y
IIiI and
-
x
y
IIiI :
^`
^`
00
ȦȦ
±±
Tr ȡ 0Tr I ȡ 0
zz
iIt iIt
±
It It e e
.
(1.47)
From 1.47 it is straightforward to obtain [4]:
0
0
Ȧ
++
Ȧ
0
0.
it
it
Ite I
Ite I
(1.48)
Separating in the components
x
It and
y
It:
00
00
0cosȦ 0sinȦ
0sinȦ 0cosȦ .
xx y
yx y
It I t I t
It I t I t
(1.49)
The oscillations of
x
I and
y
I correspond to rotations around the magnetic
field with Larmor frequency
00
ȦȖB . In conclusion, the time-dependence
of the expectation value of the total angular momentum ¢I² corresponds to a
precession around the static magnetic field. Another way of expressing this
important result is through the precession equation
00
ˆ
ȖȦ
d
IBzI I
dt
GGG
G
.
(1.50)
Considering that
µ Ȗ I
G
G
= and that the bulk magnetization is given by
µ
i
i
M
¦
G
G
, we can also write the following precession equations:
00
µ Ȧ µand Ȧ
dd
MM
dt dt
GG
GG
GG
.
(1.51)
G
0
1.4 Quantum Mechanical Calculations
11
These precession equations obtained with quantum mechanical arguments
correspond to the normal classical view presented in basic books, which are
very useful for understanding the fundamental aspects of NMR experiments.
1.4.1.3 Rotating Frame
Since the Larmor precession is a constant frequency factor for all the
interactions that affect the NMR spectra, we usually eliminate it by moving to
a frame that rotates around the z-axis with a frequency
0
ȦȦ
R
| [7]. This new
reference system is called the rotating frame (
'x ,
'y
, 'zz ).
Formally, any density operator
00
ȦȦ
ȡȡ0
zz
itI itI
te e
observed from the
frame rotating with frequency
R
Ȧ can be written as
00
00
ȦȦ
ȦȦȦ Ȧ
R
ȦȦ ȦȦ
ȡȡ ȡ0
ȡ 0
zz
R
zRzRz Rz
Rz Rz
itI itI
itI itI itI itI
itI itI
te te e e e e
ee
(1.52)
and, considering the “ideal” or “resonant” case where
0
ȦȦ
R
:
R
ȡȡ0t ,
(1.53)
where the spins no longer precess around the magnetic field. Henceforth, we
will use the rotating frame to analyze the effects of the internal interactions
and rf fields on the spin system.
1.4.1.4 The Effects of Radio Frequency Pulses in the Rotating Frame
Radio frequency (rf) fields
rf
B
G
are of central importance in pulsed NMR and
fundamental for the implementation of advanced experiments [7]. They are
generated by the probe coil, oscillate perpendicularly to the static magnetic
field with frequencies close to the Larmor frequency (
rf 0
ȦȖB| ), and have
amplitude B
1
of the order of 10 G. For the typical magnetic fields used in
modern NMR spectrometers (~ 10 T), the Larmor frequencies for
13
C and
1
H
are approximately 100 and 400 MHz, respectively, which are included in the
radio frequency range. Due to this, we call these oscillating fields rf fields.
Being a time-dependent perturbation with energy close to the separation
between adjacent Zeeman energy levels, an rf field is responsible for the
excitation of the nuclei. Normally, we express the rf fields as
rf 1 rf rf
ˆˆ
cos Ȧ sin ȦBBt t tx t ty
ªº
II
¬¼
G
(1.54)
and for special excitation procedures (transition-selective, multitransition, or
multiple quantum excitations) the rf field may be amplitude-, frequency-,
and/or phase-modulated, through the manipulation of the parameters
1
Bt,
rf
Ȧ , and/or
tI , respectively. In the rotating frame, considering that
rf 0
ȦȖB , they can be represented by the simpler equation:
1. Nuclear Magnetic Resonance Spectroscopy
12
rf 1
ˆˆ
cos ' sin 'BBt tx ty
ªº
II
¬¼
G
.
(1.55)
The phase
I
will define the direction of the rf field in the rotating frame
along the
''xy
plane. For example, for
I
0°, 90°, 180°, and 270°, we will
have the following respective arbitrary orientations for
1
B : 'x ,
'y
, 'x , and
'y
. As it is going to be shown in the applications of NMR, the possibility of
applying the rf fields along different directions in the rotating frame is of
fundamental importance for manipulating the spins during complex pulse
sequences (phase cycling). Taking into consideration the precession equations
(equations (1.47) and (1.49)), and that in the rotating frame the only external
magnetic field observed by the nuclei is the rf field, the magnetization will
precess around
rf
B
G
. The precession or flip angle can be set directly by
adjusting the duration and/or the amplitude of the rf irradiation. Usually, we
adjust the duration in order to apply rf pulses to flip the magnetization with
angles defined by
1p
ȖBt ,
(1.56)
which are normally chosen to be
2
S
or
S
.
In terms of quantum mechanics the rf Hamiltonian in the rotating frame is
given by
rf Į 1Į
ȖHIB = ,
(1.57)
where
Į can be, for example, 'x ,
'y
, 'x , and
'y
, identifying the
1
B field
orientation and the component of the angular momentum in the rotating
frame.
Now, we can calculate the evolution of the density matrix under the rf
pulse for any initial state
´
ȡ 0
a
I [4]:
1ĮĮp1ĮĮp
ȖȖI
Į'
ȡ I
iB It iB t
te e
.
(1.58)
The effect of the rf pulse is to introduce a rotation of the operator
´a
I
around the rf field
1
B
D
G
by a flip angle
Į 1Į p
ȖBt . For example, if we apply the
rf pulse on
z
I with the rf field along the 'x direction of the rotating frame
1'
x
B
during
p
t so that
'
ʌ 2
x
, we will have as a result a ʌ 2 – rotation of
z
I
around 'x bringing it to the
'y
direction, according to the left-hand rule.
Using the density matrix evolution we have:
1' 'p 1' 'p
pz
''
ȡ I
cos ʌ 2sinʌ 2.
xx xx
iBIt iBIt
zyy
te e
III
JJ
==
(1.59)
If we duplicate
p
t or
1'
x
B we will have
'
ʌ
x
, or the spin inversion:
p
ȡ
z
tI . We can generalize these results stating that in the rotating frame
the effect of the rf pulses can be represented by rotation matrices around
'x ,
'y
, 'x , and
'y
, or any oblique axis, given by the left-hand rule.
M
M
M
M

1.5 High Resolution Solid State NMR Methods
13
1.4.2 The NMR Signal – Zeeman Interaction
After applying, for example, a ʌ 2 pulse on
z
I , the operator
y
I will evolve
only under the static magnetic field
0
ˆ
Bz. This evolution, from the point of
view of an observer located in the laboratory frame, will be given by [4]
00
ȖȖ
00
ȡ
cos Ȧ sin Ȧ .
zz
iBIt iBIt
y
yx
te Ie
ItIt
(1.60)
This means that, as discussed before for the precession of the equilibrium
magnetization, the angular momentum operator will perform a precession
motion around
0
ˆ
Bz in the
xy
-plane. Considering that the magnetic moment of
the nuclear spin is given by
µ Ȗ I
G
G
=
and that for a large number of identical
spins the total magnetization is
µ
i
i
M
¦
G
G
, we can extend the precession
motion for the expected transverse magnetization:
00
cos Ȧ sin Ȧ
xy y x
Mt M t M t .
(1.61)
Normally, the same coil that generates the rf field is used for detecting the
NMR signal. In this case the precession of the bulk magnetization generates,
through Faraday’s law, an electromotive force. This alternating voltage
oscillates with the Larmor frequency and is denoted free induction decay
(FID). When the spins are evolving under the internal Hamiltonians, they will
produce signals with several different frequencies, which are detected
simultaneously by the coil as a superposition of all the individual frequency
FIDs. To separate all the frequencies, the FID is Fourier-transformed,
resulting, for example, in the spectra shown in Figure 1.4.
1.5 HIGH RESOLUTION SOLID STATE NMR METHODS
To introduce the simplest high resolution solid state NMR methods we are
going to restrict our discussion to organic samples containing only
13
C and
1
H
nuclei.
In liquid samples the molecules typically execute fast and random motions
and the only remaining contribution for the NMR spectra
comes from the
resonance lines. Given that these motions are restricted in solid samples,
the anisotropic components are not averaged or are only partially averaged. Since
the resonance of each nucleus depends on the local field at its site, and the
intensity of the these local fields depends on the orientation of the neighboring
a considerable spread in the resonance frequencies resulting in broad spectra,
so that the anisotropic components of the above discussed spin interactions
are averaged out
isotropic terms, resulting in spectra composed of very well
defined
nuclei, of these electron clouds, and of the electric gradient fields, there will be
