Vij D.R. Handbook of Applied Solid State Spectroscopy

Подождите немного. Документ загружается.

CHAPTER 6

CRYSTAL FIELD SPECTROSCOPY

Albert Furrer and Andrew Podlesnyak

Laboratory for Neutron Scattering, ETH Zurich & PSI Villigen, CH-5232 Villigen PSI,

Switzerland

6.1 INTRODUCTION

The crystal field interaction is an essential ingredient in a discussion of the

magnetic properties of materials. It is therefore important to be able to

describe and characterize the bonding between the central magnetic ion and

its (nonmagnetic) ligand ions in terms of some electronic theory. For such a

procedure it is obvious to classify the central magnetic ions according to the

character of the partly filled electronic shells giving rise to a permanent

magnetic dipole moment due to the orbital motion of the electrons, or to their

intrinsic spin, or to both.

Partly filled shells occur naturally in the transition metal groups which

comprise the 3d iron group, the 4d palladium group, the 5d platinum group,

the 4f lanthanide or rare earth group, and the 5f actinide group. In a crystal,

however, magnetic ions (rather than atoms) are by no means free, but they are

surrounded by a cage of (diamagnetic) ligand ions, the complex-forming part

of an extended lattice. The charged ligand ions have a strong interaction with

the magnetic ions, producing an electrostatic field (the crystal field or the

ligand field). The energy associated with this interaction varies roughly from

1 to 1000 meV; it may thus exceed the spin-orbit interaction, and in some

cases it may exceed the electrostatic interaction with other electrons on the

central ion responsible for the LS-coupling. Thus the crystal field is an

additional interaction in the already complicated problem of a free many-

electron atom. A perturbation approach is necessary in which terms must be

considered in order of decreasing importance (i.e., decreasing interaction

energy), and the crystal field interaction must be introduced at the appropriate

point relative to the sequence of interactions internal to the magnetic ion. In

order of decreasing interaction energy, these are [1]:

(i) The interaction of an electron with the Coulomb field of the nucleus,

modified by the repulsion field of the other electrons. With a suitably

averaged electronic field with central symmetry this interaction results in

6. Crystal Field Spectroscopy

the electronic levels being grouped into configurations. The ground

configuration of Cr

is 3d

, which lies some 10,000 meV below the first

excited configuration 3d

4s.

(ii) The residual mutual electrostatic repulsion of the electrons, not

represented by a central field, gives rise to Russell-Saunders or LS-

coupling, in which the orbital angular momenta and the spins of the

electrons are vectorially coupled to give a resultant total angular

momentum L and a total spin S, respectively. This coupling produces

energy splittings of order 1000 meV between terms of different quantum

numbers L and S associated with a particular configuration. e.g., the

ground term of 3d

is by Hund’s rule

F, with S and L = 3, some

1000 meV below the

P term, with S

same 3d

configuration.

(iii) The spin-orbit coupling H

= OL·S, with J = L ± S being a good quantum

number (upper and lower sign appropriate to electron shells being more

or less than half-filled, respectively), splits a given term into a multiplet

of levels with different values of J. The components of the multiplets are

split by about 10 meV for 3d electrons, and by larger amounts for ions

with larger atomic number (typically 1000 meV for 4f electrons).

The importance of the crystal field interaction relative to the interactions just

enumerated is different for the various transition groups. They may

conveniently be grouped as follows:

(i) Strong crystal field, i.e., crystal field interaction > LS-coupling. Typical

examples are the 4d and 5d electron systems.

(ii) Intermediate crystal field, i.e., crystal field interaction § LS-coupling >

spin-orbit coupling. Typical examples are 3d electron systems and to a

lesser extent the 5f actinide group.

(iii) Weak crystal field, i.e., crystal field interaction < spin-orbit coupling.

This group comprises the 4f electron systems.

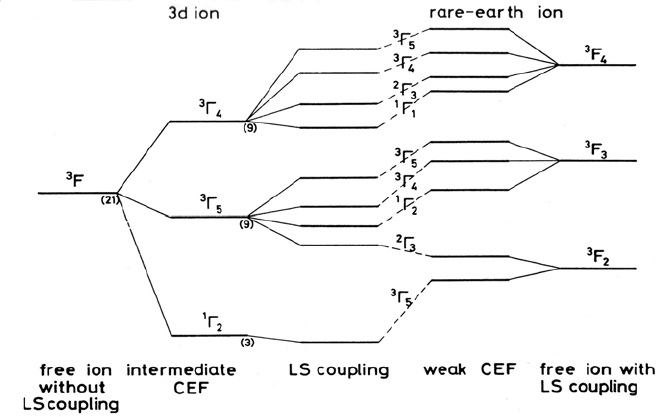
In Figure 6.1 we give an example of the effect of the crystal field interaction

on the level scheme of an ion with L = 3 and S = 1. The left hand part belongs

to case (ii) and the right hand part to case (iii).

The present chapter is organized as follows: Section 6.2 summarizes the

basic formalism and some commonly used models of the crystal field

interaction for the case (iii) mentioned above, i.e., for weak crystal fields as

realized for the 4f lanthanides. Some experimental techniques are described in

Section 6.3, with emphasis on neutron spectroscopy. Section 6.4 shows how

crystal field parameters can be determined from spectroscopic data. The effect

of interactions of crystal field split ions with phonons and conduction

electrons as well as exchange coupling effects are discussed in Section 6.5.

Section 6.6 demonstrates the relevance of crystal field studies towards under-

standing the mechanism of high temperature superconductivity. Some final

conclusions are given in Section 6.7.

3/2 and L = 1, belonging to the

3/2

258

6.2 The Crystal Field Interaction

Figure 6.1 Crystal field interaction of an ion with L = 3 and S = 1 for intermediate and weak

crystal field strength (after [2]).

6.2 THE CRYSTAL FIELD INTERACTION

6.2.1 Basic Formalism

The theory of crystal fields originates from the work of Bethe [3]. He showed

that the eigenvalues of a Hamiltonian, describing an open shell of electrons in

a crystal, can be classified according to the irreducible representations of the

point group of the site. Since that time the theory has made substantial

progress toward becoming elegant and simple especially applied to the 4f

lanthanide group as demonstrated by the work of Stevens [4, 5], Elliott and

Stevens [6], Judd [7], Hutchings [8], Newman [9], and Fulde [10]. Although

the description of crystal fields is rather well known and standard by now,

their computation from first principles is far from being satisfactory.

Nevertheless, the available models serve as a convenient parameterization

scheme to describe the crystal field interaction and the related magnetic

properties, but the model parameters have to be considered phenomenological

parameters rather than parameters reflecting the detailed electronic properties.

In the following paragraphs, and in the remainder of this article, we will

restrict our considerations to the crystal field interaction associated with the 4f

lanthanide group. This choice is motivated by the fact that most of the crystal

field studies reported in the literature have been devoted to rare earth

compounds for which the crystal field is usually the dominant interaction

looked at to understand the magnetic properties. However, the formalism can

be applied to the 5f actinide group as well as to the d-electron groups with

259

some extensions and modifications.

6. Crystal Field Spectroscopy

The mathematical problem, which has to be solved, is the calculation of

the electrostatic potential around the rare earth ion site. In elementary crystal

field theory the electrostatic potential arises from the electric charge

distribution on the surrounding ions. If overlap with the 4f electrons is

neglected, the electrostatic potential V

is a solution of Laplace’s equation

(r) = 0.

(6.1)

The solutions of this equation can be expanded in terms of multipoles of the

4f electrons as:

() ( , ).



ÇÇ

mn m

Vr arY

(6.2)

The spherical harmonics (, )

)

Y are normalized to

dcos d | ( , ) | 1.³4) 4)

The summation is restricted to n  6, since a

4f electron with orbital quantum

number l = 3 does not have multipoles

with n > 6. Alternatively one

may

expand V

in terms of tesseral

harmonics, which has the advantage

that

the

expansion is with respect

to real functions:

ĮĮ

0 Į

() Z( , ),

ÇÇ

Vr ar

(6.3)

where the tesseral harmonics

Z( , )

are defined by [8]:

Z() [ (1) ]





mmmm

nn n

mmmm

c YY

(6.4)

The index D in equation (6.3) is understood such that for a given value of n,

runs over

Z Y,Z ( ),Z ( )

nnn n

cs. The spherical and tesseral harmonics

are listed in [8]. The expansion coefficients

a are calculated from the

charge distribution U(r) as

4()

Z( , ).

2n 1



adr

(6.5)

Until now we have only considered a single 4f electron. For more than one

4f electron the potential energy of the rare earth ion in the crystal field V

given by

CF CF

(),

EeVr

(6.6)

where e is the electron charge and the index i runs over all the 4f electrons.

For rare earth compounds the spin-orbit interaction usually exceeds the crystal

field interaction by at least an order of magnitude, thus one can treat the

crystal field interaction as a perturbation of the lowest J-multiplet alone. The

n 0 m=

Z(s) [Y (1) Y ]



260

6.2 The Crystal Field Interaction

restriction to the lowest J-multiplet simplifies the calculations considerably as

demonstrated by Stevens [5]. He showed that the crystal field V

) being a

sum of polynomials in r

= (x

, y

, z

) can be replaced by a sum of polynomials

of the total angular momentum operators J = (J

, J

), which have the same

transformation properties as the original expression. They act on the unfilled

4f shell as a whole and therefore are much more convenient than the (x

, y

, z

)

polynomials that act on an individual 4f electron. The rules for transforming

an expression in the r

space to the J space are such that any products of (x

, y

) are replaced by the corresponding products of (J

) but written in a

symmetrized form [e.g., xy o

+ J

)]. Constants of proportionality F

have to be introduced, which depend on the order n as well as on the quantum

numbers L, S, and J. Furthermore, the dimensionality of the

r operators has

Some simple examples are [8]:

22 2 2 20

222

22 222 22

222

4224 4 4

444 44

444

(3 ) r [3 ( +1)] =

() [ ] =

(6 ) [()()]

[]

ii z

ii xy

iiii ii ii

zr JJJ rO

xy rJJ rO

xxyy xiy xiy

rJJ rO



FÃÓ  FÃÓ

FÃÓ FÃÓ

 

F Ã Ó  F Ã Ó

ÇÇ

(6.7)

where J

= J

± iJ

. A complete list of the operators

O which are called

Stevens

operators [4] as well as of the reduced matrix elements F

can

be found in [8].

A list of the radial integrals

ÃÓ

r can be found in

Freeman and Desclaux

[

11]. In the Stevens notation the crystal field

Hamiltonian reads

AB, FÃÓ

ÇÇ Ç

nmm mm

nnnnn

nm nm

rO O

(6.8)

where the

and B

are the crystal field parameters. We immediately

recognize

that the crystal field Hamiltonian consists of a large number

of parameters that

however, can be drastically reduced due to the point

symmetry at the rare earth

site. In particular, a center of inversion

cancels all the odd n

terms, and a

p-fold

axis of symmetry when chosen

as polar axis reduces the Hamiltonian

to terms containing

O . e.g., for

cubic point symmetry (with the four-fold

symmetry axis taken as polar

axis) the crystal field Hamiltonian reads

00 4 00 4

CF 4 4 4 6 6 6

B [ 5 ] B [ 21 , HO OO

(6.9)

i.e., the number of independent crystal field parameters is reduced to two. Lea

et al. [12] rewrote equation (6.9) in the form

to be retained by introducing the averages r over the 4f wave-functions.

261

]

6. Crystal Field Spectroscopy

1|x|

04 00 4

CF 4 4 6 6 6

F(4) F(6)

W( )[O ]



H

(6.10)

where

F(4) = Wx and

F(6) = W(1 – |x|) with –1  x  1 and W being an

energy scale factor. The eigenfunctions and eigenvalues of equation 6.10 are

tabulated in [12] as a function of the parameter x. The factors F(4) and F(6)

depend only on J and are also listed there. For hexagonal point symmetry, the

crystal field Hamiltonian has the form:

00 00 00 66

CF 22 44 66 66

BBBB H OOOO

(6.11)

with four independent parameters. For orthorhombic symmetry, there are as

much as nine adjustable crystal field parameters:

00 00 22 44 00 22 44 66

CF 22 44 44 44 66 66 66 66

BBBB+BBBB.  H OOOOOOOO

(6.12)

When the overall crystal field splitting is large and comparable to the

intermultiplet splittings, the Stevens formalism described above is no longer

appropriate. This situation was discussed in detail, e.g., by Wybourne [13]. In

this case the crystal field interaction leads to a mixing of the different J-

multiplets (J-mixing), and furthermore the J-multiplets are contaminated by

states of different quantum numbers L and S (intermediate coupling).

Therefore, the electrostatic, spin-orbit and crystal field interactions have to be

diagonalized simultaneously; the corresponding Hamiltonian reads

H = H

+ H

, with

E e

and H

(6.13)

The

adjustable

free ion

parameters

and [ correspond to Slater

electrostatic

and spin-orbit integrals, respectively , which can be taken,

e.g., from [13].

and A

represent matrix elements for the angular

parts of the electrostatic

and spin-orbit interactions, respectively, which

have been tabulated by

Nielson and Koster [

14]. The crystal field

Hamiltonian takes then the form

[13]

D(C C ),





nn n

mm mi

mni

(6.14)

where C

are one-electron tensor operators and the summation

involving i runs

over all the electrons of the ion of interest. The crystal

field parameters

of the tensor formalism are related to the crystal

field parameters

in the

Stevens notation through

DB,

(6.15)

where the reduced matrix elements F

and the numerical factors F

are

tabulated by Hutchings [8] and Wybourne [13], respectively.

5] B[OO 21

262

6.2 The Crystal Field Interaction

6.2.2 Model Calculations of the Crystal Field Interaction

6.2.2.1 Point-Charge Model

The point-charge model is characterized by ascribing effective charges q

the neighboring sites of the rare earth ion under consideration. In that case the

charge distribution U(r) is given by

() ( )U G 

r q r ,

(6.16)

where the R

denote the positions of the neighboring ions. If we only consider

the nearest-neighboring ligand ions carrying an effective charge q and being

placed at a distance d from the rare earth ion, then the expansion coefficients

of equation (6.5) can be expressed as

4(1)

,with Y ( , ),





S

ÃÓ -M



mnmm m

nnnn

aqrff

(6.17)

where the sum runs over all the nearest neighbors located at angular coordinates

,-M

. The

are called geometrical coordination

factors, which are

discussed

in detail in [8]. Thus, the crystal field

parameters can be

decomposed

in a charge and a geometrical term:

BA r || . FÃÓ FÃÓ

mm nm

nnn n n

eq r

(6.18)

For a crystal field of cubic symmetry as defined by equation (6.9), one finds

the crystal field parameters for eight fold coordination (cube):

040 6

446 6

7| | 1| |

B,B ,

18 9

FÃÓ FÃÓ

eq eq

(6.19)

and for six-fold-coordination (octahedron):

040 6

446 6

7| | 3| |

B,B.

16 64

 F Ã Ó  F Ã Ó

eq eq

(6.20)

6.2.2.2 Extended Point-Charge Model

In general the simple point-charge model is not able to reproduce observed

crystal field spectra in a satisfactory manner. In particular, the second- and

sixth-order crystal field parameters are usually found to be an order of

magnitude too large and too small, respectively, thus several extensions have

been introduced to improve the point-charge model:

(i) Sternheimer [15] showed that due to the screening of the 4f

electrons by

the outer shells, the

ÃÓ

r terms should be replaced by ÃÓ

(1 – V

). The

shielding

factors V

are given as a function of the number

of electrons N of

the R ion as [

16]:

263

6. Crystal Field Spectroscopy

= 0.6846 – 0.00854·N

= 0.02356 + 0.00182·N

(6.21)

= – 0.04238 + 0.00014·N

(ii) Morrison [16] showed that the nth moment of the radial wavefunctions

for the free ion have to be replaced by r

when it is placed into a

crystal. W as a function of N is given for the system R:CaWO

W= 0.75·(1.0387 – 0.0129·N)

(6.22)

(iii) For metallic compounds a further correction is necessary due to the

screening effect of the conduction electrons which can be taken into account

by a Yukawa-type potential:



() exp | |

V N



rrR

(6.23)

where N is the inverse screening length. As a consequence, equation (6.17)

has to be modified in the following way [17]:

1/2

() .



NÃÓ



mnm

nn n

aqKdr

(6.24)

The K

n+1/2

(x) are the modified Bessel functions, normalized to obtain

n+1/2

(0) = 1. Assuming nominal charges for the nearest neighboring ions, we

are left with a model containing only a single parameter N. As we will see in

Section 6.4.2, this model works well for metallic rare earth compounds.

6.2.2.3 Superposition Model

In its most general form the superposition model corresponds to the

assumption that the total crystal field can be built up from separate

contributions from each of the ions in the crystal [9]. The spherical symmetry

of the ions then ensures that each contribution can be represented by a

cylindrically symmetrical field, which is described by just three parameters

A ÃÓ

r (n = 2, 4, 6) in the Stevens notation if the z-axis is the polar axis. The

functional dependence of the field of a single ligand ion on its distance R from

the central ion is made explicit by introducing the “intrinsic”

parameters

A()

A()A(), N

nnmni

r i R

(6.25)

where the coordination factors N

(i) depend on the angular positions of all

ligand ions at a given distance R

from the central ion. Explicit formulae for

the coordination factors N

(i) have been given by Newman [9]. It is often

convenient to decompose the function

A()

into a value

n 0

A( )

at the

mean distance R

of the coordinated ligands and a power law t

over a

restricted range of R:

ÃÓ

264

6.2 The Crystal Field Interaction

A( ) A( )( / ). ¹

RRR

(6.26)

In practice the superposition model works well for n = 4 and n = 6, but not for

n = 2, thus the fourth- and sixth-order crystal field terms can be represented by

the four quantities

A ( ), A ( ),

R t

, t

, provided that all the ligands are of the

same type.

6.2.2.4 Angular Overlap Model

The angular overlap model (AOM) may be seen as a first-order perturbation

approach to covalent bonding [18]. Being based on covalency, the AOM is

thus in contrast to the point-charge model resting on the electrostatic

interaction. With the assumption of weak covalency the perturbation energies

of the central orbitals are proportional to the squares of the overlap integrals

of the central ion with the ligand. Like in the electrostatic theory the AOM

assumes the effects of several ligands bonded to the central ion to be additive,

which is equivalent to the assumption of no ligand-ligand overlap. The

antibonding energy E* of a given f-orbital is given as [18]





()

E eF ,

(6.27)

, the angular overlap integral, is a property of the orientations of the

overlapping orbitals and applies to equivalent O-bonding. The superscript

labels the quantum number of the wavefunction of the central ion. e

is the

angular overlap parameter for a particular bonding type.

Let us consider a specific example, namely the crystal field potential of an

octahedron formed by six identical, axially symmetric ligands with the

quantization axis along the three-fold axis. This leads to a Hamiltonian with

five crystal field parameters, which are related to four independent angular

overlap parameters e

[18]:

3214

B2 2

16 3 3

15 2 2 14

B2 2

433

13 8 8 4

224 3 5 15

65 8 8 4

128 3 5 15

143 8 8 4

256 3 5 15

VS GM

VS G M

ÈØ



ÉÙ

ÊÚ

ÈØ



ÉÙ

ÊÚ

ÈØ



ÉÙ

ÊÚ

ÈØ

   

ÉÙ

ÊÚ

ÈØ



ÉÙ

ÊÚ

ee ee

ee e e

(6.28)

where

265

6. Crystal Field Spectroscopy

Confining ourselves to V bonding only [19], we reduce the number of ligand-

field parameters to one, which is e

. This can be done for all kinds of co-

ordination symmetries, assuming identical ligands and equal bond lengths.

The reduction of the number of independent ligand-field parameters is a

particular strength of the AOM that will simplify the interpretation of the

magnetic properties and energy spectra of compounds with low symmetry.

6.2.2.5 First Principles Density Functional Theory

The density functional theory (DFT) is a first principles method to calculate

the crystal field parameters

. Within this method the electronic structure

and the corresponding distribution of the ground state charge density is

obtained using the full potential linearized augmented plane wave method

(LAPW) which is implemented in the latest version of the WIEN97 code [20].

This was demonstrated, e.g., for ErNi

C [21]. The crystal field parameters

originating from the aspherical part of the total single-particle DFT potential

in the crystal can be obtained from:

22 2 2

4f 1 4f 1

B |R ()| () |R ()| () ,

mm m

rVrrdr rWrrdr

ÈØ



ÉÙ

ÊÚ

ÔÔ

(6.29)

where

()

Vr and

()

Wr are the components of the total (Coulomb and

exchange correlation) potential inside the atomic sphere with radius R

and

in the interstitial region, respectively. The term

()

Vr is readily obtained

with the WIEN97 code. The term

()

Wr is calculated using an exact

transformation of the Fourier representation of the LAPW potential into a

spherical Bessel function expansion between the radius R

and an upper

radial limit R

beyond which the 4f charge density can be neglected. The term

(r) describes the radial shape of the localized 4f charge density of the R

ion. The conversion factors

establish the relation between the symmetrized

spherical harmonics used within LAPW and the real tesseral harmonics, which

transform in the same way as the tensor operators

O [22]. The crystal field

parameters for ErNi

C obtained from equation (6.29) were found to be in

reasonable agreement with those determined by neutron spectroscopy [23],

which suggests that the DFT crystal field parameters can be used as good

starting values in the analysis of experimental data. A more recent example

concerns the intermetallic compounds PrAl

and NdAl

for which the

substantial pressure-induced changes of the crystal field parameters could be

well reproduced by DFT calculations [24].

6.2.3 Parameterization of the Crystal Field Interaction

The determination of the crystal field parameters from experimental data is

not an easy task, except perhaps for the case of cubic systems with only two

266