Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

Lagrangian Mechanics

∂

−= =

∂

,const

qhh



(18.2.63)

which can be expressed also in the form (hence, a particular case of Painlevé’s first

integral)

−−= =

,constTTUhh

(18.2.63')

In particular, if the mechanical system is catastatic, hence scleronomic (being

holonomic), then we have

= 0T



, so that = 0



L if = 0U



, hence if the forces are

conservative, deriving from a simple potential; we obtain the first integral of

mechanical energy

=−= = =

,,constETU hTTh .

(18.2.64)

The relation (18.2.62) with

= 0



L can be written in the form

∂∂

⎡⎛ ⎞ ⎤

−=

⎜⎟

⎢⎥

∂∂

⎣⎝ ⎠ ⎦

tq q



(18.2.62')

too. Starting from this result, some authors try to obtain Lagrange’s equations, which is

not possible because the generalized velocities

q cannot by arbitrarily chosen (they

correspond to the motion in the actual state).

If the generalized forces admit a generalized quasi-potential of the form (18.2.21),

with (18.2.22), then we can express them by the relations (18.2.3); we obtain

∂

⎛⎞

=− −+

⎜⎟

∂∂ ∂

⎝⎠

∂

=−=−−=−

∂

00 0

jj j jj j

jjj jj

Qq qq Uq q

qq q

UU U

qUq UUq U

qt t







 

The relation (18.2.60') becomes

()

−− +=

TTU



(18.2.65)

Supposing that the kinetic potential

=+TUL does not depend explicitly on time

=(0)



, it results a first integral of Jacobi type (particular case of the first integral of

Painlevé)

−− = =

,constTTU hh .

(18.2.66)

As well, in case of scleronomic constraints we have

0T (and



too), being

thus sufficient that



, hence that the forces be conservative, deriving from a

generalized potential, to have a first integral of mechanical energy in the form

MECHANICAL SYSTEMS, CLASSICAL MODELS

=− = = =

,,constETU hTTh

(18.2.67)

The mechanical systems which admit a first integral of Jacobi or a first integral of

Jacobi type are called generalized conservative systems; we have seen that these

systems are natural systems for which the kinetic potential

L does not depend

explicitly on time

=(0)



The quasi-potential

or the scalar potential

are quantities of energetical nature;

hence, the potential energy is

=−VU

, in case of a simple quasi-potential, or

=−

, in case of a generalized quasi-potential. We introduce thus the generalized

mechanical energy

=−+

TTVE ,

(18.2.68)

which is constant in case of Jacobi’s first integral or of a first integral of Jacobi type. In

particular, if the forces are conservative (we have = 0V



) and the constraints are

scleronomic (we have

0T and =

TT), then the generalized mechanical energy is

equal to the mechanical energy, obtaining thus the first integral of mechanical energy.

In case of generalized forces of the form (18.2.24), the relation (18.2.60) reads

∂

⎛⎞

−+=

⎜⎟

∂

⎝⎠

qQq





(18.2.69)

If the kinetic potential does not depend explicitly on time (

= 0



), then it results

∂

⎛⎞

=−=

⎜⎟

∂

⎝⎠

qQq

ttq





(18.2.69')

We can thus state that the mechanical system

S admits a Jacobi’s first integral

(written in the form (18.2.63) or in the form (18.2.63')) for generalized non-potential

forces too. The relation (18.2.69') allows also to notice that, in case of dissipative non-

potential forces, the energy of the mechanical system diminishes.

We observe that in none of the above considered first integrals does not intervene the

component

of the kinetic energy; this is explained because the kinetic energy is

determined excepting the total derivative with respect to time of a function

( , ,..., ; )

qq qtϕ of class

C , hence excepting =∂ ∂ +dd ( )

tqqϕϕ ϕ.

In case of non-holonomic constraints, Lagrange’s equations have the form (18.2.32),

so that the relation (18.2.60) becomes

()

∂

⎛⎞

−= + −

⎜⎟

∂

⎝⎠

jjj

qT QRqT







Taking into account (18.2.29') and (18.2.33), we can also write

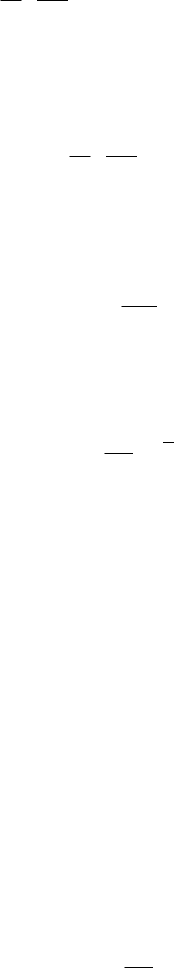
Lagrangian Mechanics

∂

⎛⎞

−= −−

⎜⎟

∂

⎝⎠

∑

jjj

qT QqT a







(18.2.70)

If the constraints are catastatic (we have

0, 1,2,...,

ak m== ), then the relation

(18.2.60) becomes (we have

= 0T



too)

∂

⎛⎞

−=

⎜⎟

∂

⎝⎠

qT Qq





(18.2.71)

so that the influence of these constraints is not seen; written in the form (18.2.60'), this

relation becomes (we have also

0TT

)



(18.2.71')

Expressing the generalized forces in the form (18.2.25), we can write

(

=+ =−

,ETVV U)

=−

Qq U



(18.2.72)

so that, in the case in which the potential part does not depend explicitly on time

(

= 0U



), while the non-potential forces are gyroscopic, we get a first integral. Hence,

in general, a natural and catastatic, non-holonomic mechanical system, for which the

generalized forces derive from a simple or generalized potential, has a first integral of

energy of the form

=+= =,constETV hh .

(18.2.73)

Such a mechanical system is called a conservative system. If these generalized forces

have only the non-potential part (

= 0U ), which is gyroscopic, even the kinetic energy

T is conserved during the motion.

We notice that this first integral of energy differs from that obtained for a discrete

mechanical system

S in

E because the holonomic constraint forces do not intervene

any more. As a matter of fact, in case of catastatic constraints no essential difference

appear, the real elementary work of the corresponding constraints forces vanishing.

We notice also that, if we take into account (18.2.25'), (18.2.26) in case of a potential

(

= 0U



), then the relation (18.2.72) leads to

=−

(18.2.74)

where

R is Rayleigh’s dissipative function, which characterizes the decrease of the

mechanical energy

E .

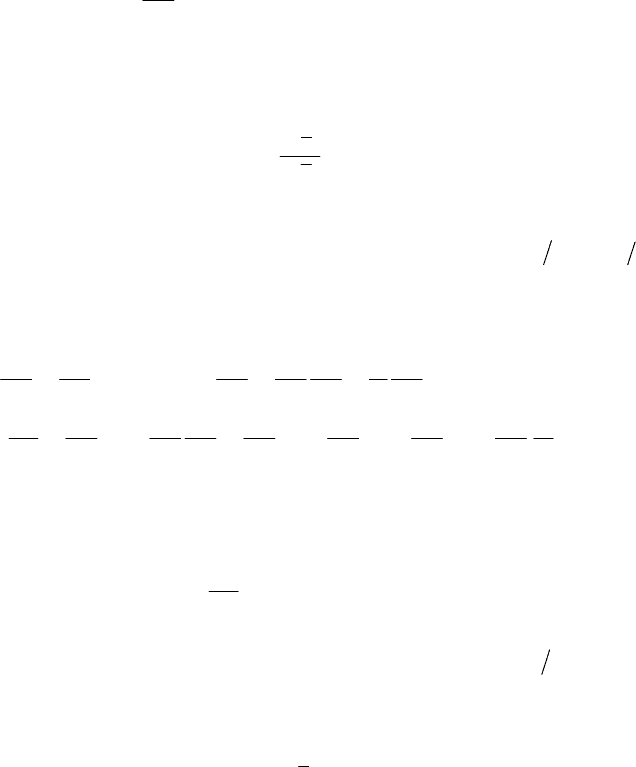
MECHANICAL SYSTEMS, CLASSICAL MODELS

18.2.3.5 Reduction of Lagrange’s System of Equations by Means of Jacobi’s First

Integral

One can state

Theorem 18.2.5. Jacobi’s first integral allows the reduction of Lagrange’s system of

equations (18.2.38), corresponding to a holonomic and natural mechanical system with

s degrees of freedom, to a system of equations corresponding to a mechanical system

with

− 1s degrees of freedom (Jacobi,C.G.J., 1882).

In particular, in case of a mechanical system with a single degree of freedom (a

mechanical system with complete constraints), the first integral

∂

−= =

∂

,constqhh



(18.2.75)

reduces the problem to a quadrature; indeed, from (18.2.75) we can get

= ()qfq ,

wherefrom

−=

∫

()

(18.2.75')

In case of a mechanical system with

s degrees of freedom, we make the change of

generalized velocities

′′

→→ →

11212 1

, ,...,

qqqqqqqq , with

′

kk k

qqqqq,

= 1,2,...,ks

, so that

′′

12 12 12 12

( , ,..., , , ,..., ) ( , ,..., , , ,..., )

ss ss

qq qqq q qq qqq qΛ  L .

Differentiating, we can write too

()

===

′

∂

∂∂ ∂∂ ∂

== = = =

′′

∂∂ ∂∂∂ ∂

∂

∂∂∂ ∂∂∂∂

′

=+ =+ =+

∂∂ ∂∂∂ ∂ ∂ ∂

∑∑∑

11 11 1 1

222

, 1,2,..., , ! , 2,3,..., ,

kkk k

sss

kkk

js ks

qq qqqqq

qq qqq q q qq

ΛΛΛ







LL LLLL

By the above mentioned change of variables in the first integral (18.2.63) and by

taking into account the preceding results, we can write

∂

−=

∂



(18.2.76)

wherefrom

′′ ′

1112 23

( , ,..., , , ,..., )

qqqq qqq q . We denote =∂ ∂

′

qΛ L , so that

′′ ′

′′

12 23

( , ,..., , , ,..., )

qq qqq qLL . Using the notations thus introduced and taking

into account the form (18.2.15) of the kinetic energy, we can write

′′

12 12 12 12

( , ,..., , , ,..., ) ( , ,..., , , ,..., )

ss ss

Tqq qqq q Tqq qqq q   ,

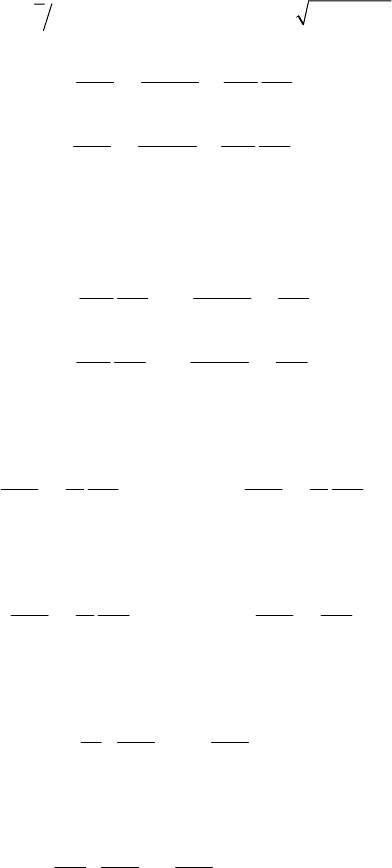
Lagrangian Mechanics

so that

TqT



 , where

′′ ′

12 23

( , ,..., , , ,..., )

TTqq qqq q



; on the other hand,

assuming the existence of a simple quasi-potential, we have

=+ =,constTUhh ,

while

=∂ ∂ =

′

2Tq qT



L , whence

′

2( )TU h



. We can calculate

, 1,2,..., ,

jj j

qqq q

ΛΛ

∂

∂∂∂

=+ =

∂∂∂ ∂

∂

′



∂

∂∂∂

=+ =

′′ ′

∂∂∂ ∂

∂

′

,2,3,...,;

kk k

qqq q

ΛΛ



as well, by a partial differentiation of the relation (18.2.76) with respect to the

generalized co-ordinates and the new generalized velocities, we obtain

, 1,2,..., ,

jj j

qq js

qqqq

ΛΛΛ

∂

∂∂∂

+==

∂∂∂∂

∂







∂

∂∂∂

+==

′′ ′

∂∂∂∂

∂

, 2,3,..., .

kk k

qq ks

qqqq

ΛΛΛ







Comparing with the previous results, we may write

∂∂ ∂∂

== ==

′′

∂∂ ∂∂

′′

, 1,2,..., , , 2,3,...,

js ks

qqq qqq

ΛΛ



wherefrom, using the above obtained relations, we get

∂∂ ∂∂

== ==

′

∂∂ ∂∂

′′

, 1,2,..., , , 2,3,...,

js ks

qqq q q

LL LL

Lagrange’s equations (18.2.38) lead to

∂∂

⎛⎞

−==

⎜⎟

′

∂∂

⎝⎠

′′

0, 2,3,...,

qks

tq q



or to

∂∂

⎛⎞

−==

⎜⎟

′

∂∂

⎝⎠

′′

0, 2, 3,...,

qq q

(18.2.77)

so that the functions

( ), 2,3,...,

qq k s are determined by a system of

− 1s

equations of second order of Lagrange type (the equations of Jacobi, who has put in

evidence this method of calculation) in a

−(1)s -dimensional space. Thus, the number

of degrees of freedom of the initial mechanical system has been reduced by a unity.

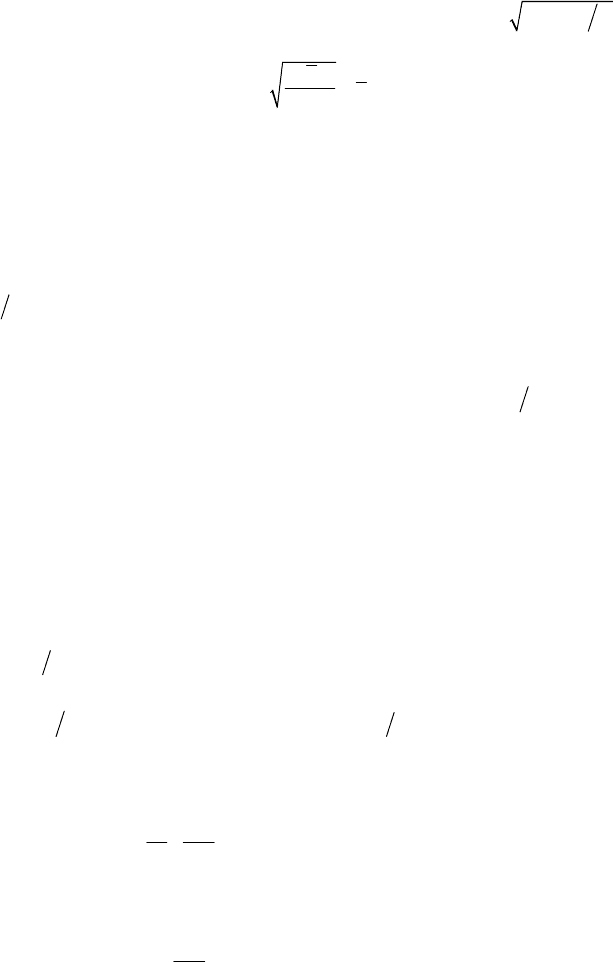
MECHANICAL SYSTEMS, CLASSICAL MODELS

From the expression of the function

′

L of Lagrangian type, one observes that – in

general – the new independent variable

q intervenes explicitly, so that it is no more

possible to obtain a first integral of the type of Jacobi’s first integral.

By means of the notations thus introduced, we notice that

()qUhT



 , so that

−=

∫

tt q

(18.2.77')

completing thus the information given by the equations (18.2.77) about the dependence

with respect to time.

18.2.3.6 Hidden Co-ordinates. Ignorable Co-ordinates

We call, after Helmholtz, hidden co-ordinate

q that co-ordinate which does not

intervene explicitly in the expression of the kinetic energy

T , hence for which

∂∂ =0

Tq ; because we can have ≤hs hidden co-ordinates, it is convenient to

denote them

, ,...,

qq q. If the co-ordinate

q does not intervene explicitly in the

expression of the kinetic potential

=+TUL , corresponding to a natural system

which admits a simple or generalized quasi-potential, hence if

∂∂=0

qL , then this

co-ordinate is called an ignorable co-ordinate (after W. Thomson) or a kinostenic co-

ordinate (after J. J. Thomson); this denomination is used also in the case of a non-

natural mechanical system which admits a Lagrangian, with the condition (18.2.34''').

We can have

≤rs ignorable co-ordinates

, ,...,

qq q. Sometimes only the

denomination of hidden co-ordinates is used; but we will use both the denominations of

hidden and ignorable co-ordinates, to can make distinction in various situations. The

mechanical systems in which appear ignorable co-ordinates are called – by some

authors – gyroscopic systems (because such situations appear often in case of the

gyroscope).

∂∂=0

Uq , where U is a simple or generalized quasi-potential, then an eventually

corresponding ignorable co-ordinate is also a hidden one. We notice that we can have

∂+ ∂=() 0

TU q , without having necessarily ∂∂ =0

Tq (Pars, L., 1965).

In the case of

h hidden co-ordinates

, ,...,

qq q, the first h equations of Lagrange

for holonomic constraints (18.2.29) read

∂

⎛⎞

⎜⎟

∂

⎝⎠

, 1,2,...,

Qk h

tq

(18.2.78)

If we have

= 0

Q too, then there result h first integrals

∂

, 1,2,...,

ck h

q

(18.2.78')

Lagrangian Mechanics

each first integral corresponding to a hidden co-ordinate.

Assuming the existence of

r ignorable co-ordinates

, ,...,

qq q, Lagrange’s

equations (18.2.38) take the form

∂

⎛⎞

⎜⎟

∂

⎝⎠

0, 1,2,...,

tq

(18.2.79)

wherefrom the first integrals

∂

, 1,2,...,

Ck r

q

(18.2.79')

By convention, we denote

∂

,1,2,...,

pjs

q

(18.2.80)

In the case of a simple quasi-potential (or in the absence of a quasi-potential), it results

∂

, 1,2,...,

pjs

q

(18.2.80')

If, in particular, the mechanical system is free or none holonomic constraint has been

eliminated, the space of Lagrange being just the space

E , then – starting from the

kinetic energy corresponding to the

n particles – we can write

∂∂

⎛⎞

⎜⎟

∂∂

⎝⎠

∑

ii j j

mv mv

We notice that this represents just the magnitude of the momentum of the particle

corresponding to the velocity

. Starting from this observation, we will call the

magnitudes

=, 1,2,...,

pj s

, components of the generalized momentum; the first

integrals (18.2.78') will be written in the form

==, 1,2,...,

pck h,

(18.2.78'')

while the first integrals (18.2.79') read

==,1,2,...,

pCk r.

(18.2.79'')

U is a generalized quasi-potential of the form (18.2.22), then it results

∂

=− =

∂

,1,2,...,

pUj s

q

(18.2.81)

MECHANICAL SYSTEMS, CLASSICAL MODELS

and in case of hidden co-ordinates we obtain

()

−=

kk k

pU Q

If we have

= 0

Q too, then we can write the first integrals

−= =, 1,2,...,

kkk

pU ck h.

(18.2.81')

We notice also that the notion of generalized momentum has a larger sense than the

classical one, corresponding to the case in which the generalized co-ordinate is a length. Let

us consider, e.g., a particle for which the kinetic energy is expressed in cylindrical co-

ordinates (

=++

2222

(2)( )Tmrr zθ





); in this case, =∂ ∂ =∂ ∂ =

pTmr

θθθ



=×rr()



. Hence, to a generalized co-ordinate which is an angle corresponds a

generalized momentum which is a component of a moment of momentum.

If the relation (18.2.80') takes place, then the relation (18.2.78) reads

== =

, 1,2,...,

pQkh



(18.2.82)

Hence, in case of a hidden co-ordinate and in the absence of a quasi-potential, we can

write an universal theorem of mechanics. If the generalized co-ordinate is a length, then

the generalized force is of the nature of a force (so that the product

Qq be a work),

obtaining thus a theorem of momentum corresponding to the respective linear co-

ordinate; but if the generalized co-ordinate is an angle, then the generalized force –

from the same reasons – is of the nature of a moment, resulting a theorem of moment of

momentum corresponding to the respective angular co-ordinate. As a matter of fact, we

can state

Theorem 18.2.6 (theorem of generalized momentum). In case of several hidden co-

ordinates and in the absence of a quasi-potential, in the free motion of the

representative point in the space of configurations, a theorem of generalized momentum

of the form (18.2.82) is verified.

In the case in which

==0, 1,2,...,

Qk h, we can write a conservation theorem of

generalized momentum, which is – in fact – a first integral of the form (18.2.78'').

As well, in case of generalized forces of the form (18.2.24) and of several ignorable

co-ordinates, Lagrange’s equations lead to the relations

== =

, 1,2,...,

pQkr



(18.2.82')

where

Q are non-potential generalized forces; hence, we can state

Theorem 18.2.6' (theorem of generalized momentum; second form). In case of several

ignorable co-ordinates, in the free motion of the representative point in the space of

configurations, a theorem of generalized momentum of the form (18.2.82') is verified.

Lagrangian Mechanics

==0, 1,2,...,

Qk r, then we get a conservation theorem of generalized

momentum, hence a first integral of the form (18.2.79'').

18.2.3.7 The Routh–Helmholtz Theorem

We can state

Theorem 18.2.7 (Routh–Helmholtz). If a discrete mechanical system S , with

s degrees of freedom, has r ignorable co-ordinates, then the determination of the free

motion of the representative point in the space of configurations is reduced to the

integration of a system of differential equations of Lagrange type, corresponding to a

mechanical system with

−sr degrees of freedom and to the calculation of

r quadratures.

Indeed, let us perform the transformation of Routh, E.J. 1892, 1898]

∂

=−

∂

∑



(18.2.83)

The first integrals (18.2.79'), where

L is of the form (18.2.34'), can be seen as a

system of

r linear equations in the generalized velocities =, 1,2,...,

qk r ; the

condition (18.2.34''') being fulfilled, we determine the functions

++ ++

12 12 12

( , ,..., , , ,..., ; , ,..., ; ), 1,2,...,

ssr

rr rr

qqqq qqq qCC Ctk r   ,

where the generalized co-ordinates

++12

, ,...,

qq q are called palpable co-ordinates

(unlike the ignorable co-ordinates). Replacing in (18.2.83), we get

++ ++

12 12 12

( , ,..., , , ,..., ; , ,..., ; )

ssr

rr rr

qq qqq qCC Ct RR .

In this case, applying a variation

δ to the function R of Routh, we obtain

=+ =+ =

∂∂∂

δ= δ+ δ+ δ

∂∂∂

∑∑∑

111

ssr

jr jr k

qqC



RRR

Noting that

=+ =

∂∂

δ= δ+ δ

∂∂

∑∑

jr j



== =

∂∂

δ=δ+δ

∂∂

∑∑ ∑

11 1

rr r

kkkk

kk k

qqqC





and taking into account the relation of definition (18.2.83), we get, as well,

=+ =+ =

∂∂

δ=− δ− δ+ δ

∂∂

∑∑∑

111

ssr

jr jr k

qqqC





MECHANICAL SYSTEMS, CLASSICAL MODELS

The variation

δ being arbitrary, by comparison of the two relations, it results

∂∂∂∂

−= −= =++

∂∂∂∂

∂

, , 1, 2,..., ,

, 1,2,..., ,

jjjj

jr r s

qqqq

qkr





LRLR

so that, replacing in Lagrange’s equations (18.2.38), we get the equations

∂∂

⎛⎞

−==++

⎜⎟

∂∂

⎝⎠

0, 1, 2,...,

jr r s

tq q

(18.2.84)

which are of Lagrange type, corresponding to a mechanical system with

−sr degrees

of freedom; these

−sr equations determine the generalized co-ordinates = ()

qqt,

=+ +1, 2,...,

rr s. Replacing these co-ordinates in Routh’s function, it results

( , ,..., ; )

CC C tRR and one can obtain the other generalized co-ordinates in the

form

∂

−= = =

∂

∫

d 0, 1,2,...,

qq t k r

(18.2.85)

the theorem being thus justified.

Starting from (18.2.83), Hertz introduced a generalized kinetic energy of the form

⎛⎞

=− +

⎜⎟

⎝⎠

∑

TV CqT

the sum being considered as being a potential of forces resulting from a unknown cyclic

motion; as a matter of fact, Hertz tried to define the potential energy of a mechanical

system by the cyclic motion of several “ignorable masses”, which are added to the

palpable (visible) masses of the mechanical system

S , which justifies the

denomination given to the corresponding generalized co-ordinates.

Let be the classical model of atom, called “rotator”, which has a motion of rotation about

a fixed axis in the space. Because the kinetic energy

(/2)Tm= ++

2222

()rr zθ





thus that

/0T θ∂∂=, the theorem of areas is / θ=∂ ∂L

constmr θ



; taking into

account

= constr , it results =+ =

0000

,, consttθω θωθ .

We notice that the function

R of Routh plays the rôle of a kinetic potential for the

new problem which remains to be solved. But we mention that in this catastatic

mechanical system appear always terms linear in the generalized velocities too (even in

case of the catastatic mechanical systems for which the kinetic energy is a positive

definite quadratic form and which is acted upon by generalized forces which admit a

simple quasi-potential).