Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

Lagrangian Mechanics

∂

⎡⎤

≠

⎢⎥

∂∂

⎣⎦

det 0

qq

(18.2.34''')

As a matter of fact, if the system is natural, then we have

∂∂

∂∂ ∂∂

qq qq

 

so that the considered Hessian is given by

=≠det[ ] 0

, as it has been shown in

Sect. 18.2.1.3.

To the state of rest of the discrete mechanical system

S with respect to an inertial

frame of reference corresponds a position of rest of the representative point

P in the

space

Λ . From (18.2.32) it results that (we make ===0, 0, 1,2,...,

qq j s )

+= =0, 1,2,...,

QR j s.

(18.2.39)

Obviously, these relations must be verified also by the initial conditions, where

0, 1,2,...,

qj s . More precisely, the conditions (18.2.39) should be written in the

form

+=∈

00 0

12 1

( , ,..., , 0,0,...,0; )

( , ,..., , 0,0,...,0; ) 0, [ , ],

Qqq q t

Rqq q t t tt

(18.2.39')

where we have put in evidence also the position of equilibrium (corresponding to the

initial conditions). If the discrete mechanical system

S is subjected only to holonomic

constraints, then the conditions of equilibrium are written in the form

==0, 1,2,...,

Qj s

(18.2.40)

If the generalized forces derive from a simple quasi-potential, we obtain

∂

0, 1,2,...,

(18.2.40')

hence a necessary condition for the quasi-potential

U to admit an isolated extremum.

Concerning the state of rest, one puts important problems of stability, which will be

considered afterwards.

18.2.2.3 Normal Form of Lagrange’s Equations

Taking into account the expression (18.2.15), (18.2.15'), (18.2.15 18.2.15''')

of the kinetic energy, we can find an explicit form of Lagrange’s equations (18.2.32).

We calculate thus

∂∂ = +

jk k

Tq gq g and then

''), and (

MECHANICAL SYSTEMS, CLASSICAL MODELS

∂

⎛⎞

=+ ++ +

⎜⎟

∂∂∂

⎝⎠

∂∂

∂

=++

∂∂ ∂ ∂

;

jk j

jk k k l jk k

kl k

jj j j

gq qq g q g

tq q q

qq q

qq q q

     



 

noting that the product

qq is symmetric in the indices k and l , it results

∂∂∂

⎛⎞

⎜⎟

∂∂∂

⎝⎠

jk jk jl

kl kl

llk

ggg

qq qq

qqq

 

so that we write the equations (18.2.32) in the form

+++

∂

−+=+ =

∂

[,] (2 )

, 1,2,..., ,

k k k l jk jk k

jjj

gq kljqq g q

gQRj s

γ    



(18.2.41)

where we have introduces Christoffel’s symbols of the first kind

∂∂

∂

⎛⎞

=+− =

⎜⎟

∂∂∂

⎝⎠

[ , ] , , , 1,2,...,

jk jl

kl j j k l s

qqq

(18.2.42)

as well as the skew-symmetric quantities

∂

⎛⎞

=− = − =

⎜⎟

∂∂

⎝⎠

, , 1,2,...,

jk kj

jk s

γγ

(18.2.43)

We have thus obtained a system of linear equations in the generalized accelerations

=, 1,2,...,

qk s .

Let

kkj

gg be the algebraic complement of the element

g in the determinant

g , which is definite by (18.2.17); the normalized algebraic complement will be

kkj jk

gggg. We notice that the relations

==δ

det[ ] ,

kmjm

ggg

(18.2.44)

where

is Kronecker’s symbol (equal to unity for =km and equal to zero for

≠km), take place. Multiplying the system of equations (18.2.41) by

g and

summing with respect to

j , it results (we replace then the free index m by j )

∗∗

⎧⎫

⎪⎪

+++=+=

⎨⎬

⎪⎪

⎩⎭

, 1,2,...,

jjjj

kl jkk

qqqqQRjs

αβ   

(18.2.45)

Lagrangian Mechanics

where we have introduced Christoffel’s symbols of second kind, defined by (the

relations of definitions for Christoffel’s symbols correspond to the relations of

definition (A.1.44) and (A.1.45) from

E )

⎧⎫

⎪⎪

⎨⎬

⎪⎪

⎩⎭

[ , ] , , , 1,2,...,

kl m g j k l s

(18.2.42')

as well as the notations (as everywhere in this work, we make not considerations on

covariance or on contravariance, the position of the indices – up or down – having no

one signification)

∗∗

∂

⎛⎞

=+ =−+

⎜⎟

∂

⎝⎠

===

(2 ) , ,

, , 1,2,..., .

mj mj

mk jk jk

mj mj

gg qg

QQgRRgm s

αγ β

(18.2.46)

The system of equations (18.2.45) represents thus the normal form of Lagrange’s

equations, in which the generalized accelerations are expressed as functions of the

generalized velocities and of the generalized co-ordinates; this form of the equations

can be always obtained, because

≠ 0g .

In the case of catastatic constraints we have

, and the equations (18.2.45)

take the form

∗∗

⎧⎫

⎪⎪

+=+=

⎨⎬

⎪⎪

⎩⎭

, 1,2,...,

jjj

qqqQRjs

  

(18.2.47)

If the constraints are holonomic, then the equations (18.2.45) become

∗

⎧⎫

⎪⎪

+++==

⎨⎬

⎪⎪

⎩⎭

, 1,2,...,

jjj

kl jkk

qqqqQjs

αβ   

(18.2.45')

while the equations (18.2.47) read (in this case, the constraints are scleronomic too)

∗

⎧⎫

⎪⎪

+==

⎨⎬

⎪⎪

⎩⎭

, 1,2,...,

qqqQjs

  

(18.2.47')

In case of a single particle, the equations (18.2.47') become (6.1.24''').

18.2.2.4 Theorems of Existence and Uniqueness

We can replace the system of s differential equations of second order (18.2.45') by a

system of

differential equations of first order in the normal form

∗

⎧⎫

⎪⎪

===− −−=

⎨⎬

⎪⎪

⎩⎭

, , , 1,2,...,

jjjjj j j

kl jkk

quu Q uu u j s

ϕϕ α β

(18.2.48)

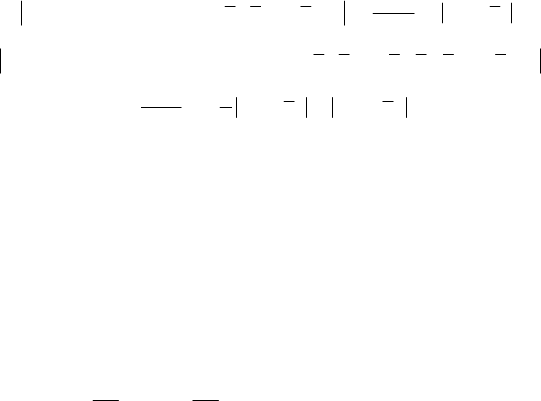
MECHANICAL SYSTEMS, CLASSICAL MODELS

where

12 12 12

( , ,..., ; ), ( , ,..., , , ,..., ; )

sss

jj jj

uuqq qt qq quu utϕϕ . This system is

autonomous; if the time does not intervene explicitly in

and

, then the system is

autonomous (or dynamical). We associate to this system the initial conditions (18.2.30)

in the form

===

( ) , ( ) , 1,2,...,

jjjj

qt q ut q j s ,

(18.2.48')

the problem at the limit (18.2.48), (18.2.48') being thus a problem of Cauchy type. The

problem (18.2.45'), (18.2.30) is equivalent to the problem (18.2.48), (18.2.48'); for the

latter one we can state

Theorem 18.2.1 (of existence and uniqueness; Cauchy–Lipschitz). If the functions

and

, = 1,2,...,js, are continuous on the interval +(2 1)s -dimensional D ,

specified by

00 0000 00

jjjjjjjjj

qQ qqQqU uqU− ≤≤+ − ≤≤+



tTt−≤

tT≤+,

, , const, 1,2,...,

QUT j s

, and definite on the space

Cartesian product of the phase space (of canonical co-ordinates

12 12

, ,..., , , ,...,

qq quu u) by the time space (of co-ordinate t ) and if Lipschitz’s

conditions

−≤−

−

⎡⎤

≤−+−

⎢⎥

⎣⎦

12 12

12 12 12 12

( , ,..., ; ) ( , ,..., ; ) ,

( , ,..., , , ,..., ; ) ( , ,..., , , ,..., ; )

kk k

ss ss

kkkkk

uqq qt uqq qt q q

qq quu ut qq quu ut

qq uu

ϕϕ

ντ

are satisfied for

= 1,2,...,js, where > 0T is a time constant independent on ,

and

,t τ is a time constant equal to unity, while ,,

λμν are constants equal to unity

(dimensionless if the corresponding generalized co-ordinate is a length and having the

dimension of a length if the corresponding generalized co-ordinate is non-

dimensional), then there exists a unique solution

==(), ()

jj jj

qqtuut of the system

(18.2.48), which satisfies the initial conditions (18.2.48') and is definite on the interval

−≤≤+

tTttT, where

() () () ()

()

⎡⎤

≤=

⎢⎥

⎣⎦

min , !, !, , max !, ! in

jj jjjj

TT u

λτμ μτνϕVTD

the sign (!) indicating “without summation”.

The continuity of the functions

u and

ϕ on the interval D ensures the existence of

the solution, according to Peano’s theorem. The condition of Lipschitz must be fulfilled

too for the uniqueness of the solution; these latter conditions may be replaced by other

ones less restrictive, in accordance with which the partial derivatives of the first order

of the functions

u and

ϕ , = 1,2,...,

s , must exist and be bounded in absolute value

Lagrangian Mechanics

on the interval

D . Besides, the conditions in the Theorem 18.2.1 are sufficient

conditions of existence and uniqueness, which are not necessary too.

The existence and the uniqueness of the solution have been put in evidence only on

the interval

−+

[, ]tTtT, in the neighbourhood of the initial moment

t (as a

matter of fact, the moment

t must not be the initial moment, but can be a moment

arbitrarily chosen); taking, e.g., the moment

tT as initial moment, it is possible –

repeating the above reasoning – to extend the solution on a interval of length

2T if,

naturally, the sufficient conditions of existence and uniqueness of the theorem are

verified in the neighbourhood of the new initial moment. Thus, we can prolong the

solution for

∈

[, ]ttt, corresponding to a certain interval of time in which the

considered mechanical phenomenon takes place, or even for

∈∞

[,)tt or ∈−∞

(,]tt

∈−∞∞(,)t .

We can state also theorems on the continuous or analytical dependence of the

solution on a parameter, analogous to the Theorems 6.1.3 and 6.1.4; as well, we can

state

Theorem 18.2.2 (on the differentiability of the solutions). If, in the neighbourhood of a

point

∈

12 1 2

( , ,..., , , ,..., ; )

qq quu utPD, the functions

( , ,..., ; )

uqq qt and

12 1 2

( , ,..., , , ,..., ; ), 1,2,...,

qq quu ut j sϕ , are of the class

C , then the solutions

()

and

()

of the system (18.2.48), which satisfy the initial conditions (18.2.48'),

are of class

+1k

C in a neighbourhood of the point P .

In particular, if

q and

() 0

, which leads also to

() 0

tϕ

= 1,2,...,

, the constraints being scleronomic, then we obtain

()

qt q

= 1,2,...,js, the solution being unique, according to the Theorem 18.2.1; hence, the

representative point is at rest with respect to a given fixed frame of reference.

18.2.3 Transformations. First Integrals

In the study of Lagrange’s equations an important rôle is played by the

transformations of co-ordinates and by the gauge transformations which will be

considered in what follows. As well, to integrate this system of equations, the problem

is put to find first integrals; in this order of ideas, we will put in evidence – especially –

the first integral of energy.

18.2.3.1 Point Transformations

We call point transformation a transformation of generalized co-ordinates of the

form

( , ,..., ; ), 1,2,...,

qqqq qtj s,

(18.2.49)

where

q are functions of class

with respect to the new generalized co-ordinates;

we assume that

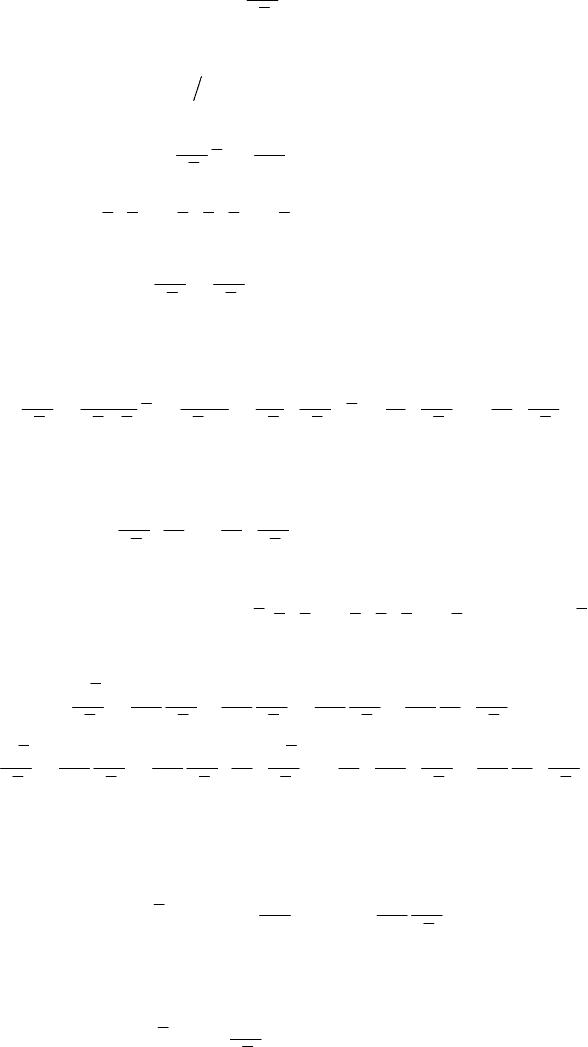
MECHANICAL SYSTEMS, CLASSICAL MODELS

∂

⎡⎤

≠

⎢⎥

∂

⎣⎦

det 0

(18.2.49')

the correspondence between the two systems of co-ordinates being one-to-one. In this

section, we will denote

=dd

qtq

and will have

∂∂

=+=

∂∂

, 1,2,...,

qq j s



(18.2.49'')

hence,

12 12

( , ,..., , , ,..., ; )

qqqq qqq qt

 

 , so that

∂∂

∂

, , 1,2,...,

jk s



(18.2.50)

One the other hand

∂∂ ∂ ∂ ∂ ∂

∂∂

⎛⎞ ⎛⎞ ⎛⎞

=+= +=

⎜⎟ ⎜⎟ ⎜⎟

∂∂∂ ∂∂∂∂ ∂∂ ∂

⎝⎠ ⎝⎠ ⎝⎠

jj j j j

kkl k lk k k

qq q q q q

qqq qtqq tq tq





wherefrom it result the relation between operators

()

∂∂

⎛⎞

⎜⎟

∂∂

⎝⎠

,1,2,...,

qt tq

(18.2.51)

By the transformation (18.2.49), (18.2.49'), and (18.2.49'') we obtain

12 12

( , ,..., , , ,..., ; )

Tq q q q q q t  =

12 12

( , ,..., , , ,..., ; )

Tq q q q q q t

 

, where T is a new

function which represents the kinetic energy in the generalized co-ordinates. We can write

∂∂∂ ∂

∂∂ ∂ ∂ ∂

⎛⎞

=+=+

⎜⎟

∂∂∂∂∂∂∂∂ ∂

⎝⎠

∂∂ ∂ ∂

∂∂ ∂ ∂ ∂ ∂

⎛⎞

== = +

⎜⎟

∂∂∂ ∂∂∂∂

∂∂ ∂

⎝⎠

dd d

jj j j

jj jj

kkkk k

jj j j

kkk

kk k

qqq q

TT T T T

qqqqq qqqtq

qq q q

TT T T T T

q qq t tq q qtq

qq q







 

  

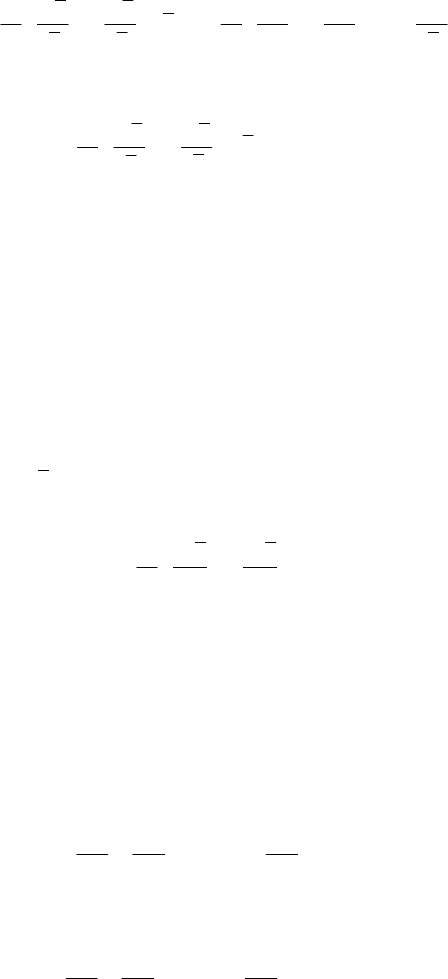
where we took into account (18.2.50), (18.2.51); analogously, the generalized forces

read

∂

∂∂

=⋅=⋅

∂∂∂

∑∑

qqq

wherefrom

∂

,1,2,...,

QQ k s

(18.2.52)

Lagrangian Mechanics

Thus, it results

∂

∂∂ ∂∂

⎡⎛ ⎞ ⎤

⎛⎞

−−= −−

⎜⎟

⎢⎥

∂∂∂∂

∂

⎝⎠

⎣⎝ ⎠ ⎦

TT TT

tq tqqq



(18.2.53)

Hence, if the equations (18.2.29) take place, then we will have

∂∂

⎛⎞

−= =

⎜⎟

∂

⎝⎠

,1,2,...,

Qk s,



(18.2.54)

too. On the other hand, taking into account the condition (18.2.49'), the relations

(18.2.54) entail the equations (18.2.29).

We can thus state

Theorem 18.2.3 Lagrange’s equations are invariant to a point transformation of the

form (18.2.49), (18.2.49') in case of a holonomic discrete mechanical system.

18.2.3.2 Gauge Transformations

We have seen in Sect. 18.2.2.2 that the motion of a natural mechanical system is

governed be Lagrange’s equations (18.2.38), for which it is necessary to determine

Lagrange’s kinetic potential

L . We notice that the Lagrangian

=,constKKL

leads to the same equations. One puts the problem to find a kinetic potential

′

LLL

, if this one exists, to obtain the same equations (18.2.38); in this case,

one must have

∂∂

⎛⎞

−≡

⎜⎟

∂∂

⎝⎠

tq q

(18.2.55)

for any

( ), 1,2,...,

qqtj s==.

For this, it is necessary that

=+ = = =

000

12 12

, ( , ,..., ; ), 1,2,.., , ( , ,..., ; ),

jj j j

aqaaaqqqtj saaqqqtL

otherwise the coefficients of the generalized accelerations

q cannot vanish, Replacing

in (18.2.25), it results (the point corresponds to a partial derivative with respect to time)

∂

⎛⎞

−+−≡=

⎜⎟

∂∂ ∂

⎝⎠

0, 1,2,...,

qa j s

qq q

 ,

so that we obtain the conditions

∂

−= −= =

∂∂ ∂

0, 0, , 1,2,...,

ajks

qq q

 .

Hence, it exists a function

( , ,..., ; )

qq qtϕϕ , so that

MECHANICAL SYSTEMS, CLASSICAL MODELS

∂

== =

∂

, 1,2,..., ,

ajsa

 .

We find thus

∂

=+=

∂

ϕϕ

L

(18.2.56)

We can state

Theorem 18.2.4 In case of a discrete mechanical system which admits a kinetic

potential

L , this one is determined excepting the total derivative with respect to time

of an arbitrary function of class

, of generalized co-ordinates and time.

In particular, the Lagrangian can be of the form (18.2.34).

This gauge transformation corresponds to the analogous transformation put in

evidence in Sect. 18.2.1.3 for a generalized quasi-potential.

18.2.3.3 First Integrals of Lagrange’s Equations

A function

12 12

( , ,..., , , ,..., ; )

fq q q q q q t  which is reduced to a constant along the

integral curves of Lagrange’s system of equations (18.2.29) is a first interval of this

system. If we determine

≤ 2ls first integrals

== =

12 12

( , ,..., , , ,..., ; ) , const, 1,2,...,

kkk

fqq qqq qt cc k l  ,

(18.2.57)

the matrix

∂

⎡

⎤

⎢

⎥

∂

⎣

⎦

12 12

( , ,..., )

( , ,..., , , ,..., )

ff f

qq qqq q

 

being of rank

l , then all these integrals are functionally independent (independent first

integrals) and we can express

l unknown functions of the system (18.2.29) by means

of the other ones; replacing in this system of equations, the problem is reduced to the

integration of a system of equations with

−2sl unknowns (hence, a smaller number of

unknowns). If

= 2ls, then all the first integrals are independent, so that the system

(18.2.57) of first integrals determines all the unknown functions. We notice that the first

integrals (18.2.57) are no more independent for

> 2ls; hence we can set up at the most

independent first integrals.

Solving the system (18.2.57) for

= 2ls, we obtain (the matrix M is a square matrix

of order

2s , for which ≠Mdet 0 )

===

12 2 12 2

( ; , ,..., ), ( ; , ,..., ), 1,2,...,

jj jj

qqtcc cqqtcc cj s ,

(18.2.58)

hence the general integral of the system of equations (18.2.29). Thus, one puts in

evidence

2s scalar constants of integration. Imposing the initial conditions (18.2.30),

we get

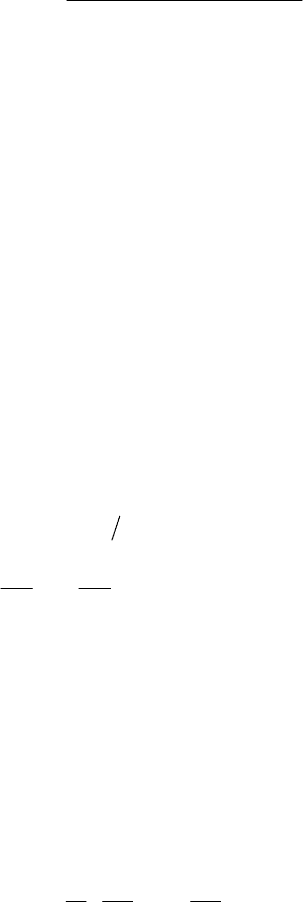
Lagrangian Mechanics

===

12 2 12 2

( ; , ,..., ) , ( ; , ,..., ) , 1,2,...,

jjjj

qtcccqqtcccqj s;

the conditions (18.2.30) being independent, we can write

∂

⎡⎤

≠

⎢⎥

∂

⎣⎦

00 000 0

12 12

12 2

( , ,..., , , ,..., )

det 0

( , ,..., )

qq qqq q

cc c

 

and – on the basis of the theorem of implicit functions – we deduce

00 000 0

12 12

( ; , ,..., , , ,..., ), 1,2,...,2

cctqq qqq qk s  .

Thus, it results, finally,

00 000 0

12 12

( ; , , ,..., , , ,..., )

q qttqq qqq q 

(18.2.59)

00 000 0

12 12

( ; , , ,..., , , ,..., )

q qttqq qqq q  .

Hence, in the frame of the conditions of the theorem of existence and uniqueness, the

principle of virtual work and the principle of initial conditions determine, univocally,

the motion of the discrete mechanical system

S , subjected to ideal and holonomic

constraints, in a finite interval of time; by prolongation, the statement can become valid

for any

t . Thus, the deterministic aspect of mechanics is put in evidence in its

Lagrangian presentation too.

fc and =

fc are two distinct first integrals, then =

(, )ff f c will be also

a first integral. Any other first integral is expressed, in general, as a function of the

distinct first integrals of Lagrange’s equations.

From (18.2.57) it results

=dd 0

ft ; hence

++==

0, 1,2,..,

qqfk l





(18.2.57')

relations which must be identically satisfied if one takes into account the equations of

motion (or the general integral of these equations, for any constants of integration).

18.2.3.4 First Integrals of Painlevé and Jacobi. First Integral of Mechanical

Energy

We can find a first integral particularly important and useful of the system of

equations (18.2.29), multiplying these equations by the generalized velocities

=, 1,2,...,

qj s , and summing for all indices

; we obtain

∂∂

⎛⎞

−=

⎜⎟

∂∂

⎝⎠

jjj

qqQq

tq q





We notice that

MECHANICAL SYSTEMS, CLASSICAL MODELS

∂∂∂

⎛⎞ ⎛ ⎞

=−

⎜⎟ ⎜ ⎟

∂∂∂

⎝⎠ ⎝ ⎠

jjj

TTT

qqq

tq tq q





Because

12 12

( , ,..., , , ,..., ; )

TTqq qqq qt  , we have, as well,

∂∂

=++

∂∂

TT T

qqT

tq q







so that

∂

⎛⎞

−= −

⎜⎟

∂

⎝⎠

jjj

qT QqT







(18.2.60)

Taking into account (18.2.15) and noting that

∂∂

qT qT



according to Euler’s theorem concerning the homogeneous functions, we can also write

()

−= −

TT QqT



(18.2.60')

If it exists a function

( , ,..., ; )

WWqq qt, so that −=dd

Qq T W t



(the

function

cannot depend on the generalized velocities too, because

ddWt

would

introduce also generalized accelerations), then we state Painlevé’s first integral

−−= =

,constTTWhh .

(18.2.61)

We notice that one must have

−=



, because they are terms which do not depend

on the generalized velocities

q ; as well, if the given forces

=F , 1,2,...,

, do not

depend on the velocities, hence if the generalized forces

=, 1,2,...,

Qj s

, do not

depend on the generalized velocities, then cannot appear quadratic terms in the

generalized velocities, resulting



. In these conditions, we state that Painlevé’s

first integral does not contain explicitly the time.

If the generalized forces admit a simple quasi-potential of the form (18.2.20), then

we can introduce the kinetic potential (18.2.34), so that the relation (18.2.60) reads

∂

⎛⎞

−+=

⎜⎟

∂

⎝⎠



;

(18.2.62)

assuming that the kinetic potential

L does not depend explicitly on time =(0)



L , we

get Jacobi’s first integral (Jacobi,C.G.J., 1882)