Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

Variational Principles. Canonical Transformations

261

and with the displacement acceleration

∂∂

=== =

∂



av u

(20.1.90'')

In this case, the kinetic energy density will be given by

uuμ= T ,

(20.1.91)

where

(;)tμμ= r is the density.

The work effected by the external given forces is stored by the elastic solid and

given back by unloading and bringing to the initial form as a work of internal forces (or

internal work); it is called also deformation work or strain energy. We denote by w the

unit deformation work (the deformation work density or the elastic potential), that is the

work corresponding to the unit volume in the vicinity of a point of the body, and which

the state of stress (the totality of stresses around a point) yields by the state of strain (the

totality of strains around a point) of the solid at the very same point. We call elementary

deformation work (elementary strain energy) the work corresponding to a volume

element

dΩ of the domain Ω occupied by the solid; we have

int

ddWwΩ= . (20.1.92)

The internal work is then given by

int

dWw

Ω

Ω=

∫

(20.1.92')

where

ij ij

w σε=

(20.1.93)

is the elastic potential. We have introduced the stress tensor

σ , ,1,2,3ij= (if ij=

we obtain the normal stresses, while if

ij≠ we obtain the tangential stresses). If we

take into account the linear constitutive law of R. Hooke

()

ij ij ij

σλεδ ε

⎡⎤

=−−

⎢⎥

⎣⎦

, ,1,2,3ij= ,

(20.1.94)

in case of a homogeneous, isotropic body, where λ is Lamé’s elastic constant and ν is

the transverse contraction coefficient of Poisson (Poisson’s ratio), we may write also

()

() 2

ij ij

w λε εε

⎡

⎤

=−−

⎢

⎥

⎣

⎦

(20.1.93')

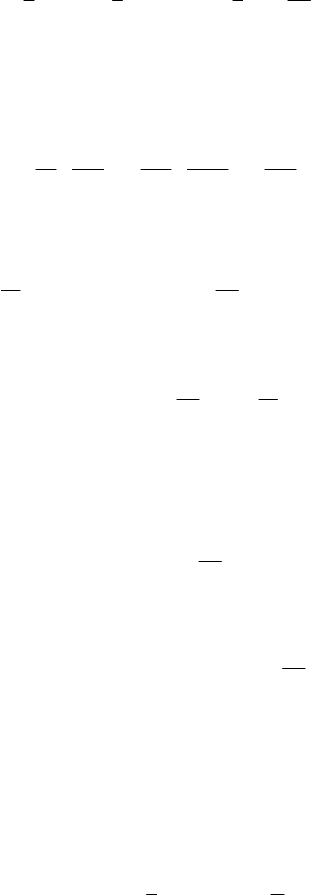
MECHANICAL SYSTEMS, CLASSICAL MODELS

262

Finally, the potential energy density is given by

w=−⋅V Fu

(20.1.95)

where

F is the volumic force (the external given force on the unit volume).

We find thus the kinetic potential density of Lagrange

()

,,,,,

11 11

() 1 ( )( )

22 22

j j jj ij ji ij ji

uu u u u u uμλ

⎡⎤

=− −− + ++⋅

⎢⎥

⎣⎦

L Fu;

(20.1.96)

Euler–Ostrogradskiĭ equations will be of the form (we remind observations in Sect.

20.1.1.3)

jijij

tu x u u

∂∂∂

⎛⎞ ⎛ ⎞

+−=

⎜⎟ ⎜ ⎟

∂∂∂

⎝⎠ ⎝ ⎠



LLL

, 1, 2, 3j = .

(20.1.96')

Finally,

()

,,,

() 1 ( ) 0

jijjiij

llj

uu uu F

μλ

∂

⎡⎤

−−− + +=

⎢⎥

∂

⎣⎦

 , 1, 2, 3

= ,

so that

(1 2 ) ( ) 0

jiij j j

uu F u

νμ

∂

⎡⎤

−Δ+ + − =

⎢⎥

∂

⎣⎦

 , 1, 2, 3j = .

(20.1.97)

We obtain thus the equations of motion of the homogeneous, isotropic linear elastic

solid, subjected to infinitesimal deformations; these equations, expressed in

displacements, are G. Lamé’s equations. If

constμ = , we can write

[]

(1 2 ) 0

jiij j j

uu F u

νμ

−Δ+ + − = , 1, 2, 3j = .

(20.1.97')

In vectorial form, we have

(1 2 ) grad div ( )

−Δ+ + −=



uuFu0,

(20.1.97'')

where the unknown function is the displacement vector

(;)t=uur.

20.1.5.4 Motion of Strings

Let be a string of length l, extended along the axis

Ox by a static tension

T ,

without any external given force; in this case, the kinetic energy is given by

33 33

Tux ux

∫∫

,

(20.1.98)

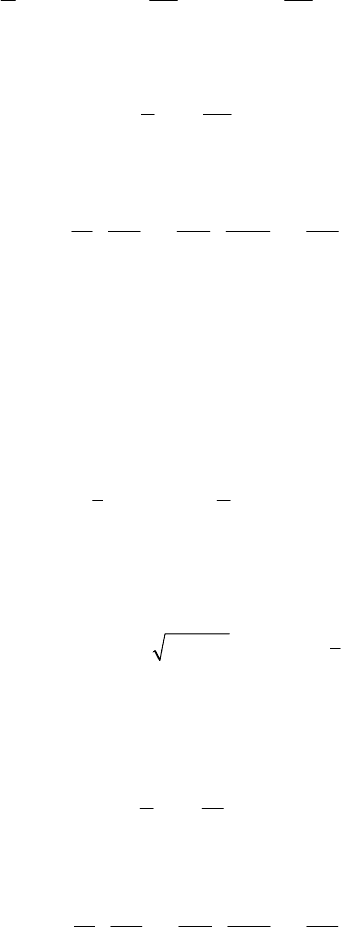
Variational Principles. Canonical Transformations

263

where

constμ = is the linear density of the string (see Sect. 12.2.1.3).

The potential energy is written in the form (we assume that both states of stress and

strain are one-dimensional)

33 33 3 33 3 3,3 3

000

dd d

222

lll

AEAEA

Vxxux

σε ε===

∫∫∫

(20.1.98')

In this case,

33,3

=−L .

(20.1.98'')

The Euler–Ostrogradskiĭ equation

333,33

ddtu x u u

∂∂∂

⎛⎞

+−=

⎜⎟

∂∂∂

⎝⎠



LLL

leads to the equation of longitudinal proper vibration of strings

3,33 3

0EAu uμ−= ,

(20.1.99)

corresponding to the equations (12.2.20') and (20.1.75').

In case of displacements normal to the axis, let be this one along the

Ox –axis, the

kinetic energy is given by

13 13

Tux ux

∫∫

,

(20.1.100)

the linear density being constant. The potential energy is calculated in the form (ds is

along the deformed form of the string; see also Fig. 12.8)

00 0

31,331,33

00 0

(d d ) ( 1 1)d d

ll l

VTsxT u xTux=−=+−=

∫∫ ∫

(20.1.100')

where we have expanded the integrand into a series after Newton’s binomial and we

have neglected terms of superior order (corresponding to the linearization hypothesis).

We obtain

11,3

=−L .

(20.1.100'')

The Euler–Ostrogradskiĭ equation

131,31

ddtu x u u

∂∂∂

⎛⎞

+−=

⎜⎟

∂∂∂

⎝⎠



LLL

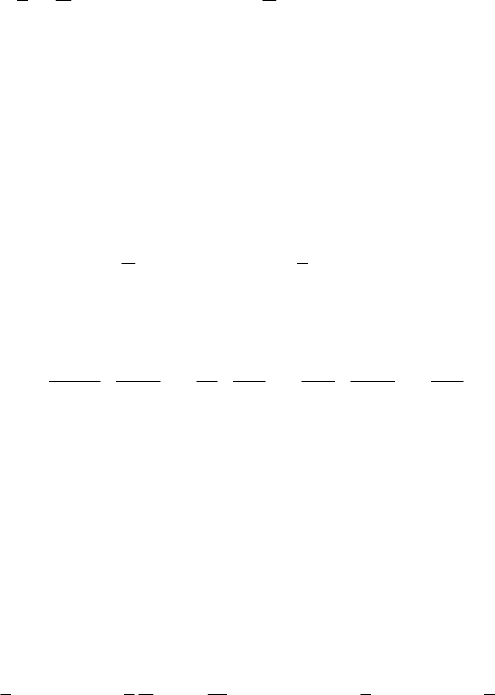
MECHANICAL SYSTEMS, CLASSICAL MODELS

264

leads to

11,33

0uTuμ −= ,

(20.1.101)

hence to the equation of proper transverse vibrations of strings, corresponding to the

equations (12.2.22').

20.1.5.5 Motion of Bars

In case of a straight bar one must take into account the transverse contraction, so that

the transverse displacements of the bar

Ox –axis will be

113,3

uxuν=− ,

223,3

uxuν=− , the corresponding displacement velocities being given by

113,3

uxuν=−,

223,3

uxuν=−. We obtain the kinetic energy

()()

222 2222

123 3 3,33

Tuuu uiux

Ω

μμ

Ων

=++=+

∫∫

   ,

(20.1.102)

where

i is the gyration radius of the cross section with respect to the pole O, given by

()

222

Ai x x A=+

∫∫

(20.1.102')

while

μ is the linear density, constant on the cross section. The potential energy is given

by a formula of the form (20.1.98'), so that

()

2222 2

33,3 3,3

uiu EAu

=+ −L .

(20.1.102'')

The Euler–Ostrogradskiĭ equation (in the form of the equation (7.2.11'))

33,3 3 33,3 3

ddd

dd d d

xt u t u x u u

∂∂ ∂∂

⎛⎞ ⎛⎞

⎛⎞

−− +=

⎜⎟

⎜⎟ ⎜⎟

∂∂∂∂

⎝⎠

⎝⎠ ⎝⎠



LL LL

leads to the A.E.H. Love’s equation

()

3,33 3 3,33

EAu u i uμν−− =  .

(20.1.103)

Neglecting the term which multiplies

ν , we find the longitudinal proper vibrations

equation of the straight bars in the classical form (20.1.99), in fact the equation

(12.2.42).

In case of the transverse vibrations of the straight bar, the kinetic energy due to the

motion of translation and to the rotation of the cross section is

13 2 1,3 3

d()d

TuxIux

∂

⎡⎤

⎢⎥

∂

⎣⎦

∫∫



222

13 2 1,33

ux i u xμμ=+

∫∫



(20.1.104)

Variational Principles. Canonical Transformations

265

where

I is the moment of inertia with respect to the neutral axis of the bar (

Ox -axis),

i being the corresponding gyration radius. Observing that

33 1 1,33

xuε =− ,

33 33

Eσε= , we can write

22 2

1,33 3 1 1 2 2 1,33

ddd

VEuxxxx EIu==

∫∫

(20.1.104')

so that

()

222 2

1 2 1,3 2 1,33

uiu EIuμ=+−L .

(20.1.104'')

As in the preceding case, the Euler–Ostrogradskiĭ equation

3 1,3 1,33 1 3 1,3 1

dddd

dd d d

xt u u t u x u u

∂∂∂∂∂

⎛⎞ ⎛ ⎞ ⎛⎞

⎛⎞

+−−+=

⎜⎟

⎜⎟ ⎜ ⎟ ⎜⎟

∂∂∂∂∂

⎝⎠

⎝⎠ ⎝ ⎠ ⎝⎠



LLLLL

leads to the proper transverse vibrations equation of the straight bar in the form

()

2 1,3333 1 2 1,33

0EI u u i uμ+− =  ,

(20.1.105)

corresponding to the equation (12.2.47).

20.2 Canonical Transformations

In general, applying directly the Hamiltonian formalism (instead of the Lagrangian

one), the difficulty to solve a problem of mechanics does not diminish sensibly; the

differential equations are practically equivalent. The advantage of using a Hamiltonian

formalism consists – particularly – in a profound understanding of the formal structure

of mechanics; there appears a greater liberty to choose canonical co-ordinates

(generalized co-ordinates and momenta). The corresponding formulations, just because

of their abstraction, allow to elaborate new theories concerning matter, nature,

Universe; they constitute a starting point for quantum as well as for statistical

mechanics.

In Sect. 18.2.3.1 we have seen that point transformations let invariant Lagrange’s

equations; a study of the transformations which let invariant Hamilton’s equations is

thus necessary, so that the use of the Hamiltonian formalism is once more justified.

20.2.1 General Considerations. Conditions of Canonicity

In what follows, canonical transformations are introduced; especially, accent is put

on the statement of canonicity conditions. We mention also various applications,

including those in the perturbation theory. A particular attention is given to

infinitesimal canonical transformations.

MECHANICAL SYSTEMS, CLASSICAL MODELS

266

20.2.1.1 Preliminary Considerations. Examples

Let be a transformation of canonical variables of the form

()

QQqpt= ,

()

PPqpt= , 1,2,...,

s= , (20.2.1)

where

Q and

P are functions of class

C , having the Jacobian

()

det 0

∂

⎡⎤

=≠

⎢⎥

⎣⎦

(20.2.1')

with

{

}

, ,...,

QQQ Q≡ ,

{

}

, ,...,

PPP P≡ ,

{

}

, ,...,

qqq q≡ ,

{

,,ppp≡

}

...,

p ; the time

plays the rôle of a parameter in this transformation.

Hamilton’s function becomes

()

,;Hqpt =

()(

,;,HqQPt

())

,; ;pQPt t

()

,;HQPt= . The transformation (20.2.1), (20.2.1') is called canonical

transformation if Hamilton’s canonical system (19.1.14) keeps its form, hence if

∂



∂

=−



1,2,...,

(20.2.2)

The theorem of implicit functions and the condition (20.2.1') ensure the existence of

the inverse transformation

()

qqQPt=

()

ppQPt=

, 1,2,...,ks= , (20.2.1'')

these functions being also of class

C . The canonical co-ordinates ,qp and ,QP

correspond to the same representative point in the space

Γ .

The transformations (20.2.1), (20.2.1'), and (20.2.1'') are called complete canonical

transformations if they do not depend explicitly on time. Together with A. Sommerfeld,

these transformations are called contact transformations too (Sommerfeld, A., 1962).

Other authors give this denomination to the transformations (20.2.1) to which we add

the function

()

,;TTqpt= , (20.2.3)

of the same class

C (hence, for which t is no more a parameter), so that

()

det 0

QPT

qpt

∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.3')

But it is not necessary to consider such transformations in the following study; they can

be useful in a relativistic modelling of mechanical phenomena.

Starting from canonical equations of a complicated form, we can obtain simpler

equations of motion by means of canonical transformations; practically, the

Hamiltonian

H can have a simpler structure than Hamilton’s function H . In particular,

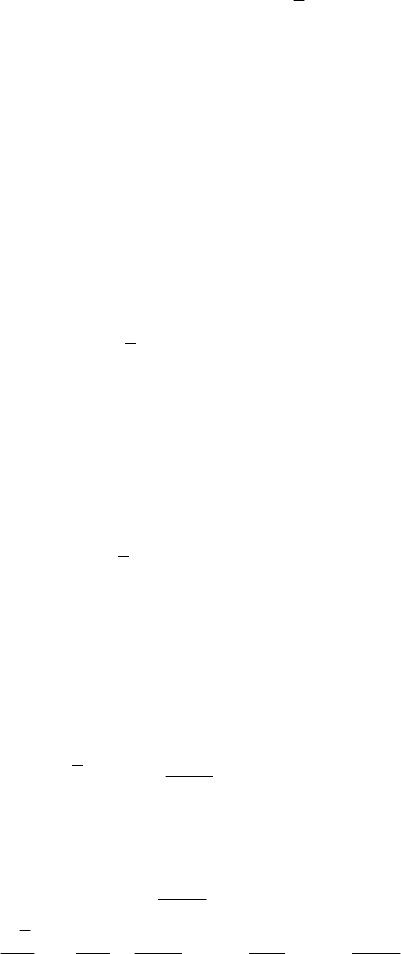
Variational Principles. Canonical Transformations

267

if we find the canonical transformation for which

constH = (independent of Q and

P ) along the trajectory of the representative point, then the solution of the canonical

system (20.2.2) will be

QA= ,

PB= , 1,2,...,js= ,

(20.2.4)

where

A and

B are constants; the functions (20.2.1) are, in this case, first integrals

of the canonical system (19.1.14).

Let be the transformation

Qqα= ,

Ppδ= , 1,2,...,

s= ,

(20.2.5)

where

α and δ are non-vanishing constants; the equations (20.2.2) are easily verified,

together with the equations (19.1.14), in case of the new Hamiltonian

HHαδ= .

(20.2.5')

Hence, this transformation is a complete canonical transformation.

As well, the transformation

Qpβ= ,

Pqγ= , 1,2,...,js= , (20.2.6)

where

β and γ are non-zero constants, and for which

HHβγ=− ,

(20.2.6')

is also a complete canonical transformation.

Analogically, we can show that the transformation

tan

Qp t= , cot

Pq t= ,

1,2,...,

, (20.2.7)

with

sin2

HH QP

=− + ,

(20.2.7')

is a canonical one. The direct proof of this affirmation is rather complicated. For

instance, we have

tan

cos

jjj

Qppt



tan

sin2

cos

jj j

HH H

Qtp

PP t Q

∂∂ ∂

=− + =− + ;

taking into account (19.1.14), the first equation (20.2.2) is verified.

As well, it is not completely clear how one can obtain such transformations. For this

goal, we will give – in what follows – various conditions of canonicity and will put in

evidence the possibility to construct canonical transformations.

MECHANICAL SYSTEMS, CLASSICAL MODELS

268

20.2.1.2 Complete Canonical Transformations

Let be a complete canonical transformation

(, )

QQqp= , (, )

PPqp= , 1,2,..,

s= ,

(20.2.8)

where

Q ,

P are functions of class

C which verify the condition (20.2.1'). We

consider the differential form of the first degree (the one-form)

pq PQψ =−. (20.2.9)

The canonical form (20.1.41) of Hamilton’s principle leads to

()

[]

()

[]

d,;d ,;d

pq Hqpt t PdQ HQPt tδδ−=−

∫∫

To obtain the canonical equations (20.2.2), corresponding to Livens’s Theorem 20.1.6,

as a consequence of the complete canonical transformation (20.2.1), it is sufficient that

the one-form (20.2.9) be an exact differential, hence

d0ψ = ; as a matter of fact, this

represents a sufficient condition of complete canonicity for the transformation (20.2.8).

On the basis of Poincaré’s lemma, we have

d(d ) 0ψ = , the operator

being Cartan’s

external differential operator (see App., Subsec. 1.2.2). Corresponding to the reciprocal

of this lemma, if

d0ψ = , then there exists a scalar function

so that

dVψ = , (20.2.9')

with

()

,;,VVqpQP=

, which cannot depend explicitly on time; in fact, the function

V can depend only on two from the four sets of co-ordinates ,,qpQ and P .

Corresponding to the form (19.1.45), we can write Pfaff’s forms of Hamilton

()

d,;d

pq Hqpt tω =− ,

()

d,;d

PQ HQPt tΩ =− .

(20.2.10)

We have proved in Sect. 19.1.1.9 that the two forms have the same bilinear covariant,

hence they lead both to the same canonical equations (the same associate differential

system), if and only if

dcVΩω=− , (20.2.10')

where

c is a constant, while

()

,;,VVqpQP= is a function of class

C ; we make

the same observation as above for the arguments of the function

V . The necessity of

introducing the constant

c will be clearly put in evidence in Sect. 21.1.2.3, in the study

of integral invariants. We notice that

()

dd dd

ccpqPQcHHtVωΩ−= − − − = ;

because

/0VVt∂∂==



, it follows

Variational Principles. Canonical Transformations

269

HcH= .

(20.2.10'')

We introduce thus the differential form

ddd

cp q P Q Vψ =−=

0c ≠

constc =

. (20.2.11)

The constant

c is called the valency of the canonical transformation. If 1c = , then the

transformation is univalent; in this case

HH= .

Usually, one has to do with univalent canonical transformations. We observe that the

condition (20.2.9') (with

1c =

) is always sufficient; in some particular cases, it is also

necessary (the transformations are univalent).

In the particular case in which

0V =

, the complete canonical transformation is

called, together with E. Mathieu, homogeneous, and the relation

kk k k

pq PQ=

(20.2.9'')

takes place; in this case, if the variable

p is multiplied by a factor, then the variable

P is multiplied by the same factor.

Taking into account (20.2.9), (20.2.9'), we may write

dddddp

kk k k k k

kk kk

pq P q p q

qp qp

∂∂

∂∂ ∂∂

⎛⎞

−+=+

⎜⎟

⎝⎠

where we have considered

(, )VVqp= ; there results

∂

∂∂

−=

∂

∂∂

−=

, 1,2,...,ks= .

Eliminating the function

V between these relations or (it is the same thing) writing

that the mixed derivatives of second order of this function

ll l

jjj

kkk

PQ Q

q q qq qq

∂∂ ∂

∂

∂ ∂ ∂∂ ∂∂

−− =,

ll l

kkk

PQ Q

qq qq qq

∂∂ ∂

∂

∂∂ ∂∂ ∂∂

−− =,

ll l

jjj

kkk

PQ Q

pp pp pp

∂∂ ∂

∂

∂∂ ∂∂ ∂∂

−− =

ll l

kkk

PQ Q

p p pp pp

∂∂ ∂

∂

∂∂ ∂∂ ∂∂

−− =

ll l

jk l

jjj

kkk

PQ Q

pq pq pq

∂∂ ∂

∂

∂ ∂ ∂∂ ∂∂

−− =,

ll l

kkk

PQ Q

qp qp qp

∂∂ ∂

∂

∂ ∂ ∂∂ ∂∂

−− =

do not depend on the order of differentiation, we obtain the conditions of complete

canonicity of the considered transformation in the form

[

]

qq = ,

[

]

pp = ,

[

]

kjk

qp δ= , , 1,2,...,jk s= ,

(20.2.12)

MECHANICAL SYSTEMS, CLASSICAL MODELS

270

where

δ is Kronecker’s symbol; we can write

[

]

2 ( 1)/2 (2 1)ss s s s−+=− such

conditions. We have introduced the Lagrange brackets by relations of the form

(19.1.50), where

qp, 1,2,...,js= , play the rôle of integration constants in the

integrals (20.2.8) of the canonical system (20.2.2). The conditions (20.2.12) can be

written also in the form

kl l

pP pP

qqqq

∂∂

∂∂∂∂

⎛⎞

−=−

⎜⎟

⎝⎠

pppp

∂∂

∂∂ ∂∂

⎛⎞

⎜⎟

⎝⎠

kl l

pP P

pqqp

∂∂

∂∂∂∂

⎛⎞

−=−

⎜⎟

⎝⎠

;

there exists thus a function V so that /

Vq∂∂ and /

Vp∂∂ be given by the relations

from which we started. We obtain then (20.2.9) and (20.2.9'). Hence, the conditions

(20.2.12) are equivalent to (20.2.9), (20.2.9'), being thus sufficient conditions of

complete canonicity.

1c ≠ , then the conditions (20.2.12) take the form

[

]

qq = ,

[

]

pp = ,

[

]

kjk

qp cδ= , , 1,2,...,jk s= ,

(20.2.12')

being necessary and sufficient conditions of complete canonicity.

Obviously, we can write also the sufficient conditions of complete canonicity

[

]

QQ = ,

[

]

PP = ,

[

]

kjk

QP δ= , , 1,2,...,

ks= ,

(20.2.12'')

corresponding to the inverse complete canonical transformations. As well, if

1c ≠ ,

then we may write the necessary and sufficient conditions of complete canonicity

[

]

QQ =

[

]

PP =

[]

kjk

δ= ,

, 1,2,...,

ks=

(20.2.12''')

Using the Poisson brackets (defined by the relation (19.1.54)), we see that one can

write

()

jj j j

kk kkkk

QQ Q Q

Qqp QH

qpqppq

∂∂ ∂ ∂

∂∂

∂∂ ∂∂∂∂

=+= − =





()

PPH=



along the integral curves of the canonical system (19.1.14); as well, we have

ddd

Qqp

∂∂

=+, ddd

Pqp

∂∂

=+.

Taking into account the Lagrange brackets (20.2.12), we obtain