Teodorescu P.P. Mechanical Systems, Volume III: Analytical Mechanics

Подождите немного. Документ загружается.

Variational Principles. Canonical Transformations

281

again the transformation (20.2.5), which is not free; as well, if

0αδ==, then we

obtain the transformation (20.2.6), which is free. We mention that the transformation

(20.2.7) is also a free transformation too.

For a natural mechanical system, the generalized co-ordinates

, ,...,

qq q define its

position and, together with the generalized momenta

, ,...,

pp p, define its state

(positions and velocities of its points). But this specificity of canonical co-ordinates

does not remain valid, in general, in case of a canonical transformation; indeed, the

quantities

, ,...,

QQ Q do no more define the position of the mechanical system, but

define its state only together with the quantities

, ,....,

PP P. The quantities

, ,...,

QQ Q define the position of the mechanical system only in the case of a point

transformation. However, the non-point canonical transformations are important in the

theory of Hamiltonian systems, because they can lead to a Hamiltonian having a

simpler structure.

Let be the canonical transformation generated by the function

()

SQq Qq= ; (20.2.31)

we obtain

Qp= ,

Pq=− , 1,2,...,js= . (20.2.31')

The canonical co-ordinates invert their rôles, the above affirmations being thus

justified. At the same time, the denomination of conjugate co-ordinates given to

q and

p is also justified.

In Sect. 19.2.1.6 we have introduced Hamilton’s principal function

S , which

satisfies the Hamilton–Jacobi equation; indeed, taking into account the deterministic

character of mechanics (we have

[

]

det / 0

qp∂∂ ≠ ) and using the final equations of

motion (20.2.20), Hamilton obtained

()

000

ppqqt= , 1,2,...,ks= . In this case,

the function

()

,;SSqqt= will be a generating function of canonical

transformations, so that the transformation (20.2.20) can be considered as a univalent

free canonical transformation.

20.2.1.6 Other Functions Generating Canonical Transformations

We notice that

()

ddd

jj j j j j

QP P Q Q P=+; the sufficient condition of canonicity

(20.2.17), (20.2.17') has the form

()

dd dd

pq QP H H t W+−−=,

WWQP=+

in this case. Assuming the supplementary condition

det 0

∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.32)

MECHANICAL SYSTEMS, CLASSICAL MODELS

282

the second group of relations (20.2.1) leads to

()

ppPqt= and then to

()

QQPqt= ,

, 1,2,...,

ks=

; as well, the function

W can be expressed in the

form

()()

,,,; ,;WQPqpt S Pqt= . The condition of canonicity allows us to write

∂

, 1,2,...,

ks=

(20.2.33)

as well as

HHS=+



(20.2.33')

If the Hessian

det 0

∂

∂∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.32')

then we obtain the canonical transformation (20.2.1) from the first subsystem (20.2.33).

Hence, a function

()

,;SPqt of class

C , which verifies the condition (20.2.32'),

determines a canonical transformation (20.2.33), (20.2.33').

As well, the relation

()

ddd

kk k k k k

qp p q q p=+ leads to the sufficient condition of

canonicity

()

dd dd

qp PQ H H t W−− −− =,

WWqp=− .

If the supplementary condition

det 0

∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.34)

is fulfilled, then the first group of relations (20.2.1) leads to

()

qqQpt= , so that

()

PPQpt= ,

, 1,2,...,

ks=

; analogically, the function

W can be expressed in

the form

()()

,,,; ,;W QPqpt S Qpt= . The sufficient condition of canonicity leads

to the representation

∂

=−

∂

=−

, 1,2,...,

ks=

(20.2.35)

as well as to

HHS=+



(20.2.35')

If the Hessian

det 0

∂

∂∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.34')

Variational Principles. Canonical Transformations

283

then we obtain the generalized co-ordinates

Q from the first subsystem (20.2.35);

replacing then in the second subsystem (20.2.35), the canonical transformation (20.2.1)

is completely specified. By way of consequence, a function

()

,;SQpt of class

C ,

which verifies the condition (20.2.34'), leads to a canonical transformation (20.2.35),

(20.2.35').

Analogically, using the relations

()

ddd

jj j j j j

QP P Q Q P=+,

()

ddd

kk k k k k

qp p q q p=+,

the sufficient condition of canonicity

()

dd dd

qp QP H H t W−+ −− =,

WWqpQP=− +

is resulting. If

det 0

∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.36)

then the second group of relations (20.2.1) allows us to write

()

qqPpt= and

then

()

QQPpt= ,

, 1,2,...,

ks=

, wherefrom

()()

,,,; ,;W QPqpt S P pt= .

By means of the sufficient canonicity condition, we obtain the representation

∂

=−

∂

, , 1,2,...,jk s= ,

(20.2.37)

as well as

HHS=+



(20.2.37')

We assume that

det 0

∂

∂∂

⎡⎤

≠

⎢⎥

⎣⎦

(20.2.36')

In this case, from the first subsystem (20.2.37) there result the generalized momenta

P ; replacing in the second subsystem (20.2.37), we obtain also the generalized co-

ordinates

Q , the canonical transformation (20.2.1) being thus specified. Wherefrom, a

function

()

,;SPpt of class

C , which verifies the condition (20.2.36'), determines

the canonical transformation (20.2.37), (20.2.37').

Using the necessary and sufficient condition of canonicity (20.2.18'), we obtain

representations corresponding to canonical transformations of non-unitary valency

c . If

the functions which occur do not depend explicitly on time (for instance,

()

,SSPq= ,

()

,SSQp= ,

()

,SSPp= ), then one obtains representations

corresponding to complete canonical transformations.

MECHANICAL SYSTEMS, CLASSICAL MODELS

284

Starting from the free transformation (20.2.27), (20.2.28) with

0H = , we find again

the Hamilton–Jacobi equation (19.2.9); one obtains the same result starting from the

transformation (20.2.33), (20.2.33'). As well, starting from the transformation (20.2.35),

(20.2.35') or from the transformation (20.2.37), (20.2.37'), we find again the Hamilton-

Jacobi type equation (19.2.68).

As an application, let us consider the generating function

()()

,; ;

SPqt fqtP= , (20.2.38)

for which

det det 0

Pq q

∂

∂∂ ∂

⎡⎤

=≠

⎢⎥

⎣⎦

(20.2.38')

The formulae (20.2.33) allow us to write

()

;

Qfqt= ,

∂

, , 1,2,...,jk s= ,

(20.2.38'')

obtaining thus a point transformation; if we take into account (20.2.38'), then we may

calculate

()

PPqpt= from the second relation (20.2.38''). We also notice that

HHfP=+



(20.2.38''')

finding thus again the results of Sect. 20. 2.1.4. An interesting particular case is

SqP= , (20.2.39)

to which corresponds the identical transformation

Qq= ,

Pp= , 1,2,...,js= . (20.2.39')

As well, let

qp be a stationary point for an autonomous system (which does not

depend explicitly on

t ) of Hamiltonian H , so that

qq= ,

pp= , 1,2,...,

s= ,

is a solution for the canonical equations. The generating function

()( )

jj j j

SqqPp=− −

(20.2.40)

leads to the transformation

jjj

Qqq=+,

jjj

Ppp=+, 1,2,...,js= ,

(20.2.40')

which measures the deviation with respect to the equilibrium solution. More general,

may be

()

qqt= and

()

ppt= , 1,2,...,js= , do represent a known solution

Variational Principles. Canonical Transformations

285

of the canonical equations of an autonomous system; it can be a first application in the

perturbation theory, which will be considered in the next subsection.

20.2.1.7 Applications to the Perturbation Theory

Let (20.2.20) be the solution of the canonical equations (19.1.14) of a mechanical

system of Hamiltonian

and initial conditions

()

qqt= and

()

ppt= ; we try

now to obtain the motion of a "perturbed" system of Hamiltonian

HH+

, hence the

solution of the canonical system

()

qHH

∂



()

pHH

∂

=− +



1,2,...,ks=

(20.2.41)

If the initial conditions are considered as new variables in (20.2.20), then these

formulae determine a univalent free canonical transformation (as we have seen in Sects.

20.2.1.3 and 20.2.1.5), which transforms the canonical system (19.1.14) into a

canonical system of Hamiltonian

0H =



, so that

q =



and

p =



, 1,2,...,

s= ;

as well, (20.2.20) transforms the canonical system (20.2.41) into a canonical system of

Hamiltonian

HH=



(because

()

0HHH HS−+ =−=





, where S is the

generating function), from which

∂



∂

=−



, 1,2,...,js= .

(20.2.42)

So, the variables

q and

p have the following property: they are constant for the non-

perturbed motion (equal to the initial conditions), while for the perturbed motion they

are functions of time and initial values; by convention, we represent these variables in

the form

[] []

()

qqqpt= ,

[] []

()

ppqpt= , 1,2,...,ks= , being – in fact

– the general solution of the system (20.2.20), in which the Hamiltonian is the

"perturbed energy"

H . By replacing this function in formulae (20.2.20), we find the

solution corresponding to the perturbed motion.

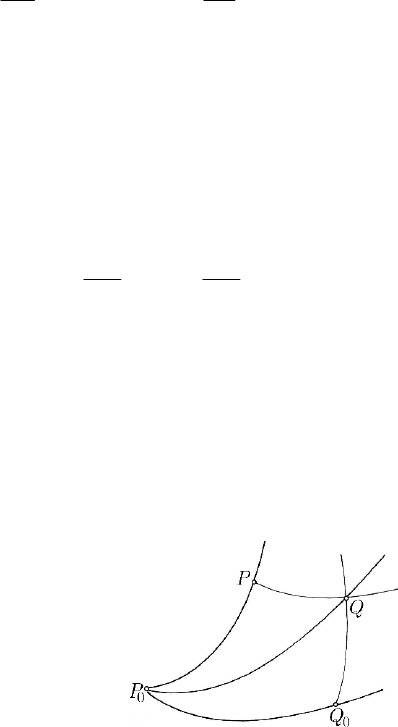
Fig. 20.5. Perturbed motion of a particle.

Using the theory of the canonical transformations, the integration of the canonical

system (20.2.41) was replaced by the integration of the canonical system (19.1.14),

(20.2.42); the general solution of the system (20.2.41) will thus be

MECHANICAL SYSTEMS, CLASSICAL MODELS

286

[]

()

[]

()

[]

()

[]

()

000 000

,;, ,; ,

, ; , , ; , 1,2,..., .

q q q q pt p qpt

p pq qpt p qpt j s

(20.2.41')

We notice that the “energy perturbation” of the mechanical system is equivalent to the

perturbation of the “initial conditions”.

Let us consider a fixed representative point

P in the hyperplane

tt= and let us

build up in this hyperplane a non-perturbed trajectory

PP, given by (20.2.20); as well,

the trajectory

PQ in the same hyperplane corresponds to the displacement of the

initial position

P (at the moment

t ) till the initial position

Q (at the moment t ),

given by (20.2.41') (Fig. 20.5). From the representative point

Q , let us set up the non-

perturbed corresponding trajectory

QQ. The representative point

will correspond

to the position of the system in the perturbed motion at the moment

t , while

PQ will

correspond to the trajectory in the perturbed motion. The perturbation is thus put in

evidence by the “displacement”

. Thus, the perturbed motion can be considered as

a “compound motion” in the phase space; the representative point is in motion along the

non-perturbed trajectory, but this trajectory is displaced (in general, deformed) because

of the perturbations of the initial conditions.

20.2.1.8 Infinitesimal Canonical Transformations

Let be the infinitesimal transformation

()

jj jj j

Qq qq fqptδε=+ =+ ,

()

jj jj j

Pp pp gqptδε=+ =+ ,

1,2,...,

(20.2.43)

where

ε is a small parameter. One can obtain such a transformation starting from the

canonical transformation

()

,;;

QQqptλ= ,

()

,;;

PPqptλ= , where λ is a

parameter which for

λλ= leads to the identical transformation (20.2.40'); if we put

λλ ε=+

, and expand into a series after the powers of ε , neglecting

()

εO , then

we find the canonical transformation (20.2.43), which is completely determined if we

know the functions

f and

g ,

1,2,...,

. Hamilton’s function is transformed in the

form

HH HH Hδε=+ =+ ,

(20.2.43')

while the generating function will be of the form

SSqPKδε+= +, (20.2.43'')

where

()

,;KKqPt= ; we notice that

()()

,; ,; ()KqPt Kqpt ε=+O . Applying the

formulae (20.2.33), one has

∂

=+ ,

∂

=− , 1,2,...,js= ,

(20.2.44)

Variational Principles. Canonical Transformations

287

as well as

HH Kε=+



;

(20.2.44')

hence

∂

δεε

∂

δε ε

∂

==−

HH Kδε ε==



(20.2.44'')

Thus, for each function

()

,;KKqpt=

of class

one obtains an infinitesimal

canonical transformation; as a matter of fact, taking into account the condition

(20.2.32'), the function

K must be of class

C . If

()

,KKqp= , non-depending

explicitly on time, then

HH= , and the transformation is an infinitesimal complete

canonical transformation. In this case, let

()

,Fqp be a function of class

C ; for an

infinitesimal complete canonical transformation given by (20.2.44) we have a variation

of the form

()

jj jjjj

FF FKFK

Fq p FK

qp qppq

∂ ∂ ∂∂ ∂∂

δδ δε ε

∂∂ ∂∂∂∂

⎛⎞

=+ = − =

⎜⎟

⎝⎠

(20.2.45)

where a Poisson bracket has been put in evidence. The operator

jjj

pq qp

∂∂ ∂∂

=−

(20.2.45')

is the operator of the infinitesimal canonical transformation; in this case

DFFδε=

. (20.2.45'')

We notice that the infinitesimal canonical transformation (20.2.44) transforms the

function

F in itself if

()

,0FK = . (20.2.45''')

In particular, for

Fq= and

Fp= respectively, we get

()

kk k

qqK qδε ε==,

()

kk k

ppK pδε ε==, 1,2,...,ks= ;

(20.2.46)

we can thus express the variations of the canonical co-ordinates, hence the infinitesimal

complete canonical transformation corresponding to a generating function

()

,Kqp of

class

C by means of a Poisson bracket, using the relations (20.2.46). By these

relations one can show that the infinitesimal generator of the spatial translations along a

fixed direction is the projection of the linear momentum of the mechanical system on

the same direction; as well, the infinitesimal generator of the spatial rotations about a

fixed axis is the projection of the angular momentum of the mechanical system on the

very same axis.

MECHANICAL SYSTEMS, CLASSICAL MODELS

288

FH= in (20.2.45), then we have

()

,HHKδε= ; (20.2.46')

comparing with the third relation (20.2.44''), we obtain (

()()

,,HK KH=− )

()

,0KH K+=



(20.2.47)

Taking into account Theorem 19.1.5, we may state that the generating function

K is a

first integral of the canonical system (19.1.14). As well, we may state that the first

integrals of the motion are generators of the infinitesimal canonical transformations.

Particularly, for

KH= we see that we must have 0H =



, hence

()

,HHqp= ;

wherefrom, an infinitesimal canonical transformation for which the Hamiltonian is a

generating function is an infinitesimal complete canonical transformation. If, in this

case, we put

tεδ=

, introducing a time variation, then we find again Hamilton’s

equations written by means of the Poisson brackets in the form

()

qqHtδδ= ,

()

ppHtδδ= , 1,2,...,ks= .

(20.2.48)

To pass from the co-ordinates

,qp at the moment t to the co-ordinates qq+δ ,

pp+δ at the moment tt+δ , it is sufficient to effect an infinitesimal complete

canonical transformation of the co-ordinates

,qp, of small parameter tδ and of

generating function

()

,Hqp. Especially, starting from the initial values of the

generalized co-ordinates, we attain their values at a neighbouring moment, obtaining

the incipient motion. One may thus state that the motion of the representative point on

its trajectory is obtained by a succession of infinitesimal complete canonical

transformations. Hence, the motion of a mechanical system corresponds to the

evolution or to the continuous development of a canonical transformation; we can say

that the Hamiltonian is the generator of this motion in time. If

D0F = , then

()

,Fqp

is an invariant of the canonical transformation, hence a first integral of the equations of

motion.

We can consider the equations (20.2.48) as being the canonical equations written in

finite differences; we are thus led to an iterative method to integrate them (obtaining a

finite number of points of the trajectory of the representative point).

Let

()

qt,

()

pt and

()

qt qδ+ ,

()

pt pδ+ , 1,2,...,ks= , be two

neighbouring solutions of the system of canonical equations (19.1.14), corresponding to

two neighbouring initial states. In this case, the functions

()

qtδ and ()

ptδ , of class

C , verify the equations of the variations

qqp

tpq pp

∂∂

δδδ

∂∂ ∂∂

=+,

pqp

tqqqp

∂∂

δδδ

∂∂ ∂∂

=− − ,

1,2,...,

(20.2.49)

The knowledge of a first integral

()

,; constfqpt = of the canonical system leads to

the obtaining of a particular solution for these equations. We will thus show that

Variational Principles. Canonical Transformations

289

∂

δε

∂

= ,

∂

δε

∂

=− , 1,2,...,js= ,

(20.2.49')

are the solutions of the equations (20.2.49). Indeed, introducing in these equations, we

have

jj j

kk kk kk kk

ff ff

HH HH

t pqp ppq p qp pq

∂∂ ∂∂

∂∂ ∂∂∂

δε ε

∂∂ ∂ ∂∂ ∂ ∂ ∂ ∂ ∂ ∂

⎛⎞⎡

⎛⎞

=−= −

⎜⎟

⎢

⎝⎠

⎝⎠⎣

jj j

kkkk k k

ff ff

qpp ppq p p q

∂∂ ∂∂

∂∂ ∂

∂∂∂ ∂ ∂∂ ∂ ∂ ∂

⎛⎞⎤

⎛⎞

−− =− +

⎜⎟

⎥

⎝⎠

⎝⎠⎦



()

jj j jj

ff f ff

pq f

pp pq p t tp tp

∂∂ ∂∂

∂∂

εεε

∂∂ ∂∂ ∂ ∂ ∂ ∂

⎛⎞⎡⎛⎞⎛⎞⎤

++=−−+−

⎜ ⎟ ⎜⎟ ⎜⎟

⎢

⎥

⎝⎠⎣⎝⎠⎝⎠⎦





dd d

ff f

pt t p t p

∂∂

∂

εε ε

∂∂∂

⎛⎞ ⎛ ⎞

=− + =

⎜⎟ ⎜ ⎟

⎝⎠ ⎝ ⎠

, 1,2,...,

s= ,

where we have supposed that

f is a function of class

C and have taken into

consideration that

d/d 0ft= ; analogically,

ttq

∂

δε

∂

⎛⎞

=−

⎜⎟

⎝⎠

, 1,2,...,js= ,

the above affirmation being thus proved. Hence, the infinitesimal transformation

(20.2.49') transforms each trajectory in a neighbouring one and the whole family of

paths in itself.

20.2.2 Structure of Canonical Transformations. Properties

In what follows, we will consider some structure properties of the canonical

transformations; we mention especially the group properties and the rôle played by the

Symplectic group. The invariance of Lagrange and Poisson brackets and of the volume

element to such transformations, as well as the important rôle played by the involution

functions are put into evidence.

20.2.2.1 Structure of a Canonical Transformation. The Generating Function

In Sects. 20.2.1.5 and 20.2.1.6 we have shown how one can obtain canonical

(eventually, complete canonical) transformations, starting from the generating functions

,,SS S or

S . These generating functions characterize the structure of the respective

canonical transformations; for instance, if there exists a generating function

S , then we

have to do with a free transformation.

In general, let be

4s quantities ,,,

qpQP, , 1,2,...,

ks= , linked by the

canonical transformations (20.2.1), for which the condition (20.2.1') is verified; one can

choose

independent quantities in the form

MECHANICAL SYSTEMS, CLASSICAL MODELS

290

12 1 2 1 2 1 2

, ,..., , , ,..., , , ,..., , , ,...,

rsm s

rr mm

qq q p p pQQ Q P P P

++ + +

0,rm s≤≤

(20.2.50)

Indeed, we may state

Theorem 20.2.2 (Carathéodory’s lemma). Let be 2s dependent functions

12 12

, ,..., , , ,...,

QQ QPP P, which depend on the independent quantities

, ,...,

qq q,

, ,...,

pp p; from the 4s quantities ,,,QPqp one may always choose 2s

independent quantities so that between them do not be any pair of conjugate quantities

qp or ,

QP, , 1,2,...,

ks= .

We must mention that the proof of this lemma has been given by Carathéodory for

the particular case in which the relations connecting the dependent variables to the

independent ones correspond to a canonical transformation; in fact, the lemma has a

general character.

If we conveniently index again the generalized co-ordinates

and Q , and the

generalized momenta

p and P , then the 2s independent quantities can be represented

in the form (20.2.50). We use the relations

111

dd d

sss

kk kk kk

kr kr kr

pq qp qp

=+ =+ =+

=−

∑∑∑

111

dd d

sss

jjjjj

jm jm jm

PQ QP QP

=+ =+ =+

=−

∑∑∑

;

in this case, the necessary and sufficient condition of canonicity (20.2.18') is written in

the form

()

11 1 1

dd d d dd

rs m s

jj jj

kk kk

kkr j jm

cpq qp PQ QP cHHtU

==+ = =+

⎛⎞

−−− −−=

⎜⎟

⎝⎠

∑∑ ∑ ∑

(20.2.51)

where

kr jm

UWc qp QP

=+ = +

=− +

∑∑

;

(20.2.51')

we may assume that the function

U depends on the variables (20.2.50) and on time. In

this case,

∂

= ,

∂

=− ,

1,2,...,kr=

1, 2,...,lr r s=+ +

(20.2.52)

∂

=− ,

∂

, 1,2,...,im= , 1, 2,...,jm m s=+ + ,

(20.2.52')