Stone M., Goldbart P. Mathematics for Physics: A Guided Tour for Graduate Students

Подождите немного. Документ загружается.

13.3 Homology 465

Exercise 13.4: Let α : A → B be a linear map. Show that

{0}→Ker α

→ A

→ B

→ Coker α →{0}

is an exact sequence. (Recall that Coker α ≡ B/Im α.)

13.3.2 Relative homology

Mathematicians have invented powerful tools for computing homology. In this section

we introduce one of them: the exact sequence of a pair. We describe this tool in detail

because a homotopy analogue of this exact sequence is used in physics to classify defects

such as dislocations, vortices and monopoles. Homotopy theory is, however, harder, and

requires more technical apparatus than homology, so the ideas are easier to explain here.

We have seen that it is useful to think of complicated manifolds as being assembled

out of simpler ones. We constructed the torus, for example, by gluing together edges of

a rectangle. Another construction technique involves shrinking parts of a manifold to a

point. Think, for example, of the unit 2-disc as being a circle of cloth with a drawstring

sewn into its boundary. Now pull the string tight to form a spherical bag. The continuous

functions on the resulting 2-sphere are those continuous functions on the disc that took

the same value at all points on its boundary. Recall that we used this idea in Section

12.4.2, where we claimed that those spin textures in R

that point in a fixed direction at

infinity can be thought of as spin textures on the 2-sphere. We now extend this shrinking

trick to homology.

Suppose that we have a chain complex consisting of spaces C

and boundary opera-

tions ∂

. We denote this chain complex by (C, ∂). Another set of spaces and boundary

operations (C



, ∂



) is a subcomplex of (C, ∂) if each C



⊆ C

and ∂



c ∈ C



. This situation arises if we have a simplicial complex S and some subset S



that

is itself a simplicial complex, and take C



= C



Since each C



is a subspace of C

we can form the quotient spaces C



and make

them into a chain complex by defining, for c + C



∈ C



∂

(c + C



) = ∂

c + C



p−1

. (13.41)

It easy to see that this operation is well defined (i.e. it gives the same output independent

of the choice of representative in the equivalence class c +C



), that ∂

: C

→ C

p−1

is a

linear map, and that

∂

p−1

∂

= 0. We have constructed a new chain complex (C/C



, ∂).

We can therefore form its homology spaces in the usual way. The resulting vector space,

or abelian group, H

(C/C



) is the p-th relative homology group of C modulo C



. When



and C arise from simplicial complexes S



⊆ S, these spaces are what remains of

the homology of S after every chain in S



has been shrunk to a point. In this case, it is

customary to write H

(S, S



) instead of H

(C/C



), and similarly write the chain, cycle

and boundary spaces as C

(S, S



), Z

(S, S



) and B

(S, S



) respectively.

466 13 An introduction to differential topology

Example: Constructing the 2-sphere S

from the 2-ball (or disc) B

. We regard B

be the triangular simplex P

, and its boundary, the 1-sphere or circle S

,tobethe

simplicial complex containing the points P

, P

and the sides P

, P

, but

not the interior of the triangle. We wish to contract this boundary complex to a point, and

form the relative chain complexes and their homology spaces. Of the spaces we quotient

by, C

) is spanned by the points P

, P

, the 1-chain space C

) is spanned by

the sides P

, P

, while C

) ={0}. The space of relative chains C

, S

)

consists of multiples of P

+ C

), and the boundary

∂



+ C

)

= (P

+ P

) + C

) (13.42)

is equivalent to zero because P

+ P

∈ C

). Thus P

+ C

)

is a non-bounding cycle and spans H

, S

), which is therefore one-dimensional.

This space is isomorphic to the one-dimensional H

). Similarly H

, S

) is zero

dimensional, and so isomorphic to H

). This is because all chains in C

, S

) are

in C

) and therefore equivalent to zero.

A peculiarity, however, is that H

, S

) is not isomorphic to H

) = R. Instead,

we find that H

, S

) ={0} because all the points are equivalent to zero. This vanish-

ing is characteristic of the zeroth relative homology space H

(S, S



) for the simplicial

triangulation of any connected manifold. It occurs because S being connected means

that any point P in S can be reached by walking along edges from any other point, in

particular from a point P



in S



. This makes P homologous to P



, and so equivalent to

zero in H

(S, S



Exact homology sequence of a pair

Homological algebra is full of miracles. Here we describe one of them. From the ingre-

dients we have at hand, we can construct a semi-infinite sequence of spaces and linear

maps between them

···

∂

∗p+1

−→ H



)

∗p

−→ H

(S)

∗p

−→ H

(S, S



)

∂

∗p

−→

p−1



)

∗p−1

−→ H

p−1

(S)

∗p−1

−→ H

p−1

(S, S



)

∂

∗p−1

−→

∂

∗1

−→ H



)

∗0

−→ H

(S)

∗0

−→ H

(S, S



)

∂

∗0

−→ {0}. (13.43)

The maps i

∗p

and π

∗p

are induced by the natural injection i

: C



) → C

(S) and

projection π

: C

(S) → C

(S)/C



). It is only necessary to check that

p−1

∂

= ∂

p−1

∂

= ∂

, (13.44)

13.3 Homology 467

to see that they are compatible with the passage from the chain spaces to the homology

spaces. More discussion is required of the connection map ∂

∗p

that takes us from one

row to the next in the displayed form of (13.43).

The connection map is constructed as follows: let h ∈ H

(S, S



). Then h = z +

(S, S



) for some cycle z ∈ Z(S, S



), and in turn z = c + C



) for some c ∈ C

(S).

(So two choices of representative of equivalence class are being made here.) Now

∂

z = 0

which means that ∂

c ∈ C

p−1



). This fact, when combined with ∂

p−1

∂

= 0, tells us

that ∂

c ∈ Z

p−1



). We now define the ∂

∗p

image of h to be

∂

∗p

(h) = ∂

c + B

p−1



). (13.45)

This sounds rather involved, but let’s say it again in words: an element of H

(S, S



) is a

relative p-cycle modulo S



. This means that its boundary is not necessarily zero, but may

be a non-zero element of C

p−1



). Since this element is the boundary of something its

own boundary vanishes, so it is a (p − 1)-cycle in C

p−1



) and hence a representative

of a homology class in H

p−1



). This homology class is the output of the ∂

∗p

map.

The miracle is that the sequence of maps (13.43)isexact. It is an example of a standard

homological algebra construction of a long exact sequence out of a family of short exact

sequences, in this case out of the sequences

{0}→C



) → C

(S) → C

(S, S



) →{0}. (13.46)

Proving that the long sequence is exact is straightforward. All one must do is check each

map to see that it has the properties required. This exercise in what is called diagram

chasing is left to the reader.

The long exact sequence that we have constructed is called the exact homology

sequence of a pair. If we know that certain homology spaces are zero dimensional, it

provides a powerful tool for computing other spaces in the sequence. As an illustration,

consider the sequence of the pair B

n+1

and S

for n > 0:

···

∗p

−→ H

n+1

)

BC D

={0}

∗p

−→H

n+1

, S

)

∂

∗p

−→ H

p−1

)

∗p−1

−→ H

p−1

n+1

)

BC D

={0}

∗p−1

−→ H

p−1

n+1

, S

)

∂

∗p−1

−→ H

p−2

)

∗1

−→ H

n+1

)

BC D

={0}

∗1

−→H

n+1

, S

)

∂

∗1

−→ H

)

BC D

= R

∗0

−→ H

n+1

)

BC D

= R

∗0

−→H

n+1

, S

)

∂

∗0

−→ {0}. (13.47)

468 13 An introduction to differential topology

We have inserted here the easily established data that H

n+1

) ={0} for p > 0 (which

is a consequence of the (n + 1)-ball being a contractible space), and that H

n+1

) and

) are one-dimensional because they consist of a single connected component. We

read off, from the {0}→A → B →{0} exact subsequences, the isomorphisms

n+1

, S

)

∼

p−1

), p > 1, (13.48)

and from the exact sequence

{0}→H

n+1

, S

) → R → R → H

n+1

, S

) →{0} (13.49)

that H

n+1

, S

) ={0}=H

n+1

, S

). The first of these equalities holds because

n+1

, S

) is the kernel of the isomorphism R → R, and the second because

n+1

, S

) is the range of a surjective null map.

In the case n = 0, we have to modify our last conclusion because H

) = R ⊕R is

two-dimensional. (Remember that H

(M ) counts the number of disconnected compo-

nents of M, and the 0-sphere S

consists of the two disconnected points P

, P

lying in

the boundary of the interval B

= P

.) As a consequence, the last five maps in (13.47)

become

{0}→H

, S

) → R ⊕ R → R → H

, S

) →{0}. (13.50)

This tells us that H

, S

) = R and H

, S

) ={0}.

Exact homotopy sequence of a pair

The construction of a long exact sequence from a short exact sequence is a very powerful

technique. It has become almost ubiquitous in advanced mathematics. Here we briefly

describe an application to homotopy theory.

We have met the homotopy groups π

(M ) in Section 12.4.4. As we saw there, homo-

topy groups can be used to classify defects or textures in physical systems in which some

field takes values in a manifold M . Suppose that the local physical properties of a system

are invariant under the action of a Lie group G – for example the high-temperature phase

of a ferromagnet may be invariant under the rotation group SO(3). Now suppose that

system undergoes spontaneous symmetry breaking and becomes invariant only under a

subgroup H. Then manifold of inequivalent states is the coset G/H . For a ferromagnet

the symmetry breaking will be from G = SO(3) to H = SO(2) where SO(2) is the group

of rotations about the axis of magnetization. G/H is then the 2-sphere of the direction

in which the magnetization can point.

The group π

(G) can be taken to be the set of continuous maps of an n-dimensional

cube into the group G, with the surface of the cube mapping to the identity element

13.4 De Rham’s theorem 469

e ∈ G. We similarly define the relative homotopy group π

(G, H ) of G modulo H to be

the set of continuous maps of the cube into G , with all but one face of the cube mapping

to e, but with the remaining face mapping to the subgroup H . It can then be shown that

(G/H )

∼

(G, H ) (the hard part is to show that any continuous map into G/H can

be represented as the projection of some continuous map into G).

The short exact sequence

{e}→H

→ G

→ G/H →{e} (13.51)

of group homomorphisms (where {e}is the group consisting only of the identity element)

then gives rise to the long exact sequence

···→π

(H ) → π

(G) → π

(G, H ) → π

n−1

(H ) →···. (13.52)

The derivation and utility of this exact sequence is very well described in the review

article by Mermin cited in Section 12.4.4. We have therefore contented ourselves with

simply displaying the result so that the reader can see the similarity between the homology

theorem and its homotopy-theory analogue.

13.4 De Rham’s theorem

We still have not related homology to cohomology. The link is provided by integration.

The integral provides a natural pairing of a p-chain c and a p-form ω:ifc = a

+···+a

, where the s

are simplices, we set

(c, ω) =





ω. (13.53)

The perhaps mysterious notion of “adding” geometric simplices is thus given a concrete

interpretation in terms of adding real numbers.

Stokes’ theorem now reads

(∂c, ω) = (c, dω), (13.54)

suggesting that d and ∂ should be regarded as adjoints of each other. From this observation

follows the key fact that the pairing between chains and forms descends to a pairing

between homology classes and cohomology classes. In other words,

(z + ∂c, ω +dχ) = (z, ω), (13.55)

so it does not matter which representatives of the two equivalence classes we take when

we compute the integral. Let us see why this is so.

470 13 An introduction to differential topology

Suppose z ∈ Z

and ω

= ω

+ dη. Then

(z, ω

) =



dη



∂z



= (z, ω

) (13.56)

because ∂z = 0. Thus, all elements of the cohomology class of ω return the same answer

when integrated over a cycle.

Similarly, if ω ∈ Z

and c

= c

+ ∂a then

, ω) =



ω +



∂a



ω +



dω



= (c

, ω),

since dω = 0.

All this means that we can consider the equivalence classes of closed forms composing

(M ) to be elements of (H

(M ))

∗

, the dual space of H

(M ) – hence the “co” in

cohomology. The existence of the pairing does not automatically mean that H

is the

dual space to H

(M ), however, because there might be elements of the dual space that

are not in H

, and there might be distinct elements of H

that give identical answers

when integrated over any cycle, and so correspond to the same element in (H

(M ))

∗

This does not happen, however, when the manifold is compact: De Rham showed that,

for compact manifolds, (H

(M , R))

∗

= H

(M , R). We will not try to prove this, but

be satisfied with some examples.

The statement (H

(M ))

∗

= H

(M ) neatly summarizes de Rham’s results, but, in

practice, the more explicit statements given below are more useful.

Theorem: (de Rham) Suppose that M is a compact manifold.

(1) A closed p-form ω is exact if and only if



ω = 0 (13.57)

for all cycles z

∈ Z

. It suffices to check this for one representative of each

homology class.

13.4 De Rham’s theorem 471

(2) If z

∈ Z

,i= 1, ..., dim H

, is a basis for the p-th homology space, and α

a set of

numbers, one for each z

, then there exists a closed p-form ω such that



ω = α

. (13.58)

If ω

constitute a basis of the vector space H

(M ) then the matrix of numbers



= (z

, ω

) =



(13.59)

is called the period matrix, and the 

themselves are the periods.

Example: H

) = R⊕R is two-dimensional. Since a finite-dimensional vector space

and its dual have the same dimension, de Rham tells us that H

) is also two-

dimensional. If we take as coordinates on T

the angles θ and φ, then the basis elements,

or generators, of the cohomology spaces are the forms “dθ” and “dφ”. We have inserted

the quotes to stress that these expressions are not the d of a function. The angles θ and

φ are not functions on the torus, since they are not single-valued. The homology basis

1-cycles can be taken as z

running from θ = 0toθ = 2π along φ = π, and z

running

from φ = 0toφ = 2π along θ = π . Clearly, ω = α

dθ/2π + α

dφ/2π returns



ω = α

and



ω = α

for any α

, α

,so{dθ/2π, dφ/2π} and {z

, z

} are dual

bases.

Example: We have earlier computed the homology groups H

(RP

, R) ={0} and

(RP

, R) ={0}. De Rham therefore tells us that H

(RP

, R) ={0} and

(RP

, R) ={0}. From this we deduce that all closed 1- and 2-forms on the projective

plane RP

are exact.

Example: As an illustration of de Rham part (1), observe that it is easy to show that a

closed 1-form φ can be written as df , provided that



φ = 0 for all cycles. We simply

define f =



φ, and observe that the proviso ensures that f is not multivalued.

Example: A more subtle problem is to show that, given a 2-form ω on S

, with



ω = 0

there is a globally defined χ such that ω = dχ . We begin by covering S

by two open

sets D

and D

−

which have the form of caps such that D

includes all of S

except for

a neighbourhood of the south pole, while D

−

includes all of S

except a neighbourhood

of the north pole, and the intersection, D

∩ D

−

, has the topology of an annulus, or

cingulum, encircling the equator (Figure 13.10).

Since both D

and D

−

are contractible, there are one-forms χ

and χ

−

such that

ω = dχ

in D

and ω = dχ

−

in D

−

. Thus,

d(χ

− χ

−

) = 0, in D

∩ D

−

. (13.60)

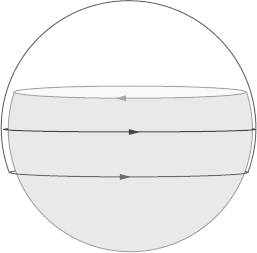
472 13 An introduction to differential topology







Figure 13.10 A covering of the 2-sphere by a pair of contractable caps.

Dividing the sphere into two disjoint sets with a common (but opposingly oriented)

boundary  ∈ D

∩ D

−

, we have

0 =



ω =



(χ

− χ

−

), (13.61)

and this is true for any such curve . Thus, by the previous example,

φ ≡ (χ

− χ

−

) = df (13.62)

for some smooth function f defined in D

∩D

−

. We now introduce a partition of unity

subordinate to the cover of S

by D

and D

−

. This partition is a pair of non-negative

smooth functions, ρ

, such that ρ

is non-zero only in D

, ρ

−

is non-zero only in D

−

and ρ

+ ρ

−

= 1. Now

f = ρ

f − (−ρ

−

)f , (13.63)

and f

−

= ρ

f is a function defined everywhere on D

−

. Similarly f

= (−ρ

−

)f is a

function on D

. Notice the interchange of ± labels! This is not a mistake. The function

f is not defined outside D

∩ D

−

, but we can define ρ

−

f everywhere on D

because

f gets multiplied by zero wherever we have no specific value to assign to it. We now

observe that

+ df

= χ

−

+ df

−

,inD

∩ D

−

. (13.64)

Thus ω = dχ, where χ is defined everywhere by the rule

χ =



+ df

,inD

−

+ df

−

,inD

−

(13.65)

It does not matter which definition we take in the cingular region D

∩D

−

, because the

two definitions coincide there.

The methods of this example can be extended to give a proof of de Rham’s claims.

13.5 Poincaré duality 473

13.5 Poincaré duality

De Rham’s theorem does not require that our manifold M be orientable. Our next results

do, however, require orientability. We therefore assume throughout this section that M is

a compact, orientable, D-dimensional manifold. We will also require that M is a closed

manifold – meaning that it has no boundary.

We begin with the observation that if the forms ω

and ω

are closed then so is ω

∧ω

Furthermore, if one or both of ω

, ω

is exact then the product ω

∧ ω

is also exact. It

follows that the cohomology class [ω

∧ω

]of ω

∧ω

depends only on the cohomology

classes [ω

] and [ω

]. The wedge product thus induces a map

(M , R) × H

(M , R)

∧

→ H

p+q

(M , R), (13.66)

which is called the “cup product” of the cohomology classes. It is written as

[ω

∧ ω

]=[ω

]∪[ω

], (13.67)

and gives the cohomology the structure of a graded-commutative ring, denoted by

•

(M , R).

More significant for us than the ring structure is that, given ω ∈ H

(M , R), we can

obtain a real number by forming



ω. (This is the point at which we need orientability.

We only know how to integrate over orientable chains, and so cannot even define



when M is not orientable.) We can combine this integral with the cup product to make

any cohomology class [f ]∈H

D−p

(M , R) into an element F of (H

(M , R))

∗

.Wedo

this by setting

F([g]) =



f ∧ g (13.68)

for each [g]∈H

(M , R). Furthermore, it is possible to show that we can get any element

F of (H

(M , R))

∗

in this way, and the corresponding [f ] is unique. But de Rham has

already given us a way of identifying the elements of (H

(M , R))

∗

with the cycles in

(M , R)! There is, therefore, a one-to-one onto map

(M , R) ↔ H

D−p

(M , R). (13.69)

In particular the dimensions of these two spaces must coincide:

(M ) = b

D−p

(M ). (13.70)

This equality of Betti numbers is called Poincaré duality. Poincaré originally conceived

of it geometrically. His idea was to construct from each simplicial triangulation S of

M a new “dual” triangulation S



, where, in two dimensions for example, we place a

new vertex at the centre of each triangle, and join the vertices by lines through each

474 13 An introduction to differential topology

side of the old triangles to make new cells – each new cell containing one of the old

vertices. If we are lucky, this process will have the effect of replacing each p-simplex

by a (D − p)-simplex, and so set up a map between C

(S) and C

D−p



) that turns the

homology “upside down”. The new cells are not always simplices, however, and it is

hard to make this construction systematic. Poincaré’s original recipe was flawed.

Our present approach to Poincaré’s result is asserting that for each basis p-cycle class

] there is a unique (up to cohomology) (D − p)-form ω

D−p

such that



f =



D−p

∧ f . (13.71)

We can construct this ω

D−p

“physically” by taking a representative cycle z

in the homol-

ogy class [z

] and thinking of it as a surface with a conserved unit (d − p)-form current

flowing in its vicinity. An example would be the two-form topological current running

along the one-dimensional world-line of a skyrmion. (See the discussion surrounding

Equation (12.64).) The ω

D−p

form a basis for H

D−p

(M , R). We can therefore expand

f ∼ f

D−p

, and similarly for the closed p-form g, to obtain



g ∧ f = f

I(i, j), (13.72)

where the matrix

I(i, j)

def

= I (z

, z

D−p

) =



D−p

∧ ω

(13.73)

is called the intersection form. From its definition we see that I (i, j) satisfies the symmetry

I(i, j) = (−1)

p(D−p)

I(j, i). (13.74)

Less obvious is that I (i, j) is an integer that reports the number of times (counted with

orientation) that the cycles z

and z

D−p

intersect. This latter fact can be understood from

our construction of the ω

as unit currents localized near the z

D−p

cycles. The integrand

in (13.73) is non-zero only in the neighbourhood of the intersections of z

with z

D−p

and at each intersection constitutes a D-form that integrates up to give ±1.

This claim is illustrated in the left-hand part of Figure 13.11, which shows a region

surrounding the intersection of the α and β 1-cycles on the 2-torus. The coordinate

system has been chosen so that the α cycle runs along the x-axis and the β cycle along

the y-axis. Each cycle is surrounded by the narrow shaded regions −w < y < w and

−w < x < w, respectively. To construct suitable forms ω

and ω

we select a smooth

function f (x) that vanishes for |x|≥w and such that



fdx= 1. In the local chart we

can then set

= f (y) dy,

=−f (x) dx,