Stone M., Goldbart P. Mathematics for Physics: A Guided Tour for Graduate Students

Подождите немного. Документ загружается.

12.4 Applications 435

We have now established that

) = Z. (12.69)

This result, implying that there are many maps from the 3-sphere to the 2-sphere that are

not smoothly deformable to a constant map, was a great surprise when Hopf discovered it.

One of the principal physics consequences of the existence of the Hopf index is that

“quantum lump” quasi-particles such as the skyrmion can be fermions, even though they

are described by commuting (and therefore bosonic) fields.

To understand how this can be, we first explain that the collection of homotopy classes

(M ) is not just a set. It has the additional structure of being a group: we can compose

two homotopy classes to get a third, the composition is associative, and each homotopy

class has an inverse. To define the group composition law, we think of S

as the interior

of an n-dimensional cube with the map f : S

→ M taking a fixed value m

∈ M at all

points on the boundary of the cube. The boundary can then be considered to be a single

point on S

. We then take one of the n dimensions as being “time” and place two cubes

and their maps f

, f

into contact, with f

being “earlier” and f

being “later”. We thus

get a continuous map from a bigger box into M. The homotopy class of this map, after

we relax the condition that the map takes the value m

on the common boundary, defines

the composition [f

]◦[f

] of the two homotopy classes corresponding to f

and f

. The

composition may be shown to be independent of the choice of representative functions

in the two classes. The inverse of a homotopy class [f ] is obtained by reversing the

direction of “time” for each of the maps in the class. This group structure appears to

depend on the fixed point m

. As long as M is arcwise connected, however, the groups

obtained from different m

’s are isomorphic, or equivalent. In the case of π

) = Z

and π

) = Z, the composition law is simply the addition of the integers N ∈ Z that

label the classes. A useful exposition of homotopy theory for physicists is to be found in

a review article by David Mermin.

When we quantize using Feynman’s “sum over histories” path integral, we have the

option of multiplying the contributions of histories f that are not deformable into one

another by distinct phase factors exp{iφ([f ])}. The choice of phases must, however,

be compatible with the composition of histories by concatenating one after the other

– the same operation as composing homotopy classes. This means that the product

exp{iφ([f

]))}exp{iφ([f

])} of the phase factors for two possible histories must be the

phase factor exp{iφ([f

]◦[f

])} assigned to the composition of their homotopy classes.

If our quantum system consists of spins n in two space and one time dimension we can

consistently assign a phase factor exp(iπ N

Hopf

) to a history. The rotation of a single

skyrmion twists the world-line cable through 2π and so makes N

Hopf

= 1. The rotation

therefore causes the wavefunction to change sign. We will show, in the next section,

that a history where two particles change places can be continuously deformed into a

history where they do not interchange, but instead one of them is twisted through 2π. The

N. D. Mermin, Rev. Mod. Phys., 51 (1979) 591.

436 12 Integration on manifolds

wavefunction of a pair of skyrmions therefore changes sign when they are interchanged.

This means that the quantized skyrmion is a fermion.

12.4.6 Twist and writhe

Consider two oriented non-intersecting closed curves γ

and γ

in R

. Imagine that γ

carries a unit current in the direction of its orientation and so gives rise to a magnetic

field. Ampère’s law then tells us that the number of times γ

encircles γ

Lk(γ

, γ

) =

B(r

) · dr

4π

− r

) · (dr

× dr

)

− r

. (12.70)

Here the second expression follows from the first by an application of the Biot–Savart

law to compute the B field due to the current. This expression also shows that Lk(γ

, γ

which is called the Gauss linking number, is symmetric under the interchange γ

↔ γ

of the two curves. It changes sign, however, if one of the curves changes orientation, or

if the pair of curves is reflected in a mirror.

We can relate the Gauss linking number to the Brouwer degree of a map. Introduce

parameters t

, t

with 0 < t

, t

≤ 1 to label points on the two curves. The curves are

closed, so r

(0) = r

(1), and similarly for r

. Let us also define a unit vector

n(t

, t

) =

) − r

)

) − r

. (12.71)

Then

Lk(γ

, γ

) =

4π

) − r

)

) − r



∂r

∂t

∂r

∂t



=−

4π



n ·



∂n

∂t

∂n

∂t



(12.72)

is seen to be (minus) the winding number of the map

n : [0, 1]×[0, 1]→S

(12.73)

of the 2-torus into the sphere. Our previous results on maps into the 2-sphere therefore

confirm our Ampère-law intuition that Lk(γ

, γ

) is an integer. The linking number is

also topological invariant, being unchanged under any deformation of the curves that

does not cause one to pass through the other.

An important application of these ideas occurs in biology, where the curves are the

two complementary strands of a closed loop of DNA. We can think of two such parallel

curves as forming the edges of a ribbon {γ

, γ

} of width . Let us denote by γ the curve

12.4 Applications 437



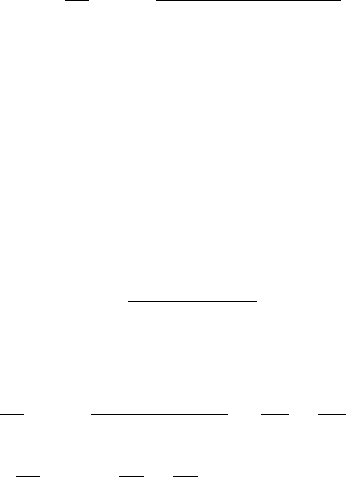
Figure 12.10 An oriented ribbon {γ

, γ

} showing the vectors t and u.

r(t) running along the axis of the ribbon midway between γ

and γ

. The unit tangent

to γ at the point r(t) is

t(t) =

˙r(t)

|˙r(t)|

, (12.74)

where, as usual, the dots denote differentiation with respect to t. We also introduce a

unit vector u(t) that is perpendicular to t(t) and lies in the ribbon, pointing from r

(t)

to r

(t); see Figure 12.10.

We will assign a common value of the parameter t to a point on γ and the points

nearest to r(t) on γ

and γ

. Consequently

(t) = r(t) −

 u(t)

(t) = r(t) +

 u(t). (12.75)

We can express ˙u as

˙u = ω ×u (12.76)

for some angular-velocity vector ω(t). The quantity

Tw =

2π

(ω · t) dt (12.77)

is called the twist of the ribbon. It is not usually an integer, and is a property of the ribbon

{γ

, γ

} itself, being independent of the choice of parametrization t.

If we set r

(t) and r

(t) equal to the single axis curve r(t) in the integrand of (12.70),

the resulting “self-linking” integral, or writhe,

def

4π

(r(t

) − r(t

)) · (˙r(t

) ×˙r(t

))

|r(t

) − r(t

(12.78)

438 12 Integration on manifolds

remains convergent despite the factor of |r(t

) − r(t

in the denominator. However,

if we try to achieve this substitution by making the width of the ribbon  tend to zero,

we find that the vector n(t

, t

) abruptly reverses its direction as t

passes t

. In the limit

of infinitesimal width this violent motion provides a delta-function contribution

−(ω · t)δ(t

− t

) dt

∧ dt

(12.79)

to the 2-sphere area swept out by n, and this contribution is invisible to the writhe

integral. The writhe is a property only of the overall shape of the axis curve γ , and is

independent both of the ribbon that contains it, and of the choice of parametrization.

The linking number, on the other hand, is independent of , so the  → 0 limit of the

linking-number integral is not the integral of the  → 0 limit of its integrand. Instead

we have

Lk(γ

, γ

) =

2π

(ω · t) dt +

4π

(r(t

) − r(t

)) · (˙r(t

) ×˙r(t

))

|r(t

) − r(t

(12.80)

This formula

Lk = Tw + Wr (12.81)

is known as the Calugareanu–White–Fuller relation, and is the basis for the claim, made

in the previous section, that the world-line of an extended particle with an exchange

(Wr =±1) can be deformed into a world-line with a 2π rotation (Tw =±1) without

changing the topologically invariant linking number.

By setting

n(t

, t

) =

r(t

) − r(t

)

|r(t

) − r(t

(12.82)

we can express the writhe as

Wr =−

4π



n ·



∂n

∂t

∂n

∂t



, (12.83)

but we must take care to recognize that this new n(t

, t

) is discontinuous across the line

t = t

= t

. It is equal to t(t) for t

infinitesimally larger than t

, and equal to −t(t)

when t

is infinitesimally smaller than t

. By cutting the square domain of integration

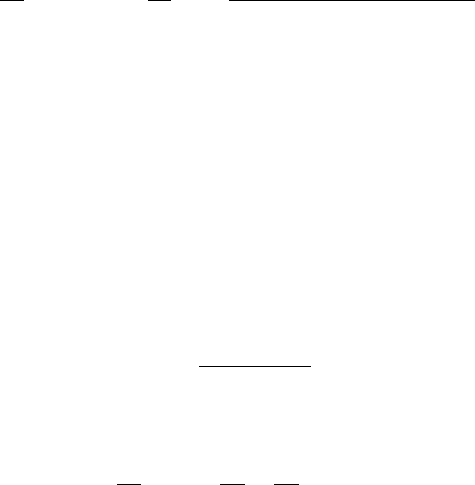
and reassembling it into a rhomboid, as shown in Figure 12.11, we obtain a continuous

integrand and see that the writhe is (minus) the 2-sphere area (counted with multiplicities

and divided by 4π ) of a region whose boundary is composed of two curves , the tangent

indicatrix,ortantrix, on which n = t(t), and its oppositely oriented antipodal counterpart





on which n =−t(t).

12.4 Applications 439

–t



t( t)

–t( t)



Figure 12.11 Cutting and reassembling the domain of integration in (12.83).

The 2-sphere area () bounded by  is only determined by  up to the addition

of integer multiples of 4π. Taking note that the “wrong” orientation of the boundary 

(see Figure 12.11 again) compensates for the minus sign before the integral in (12.83),

we have

4πWr = 2() + 4πn. (12.84)

Thus,

Wr =

2π

(), mod 1. (12.85)

We can do better than (12.85) once we realize that by allowing crossings we can contin-

uously deform any closed curve into a perfect circle. Each self-crossing causes Lk and

Wr (but not Tw which, being a local functional, does not care about crossings) to jump

by ±2. For a perfect circle Wr = 0 whilst  = 2π. We therefore have an improved

estimate of the additive integer that is left undetermined by , and from it we obtain

Wr = 1 +

2π

(), mod 2. (12.86)

This result is due to Brock Fuller.

We can use our ribbon language to describe conformational transitions in long

molecules. The elastic energy of a closed rod (or DNA molecule) can be approximated by

E =





α(ω · t)

βκ



ds. (12.87)

Here we are parametrizing the curve by its arc-length s. The constant α is the torsional

stiffness coefficient, β is the flexural stiffness and

κ(s) =

r(s)

dt(s)

(12.88)

F. Brock Fuller, Proc. Natl. Acad. Sci. USA, 75 (1978) 3557.

440 12 Integration on manifolds

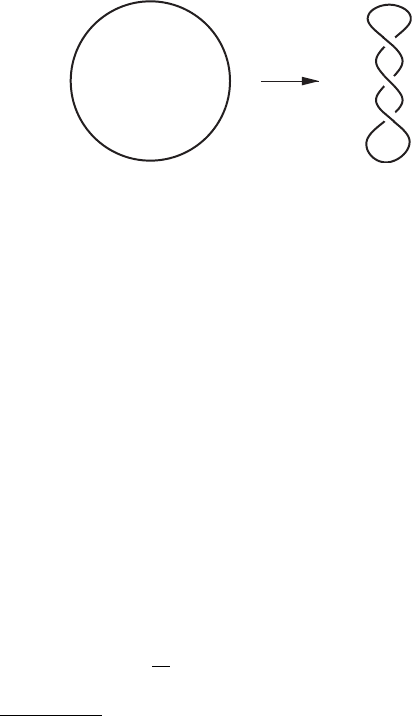
Figure 12.12 A molecule initially with Lk = 3, Tw = 3, Wr = 0 writhes to a new configuration

with Lk = 3, Tw = 0, Wr = 3.

is the local curvature. Suppose that our molecule has linking number n, i.e. it was twisted

n times before the ends were joined together to make a loop.

When β  α the molecule will minimize its bending energy by forming a planar

circle with Wr ≈ 0 and Tw ≈ n. If we increase α, or decrease β, there will come a point

at which the molecule will seek to save torsional energy at the expense of bending, and

will suddenly writhe into a new configuration with Wr ≈ n and Tw ≈ 0 (Figure 12.12).

Such twist-to-writhe transformations will be familiar to anyone who has struggled to

coil a garden hose or electric cable.

12.5 Further exercises and problems

Exercise 12.7: A 2-form is expressed in cartesian coordinates as

ω =

(zdxdy + xdydz +ydzdx)

where r =



+ y

+ z

(a) Evaluate dω for r = 0.

(b) Evaluate the integral

 =



ω,

over the infinite plane P = {−∞ < x < ∞, −∞ < y < ∞, z = 1}.

by the map ϕ, which takes the point (θ , φ) ∈ S

the point (x, y, z) ∈ R

, where

x = R cos φ sin θ,

y = R sin φ sin θ ,

z = R cos θ .

12.5 Further exercises and problems 441

Pull back ω and find the 2-form ϕ

∗

ω on the sphere. (Hint: the form ϕ

∗

ω is both famil-

iar and simple. If you end up with an intractable mess of trigonometric functions,

you have made an algebraic error.)

(d) By exploiting the result of part (c), or otherwise, evaluate the integral

 =



(R)

where S

(R) is the surface of a 2-sphere of radius R centred at the origin.

The following four exercises all explore the same geometric facts relating to Stokes’

theorem and the area 2-form of a sphere, but in different physical settings.

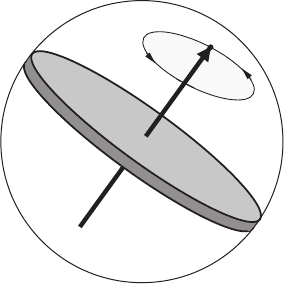
Exercise 12.8: A flywheel of moment of inertia I can rotate without friction about an

axle whose direction is specified by a unit vector n (Figure 12.13). The flywheel and axle

are initially stationary. The direction n of the axle is made to describe a simple closed

curve γ = ∂ on the unit sphere, and is then left stationary.

Show that once the axle has returned to rest in its initial direction, the flywheel has

also returned to rest, but has rotated through an angle θ = Area() when compared

with its initial orientation. The area of  is to be counted as positive if the path γ

surrounds it in a clockwise sense, and negative otherwise. Observe that the path γ

bounds two regions with opposite orientations. Taking into account the fact that we

cannot define the rotation angle at intermediate steps, show that the area of either region

can be used to compute θ, the results being physically indistinguishable. (Hint: show

that the component L

= I(

ψ +

φ cos θ)of the flywheel’s angular momentum along the

axle is a constant of the motion.)

Exercise 12.9: A ball of unit radius rolls without slipping on a table. The ball moves in

such a way that the point in contact with table describes a closed path γ = ∂ on the

ball. (The corresponding path on the table will not necessarily be closed.) Show that the

final orientation of the ball will be such that it has rotated, when compared with its initial





Figure 12.13 Flywheel.

442 12 Integration on manifolds

Figure 12.14 Serret–Frenet frames.

orientation, through an angle φ = Area() about a vertical axis through its centre. As in

the previous problem, the area is counted positive if γ encircles  in an anticlockwise

sense. (Hint: recall the no-slip rolling condition

φ +

ψ cos θ = 0 from (11.29).)

Exercise 12.10: Let a curve in R

be parametrized by its arc-length s as r(s). Then the

unit tangent to the curve is given by

t(s) =˙r

def

The principal normal n(s) and the binormal b(s) to the curve are defined by the require-

ment that

t = κn with the curvature κ(s) positive, and that t , n and b = t × n form a

right-handed orthonormal frame (Figure 12.14).

(a) Show that there exists a scalar τ(s), the torsion of the curve, such that t, n and b

obey the Serret–Frenet relations

⎛

⎝

˙n

⎞

⎠

⎛

⎝

0 κ 0

−κ 0 τ

0 −τ 0

⎞

⎠

⎛

⎝

⎞

⎠

(b) Any pair of mutually orthogonal unit vectors e

(s), e

(s) perpendicular to t and such

that e

× e

= t can serve as an orthonormal frame for vectors in the normal plane.

A basis pair e

, e

with the property

˙e

· e

−˙e

· e

= 0

is said to be parallel,orFermi–Walker, transported along the curve. In other words,

a parallel-transported 3-frame t, e

, e

slides along the curve r(s) in such a way that

the component of its angular velocity in the t direction is always zero. Show that the

Serret–Frenet frame e

= n, e

= b is not parallel transported, but instead rotates

at angular velocity

θ = τ with respect to a parallel-transported frame.

frames are parallel, and so t(s) defines a closed path γ = ∂ on the unit sphere. Fill

12.5 Further exercises and problems 443

in the line-by-line justifications for the following sequence of manipulations:



τ ds =



(b ·˙n − n ·

b) ds



(b · dn − n · db)





(db · dn − dn · db)(∗)





{(db · t)(t · dn) − (dn · t)(t · db)}





{(b · dt)(dt · n) − (n · dt)(dt · b)}

=−





t · (dt × dt)

=−Area().

(The line marked ‘(∗)’ is the one that requires most thought. How can we define “b”

and “n” in the interior of ?)

(d) Conclude that a Fermi–Walker transported frame will have rotated through an angle

θ = Area(), compared to its initial orientation, by the time it reaches the end of

the curve.

The plane of transversely polarized light propagating in a monomode optical fibre is

Fermi–Walker transported, and this rotation can be studied experimentally.

Exercise 12.11: Foucault’s pendulum (in disguise). A particle of mass m is constrained

by a pair of frictionless plates to move in a plane  that passes through the origin O.

The particle is attracted to O by a force −κr, and it therefore executes harmonic motion

within . The orientation of the plane, specified by a normal vector n, can be altered in

such a way that  continues to pass through the centre of attraction O.

(a) Show that the constrained motion is described by the equation

m¨r + κr = λ(t)n,

and determine λ(t) in terms of m, n and ¨r.

(b) Initially the particle motion is given by

r(t) = A cos(ωt + φ).

A. Tomita, R. Y. Chao, Phys. Rev. Lett., 57 (1986) 937.

444 12 Integration on manifolds

Now assume that n changes direction slowly compared to the frequency ω =

√

κ/m.

Seek a solution in the form

r(t) = A(t) cos(ωt + φ),

and show that

A =−n( ˙n ·A). Deduce that |A| remains constant, and so

A = ω ×A

for some angular velocity vector ω. Show that ω is perpendicular to n.

allel transported”, in the sense of the previous problem. Conclude that if n slowly

describes a closed loop γ = ∂ on the unit sphere, then the direction of oscillation

A ends up rotated through an angle θ = Area().

The next exercise introduces a clever trick for solving some of the nonlinear partial

differential equations of field theory. The class of equations to which it and its general-

izations are applicable is rather restricted, but when they work they provide a complete

multi-soliton solution.

Problem 12.12: In this problem you will find the spin field n(x) that minimizes the

energy functional

E[n]=





|∇n

+|∇n

for a given positive winding number N .

(a) Use the results of Exercise 12.6 to write the winding number N , defined in (12.35),

and the energy functional E[n] as

4πN =



(1 + ξ

+ η

)

(

∂

ξ∂

η − ∂

η∂

)

E[n]=



(1 + ξ

+ η

)



(∂

ξ)

+ (∂

ξ)

+ (∂

η)

+ (∂

η)

where ξ and η are stereographic coordinates on S

specifying the direction of the

unit vector n.

(b) Deduce the inequality

E − 4π N

def



(1 + ξ

+ η

)

|(∂

+ i∂

)(ξ + iη)|

≥ 0.

E = 4π N and are obtained by solving the first-order linear partial differential

equation



∂

∂x

+ i

∂

∂x



(ξ + iη) = 0.