Stewart J. College Algebra: Concepts and Contexts
Подождите немного. Документ загружается.


364 CHAPTER 4
■
Logarithmic Functions and Exponential Models
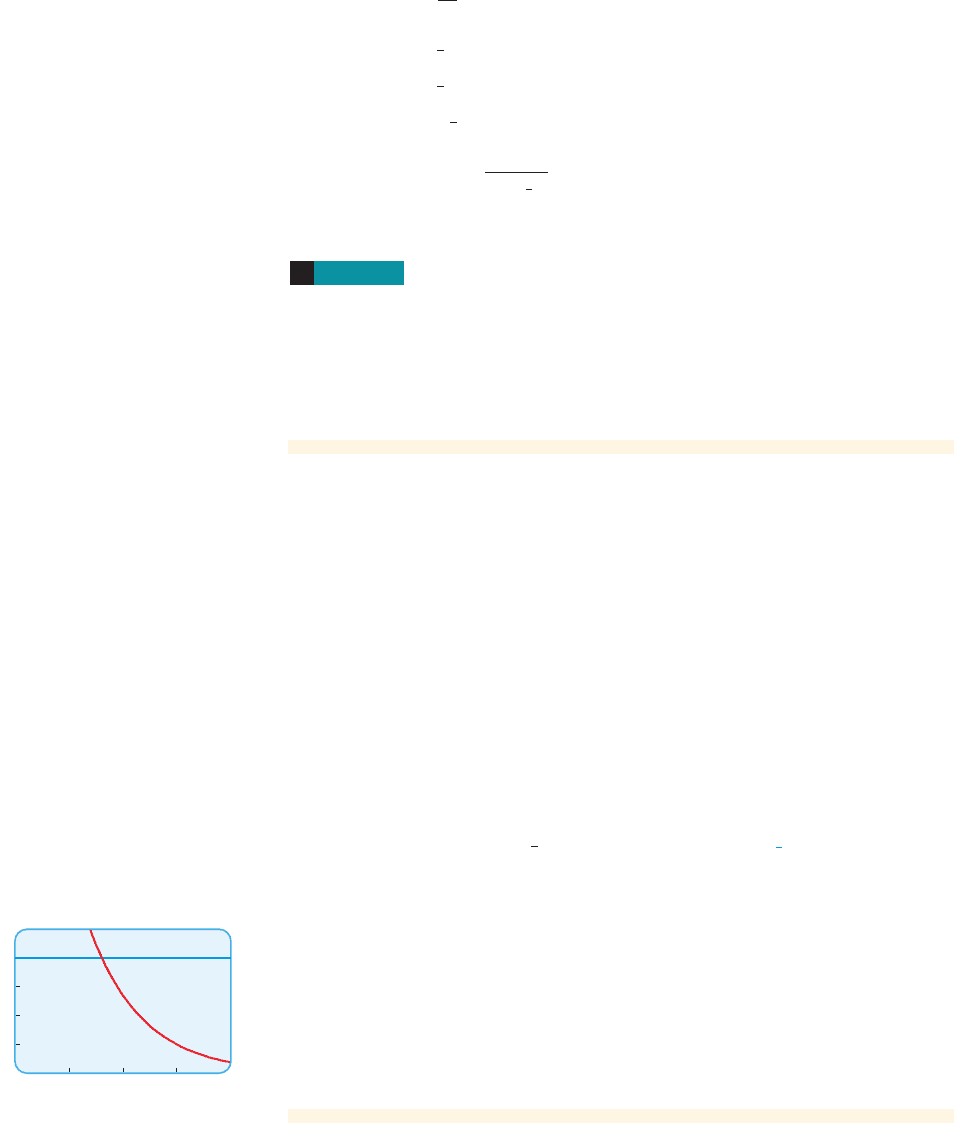
(c) What is the “murkiness” constant k for this lake?
(d) Use the model to estimate the light intensity at the surface.
(e) Make a scatter plot of the data, and graph the function that you found in part (a) on
your scatter plot.
I
0
Depth (ft) Light intensity (lm)
5 13.0
10 7.6
15 4.5
20 2.7
Depth (ft) Light intensity (lm)
25 1.8
30 1.1
35 0.5
40 0.3
4.5 Exponential Equations: Getting Information
from a Model
CSI Miami
■
Solving Exponential and Logarithmic Equations
■
Getting Information from Exponential Models:
Population and Investment
■
Getting Information from Exponential Models:
Newton’s Law of Cooling
■
Finding the Age of Ancient Objects: Radiocarbon Dating
IN THIS SECTION… we learn how to get information from an exponential model about
the situation being modeled. Getting this information requires us to solve exponential
equations.
The applications of algebra range over every field of human endeavor in which quan-
titative problems arise. In this section we see how algebra is used in crime scene in-
vestigations. One of the key pieces of evidence in any homicide case is the time of
death; this is often determined by comparing the temperature of the victim’s body
with normal body temperature. As a warm object cools, its temperature decreases
with time according to an exponential model. Using this model, we can find the tem-
perature of a cooling object if we know the initial temperature and the length of time
it has been cooling. When a homicide victim is discovered, we know the initial tem-
perature of the body (normal body temperature) and can measure the current body
temperature; we need to find the time when the body started to cool (the time of
death). So here is the situation:
■
The model gives us the temperature of a body if we know how long the
body has been cooling.
■
We want to find out how long the body has been cooling if we know its
current temperature.
We can get the information we want from the model; to do so, we need to solve an
equation involving exponential functions. So we start this section by learning how to
solve such equations.
Robert Voets/CBS Photo Archive via Getty Images

SECTION 4.5
■
Exponential Equations: Getting Information from a Model 365
2
■ Solving Exponential and Logarithmic Equations
An exponential equation is one in which the variable occurs in the exponent. For
example,
is an exponential equation. To solve this equation, we take the logarithm of each side
and then use the Laws of Logarithms to “bring down x” from the exponent as follows.
Given equation
Take the log of each side
Law 3: “Bring down the exponent”
Divide by
Calculator
The method we used to solve this equation is typical of how we solve exponential
equations in general.
x L 3.17
log 2 x =
log 9
log 2
x log 2 = log 9
log 2
x
= log 9
2
x
= 9
2
x
= 9
example
1
Solving Exponential Equations
Solve the exponential equation for the unknown x.
Solution
We first put the exponential term alone on one side of the equation.
Given equation
Divide by 5
Take the log of each side
Law 3: “Bring down the exponent”
Divide by
Calculator
Substituting into the original equation and using a calcu-
lator, we get .
■ NOW TRY EXERCISE 7 ■
5
#
2
2.848
L 36
x = 2.848
✓ CHECK
x L 2.848
log 2 x =
log 7.2
log 2
x log 2 = log 7.2
log 2
x
= log 7.2
2
x
= 7.2
5
#
2
x
= 36
5
#
2
x
= 36
example
2
Solving Exponential Equations
Solve the exponential equation for the unknown x.
Solution
To put the exponential term alone on one side of the equation, we divide each side
by .3
x
8
x
= 512
#
3
x
366 CHAPTER 4
■
Logarithmic Functions and Exponential Models
example
3
Solving Exponential Equations
Solve the exponential equation for the unknown x.
Solution 1 Algebraic
Since the exponential term is alone on one side, we begin by taking the logarithm of
each side. (We take the natural logarithm because the base in the equation is e.)
Given equation
Take ln of each side
Property of ln
Subtract 3
Multiply by
Calculator
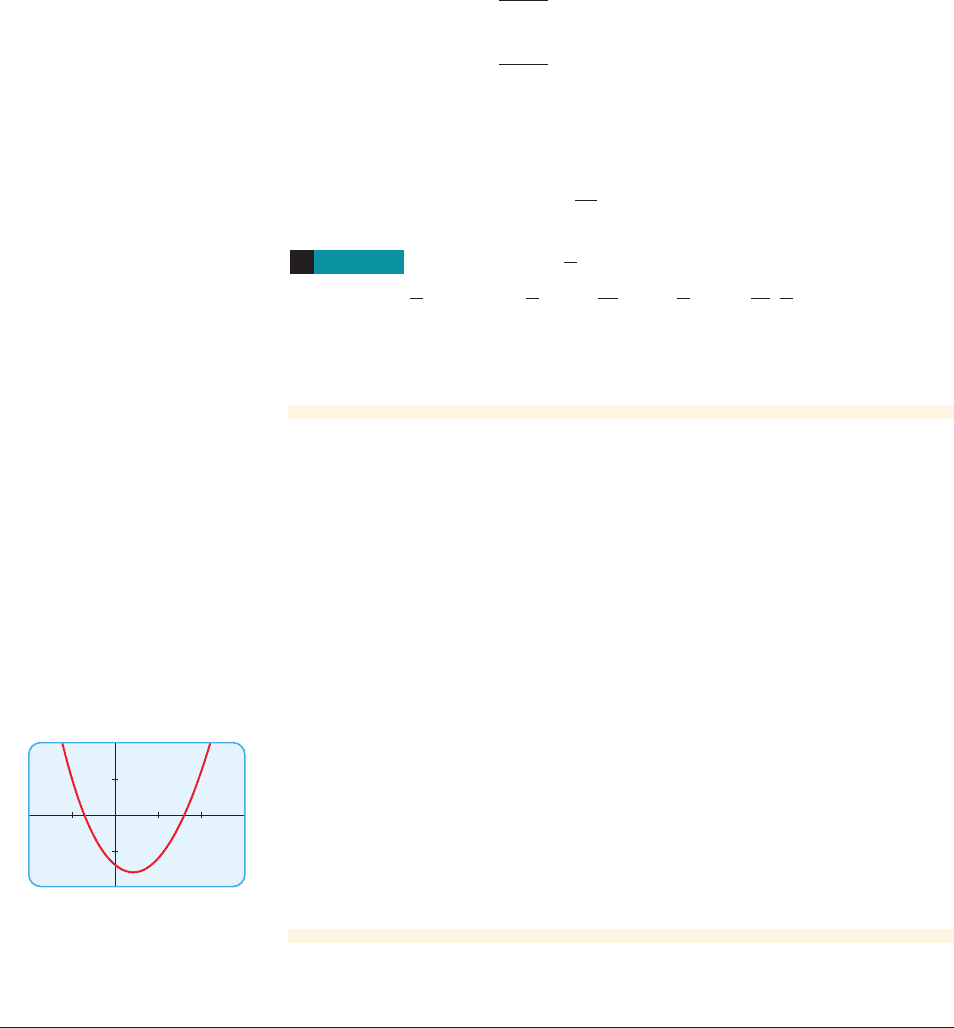
Solution 2 Graphical
We graph the equations
in the same viewing rectangle, as in Figure 1. The solution occurs where the graphs
intersect. Zooming in on the point of intersection of the two graphs, we see that
.
■ NOW TRY EXERCISE 21 ■
x L 0.807
y = e
3 -2x
andy = 4
x L 0.807
-
1
2
x =-
1
2
1- 3 + ln 42
- 2x =-3 + ln 4
3 - 2x = ln 4
ln e
3 -2x
= ln 4
e
3 -2x
= 4
e
3 -2x
= 4
5
0
2
y=4
y=e
3_2x
figure 1
Given equation
Divide each side by
Rules of Exponents
Take the log of each side
Law 3: “Bring down the exponent”
Solve for x
Calculator
We substitute into each side of the original equation and
use a calculator:
✓
■ NOW TRY EXERCISE 15 ■
LHS = RHS
RHS:512
#
3
x
= 512
#
3
6.36
L 554,000
LHS:8
x
= 8
6.36
L 554,000
x = 6.36
✓ CHECK
x L 6.36
x =
log 512
logA
8
3
B
x log
8
3
= log 512
logA
8
3
B
x
= log 512
A
8
3
B
x
= 512
3
x
8
x
3
x
= 512
8
x
= 512
#
3
x
Rules of Exponents are
reviewed in Algebra Toolkits A.3
and A.4, pages T14 and T20.
SECTION 4.5
■
Exponential Equations: Getting Information from a Model 367
example
4
Solving Logarithmic Equations
Solve the following logarithmic equations for the unknown x.
(a)
(b)
(c)
Solution
(a) We first isolate the logarithm term.
Given equation
Divide by 2
Exponential form
Calculator
(b) We first combine the logarithm terms using the Laws of Logarithms.
Given equation
Laws of Logarithms
Exponential form
Divide by 5
Subtract 1
Calculator
We substitute into each side of the original equation:
✓
(c) We first combine the logarithm terms using the Laws of Logarithms.
LHS = RHS
RHS:
1
LHS:ln1- 0.46 + 12+ ln 5 = ln 0.54 + ln 5 = ln10.54
#
52= ln 2.7 L 1
x =-0.46
✓ CHECK
x L-0.46
x =
e
5
- 1
x + 1 =
e
5
5 1x + 12= e
ln 51x + 1 2= 1
ln1x + 12+ ln 5 = 1
x L 31.62
x = 10
3>2
log x =
3
2
2 log x = 3
log1x + 12- log x = 2
ln1x + 12+ ln 5 = 1
2 log x = 3
We can also solve logarithmic equations, that is, equations in which a logarithm
of the unknown occurs. For example, is a logarithmic equation. To
solve for x, we write the equation in exponential form:
Given equation
Exponential form
Solve for x
The first step in solving logarithmic equations is to combine all logarithm terms in
the equation into one term (by using the Laws of Logarithms).
x = 32 - 2 = 30
x + 2 = 2
5
log
2
1x + 2 2= 5
log
2
1x + 2 2= 5
368 CHAPTER 4
■
Logarithmic Functions and Exponential Models
Given equation
Laws of Logarithms
Exponential form
Multiply by x
Subtract x and switch sides
Divide by 99
We substitute into each side of the original equation:
✓
■ NOW TRY EXERCISES 29, 35, AND 37 ■
The equation in the next example cannot be solved algebraically, because it is
impossible to put x alone on one side of the equation, so we can only solve the equa-
tion graphically.
LHS = RHS
RHS:2
LHS:logA
1
99
+ 1B- log
1
99
= log
100
99
- log
1
99
= logA
100
99
>
1
99
B= log 100 = 2
x =
1
99
✓ CHECK
x =
1
99
99x = 1
x + 1 = 100x
x + 1
x
= 10
2
log
x + 1
x
= 2
log1x + 12- log x = 2
example
5
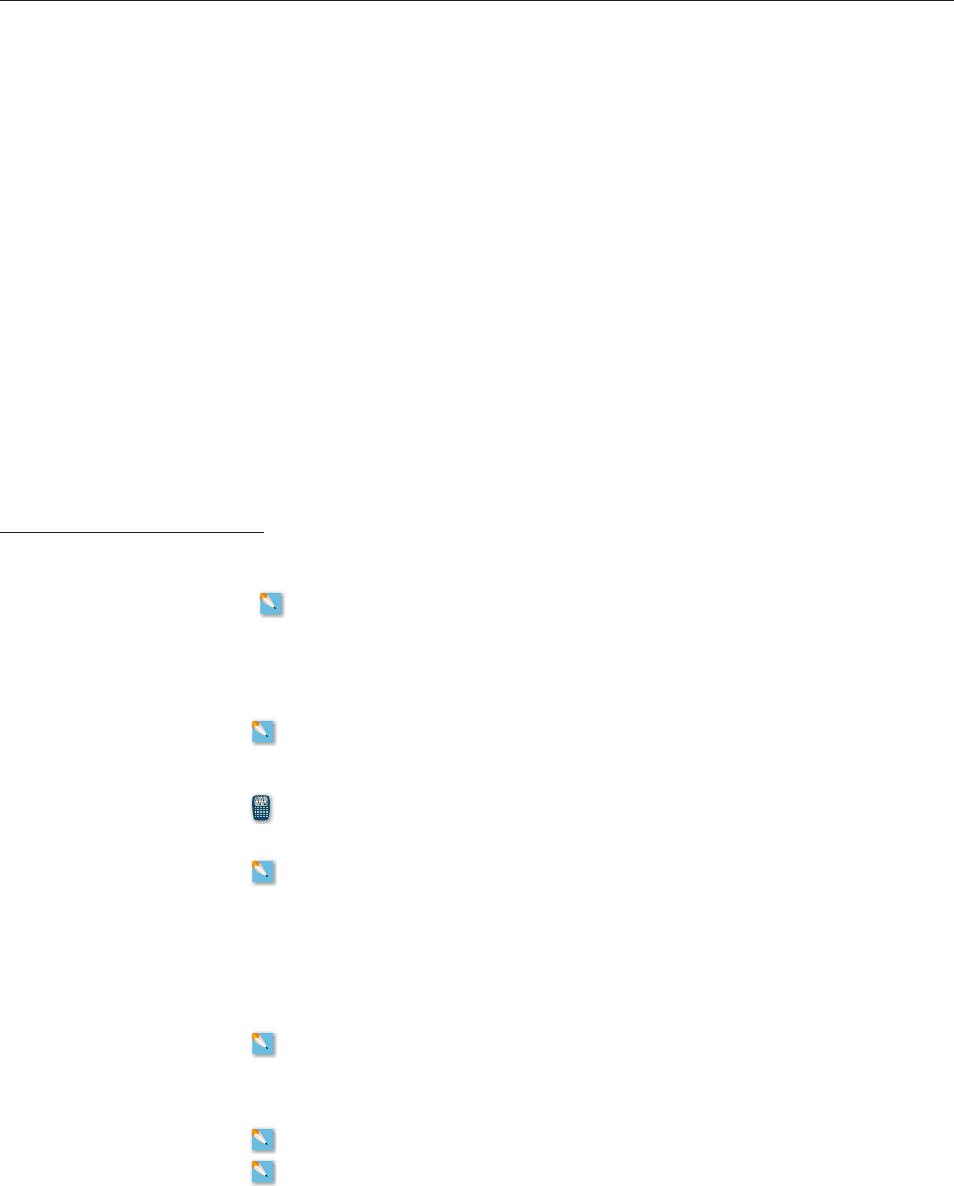
Solving a Logarithmic Equation Graphically
Solve the equation for the unknown x.
Solution
We first move all terms to one side of the equation:
Then we graph
as in Figure 2. The solutions are the x-intercepts of the graph. Zooming in on the
x-intercepts, we see that there are two solutions: and .
■ NOW TRY EXERCISE 41 ■
x L 1.60x L-0.71
y = x
2
- 2 ln1x + 2 2
x
2
- 2 ln1x + 2 2= 0
x
2
= 2 ln1x + 22
2
_2
3
_2
figure 2
2
■ Getting Information from Exponential Models:
Population and Investment
We can use logarithms to determine when quantities that grow or decay exponen-
tially reach a given level. In the next two examples we solve exponential equations
involving interest on an investment and population growth.

SECTION 4.5
■
Exponential Equations: Getting Information from a Model 369
example
6
Investment Growth
Helen invests $10,000 in a high-yield uninsured certificate of deposit that pays 12%
interest annually, compounded every 6 months.
(a) Find a formula for the amount A of the certificate after t years.
(b) What is the amount after 3 years?
(c) How long will it take for her investment to grow to $25,000?
Solution
(a) We use the formula for compound interest (Section 3.1, page 265), where the
principal P is 10,000, the interest rate r is 0.12, and the number of
compounding periods n is 2:
Compound interest formula
Substitute given values
Simplify
(b) Using the formula we found in part (a) and replacing t by 3, we get
Replace t by 3
So in 3 years the certificate is worth $14,185.20.
(c) We want the amount A to reach $25,000, and we need to find t, the time
needed to achieve this amount. We use the formula from part (a):
Formula
Replace by 25,000
Divide by 10,000
Take the log of each side
Law 3: “Bring down the exponent”
Divide by 2 log(1.06)
Calculator
It will take almost 8 years for Helen’s investment to grow to $25,000.
■ NOW TRY EXERCISE 49 ■
t L 7.863
log 2.5
2 log11.06 2
= t
log 2.5 = 2t log11.062
log 2.5 = log11.062
2t
2.5 = 11.062
2t
A1t 2 25,000 = 10,00011.06 2
2t
A1t 2= 10,00011.062
2t
= 14,185.20
A1t 2= 10,000 11.062
2132
A1t 2= 10,000 11.062
2t
A1t 2= 10,000 a1 +
0.12
2
b
2t
A1t 2= P a1 +
r
n
b
nt
example
7
Doubling the Population of the World
On January 1, 2007, the population of the world was approximately 6.6 billion and
was increasing by 1.36% every year. Assume that this rate of increase continues.
(a) Find a function P that models the population after t years.
(b) What does the model predict for the population in 2015?
(c) In what year will the population of the world have doubled?

The rate at which a hot cup of
coffee cools is proportional to the
temperature difference between
the cup and its surroundings.
370 CHAPTER 4
■
Logarithmic Functions and Exponential Models
2
■ Getting Information from Exponential Models: Newton’s Law of Cooling
When a hot object, such as a cup of coffee, is left to cool, its temperature decreases
continuously at a rate proportional to the temperature difference between the object
and its surroundings. Since this difference is continually changing, the rate at which
the object cools is also continually changing. Newton’s Law of Cooling states that
the temperature T of a cooling object is modeled by
where t is the time since the object began cooling, I is the initial temperature of the ob-
ject, A is the ambient temperature (the temperature of the surroundings), and k is a con-
stant that depends on the type of object; k is called the heat transfer coefficient.
Newton’s Law of Cooling is a powerful tool in many crime scene investigations.
For example, in the infamous 1994 O.J. Simpson case two key pieces of evidence—
a cup of ice cream and a bathtub full of warm water—could have provided com-
pelling evidence for the time of death. According to court testimony, “the first offi-
T = A + 1I - A2e
-kt
Solution
(a) We use the exponential growth model (Section 3.1, page 250)
where C is the initial population and a is the growth factor. Since the popula-
tion increases by 1.36% every year, the growth factor is
. So the model we seek is
Exponential growth model
where t is the number of years after 2007.
(b) The year 2015 is eight years after 2007. Replacing t by 8 in the model we get
So the model predicts a population of about 7.35 billion in 2015.
(c) We want to know when the population will double to billion. In
other words, we want to find the value of t for which P(t) is 13.2 billion.
Substituting these values into the formula gives the following:
Exponential growth model
Replace P(t) by 13.2
Divide by 6.6
Take the log of each side
Law 3: “Bring down the exponent”
Divide by log 1.0136
Calculator
So it will take about 51 years for the population to double. This would happen
in the year .
■ NOW TRY EXERCISE 55 ■
2007 + 51 = 2058
t L 51.3
log 2
log 1.0136
= t
log 2 = t log 1.0136
log 2 = log11.01362
t
2 = 11.0136 2
t
13.2 = 6.611.01362
t
P1t 2= 6.611.01362
t
216.6 2= 13.2
P18 2= 6.611.01362
8
L 7.35
P1t 2= 6.611.01362
t
a = 1 + 0.0136 = 1.0136
P1t 2= Ca
t

SECTION 4.5
■
Exponential Equations: Getting Information from a Model 371
example
8
Crime Scene Investigation
Police officers arrive at a crime scene and find a tub full of warm water. A ther-
mometer shows that the water temperature is and the air temperature is .
It is known that most people fill a tub with water at .
(a) Use Newton’s Law of Cooling to model the temperature of the water in the
tub. (Measure t in minutes and use the heat transfer coefficient .)
(b) How long has the bathtub been cooling?
Solution
(a) We use Newton’s Law of Cooling, where the initial temperature I is , the
ambient temperature A is , and the heat transfer constant k is 0.018.
Newton’s Law of Cooling
Replace A by 70, I by 100, and k by 0.018
Simplify
(b) We know that the temperature of the bathtub water is , so we use the
model from part (a), replace T by 76, and solve the resulting exponential
equation for t.
Model
Replace T by 76
Subtract 70
Divide by 30
Take ln of each side
Divide by 0.018 and switch sides
Calculator
So the tub has been cooling for about 89 minutes.
■ NOW TRY EXERCISE 57 ■
t L 89.41
t =
ln 0.20
- 0.018
ln 0.20 =-0.018t
0.20 = e
-0.018t
6 = 30e
-0.018t
76 = 70 + 30e
-0.018t
T = 70 + 30e
-0.018t
76°F
T = 70 + 30e
-0.018t
T = 70 + 1100 - 702e
-0.018t
T = A + 1I - A 2e
-kt
70°F
100°F
k = 0.018
100°F
70°F76°F
cer on the scene did not test the temperature of the water in . . . [the] bathtub or pick
up the cup of melting ice cream.” In the next example we investigate how bathtub
temperature could help to determine the time when the tub was filled.
2
■ Finding the Age of Ancient Objects: Radiocarbon Dating
In the next example we examine how exponential equations are used to determine
the ages of ancient artifacts. Before the twentieth century archeologists had a hard
time determining the ages of the objects they found. It’s difficult to tell whether a
shard of pottery was dropped on the ground 50 years ago or 50 centuries ago. So
archeologists generally used the principle that the deeper they had to dig to find an
object, the older it probably was and that objects found at the same level, or stratum,

372 CHAPTER 4
■
Logarithmic Functions and Exponential Models
IN CONTEXT ➤
The iceman
example
9
Dating the Iceman
Tissue samples from the iceman indicated that his body had 57.67% of the carbon-14
that is present in a living person. Use the fact that the half-life of carbon-14 is 5730
years to estimate how long ago the iceman died.
Solution
The formula for exponential decay is
where C is the initial mass of the radioactive substance, h is its half-life, and m(t) is
the mass remaining at time t (see Example 9 in Section 3.1). We are looking for the
time t at which m(t) is 57.67% of the initial mass of carbon-14 in the iceman’s body,
that is, m(t) is 0.5767C. We now substitute the known values into the formula and
solve for t.
Radioactive decay formula
Substitute given values
Divide by C
Take the log of each side
Law 3: “Bring down the exponent”
Multiply by 5730, divide by
Calculator
So the iceman died about 4550 years ago.
■ NOW TRY EXERCISE 65 ■
t L 4550
logA
1
2
B
5730 log 0.5767
logA
1
2
B
= t
log 0.5767 =
t
5730
logA
1
2
B
log 0.5767 = logA
1
2
B
t>5730
0.5767 = A
1
2
B
t>5730
0.5767C = C˛ A
1
2
B
t>5730
m1t 2= C˛ A
1
2
B
t>h
m1t 2= C˛ A
1
2
B
t>h
were close in age. This was helpful in comparing the relative ages of two items, but
how could they get the actual age? And how could they compare the ages of items
found at sites 50 miles apart? The discovery that radioactive decay is exponential al-
lowed scientists to determine the age of ancient objects by comparing the amount of
radioactive carbon-14 in the object to the amount it normally would have today.
A dramatic use of radiocarbon dating occurred with the discovery of the “iceman.”
On September 19, 1991, Erika and Helmut Simon were hiking in the Alps near the
Austrian-Italian border. As they approached an ice-filled depression, they were sur-
prised to see the frozen body of a man sticking halfway out of the ice. They reported
the find to authorities, thinking that it was a recent tragic accident. But the authorities
were baffled by the find because of the frozen man’s unusual goatskin leather clothing
and the bronze ax and quiver of arrows strapped to his body. Who was this “iceman”?
Where did he come from? To answer these questions, the primary piece of information
needed was the date of the iceman’s demise. The surprising answer, which we calcu-
late in the next example, was that he lived in the Neolithic Age (Late Stone Age).
How does radiocarbon dating work? Plants absorb radioactive carbon-14 from the
atmosphere, which then makes its way into animals through the food chain. Thus, all
living creatures contain the same fixed proportion of carbon-14 to nonradioactive
carbon-12 as is in the atmosphere. After an organism dies, it stops assimilating
carbon-14, and the carbon-14 in it begins to decay exponentially. We can then deter-
mine the time elapsed since death by measuring the amount of carbon-14 left in the relic.
© dpa/Corbis
SECTION 4.5
■
Exponential Equations: Getting Information from a Model 373
4.5 Exercises
CONCEPTS
Fundamentals
1. Let’s solve the exponential equation .
(a) First, we isolate to get the equivalent equation
______________.
(b) Next, we take ln of each side to get the equivalent equation
______________.
(c) Now we use a calculator to find
______________.
2. Let’s solve the logarithmic equation .
(a) First, we combine the logarithms to get the equivalent equation
______________.
(b) Next, we write each side in exponential form to get the equivalent equation
______________.
(c) Now we find x ⫽
_______.
Think About It
3. To solve the exponential equation , we first take logarithms of both sides.
What base logarithm leads to the easiest solution? Why does this base work so nicely?
4. Logarithms with any base work equally well to solve the equation . Why? First
solve the equation using common logarithms, and then solve the equation using natural
logarithms. Now use a logarithm with a different base to solve the equation. (You will need
to use the Change of Base Formula in the solution.) Do you always get the same answer?
5–18
■ Solve the exponential equation for the unknown x.
5. 6.
7. 8.
9. 10.
11. 12.
13. 14.
15. 16.
17. 18.
19–26
■ Solve the exponential equation (a) algebraically and (b) graphically.
19. 20.
21. 22.
23. 24.
25. 26.
27–40
■ Solve the logarithmic equation for the unknown x.
27. 28.
29. 30.
31. 32.
33. 34.
35. 36.
37. 38.
39. 40. log 2x = log 2 + log13x - 42ln 5 + ln1x - 22= ln13x 2
log
5
1x + 12- log
5
1x - 12= 2log
2
x - log
2
1x - 32= 2
log
2
13x - 12+ log
2
8 = 5log12x + 12+ log 2 = 2
4 log
5
13x + 52= 2log1x - 42= 3
ln 3x =-4log
2
8x =-2
2 log
9
x = 13 log x = 12
log x = 7log
2
x = 10
5
x
= 3
#
2
x
5
x
= 212
#
9
x
7
x>2
= 5
1 - x
10
1 - x
= 6
x
2
3x
= 342
1 - x
= 3
3
#
e
x
= 10e
2x
= 5
13.2 2
x
= 35
#
12.6 2
x
5
x
= 4
x + 1
5
x
= 50
#
3
x
6
x
= 21
#
2
x
8
4x - 1
= 52
3x + 1
= 34
e
2x + 1
= 2003
1 - 4x
= 2
9
#
4
3x
= 122e
12x
= 17
6
#
10
x
= 425
#
3
x
= 7
e
x
= 710
x
= 25
3
x
= 4
#
5
x
2
3x
= 2
x + 1
log 3 + log1x - 22= log x
x =
e
x
2e
x
= 50
SKILLS
