Steve M., Darby D.M., Geostatistics Explained - An Introductory Guide for Earth Scientists
Подождите немного. Документ загружается.

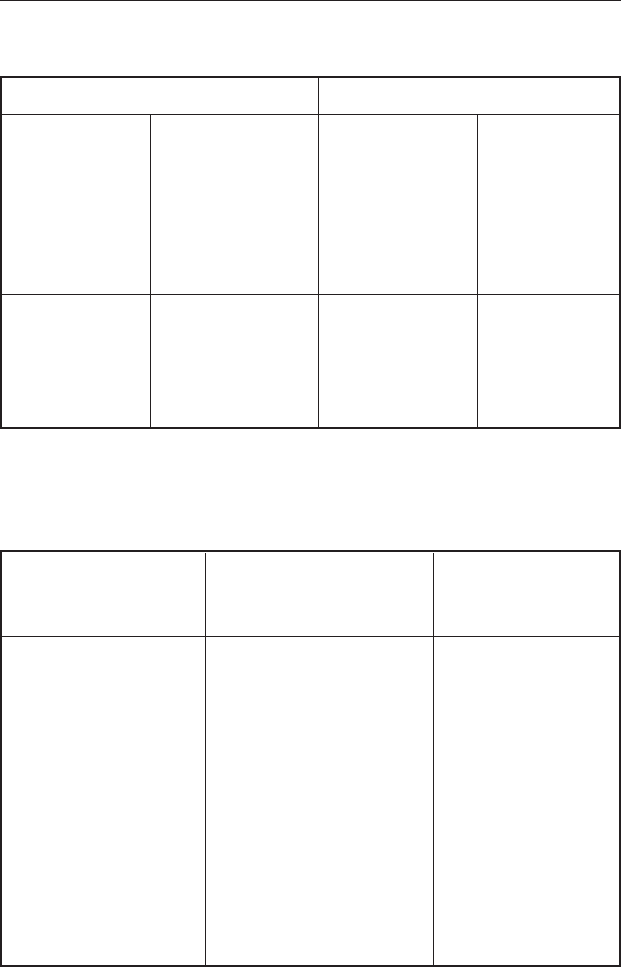
Table 23.11 Methods for analyzing and modeling a temporal sequence.
Ratio, interval or ordinal scale data Nominal scale data
Testing whether
there is
repetition or
relatedness
among different
parts of a
sequence
Modeling a
sequence to explain
within sequence
variability
Testing whether
there is repetition
or relatedness
among different
parts of a
sequence
Testing whether
there is a
pattern in the
repeated
occurrences of
an event
Autocorrelation
(Chapter 21)
Regression
modeling of
sequences
(Chapter 21)
Methods for
analyzing
transitions
(Chapter 21)
Regression
analysis and
survival
functions
(Chapter 21)
Table 23.12 Analyzing data for a pattern related to the spatial location of sampling
units occurring in a two dimensional landscape.
Testing for departures
from a random pattern
in two-dimensional space
Testing hypotheses about
the direction or orientation
of a sample of objects
Interpolation and
prediction in two
dimensions
Assessing whether the
distribution is
consistent with a
random spatial pattern,
using data for counts
within quadrats
(Chapter 22)
Assessing whether the
distribution is
consistent with a
random spatial pattern
using nearest neighbor
analysis (Chapter 22)
Tests for the direction or
orientation of a sample of
objects (Chapter 22)
Calculation of the
semivariance for use
in Kriging (the latter is
only conceptually
introduced in this text)
(Chapter 22)
23.1 Introduction 373
Appendix A: Critical values
of chi-square, t and F
Table A1 Critical values of chi-square when α =0.05,for1–120 degrees of freedom. If
the calculated value of chi-square is larger than the critical value for the appropriate
number of degrees of freedom then the probability of the result is < 0.05 (and is
therefore considered significant with an α of 0.05). For example, for three degrees of
freedom the critical value is 7.815, so a chi-square larger than this indicates P <0.05.
Values were calculated using the method given by Zelen and Severo (1964).
374

Table A1
Degrees of
freedom α = 0.05
Degrees of
freedom α = 0.05
Degrees of
freedom α = 0.05
1 3.841 41 56.942 81 103.010
2 5.991 42 58.124 82 104.139
3 7.815 43 59.304 83 105.267
4 9.488 44 60.481 84 106.395
5 11.070 45 61.656 85 107.522
6 12.592 46 62.830 86 108.648
7 14.067 47 64.001 87 109.773
8 15.507 48 65.171 88 110.898
9 16.919 49 66.339 89 112.022
10 18.307 50 67.505 90 113.145
11 19.675 51 68.669 91 114.268
12 21.026 52 69.832 92 115.390
13 22.362 53 70.993 93 116.511
14 23.685 54 72.153 94 117.632
15 24.996 55 73.311 95 118.752
16 26.296 56 74.468 96 119.871
17 27.587 57 75.624 97 120.990
18 28.869 58 76.778 98 122.108
19 30.114 59 77.931 99 123.225
20 31.401 60 79.082 100 124.342
21 32.671 61 80.232 101 125.458
22 33.924 62 81.381 102 126.574
23 35.172 63 82.529 103 127.689
24 36.415 64 83.675 104 128.804
25 37.652 65 84.821 105 129.918
26 38.885 66 85.965 106 131.031
27 40.113 67 87.108 107 132.144
28 41.337 68 88.250 108 133.257
29 42.557 69 89.391 109 134.369
30 43.773 70 90.531 110 135.480
31 44.985 71 91.670 111 136.591
32 46.914 72 92.808 112 137.701
33 47.400 73 93.945 113 138.811
34 48.602 74 95.081 114 139.921
35 49.802 75 96.217 115 141.030
36 50.998 76 97.351 116 142.138
37 52.192 77 98.484 117 143.246
38 53.384 78 99.617 118 144.354
39 54.572 79 100.749 119 145.461
40 55.758 80 101.879 120 146.567
Appendix A: Critical values of chi-square, t and F 375

Table A2 Critical two- and one-tailed values of Student’s t statistic when α = 0.05,
calculated using the method given by Zelen and Severo (1964). A t test is used for
comparison between two samples or a sample and a population, so both non-
directional and directional alternate hypotheses are possible (e.g. for the latter the
alternate hypothesis might be “The mean of Sample A is expected to be greater than
the population mean μ”).
For non-directional and therefore two-tailed alternate hypotheses, if the calculated
value of t is outside the range of zero ± the critical value then the probability of that
result is < 0.05 (and therefore considered signi ficant with an α of 0.05). For example,
for six degrees of freedom the value of t must be outside the range of zero ± 2.447.
For directional and therefore one-tailed alternate hypotheses you first need to check
whether the difference between two means is in the direction specified by the
alternate hypothesis (e.g. if the hypothesis specifies mean A is greater than mean B,
there is no point in looking up the critical value if mean B is greater than mean A,
because the null hypothesis will stand whatever the value of t).
If the difference is in the direction specified by the alternate hypothesis then the
absolute value of t (that is, the value of t written as a positive number irrespective of
whether the calculated value is positive or negative) is significant for α = 0.05 if it is
larger than the one-tailed critical value for the appropriate number of degrees of
freedom in Table A2. For example, for 20 degrees of freedom the absolute value of t
must exceed 1.725 for P < 0.05.
376 Appendix A: Critical values of chi-square, t and F

Table A2
Degrees of
freedom α (2) = 0.05 α (1) = 0.05
Degrees of
freedom α (2) = 0.05 α (1) = 0.05
1 12.706 6.314 42 2.018 1.682
2 4.303 2.920 44 2.015 1.680
3 3.182 2.353 46 2.013 1.679
4 2.776 2.132 48 2.011 1.677
5 2.571 2.015 50 2.009 1.676
6 2.447 1.934 52 2.007 1.675
7 2.365 1.895 54 2.005 1.674
8 2.306 1.860 56 2.003 1.673
9 2.262 1.833 58 2.002 1.672
10 2.228 1.812 60 2.000 1.671
11 2.201 1.796 62 1.999 1.670
12 2.179 1.782 64 1.998 1.669
13 2.160 1.771 66 1.997 1.668
14 2.145 1.761 68 1.995 1.668
15 2.131 1.753 70 1.994 1.667
16 2.120 1.746 72 1.993 1.666
17 2.110 1.740 74 1.993 1.666
18 2.101 1.734 76 1.992 1.665
19 2.093 1.729 78 1.991 1.665
20 2.086 1.725 80 1.990 1.664
21 2.080 1.721 82 1.989 1.664
22 2.074 1.717 84 1.989 1.663
23 2.069 1.714 86 1.988 1.663
24 2.064 1.711 88 1.987 1.662
25 2.060 1.708 90 1.987 1.662
26 2.056 1.706 92 1.986 1.662
27 2.052 1.703 94 1.986 1.661
28 2.048 1.701 96 1.985 1.661
29 2.045 1.699 98 1.984 1.661
30 2.042 1.697 100 1.984 1.660
31 2.040 1.696 200 1.972 1.653
32 2.037 1.694 300 1.968 1.650
33 2.035 1.692 400 1.966 1.649
34 2.032 1.691 500 1.965 1.648
35 2.030 1.690 600 1.964 1.647
36 2.028 1.688 700 1.963 1.647
37 2.026 1.687 800 1.963 1.647
38 2.024 1.686 900 1.963 1.647
39 2.023 1.685 1000 1.962 1.646
40 2.021 1.684 ∞ 1.960 1.6455
Appendix A: Critical values of chi-square, t and F 377

Table A3 Critical values of the F distribution for ANOVA when α = 0.05. If the calculated value of F is larger than the critical value for the appropriate
degrees of freedom then it indicates a probability of < 0.05 (and is therefore considered significant with an α of 0.05). The columns in Table A3 give the
degrees of freedom for the numerator of the F ratio and the rows give the degrees of freedom for the denominator. For example, the critical value for F
3,7
is
4.35, so an F statistic larger than this is considered significant at P < 0.05. Values were calculated using the method given by Zelen and Severo (1964).
Denominator
degrees of freedom
Numerator degrees of freedom
123456789101215203050100200
1 161.45 199.50 215.71 224.58 230.16 233.99 236.77 238.88 240.54 241.88 243.90 245.00 248.01 250.10 252 253 254
2 18.51 19.00 19.16 19.25 19.30 19.33 19.35 19.37 19.38 19.40 19.41 19.43 19.45 19.46 19.47 19.49 19.49
3 10.13 9.55 9.28 9.12 9.01 8.94 8.89 8.85 8.81 8.79 8.75 8.70 8.66 8.62 8.58 8.55 8.54
4 7.71 6.94 6.59 6.39 6.26 6.16 6.09 6.04 6.00 5.96 5.91 5.86 5.80 5.75 5.70 5.66 5.65
5 6.61 5.79 5.41 5.19 5.05 4.95 4.88 4.82 4.77 4.74 4.68 4.62 4.56 4.50 4.44 4.41 4.39
6 5.99 5.14 4.76 4.53 4.39 4.28 4.21 4.15 4.10 4.06 4.00 3.94 3.87 3.81 3.75 3.71 3.69
7 5.59 4.74 4.35 4.12 3.97 3.87 3.79 3.73 3.68 3.64 3.58 3.51 3.45 3.38 3.32 3.27 3.25
8 5.32 4.46 4.07 3.84 3.69 3.58 3.50 3.44 3.39 3.35 3.28 3.22 3.15 3.08 3.02 2.97 2.95
9 5.12 4.26 3.86 3.63 3.48 3.37 3.29 3.23 3.18 3.14 3.07 3.01 2.94 2.86 2.80 2.76 2.73
10 4.96 4.10 3.71 3.48 3.33 3.22 3.14 3.07 3.02 2.98 2.91 2.85 2.77 2.70 2.64 2.59 2.56
11 4.84 3.98 3.59 3.36 3.20 3.10 3.01 2.95 2.90 2.85 2.79 2.72 2.65 2.57 2.51 2.46 2.43
12 4.75 3.89 3.49 3.26 3.11 3.00 2.91 2.85 2.80 2.75 2.69 2.62 2.54 2.47 2.40 2.35 2.32
13 4.67 3.81 3.41 3.18 3.03 2.92 2.83 2.77 2.71 2.67 2.60 2.53 2.46 2.38 2.31 2.26 2.23
14 4.60 3.74 3.34 3.11 2.96 2.85 2.76 2.70 2.65 2.60 2.53 2.46 2.39 2.31 2.24 2.19 2.16
15 4.54 3.68 3.29 3.06 2.90 2.79 2.71 2.64 2.59 2.54 2.48 2.40 2.33 2.25 2.18 2.12 2.10
16 4.49 3.63 3.24 3.01 2.85 2.74 2.66 2.59 2.54 2.49 2.43 2.35 2.28 2.19 2.12 2.07 2.04
17 4.45 3.59 3.20 2.97 2.81 2.70 2.61 2.55 2.49 2.45 2.38 2.31 2.23 2.15 2.08 2.02 1.99
18 4.41 3.56 3.16 2.93 2.77 2.66 2.58 2.51 2.46 2.41 2.34 2.27 2.19 2.11 2.04 1.98 1.95
19 4.38 3.52 3.13 2.90 2.74 2.63 2.54 2.48 2.42 2.38 2.31 2.23 2.16 2.07 2.00 1.94 1.91

20 4.35 3.49 3.10 2.87 2.71 2.60 2.51 2.45 2.39 2.35 2.28 2.20 2.12 2.04 1.97 1.91 1.88
21 4.32 3.47 3.07 2.84 2.69 2.57 2.49 2.42 2.37 2.32 2.25 2.18 2.10 2.01 1.94 1.88 1.84
22 4.30 3.44 3.05 2.82 2.66 2.55 2.46 2.40 2.34 2.30 2.23 2.15 2.07 1.98 1.91 1.85 1.82
23 4.28 3.42 3.03 2.80 2.64 2.53 2.44 2.38 2.32 2.28 2.20 2.13 2.05 1.96 1.88 1.82 1.79
24 4.26 3.40 3.01 2.78 2.62 2.51 2.42 2.36 2.30 2.26 2.18 2.11 2.03 1.94 1.84 1.80 1.77
25 4.24 3.39 2.99 2.76 2.60 2.49 2.40 2.34 2.28 2.24 2.16 2.09 2.01 1.92 1.84 1.78 1.75
26 4.23 3.37 2.98 2.74 2.59 2.47 2.39 2.32 2.27 2.22 2.15 2.07 1.99 1.90 1.82 1.76 1.73
27 4.21 3.35 2.96 2.73 2.57 2.46 2.37 2.31 2.25 2.20 2.13 2.06 1.97 1.88 1.81 1.74 1.71
28 4.20 3.34 2.95 2.71 2.56 2.45 2.36 2.29 2.24 2.19 2.12 2.04 1.96 1.87 1.79 1.73 1.69
29 4.18 3.33 2.93 2.70 2.55 2.43 2.35 2.28 2.22 2.18 2.10 2.03 1.94 1.85 1.77 1.71 1.67
30 4.17 3.32 2.92 2.69 2.53 2.42 2.33 2.27 2.21 2.17 2.09 2.01 1.93 1.84 1.76 1.70 1.66
40 4.08 3.23 2.84 2.61 2.45 2.34 2.25 2.18 2.12 2.08 2.00 1.92 1.84 1.74 1.66 1.59 1.55
50 4.03 3.18 2.79 2.56 2.40 2.29 2.20 2.13 2.07 2.03 1.95 1.87 1.78 1.69 1.60 1.52 1.48
60 4.00 3.15 2.76 2.53 2.37 2.25 2.17 2.10 2.04 1.99 1.92 1.84 1.75 1.65 1.56 1.48 1.44
70 3.98 3.13 2.74 2.50 2.35 2.23 2.14 2.07 2.02 1.97 1.89 1.81 1.72 1.62 1.53 1.45 1.40
80 3.96 3.11 2.72 2.49 2.33 2.21 2.13 2.06 2.00 1.95 1.88 1.79 1.70 1.60 1.51 1.43 1.38
90 3.95 3.10 2.71 2.47 2.32 2.20 2.11 2.04 1.99 1.94 1.86 1.78 1.69 1.59 1.49 1.41 1.36
100 3.94 3.09 2.70 2.46 2.31 2.19 2.10 2.03 1.97 1.93 1.85 1.77 1.68 1.57 1.48 1.39 1.34
200 3.89 3.04 2.65 2.42 2.26 2.14 2.06 1.98 1.93 1.88 1.80 1.72 1.62 1.52 1.41 1.32 1.26
500 3.86 3.01 2.62 2.39 2.23 2.12 2.03 1.96 1.90 1.85 1.77 1.69 1.59 1.48 1.38 1.28 1.21
Appendix B: Answers to questions
2.8 (1) The “hypothetico-deductive” model is that science is done by
proposing a hypothesis, which is an idea about a phenomenon or
process that may or may not be true. The hypothesis is used to
generate predictions that can be tested by doing a mensurative or a
manipulative experiment. If the results of the experiment are con-
sistent with the predictions the hypothesis is retained. If they are
not (for an experiment that appears to be a good test of the
predictions) the hypothesis is rejected. By convention, a hypothesis
is stated as two alternatives: the null hypothesis of no effect or no
difference, and the alternate hypothesis which states an effect. For
example, “Apatite treatment affects the amount of lead leached
from soil” is an alternate hypothesis, and the null is “Apatite treat-
ment does not affect the amount of lead leached from soil.”
Importantly a hypothesis can never be proven because there is
always the possibility that new evidence may be found to disprove
it. A “negative” outcome, where the alternate hypothesis is rejected,
is still progress in our understanding of the natural world and
therefore just as important as a “positive” outcome where the null
hypothesis is rejected.
2.8 (2) The value recorded from only one sampling or experimental unit
may not be very representative of the remainder of the population.
4.9 (1) An example of confusing a correlation with causality is when two
variables are related (that is, they vary together) but neither causes
the other to change. For example, as depth in the ocean increases,
light intensity decreases and pressure increases, but the decrease in
light intensity does not cause the increased pressure or vice versa.
4.9 (2) “Apparent replication” is when an experiment (either mensurative
or manipulative) contains replicates, but the placement or collective
380

treatment of the replicates reduces the true amount of replication.
For example, if you had two different treatments replicated several
times within only two furnaces set at different temperatures the
level of replication is actually the furnace in each treatment (and
therefore one). Another example could be two different heavy
metal rehabilitation treatments applied to each of 10 plots, but
all 10 plots of one treatment were clustered together in one place
on a mining lease and all 10 of the other treatment were clustered
in another.
5.6 (1) Copying the mark for an assignment and using it to represent an
examination mark is grossly dishonest. First, the two types of
assessment are different. Second, the lecturer admitted the varia-
tion between the assignment and exam mark was “give or take
15%” so the relationship between the two marks is not very precise
and may severely disadvantage some students. Third, there is no
reason why the relationship between the assignment and exam
mark observed in past classes will necessarily apply in the future.
Fourth, the students are being misled: their performance in the
exam is being ignored.
5.6 (2) It is not necessarily true that a result with a small number of
replicates will be the same if the number of replicates is increased,
because a small number is often not representative of the popula-
tion. Furthermore, to claim that a larger number was used is
dishonest.
6.11 (1) Many scientists would be uneasy about a probability of 0.06 for the
result of a statistical test because this non-significant outcome is
very close to the generally accepted significance level of 0.05. It
would be helpful to repeat the experiment.
6.11 (2) Type 1 error is the probability of rejecting the null hypothesis when
it is true. Type 2 error is the probability of rejecting the alternate
hypothesis when it is true.
6.11 (3) The 0.05 level is the commonly agreed upon probability used for
significance testing: if the outcome of an experiment has a prob-
ability of less than 0.05 the null hypothesis is rejected. The 0.01
probability level is sometimes used when the risk of a Type 1 error
(i.e. rejecting the null hypothesis when it is true) has very impor-
tant consequences. For example, you might use the 0.01 level when
Appendix B: Answers to questions 381

assessing a new filter material for reducing the airborne concen-
tration of hazardous particles. You would need to be reasonably
confident that a new material was better than existing ones before
recommending it as a replacement.
7.12 (1) For a population of fossil shells with a mean length of 100 mm and a
standard deviation of 10 mm, the finding of a 78 mm shell is unlikely
(because it is more than 1.96 standard deviations away from the
mean) but not impossible: 5% of individuals in the population
would be expected to have shells either ≥ 119.6 mm or ≤ 80.4 mm.
7.12 (2) The variance calculated from a sample is corrected by dividing by
n − 1 and not n in an attempt to give a realistic indication of the
variance of the population from which it has been taken, because a
small sample is unlikely to include sampling units from the most
extreme upper and lower tails of the population that will never-
theless make a large contribution to the population variance.
8.10 (1) These data are suitable for analysis with a paired-sample t test
because the two samples are related (the same 10 crystals are in
each). The test would be two-tailed because the alternate hypoth-
esis is non-directional (it specifies that opacity may change). The
test gives a significant result ( t
9
= 3.161, P < 0.05).
8.10 (2) The t statistic obtained for this inappropriate independent sample t
test is −0.094 and is not significant at the two-tailed 5% level. The
lack of significance for this test is because the variation within each
of the two samples is considerable and has obscured the small but
relatively consistent increase in opacity resulting from the treat-
ment. This result emphasizes the importance of choosing an
appropriate test for the experimental design.
8.10 (3) This exercise will initially give a t statistic of zero and a probability
of 1.0, meaning that the likelihood of this difference or greater
between the sample mean and the expected value is 100%. As the
sample mean becomes increasingly different to the expected mean
the value of t will increase and the probability of the difference will
decrease and eventually be less than 5%.
9.8 (1) A non-significant result in a statistical test may not necessarily be
correct because there is always a risk of either Type 1 error or Type
2 error. Small sample size will particularly increase the risk of Type
2 error – rejecting the alternate hypothesis when it is correct.
382 Appendix B: Answers to questions
