Steve M., Darby D.M., Geostatistics Explained - An Introductory Guide for Earth Scientists

Подождите немного. Документ загружается.

(2) Explain why a statistician said “Autocorrelation is very useful for

relatively long sequences, but for a sequence of 20 the signiﬁcance of

any autocorrelation means little, especially at high lags.”

(3) The data in the table below are for summers when heatwaves occurred

(with a heatwave deﬁned as a continuous sequence of ﬁve or more days

on which the maximum temperature exceeded 40 °C), at Port Nundy in

southern Australia from 1850 to 2009. You have been asked to test the

hypothesis “The frequency of heatwaves has not changed from 1850–

2009 at Port Nundy.” (a) What analysis would you use? (b) What can

you conclude from this analysis? (c) Do heatwaves appear to occur at

random during this temporal sequence?

1850 1881 1912 1948 1974

1851 1884 1914 1950 1978

1852 1885 1919 1951 1980

1853 1890 1921 1953 1981

1854 1892 1924 1957 1982

1856 1893 1925 1958 1984

1859 1895 1926 1960 1986

1864 1899 1934 1961 1988

1865 1900 1935 1962 1990

1870 1903 1939 1964 1992

1877 1904 1944 1968 1995

1878 1907 1945 1971 2004

1880 1910 1947 1973 2009

21.16 Questions 333

22 Introductory concepts of

spatial analysis

22.1 Introduction

Earth scientists often rely on diﬀerent types of maps where the spatial

location of each sampling unit is one of the variables of interest. For

example, a geoscientist might have data for the presence or absence of

copper-bearing ore at 54 test cores drilled within the sampling space of a

10 000 square mile mining lease. The eﬀectiveness of any further prospec-

ting would be improved if you knew whether the spatial distribution of cores

showing copper-bearing ores occurred at random or in some sort of pattern

within the sampling space. The methods for summarizing and analyzing

such data are called spatial analyses.

Even though the Earth is three-dimensional, most summary spatial infor-

mation is presented as two-dimensional maps representing the Earth’ssur-

face, often with an overlay to indicate other variables. For example, maps

showing landforms are printed on two-dimensional sheets, with contour

lines and numbers to show the third dimension of elevation. Similarly, a map

of the location and ﬂow per minute of test wells for oil might indicate ﬂow

with numbers (e.g. the average number of barrels per day from each well) or

display this as the proportional height of a single bar at each well.

The location of any point on a two-dimensional surface can be accurately

and precisely deﬁned by its X and Y coordinates, which are the distances in

two directions at 90° to each other from a set reference point, in just the same

way as a graph is used to display a two-dimensional scatter plot of bivariate

data. Interpretation of a ﬂat, two-dimensional display is so easy that even

though the Earth is approximately spherical and any location on the surface

of this sphere can be deﬁned by the degrees, minutes and seconds of its

latitude and longitude, cartographers have developed several transformations

for projecting this sphere on a two-dimensional surface for use as a map.

334

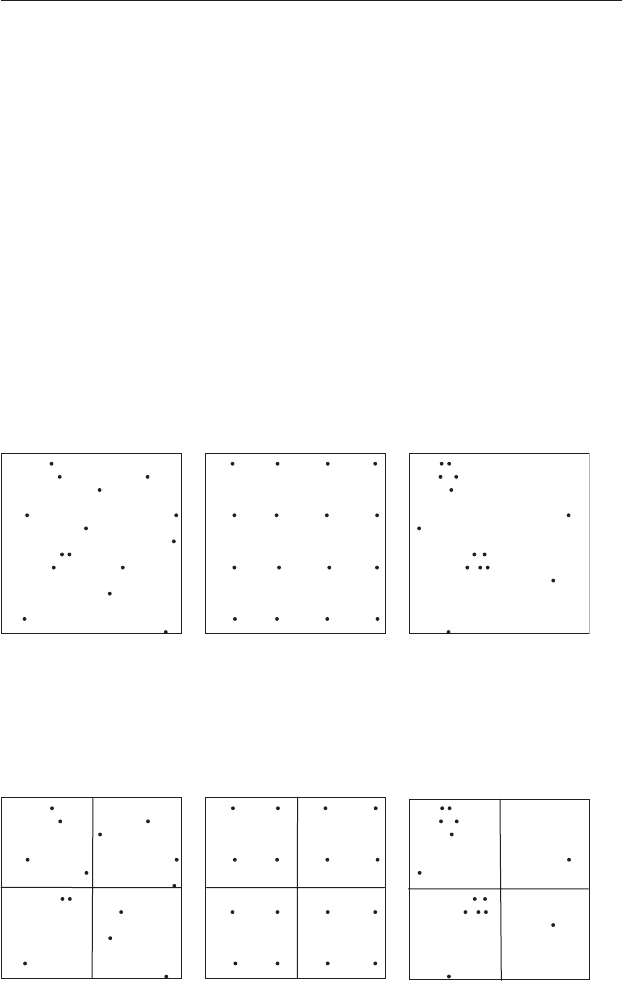
This chapter is an introduction to some of the essential concepts of two-

dimensional spatial analysis.

22.2 Testing whether a spatial distribution occurs

at random

The distribution of points within a two- (or higher) dimensional sampling

space can occur (a) at random, (b) more regularly than expected by chance and

One procedure used to test for randomness within two dimensions is to

subdivide a sampling space into several smaller replicate blocks of equal size

that are often called quadrats (Figure 22.2). The number of points within

each quadrat is counted and used to produce a summary table of frequencies

(a) Random (b) Regular (c) Clustered

Figure 22.1 Examples of the distribution of points in two-dimensional

space. (a) A random pattern. (b) A regular pattern is more uniform. (c) A

clustered pattern is less uniform than random.

(a) Random (b) Regular (c) Clustered

Figure 22.2 When a sampling space is subdivided into quadrats of equal size,

the variation among quadrats will be (a) intermediate for a random

distribution, (b) smaller for a regular one and (c) larger for a clustered one.

22.2 Testing for randomness 335

(Chapter 3) showing the number of quadrats containing zero, 1, 2 ,3 …. etc.

points that can be used to assess the spatial distribution of the variable.

If the distribution is random, then the frequency of cases per quadrat

would be expected to have a distribution and variance that would be

characteristic of a random variable.

If the points are more evenly distributed than expected by chance, the

variation among quadrats will be relatively small (because each quadrat will

contain very similar numbers).

If the points are less evenly distributed than expected by chance (and

therefore clustered), the variation among quadrats will be relatively large

(because some will contain no or very few points, but others will contain

a lot).

You can assess whether any departure from randomness is statistically

signiﬁcant by comparing the observed frequencies to those expected for a

random variable. One of the most straightforward ways of doing this is to

calculate the frequencies expected for a Poisson distribution (Chapter 7).

The mean number of points per quadrat is the total number of points (m)

within the sampling space, divided by the number of quadrats (n):

X ¼

(22:1)

This sample mean is used as an estimate of the population mean μ in the

following formula, which gives the expected proportion of quadrats con-

taining each number of points expected under the Poisson distribution:

PðXÞ¼





(22:2)

Equation (22.2) appears complicated, but it can be explained by separat-

ing it into its components.

First, P(X) is the expected probability that a quadrat will contain X

number of points (e.g. P(3) is the probability that a quadrat will contain

three points).

Second, e

−μ

speciﬁes the exponential, e, (which is the base of the natural

logarithm and a constant equal to 2.718281728) raised to the power of −μ,

where μ is the population mean. For example, if the population mean is 2.0,

then e

−μ

= (2.718281728)

−2

, which is the square root of 2.718281728.

Third, μ

is the population mean raised to the power of X, where X is the

expected number of points per quadrat. For example, for a population mean

336 Introductory concepts of spatial analysis

of 2.0 and the expected category of three points per quadrat, μ

which is 8.

Finally, X! is the symbol for the factorial of X and speciﬁes “All of the

positive integers up to and including X multiplied together and then multi-

plied by 1.0.” For example:

3! ¼ð1  2  3Þ1 ¼ 6

5! ¼ð1  2  3 4 5Þ1 ¼ 120

Importantly:

1! ¼ð1Þ1 ¼ 1

and

0! ¼ðno numerical valueÞ1 which also equals 1:

Note here that “ no numerical value” does not mean zero. It means that no

number is present (because there are no positive integers less than zero).

Using Equation (22.2) for a population mean of 3.0, the expected Poisson

probability of a quadrat containing ﬁve points is:

Pð5Þ¼

ð2:71828Þ

3

ð3:0Þ

which is 0.01. So if the distribution of points is random, 1% of the total

number of quadrats would be expected to contain ﬁve points. The same

formula can be used to calculate the expected proportions of quadrats

containing any number of points.

Equation (22.2) is tedious to calculate, but Box 22.1 gives all the functions

you need to write a Microsoft Excel® spreadsheet that will automatically give

the expected Poisson probabilities for any number per quadrat, provided the

mean is known.

One analysis for non-randomness uses the chi-square test (Chapter 18)to

compare the observed and expected frequencies. There is a worked example

in Section 22.2.1.

22.2.1 A worked example

If you ever want to ﬁnd a meteorite, go to Antarctica! The combination of

the lack of ground cover (making meteorites easy to spot) and ﬂow of ice

22.2 Testing for randomness 337

Box 22.1 A spreadsheet Poisson calculator

The following table can be written into a Microsoft Excel

spreadsheet to

give a calculator for the expected Poisson probability for quadrats containing

zero, 1, 2 3 … etc. points, provided the mean is known. It uses the formula:

PðXÞ¼





(22:3 copied from 22:2)

Open a new blank Microsoft Excel

spreadsheet. Start at cell A1 (the top

left hand cell in the spreadsheet) and write in the following descriptions and

formulae exactly as they are given below. You must start at cell A1, in which

youshouldwritetheword‘Mean’. The calculator must only occupy the

blockofcellsfromA1toB6.Makesureyouincludetheminussigninthe

worksheet function given in cell B3, which must be written as: =EXP(-B1).

1 Mean

2 Expected number per quadrat

3 =EXP(-B1)

4 =POWER(B1,B2)

5 =FACT(B2)

6 Probability =(B3*B4)/B5

Once you have entered all the functions given above, type the numerical

value of the mean into cell B1 and the number per quadrat (here you might

start with zero) in cell B2 immediately below this. Do not type numbers

into any other cells. The spreadsheet will automatically give you the

probability for each number per quadrat if the Poisson distribution

applies. This probability is displayed to the right of “Probability,” in cell

B6 where you had speciﬁed the worksheet function =(B3*B4)/B5.

As a quick check, if you put in a mean of 3.805 in cell B1 and an

expected number per quadrat of 4.0 in cell B2, the probability should be

0.19440924. Your spreadsheet should look the same, or similar to the one

here (depending on the number of decimal places you specify for the cells):

1 Mean 3.805

2 Expected number per quadrat 4

3 0.0222592

4 209.613208

524

6 Probability 0.19440924

against mountains (which brings old ice to the surface, where it is deﬂated

by winds that expose meteorites) makes Antarctica a premier hunting

ground for meteorite recovery. Every year since 1976, at least one team of

scientists has spent several weeks during the southern hemisphere summer

searching for meteorites from stranding surfaces along the Transantarctic

Mountains of Antarctica. Their job would be made easier if the distribution

of meteorites could be better understood.

Table 22.1 gives summary data for the number of quadrats containing

zero, 1, 2, etc. meteorites within 200 km

that has been subdivided into 200

quadrats of equal area. Note that the expected frequencies have been

combined for more than 7 meteorites per quadrat. In total, there were 761

meteorites distributed within the 200 quadrats.

The expected proportions of quadrats containing each number of mete-

orites were calculated using Equation (22.2) and multiplied by 200 to give

the expected frequencies. Finally the observed and expected frequencies

were used in Equation (22.4) to obtain the chi-square statistic for the nine

(0 – 8+ meteorites per quadrat) categories:

i¼1

ðo

 e

(22:4 copied from 18:1)

Table 22.1 The frequencies of quadrats containing zero, 1, 2, etc. meteorites,

and the expected frequencies if the spatial distribution of meteorites is random.

Number of meteorites

per quadrat

Observed

frequency of cases

Expected proportion

if random

Expected frequency

if random

0 10 0.022592 4.4

1 14 0.084696 16.9

2 9 0.161135 32.2

3 23 0.204372 40.9

4 65 0.194409 38.9

5 74 0.147945 29.6

6 5 0.093822 18.8

7 0 0.050999 10.2

8 and more 0 0.040621 8.1

Total n = 200 1.000000

Mean 3.805

22.2 Testing for randomness 339

which is: w

¼ 144:82, P < 0.001. The distribution of meteorites is signiﬁ-

cantly non-random.

If a chi-square analysis shows the distribution is non-random, you are

very likely to want to know whether it is more even, or more clustered. For a

Poisson distribution, the variance and the mean are numerically the same.

For a regular distribution, the variance will be smaller than the mean and for

a clustered distribution it will be larger.

Box 22.2 The number of degrees of freedom for a chi-square

comparison when the formula for the expected frequencies

includes a sample statistic

The value of chi-square for the comparison of k observed and expected

frequencies derived from the Poisson distribution has k – 2 degrees of

freedom. In Chapter 18 and elsewhere, however, it was explained that the

number of degrees of freedom for a goodness-of- ﬁt test is k – 1. For a

ﬁxed total of observations, the numbers within k – 1 categories are free to

vary, but those in the “ﬁnal” category to be ﬁlled can only be one number,

which is therefore a ﬁxed quantity.

When expected frequencies are derived externally (e.g. an expectation

of 3 : 1 on the basis of some hypothesized property of the system, or 1 : 1 :

1 on the basis of an expectation of equal frequencies, as discussed in

Chapter 18), the degrees of freedom are one less than the number of

categories (Section 18.2).

In example 22.2.1, the expected proportions per category have been

calculated using the Poisson formula (22.2), which used the sample

mean as the best estimate of the population mean. The use of a statistic

taken from the sample being tested will result in the loss of one more

degree of freedom, because for a set sample mean, all but one of the

values of the sampling units contributing to that mean are free to vary.

Therefore, the degrees of freedom for the chi-square test in this example

must be k − 2.

If instead you have an independent estimate of the population mean

(perhaps from a more extensive study), the number of degrees of free-

dom for the chi-square test is k − 1.

340 Introductory concepts of spatial analysis

The mean number per quadrat is the total number of points (here the 761

meteorites) divided by the number of quadrats (here 200), giving a mean of

3.805:

X ¼

(22:5 copied from 22:1)

The variance is calculated using the formula for the sample variance:

i¼1

ðX

 XÞ

n  1

(22:6 copied from 7:6)

where n is the number of quadrats and X

is the number of meteorites in the

ﬁrst, through to the ﬁnal quadrat (here the 200th).

The variance to mean ratio is the variance divided by the mean:

(22:7)

and should be about 1.0 for a random distribution.

The value of s

=X can be compared to an expected value of 1.0 by using a

single-sample t test, although a modiﬁcation has to be made to the formula

for that test given in Chapter 8. The variance to mean ratio is derived from

the sample variance, but the formula for the t test includes the standard

error of the mean, which is also calculated from the sample variance. To use

both in the same formula will result in bias. Instead, an independent (and

conservatively large) estimate of the standard error of the mean is made

only from n, the number of quadrats:

SEMðestÞ¼

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

n  1

(22:8)

Therefore the equation for the single-sample t test is:

n1

 1:00

SEMðestÞ

(22:9)

where n is the number of quadrats. If s

=X is 1.0, the numerator will be zero

and so will the value of t. A signiﬁcant value means that the distribution is

either even or clustered: when the variance divided by the mean is smaller

than 1.0, the distribution is more even and when it is greater than 1.0, the

distribution is more clustered. Sometimes this statistic is given as the mean

22.2 Testing for randomness 341

divided by the variance, so you need to make sure which version has been

used when interpreting results in reports and publications.

For the example in Table 22.1, the sample variance is 2.17 and the mean is

3.805. Therefore the variance to mean ratio is 0.57. The independent

estimate of the SEM is:

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

n  1

ﬃﬃﬃﬃﬃﬃﬃ

199

¼ 0:100251:

When these values are put into Equation (22.9):

199

0:57  1:00

0:100251

¼4:29

which is highly signiﬁcant (Appendix A). The distribution of meteorites is

relatively even among the 200 quadrats. The signiﬁcant result is consistent

with the procedure for comparing the distribution to the Poisson described

earlier in this section, but because the variance to mean test is relatively

conservative you may ﬁnd in some cases that the “Poisson” method is just

signiﬁcant at P < 0.05 and the less powerful variance to mean ratio test is

not. In these cases the variance to mean ratio still indicates the direction

(e.g. even or clustered) of the departure from a random distribution.

22.2.2 The problem of scale

The procedure for assessing randomness described above is sensitive to

quadrat size. For example, a distribution may show extreme clustering on a

relatively small scale that may not be detected on a larger scale (Figure 22.3).

This is another example of the usefulness of making a preliminary inspec-

tion of a set of data, which could be done by plotting the distribution of

points on a two-dimensional map and examining it for any obvious pattern

(Figure 22.3).

22.2.3 Nearest neighbor analysis

Another test for a pattern in spatial data that is not sensitive to the problem

of scale described above, is nearest neighbor analysis. This is used in

many areas of science, including studies of the spatial distributions of

plants and animals. As the number of points within a sampling space

342 Introductory concepts of spatial analysis