Simon Benninga. Financial Modelling 3-rd edition
Подождите немного. Документ загружается.

228 Chapter 7
7.4.1 Back to the Leveraged-Lease Example
We now apply this same logic to the cash fl ows of the leveraged lease.
24
25
26
27
28
29
30
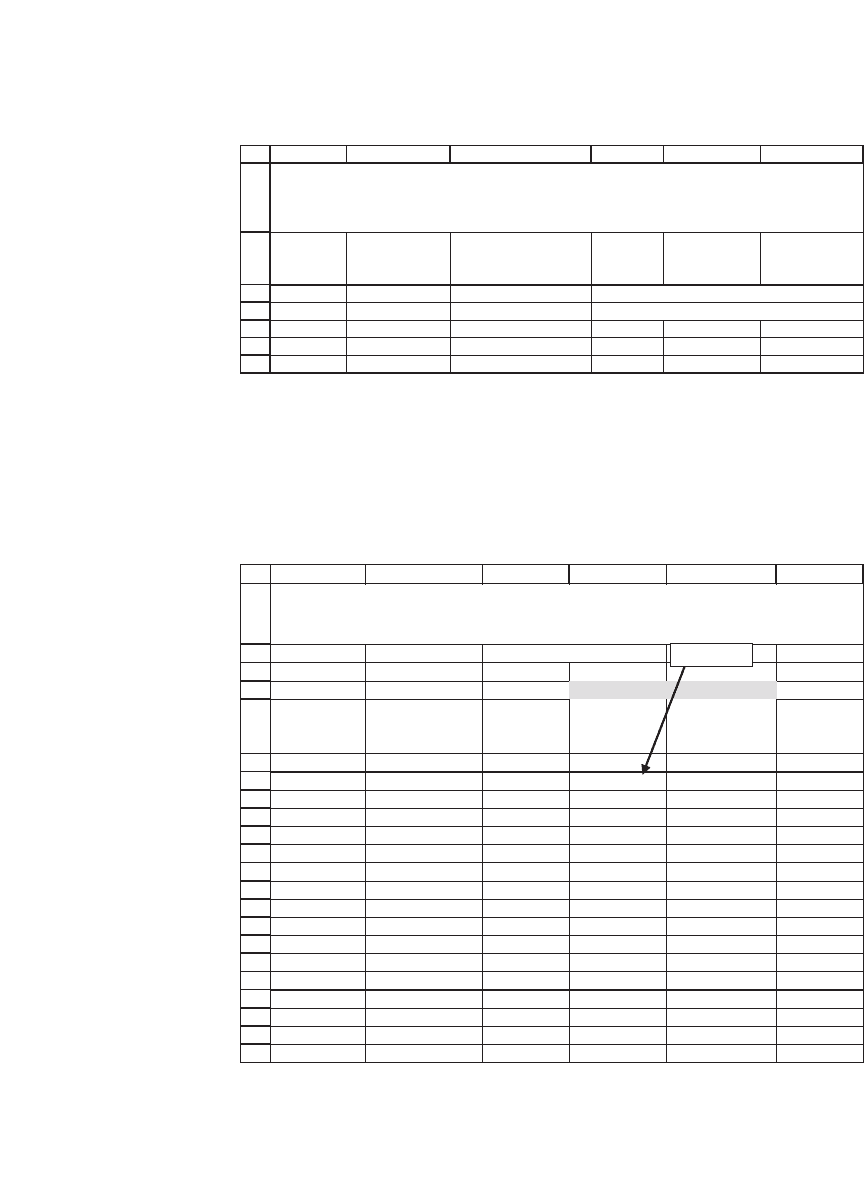
AB C DE F
Year
Cash flow at
end of year
Investment at
beginning of period
1 31,000 100,000 <-- =NPV($B$11,B26:$B$30)
2 22,000 77,097 <-- =NPV($B$11,B27:$B$30)
3 16,000 61,339
4 22,000 50,305
5 35,000 32,378
THE INVESTMENT AT THE BEGINNING OF EACH PERIOD IS THE
PRESENT VALUE (USING IRR AS DISCOUNT RATE) OF ALL FUTURE
CASH FLOWS
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
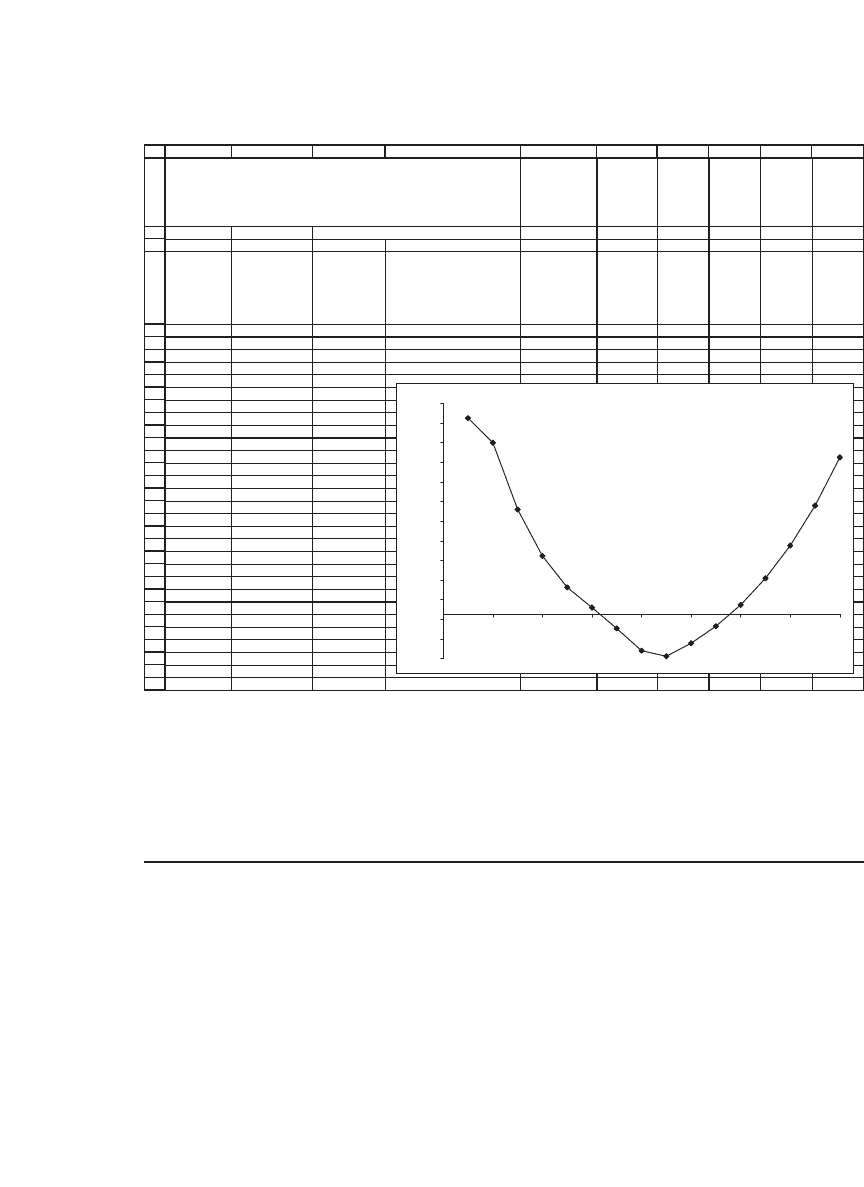
ABCDEF
IRR 12.46% <-- =IRR(C6:C22)
Attribution of cash flow
Year
Investment at
beginning of
period
Cash flow Income
Repayment of
investment
0 -200,000
1 200,000 49,941 24,913 25,028 <-- =C7-D7
2 174,972 89,774 21,796 67,978
3 106,994 60,666 13,328 47,338
4 59,656 39,487 7,431 32,056
5 27,600 23,827 3,438 20,389
6 7,212 22,352 898 21,454
7 -14,242 20,730 -1,774 22,504
8 -36,746 1,186 -4,577 5,763
9 -42,510 -18,697 -5,295 -13,401
10 -29,108 -20,856 -3,626 -17,230
11 -11,878 -23,231 -1,480 -21,751
12 9,873 -25,843 1,230 -27,073
13 36,945 -28,716 4,602 -33,319
14 70,264 -31,877 8,753 -40,630
15 110,894 -35,354 13,814 -49,168
16 160,062 180,000 19,938 160,062
ATTRIBUTION OF LEVERAGED LEASE CASH FLOWS TO
INCOME AND REPAYMENT OF INVESTMENT
=$B$2*B7

229 The Financial Analysis of Leveraged Leases
Note that—as in our simple example—the IRR of 12.46 percent suc-
cessfully attributes income in such a way that the whole of the investment
is accounted for at the end of the project’s life (after 16 years, for the
case of the leveraged lease). However, note that there are some unusual
features of the table: fi ve of the income fi gures are negative, as are seven
of the “repayment of investment” terms. There are two ways to under-
stand these features:
•
In “mechanical” terms, the only way to make the table work is to have
some negative income numbers. This interpretation, though true, is not
very interesting.
•
In economic terms, the negative income fi gures mean that in some
years the project is not worth holding onto, but that it cannot be given
away. As an example consider the lessor’s position at the beginning of
year 9. Seven years of negative cash fl ows lie ahead. Only in eight years,
in year 16, will the lessor again see a positive cash fl ow from the lease.
A rational lessor would like to give away the lease contract at this point;
the present value of the cash fl ows at the beginning of year 9 at a 10
percent discount rate is −$39,333 (and this includes the realization of the
residual value at the end of year 16!). But of course no rational investor
would take over the contract at the beginning of year 6 unless she were
paid to do so, or unless her discount rate were negative. It is this fact—
that the lessor would have to pay someone to take the contract off her
hands in year 9—that makes us attribute negative income to the project
at this point.
4
In economic terms the lease at this point is worse than
valueless; it is a burden.
Using our insight that the investment at the beginning of each period
is the present value of all future cash fl ows discounted at the IRR, we
can see this phenomenon in a different way.
4. Negative income attribution in fact starts in year 7, showing that already at this point
the project has negative economic value.
230 Chapter 7
As we shall see in the next section, the negative economic value of
leveraged leases has caused the accounting profession a considerable
headache.
7.5 Accounting for Leveraged Leases: The “Multiple Phases Method”
Financial Accounting Standards Board statement 13 (FASB 13) man-
dates that the lessor in a leveraged lease allocate the cash fl ow from the
lease between income and investment. The logical way to do so would
be to use the IRR of the lease’s cash fl ows in the way illustrated in the
preceding section. But here the promulgators of FASB 13 apparently ran
up against the troublesome facet of human nature that hates to record
a loss even if it is economically warranted. (The implausibility of the
method for leveraged leases mandated in the statement is explained only
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
AB C D EFGHI
IRR 12.46% <-- =IRR(B5:B21)
Year
Leveraged-
lease cash
flows
Value of
lease cash
flows at
beginning of
period
0-200,000
1 49,941 200,000 <-- =NPV($B$2,B6:$B$21)
2 89,774 174,972 <-- =NPV($B$2,B7:$B$21)
3 60,666 106,994 <-- =NPV($B$2,B8:$B$21)
4 39,487 59,656
5 23,827 27,600
6 22,352 7,212
7 20,730 -14,242
8 1,186 -36,746
9 -18,697 -42,510
10 -20,856 -29,108
11 -23,231 -11,878
12 -25,843 9,873
13 -28,716 36,945
14 -31,877 70,264
15 -35,354 110,894
16 180,000 160,062
ECONOMIC VALUE OF THE LEASE CASH FLOWS:
DISCOUNTING THE CASH FLOWS AT THE IRR
J
Economic Value of Lease Cash Flows at Beginning of Period
-45,000
-25,000
-5,000
15,000
35,000
55,000
75,000
95,000
115,000
135,000
155,000
175,000
195,000
215,000
0 2 4 6 8 10121416

231 The Financial Analysis of Leveraged Leases
by the assumption that lessors did not want, under any circumstances, to
record economic losses that stemmed from the leases.)
The method that was devised to avoid the reporting of negative income
is sometimes termed the multiple-phases method (MPM). A better term
might have been “bastardized IRR method.”
The fact that a somewhat silly method of recognizing income is used
shouldn’t bother us, since foolishness is rampant in this world. However,
the complexity and the opaqueness of the method have lent it respecta-
bility. (There must be a lesson in this!) A little debunking, in the form of
an explanation, is in order.
Suppose we let the multiple-phases rate of return Q (short for quirky)
be defi ned as follows:
Year MPM Explanation
1 The lessor’s investment in the lease at the
beginning of year 1 is equal to her initial
investment in the lease’s equity. In our
example, Investment(1) = $200,000
This is the same as the
calculation of the IRR.
t
The lessor’s accounting income from the
lease at the end of year t is
Q ∗
()
>
{
Investment 1
if this number
otherwise
0
0
If income is positive,
MPM follows the
attribution of income
and investment of the
standard IRR method.
Otherwise, income is set
to zero.
t
The lessor’s investment in the lease at the
beginning of any year t > 1 is defi ned as
Investment(t) = Investment(t − 1)
−[Cash fl ow(t − 1) − Income(t − 1)]
Follows IRR method.
Last year
Cash fl ow(last year) =
Investment(last year)
*
(1 + Q)
Similar to IRR method.
Calculating the MPM Q: There is no formula to do this. However, it can
be done in Excel by using an iterative method, Solver or Goal Seek (see
next subsection).
232 Chapter 7
7.5.1 Calculating the Multiple-Phases-Method Rate of Return
To calculate the MPM rate Q, we fi rst set up a spreadsheet similar to the
one we used to illustrate the IRR. The one difference is that we have
extended the years to include year 17 (one year after the project ends).
A solution Q should give a zero investment at the beginning of year 17.
The following, for example is not a solution for Q:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
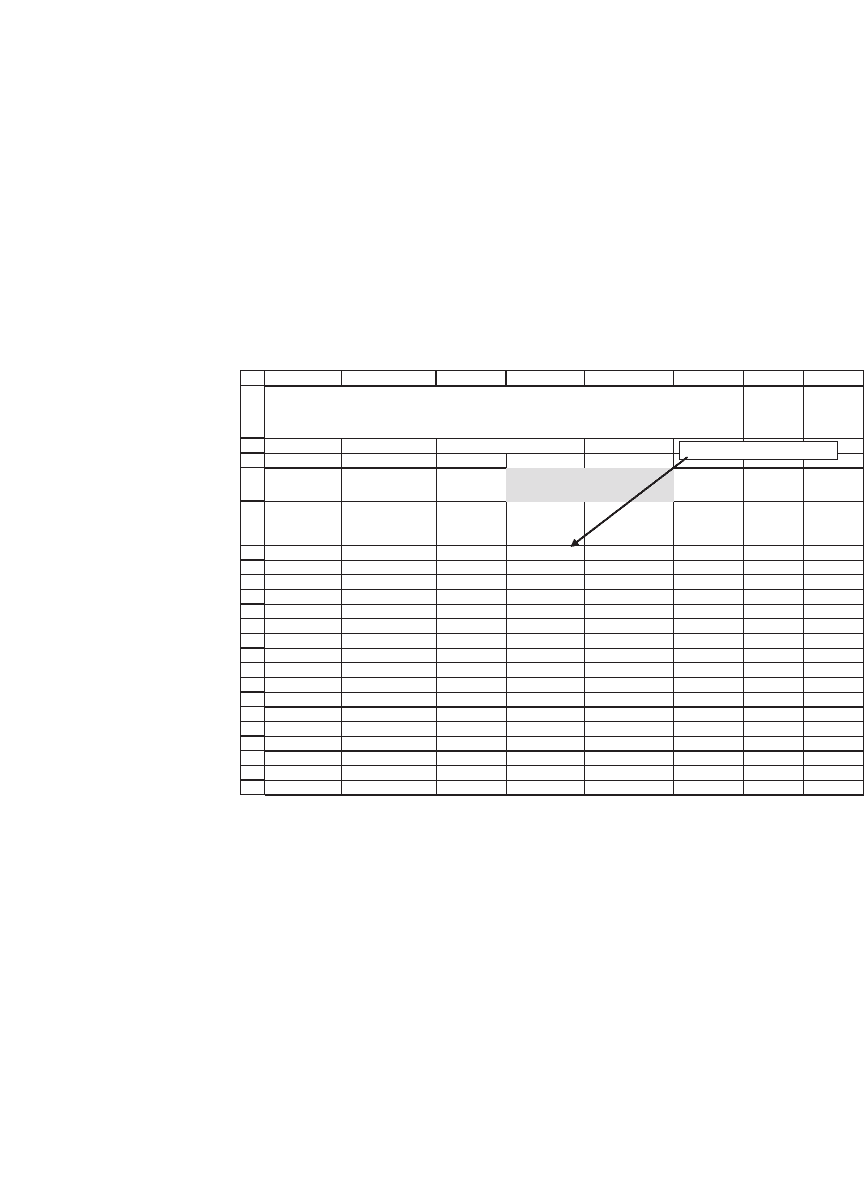
ABCDEFG
H
Q
12.00% <-- Possible MPM rate
Year
Investment at
beginning of
period
Leveraged-
lease cash
flows
Income
Repayment of
investment
1 200,000 49,941 24,000 25,941 <-- =C6-D6
2 174,059 89,774 20,887 68,887
3 105,172 60,666 12,621 48,045
4 57,127 39,487 6,855 32,632
5 24,495 23,827 2,939 20,887
6 3,608 22,352 433 21,919
7 -18,312 20,730 0 20,730
8 -39,042 1,186 0 1,186
9 -40,228 -18,697 0 -18,697
10 -21,531 -20,856 0 -20,856
11 -675 -23,231 0 -23,231
12 22,556 -25,843 2,707 -28,550
13 51,105 -28,716 6,133 -34,849
14 85,954 -31,877 10,314 -42,192
15 128,146 -35,354 15,378 -50,732
16 178,878 180,000 21,465 158,535
17 20,343
MPM METHOD: ATTRIBUTION OF LEVERAGED-LEASE CASH
FLOWS TO INCOME AND REPAYMENT OF INVESTMENT
MPM attribution of cash
flows
=IF($B$2*B6>0,$B$2*B6,0)
All the formulas in this table are the same as in the case of the IRR,
with the exception of the formulas in the income column. For example,
the income in year 1 has the following formula:
=IF($B$2*B6>0,$B$2*B6,0)
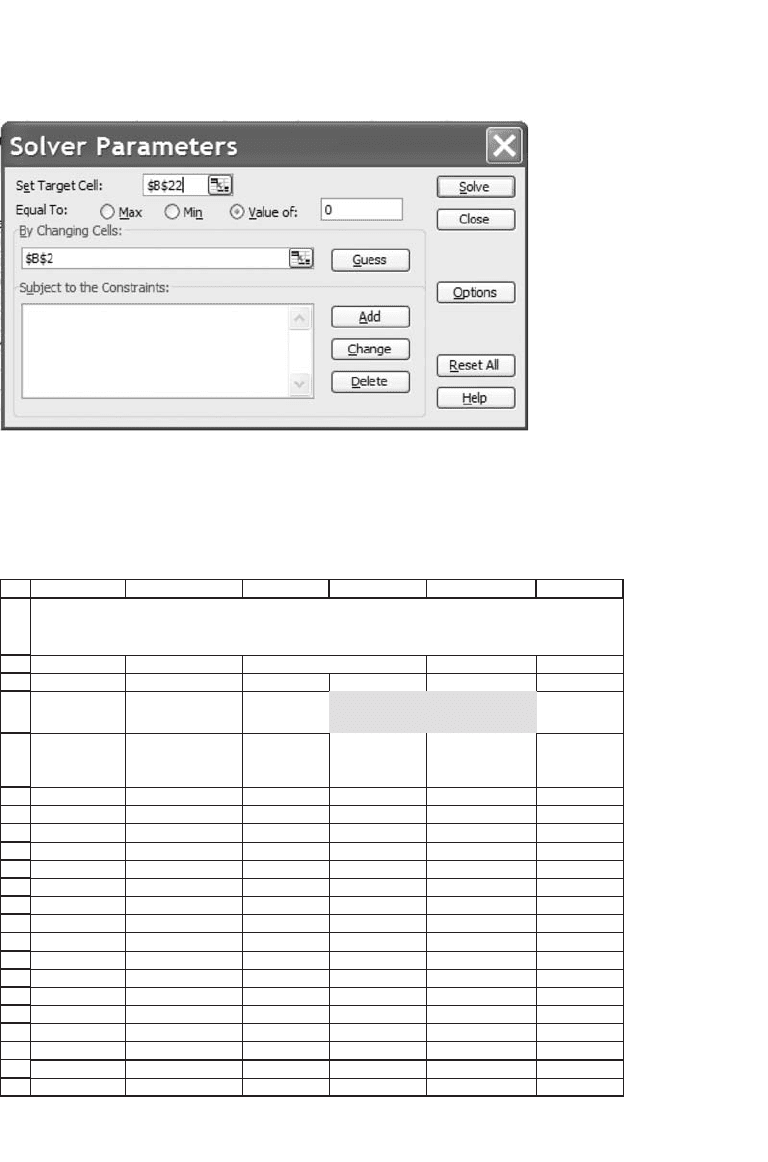
Using the Excel Solver, we fi nd the solution for Q. The solver
(Tools|Solver) dialog box looks like this:
233 The Financial Analysis of Leveraged Leases
The target cell $B$22 is the investment at the beginning of year 17; since
this is one year after the project ends, this investment should be zero.
When this method is applied in our case, the solution is as follows:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
ABCDEF
Q
10.91% <-- Possible MPM rate
Year
Investment at
beginning of
period
Leveraged-
lease cash
flows
Income
Repayment of
investment
1 200,000 49,941 21,822 28,119 <-- =C6-D6
2 171,881 89,774 18,754 71,019
3 100,862 60,666 11,005 49,661
4 51,201 39,487 5,587 33,901
5 17,301 23,827 1,888 21,939
6 -4,638 22,352 0 22,352
7 -26,990 20,730 0 20,730
8 -47,721 1,186 0 1,186
9 -48,906 -18,697 0 -18,697
10 -30,210 -20,856 0 -20,856
11 -9,354 -23,231 0 -23,231
12 13,877 -25,843 1,514 -27,357
13 41,234 -28,716 4,499 -33,216
14 74,449 -31,877 8,123 -40,001
15 114,450 -35,354 12,488 -47,842
16 162,292 180,000 17,708 162,292
17 0
USING SOLVER TO FIND THE MULTIPLE-PHASES-METHOD (MPM)
RETURN ON A LEVERAGED LEASE
MPM attribution of cash
flows
234 Chapter 7
7.6 Comparing the MPM Rate of Return with the IRR
The MPM rate of return is widely used in the leveraged-leasing industry.
How does it compare with the IRR?
•
In general, the MPM rate of return is less than or equal to the IRR.
The two will be equal if all the lease’s cash fl ows are positive. Otherwise
the MPM rate of return is less than IRR.
•
If the MPM rate of return is less than the IRR, then at some point the
IRR will attribute negative income to the lease, whereas the MPM will
attribute zero income to the lease.
Graphically, for the specifi c example of this chapter, we have
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
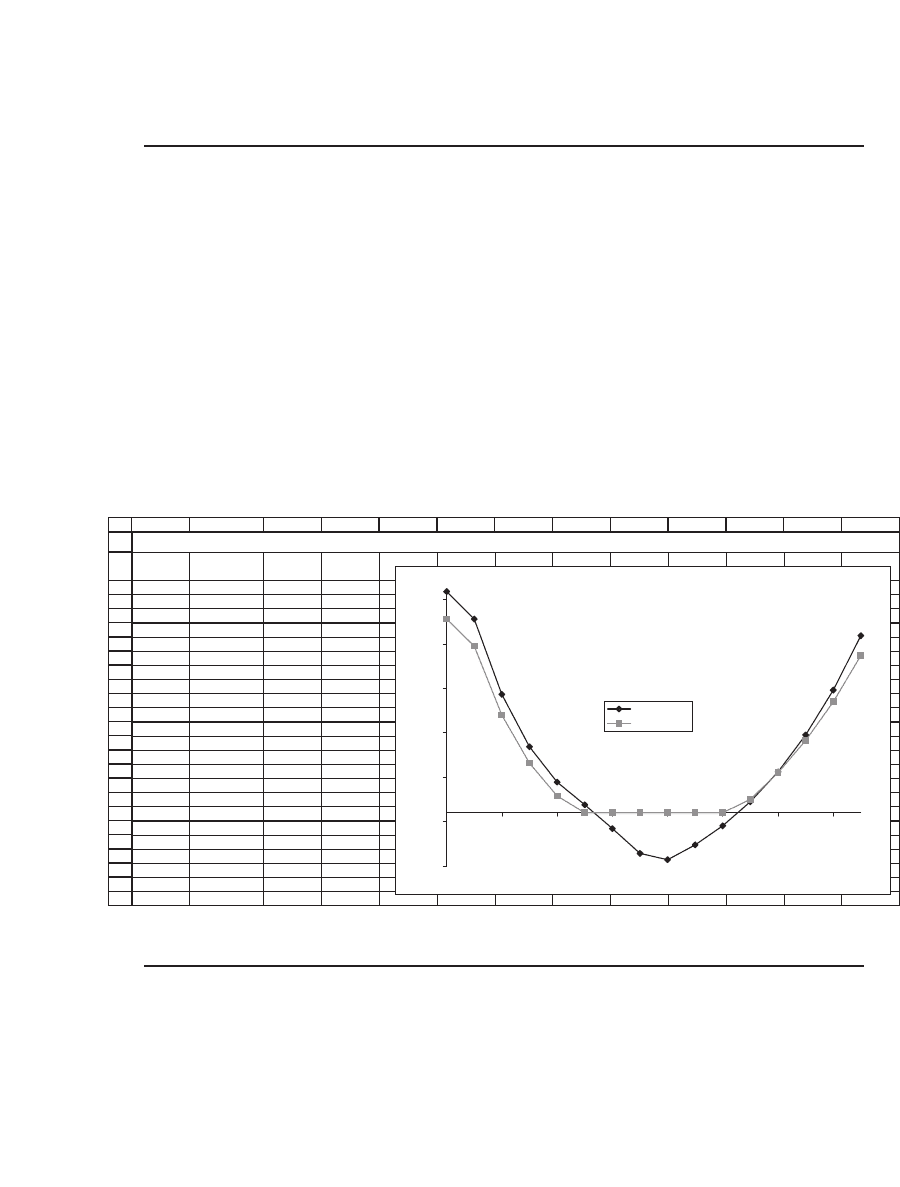
ABCDEFGHIJKLM
Year
IRR
income
MPM
income
1 24,913 21,822
2 21,796 18,754
3 13,328 11,005
4 7,431 5,587
5 3,438 1,888
68980
7 -1,774 0
8 -4,577 0
9 -5,295 0
10 -3,626 0
11 -1,480 0
12 1,230 1,514
13 4,602 4,499
14 8,753 8,123
15 13,814 12,488
16 19,938 17,708
COMPARING THE MPM INCOME WITH THE IRR INCOME
Comparing the MPM Income with the IRR Income
The IRR income is the true economic income
-6,000
-1,000
4,000
9,000
14,000
19,000
24,000
1 3 5 7 9 11 13 15
IRR income
MPM income
7.7 Summary
A leveraged lease is an arrangement whereby the lessor—the owner of
the asset—fi nances his investment with a combination of debt and equity.
In this chapter we have analyzed the equity income of the lessor in a
leveraged lease. The economic analysis of the lease cash fl ows shows that

235 The Financial Analysis of Leveraged Leases
at some point in the lease life the equity owner has negative equity value.
If properly reported, this negative equity should lead to negative account-
ing income. However, the MPM mandated by FASB 13 allows lessors to
report only positive income. We explained the MPM method and showed
how Excel can be used to compute the (otherwise meaningless) MPM
rate of return.
Exercises
1. Reconsider the leveraged-leasing example in this chapter. Show that if depreciation
is straight line over 15 years, then the MPM rate of return is equal to the IRR.
Explain.
2. In the leveraged-lease example of section 7.6, fi nd the lowest lease rental so that
the MPM is equal to the IRR (assume the original depreciation schedule).


II
Portfolio Models
Modern portfolio theory, which has its origins in the work of Harry
Markowitz, John Lintner, Jan Mossin, and William Sharpe, represents
one of the great advances in fi nance. Chapters 8–15 implement some of
the ideas of these researchers and show you how to compute the stan-
dard portfolio problems in fi nance. In these chapters we make intensive
use of Excel’s matrix functions, array functions, and data tables, which
are also discussed in Chapters 30, 31, and 34.
Chapter 8 reviews the basic mechanics of portfolio calculations. Start-
ing with price data, we calculate asset and portfolio returns. While the
bulk of the chapter deals with the simple two-asset portfolio problem,
the case of multiple assets is discussed in sections 8.4 and 8.5.
Chapter 9 discusses both the theory and the mechanics of the calcula-
tion of effi cient portfolios when there are no restrictions on short sales.
Using Excel’s matrix functions we can calculate two effi cient portfolios,
which can then be used to plot the whole effi cient frontier.
The remaining chapters of this part of the book discuss computational
and implementation issues:
•
In Chapter 10 we show how to use return data to calculate the
variance-covariance matrix. Excel’s matrix-handling capabilities make it
easy to do this calculation.
•
In Chapter 11 we discuss the computation of beta, and we replicate a
simple test of the capital asset pricing model (CAPM). We use some
market data to derive the security market line (SML). We then relate
the results to Roll’s criticism of these tests. Excel makes it easy to do the
regression analysis required for these tests. (Regressions are discussed
in Chapter 33.)
•
The preceding chapters have assumed that portfolio optimizers could
sell securities short. In Chapter 12 we show how to use Excel’s Solver to
compute effi cient portfolios when short sales are not allowed. We also
show how to integrate other portfolio constraints into the optimization
problem.
•
Chapter 13 discusses the Black-Litterman model. This widely used
model takes as its starting point the optimality of the benchmark port-
folio and uses this assumption to derive the market’s expected returns.
The optimizer can then adjust the asset allocation to account for his or
her own opinions.
