Seely F.B. Analytical Mechanics for Engineers
Подождите немного. Документ загружается.


CENTROIDS
161
In
a similar
way
the
moment
of a
volume, mass,
area,
or line
with
respect
to
an
axis or
plane
(made up
of the sum of the
moments
of
the
several
parts
or
elements
of the
volume, mass, area,
or
line)
may
be
expressed
as the
product
of the whole
volume,
mass, area,
or
line,
and
a
distance,
x
(or
y,
etc.),
from the
axis
or
plane
such
that
this
product equals
the
algebraic
sum of the moments of
the
elementary
parts
of the
volume, mass, area,
or line.
This
relation
is sometimes called the
principle
of moments
for
areas, volumes,
etc.,
and
it
leads to
an
equation
of the same form
as
does
the
same
principle
in the case of forces.
Thus,
for an
area
A,
an element of
which
is
denoted
by
a
or
dA,
the
equation is,
or
/<<"*>-/<
The
resulting
distance
(x
or
y,
etc.),
is
called the
centroidal
dis-
tance,
and
the
point
located
by
the centroidal
distances is
called
the
centroid
1
of
the
volume, mass, area,
or line.
The centroid of a
volume,
mass,
area,
or
line,
then,
is that
point
at which the whole
volume,
mass, area,
or
line
may
be
conceived to be concentrated
and have the same moment
with
respect
to an axis or
plane
as has the
volume,
mass,
area,
or line
when distributed
in its
natural
way.
Hence the
coordinates
(x
and
y)
of
the centroid of an
area,
A
}
and of a
line, L,
are
expressed
by
the
following
equations,
in
which
A
=
2a= I dA
and
L
=
2l=
j
dL.
1
The
term
center
of gravity
is
sometimes
used in
technical literature to
denote not
only
the
point
in a
body
through
which the
resultant
earth-pull
acts,
but also to
denote
what
is
here
denned
as
the
centroid of a
line, area,
volume,
or mass.
Thus,
the
phrase
"
center
of
gravity
"
of an
area,
or vol-
ume, etc.,
is
used instead of the
phrase
"
centroid
"
of
the
area,
or
volume,
etc.
Further,
the
term
centroid
is
sometimes
used in
a
restricted sense as
applying
only
to
geometrical
figures
(lines,
areas,
and
volumes),
in
which
case,
the
term
mass-center
or center
of
mass is
used
instead of
centroid of mass
in
con-
nection
with
physical
bodies.
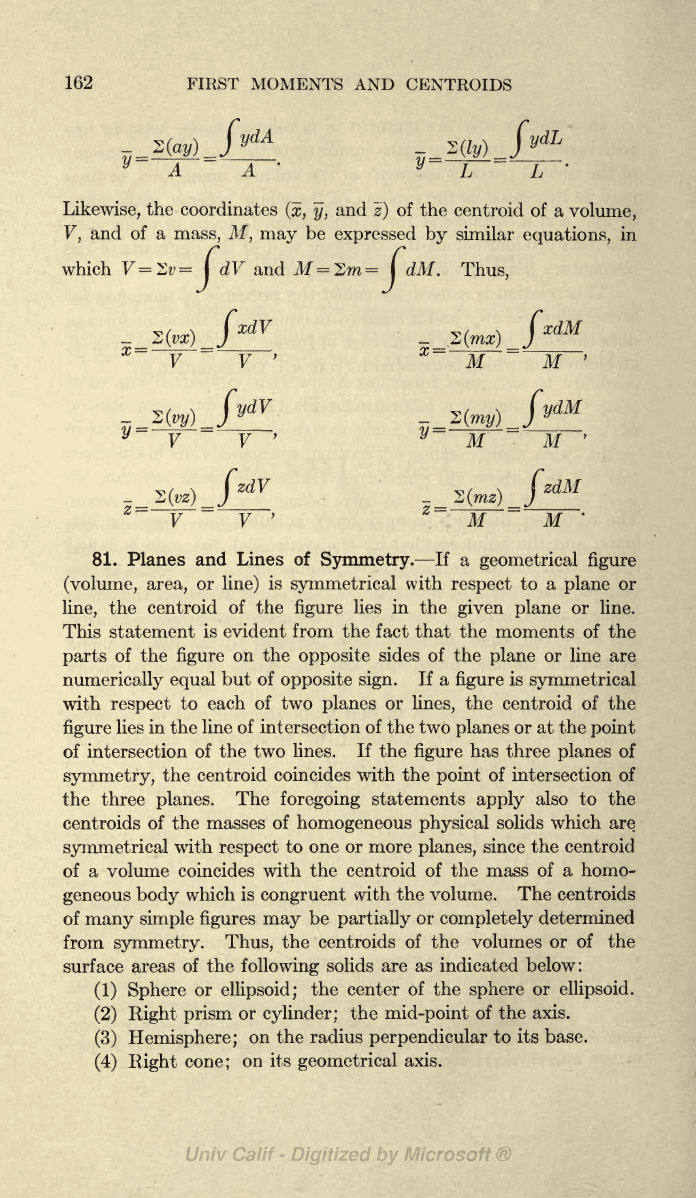
162
FIRST
MOMENTS AND
CENTROIDS
= =
_
A A
L
Likewise,
the
coordinates
(x,
y,
and
z)
of
the
centroid of a
volume,
V,
and of
a
mass, M,
may
be
expressed
by
similar
equations,
in
which V=2v=
j
dV
and
M=2m
=
I
dM.
Thus,
V
MM
=
_
=
V
V MM
MM
81. Planes
and Lines of
Symmetry.
If a
geometrical figure
(volume,
area,
or
line)
is
symmetrical
with
respect
to a
plane
or
line,
the centroid
of the
figure
lies
in
the
given plane
or
line.
This
statement
is evident from the fact that
the moments of the
parts
of
the
figure
on the
opposite
sides of the
plane
or line are
numerically
equal
but of
opposite sign.
If a
figure
is
symmetrical
with
respect
to
each of two
planes
or
lines,
the centroid of the
figure
lies in the line of
intersection of the
two
planes
or
at
the
point
of
intersection of
the two
lines.
If
the
figure
has three
planes
of
symmetry,
the centroid
coincides
with
the
point
of intersection of
the three
planes.
The
foregoing
statements
apply
also to the
centroids
of the masses
of
homogeneous
physical
solids which
are
symmetrical
with
respect
to one or more
planes,
since the
centroid
of
a
volume
coincides
with the centroid of the mass
of a homo-
geneous
body
which
is
congruent
with
the volume. The centroids
of
many
simple figures may
be
partially
or
completely
determined
from
symmetry.
Thus,
the centroids
of the
volumes
or
of
the
surface
areas
of the
following
solids are
as
indicated
below:
(1)
Sphere
or
ellipsoid;
the center of the
sphere
or
ellipsoid.
(2) Right
prism
or
cylinder;
the
mid-point
of
the axis.
(3) Hemisphere;
on
the radius
perpendicular
to
its
base.
(4)
Right
cone;
on its
geometrical
axis.

CENTROIDS
BY
INTEGRATION
163
And
the
centroids
of
the
following
areas,
and of
their
bounding
lines,
are as
indicated below:
(5)
Circle
or
ellipse;
the center
of the
circle or
ellipse.
(6)
Isosceles
triangle;
on the
median
bisecting
the
angle
between
the
equal
sides.
(7)
Semicircle;
on the radius
perpendicular
to the
base.
82. Centroids
by Integration.
In
determining
the centroid of
a
volume, mass,
area,
or line
by
the
method of
integration,
from the
equations
of Art.
80
(Vx=
I
xdV,
Ax= I
xdA,
etc.),
it is
possible
to select
the
element
of
volume, area, etc.,
in various
ways
and
to
express
the element
in terms
of either
cartesian
or
polar
coordi-
nates.
The
resulting
integral
may
be a
single,
a
double,
or a
triple
integral,
depending
on the
way
the element
is
selected. The
integral,
of
course,
is a
definite
integral,
the limits of
integration
depending
on the
boundary
curve or surface of the
figure
or
body.
In
any
case
the
element of
volume, mass,
area,
or line
must be
taken
so
that,
1. All
points
of the
element are the
same
distance from
the line
or
plane
about which moments are
taken;
otherwise,
the distance
from the
line or
plane
to the
element will be
indefinite.
Or,
so
that,
2.
The centroid
of
the
element is
known,
in
which
case
the
moment of the
element about
the moment axis or
plane
is the
product
of the element
and the
distance of its
centroid from the
axis
or
plane.
The
centroids of
some
of the common
figures
(lines,
areas,
and
volumes)
will be found
in
the
following
illustrative
problems.
ILLUSTRATIVE
PROBLEMS
Find, by
the
method of
integration,
the
centroids
of the
following figures
with
respect
to the axes
indicated.
172.
Arc
of a Circle.
The
radius
which
bisects
the arc
will
be
taken
as the x-axis
(Fig.
188).
By symmetry
the
centroid
lies on
this
axis.
Hence
y=Q.
If
r denotes
the radius
of
the
arc and
2
a
the subtended
angle,
then,
in
terms of
polar
coordinates,
the ele-
ment
of
arc, dL,
and
its distance
x from
the
7/-axis
are dL
=
rd6
and
x=r
cos
9.
Thus,
the
element of arc is
selected in
accordance
with
the first
of the
above
rules
and
x
may
be found as follows: FIG. 188.
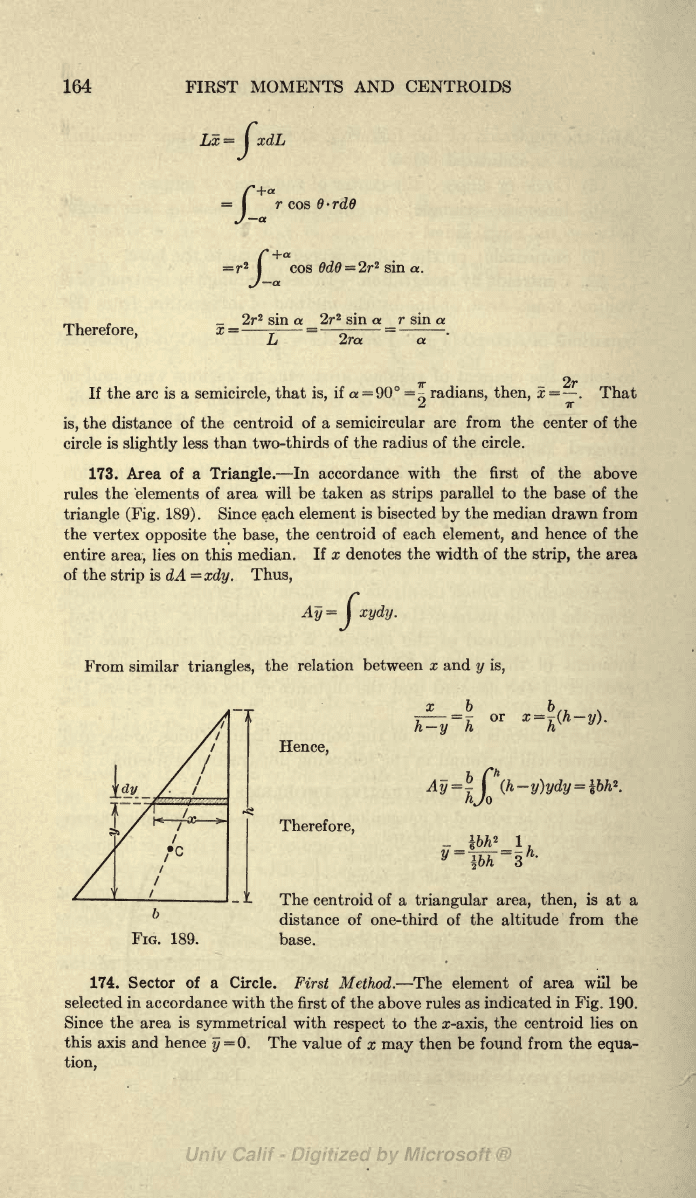
164
FIRST
MOMENTS AND
CENTROIDS
Lx
=
\xdL
-XT-
J-a
r
cos 6-rde
COS
2r
2
sin a.
Therefore,
__2r
2
sin
_2r
2
sin
_r
sin a
*~
L~
2ra
~^~~'
If the arc
is a
semicircle,
that
is,
if a
=
90
=-
radians,
then,
x=
. That
is,
the distance of the
centroid of a
semicircular arc
from
the center of the
circle
is
slightly
less than
two-thirds
of the radius of
the
circle.
173. Area
of a
Triangle.
In accordance with
the first
of
the
above
rules
the
elements
of
area will
be taken as
strips
parallel
to the base of
the
triangle (Fig.
189)
.
Since
each
element is bisected
by
the median
drawn from
the
vertex
opposite
the
base,
the
centroid of
each
element,
and
hence
of
the
entire
area,
lies on
this
median.
If x denotes the
width
of the
strip,
the area
of
the
strip
is dA
=
xdy.
Thus,
r
xydy.
From similar
triangles,
the relation
between
x and
y
is,
x
_b
_b
Hence,
/ i
A 11' 1
^
Therefore,
-
1-
The
centroid of a
triangular
area,
then,
is
at a
distance of one-third of the altitude
from
the
FIG. 189.
base.
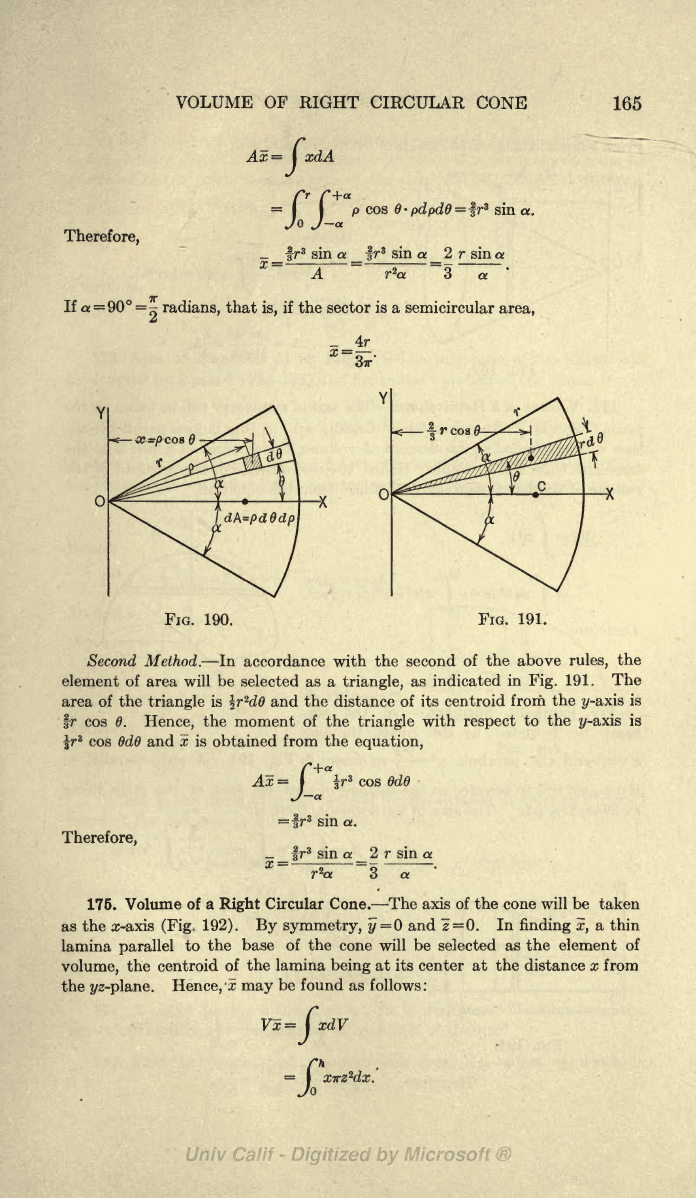
174.
Sector
of
a
Circle.
First Method.
The element
of area will be
selected in
accordance with the
first of the above rules as
indicated
in
Fig.
190.
Since the area
is
symmetrical
with
respect
to
the
z-axis,
the centroid lies on
this axis and hence
?/
=
0. The
value
of
x
may
then be found from
the
equa-
tion,
VOLUME
OF
RIGHT
CIRCULAR
CONE
165
=
C
Ax= xdA
Therefore,
+
p
cos
6-pdpdd
=
%r
s
sin
a.
sin
a
fr
3
sin
a.
2
r
sin a
A
r*a
3
a
If a;
=
90
=
2
radians,
that
is,
if
the
sector
is
a
semicircular
area,
.
4r
FIG. 190.
FIG. 191.
Second Method.
In
accordance with
the second
of
the above
rules,
the
element
of area
will
be
selected
as a
triangle,
as
indicated
in
Fig.
191.
The
area of
the
triangle
is
%r
2
d9
and the distance of its
centroid
from the
i/-axis
is
|r
cos
6.
Hence,
the moment of the
triangle
with
respect
to
the
?/-axis
is
r
3
cos ede
and
x
is
obtained
from the
equation,
/*+
Ax
=
I
f
r
3
cos
6dd
J-<*
=
|r
3
sin
.
Therefore,
__|r
3
sin
a_2
r
sin
a
r
2
*
~3~oT~'
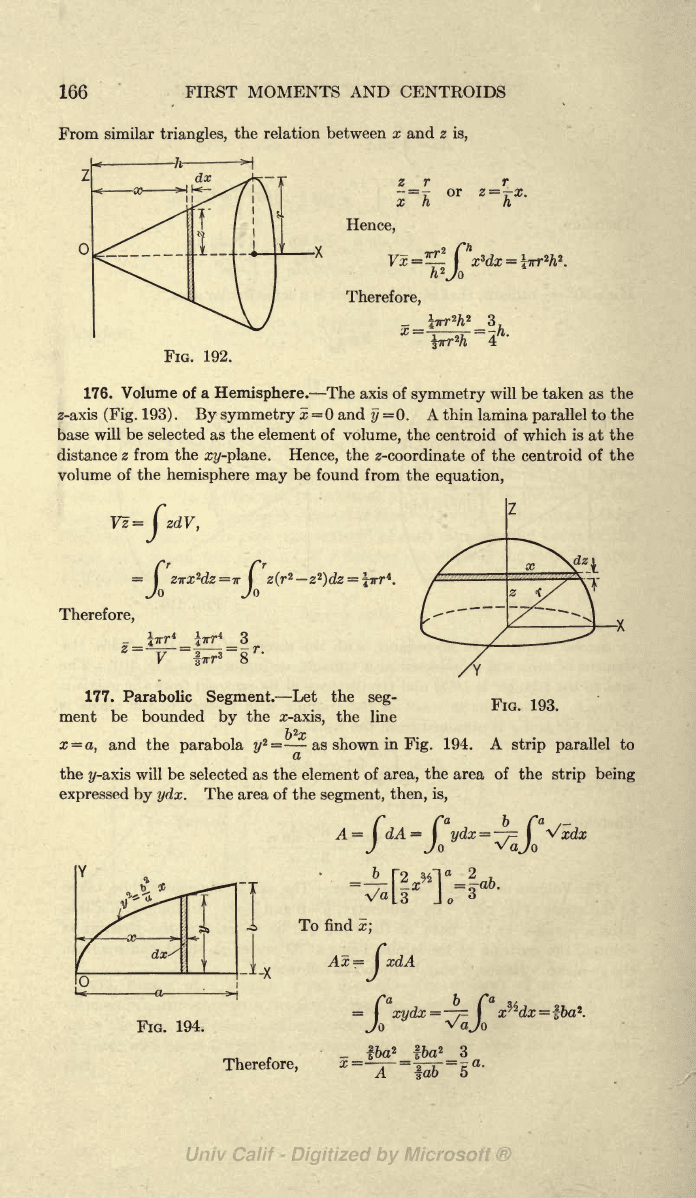
175.
Volume
of a
Right
Circular
Cone.
The
axis
of the
cone
will be taken
as
the z-axis
(Fig.
192).
By
symmetry,
i/=0
and
0=0.
In
finding x,
a
thin
lamina
parallel
to
the
base of the
cone will be
selected as the
element
of
volume,
the
centroid
of
the
lamina
being
at its center at the distance x from
the
?/z-plane.
Hence,
-x
may
be found
as
follows:
Vx=
CxdV
/
-f
"Jo
166
FIRST MOMENTS
AND
CENTROIDS
From
similar
triangles,
the
relation
between
x
and
z
is,
z
r r
-=r
or z
=
T
x.
x h
h
Hence,
.=!
r
h*Jo
Vx=^
Therefore,
FIG. 192.
176.
Volume of a
Hemisphere.
The
axis of
symmetry
will be taken as
the
z-axis
(Fig.
193).
By symmetry
x
=0
and
y
=
0. A thin
lamina
parallel
to
the
base will
be selected
as
the
element of
volume,
the
centroid of
which
is
at
the
distance
z from
the
xy-pl&ue.
Hence,
the
z-coordinate of
the
centroid
of
the
volume
of
the
hemisphere
may
be
found from
the
equation,
zdV,
/
:
I
zirx
2
dzTr I
z(r
2
i
Jo
Jo
Therefore,
z=-
'8
FIG. 193.
177. Parabolic
Segment.
Let the
seg-
ment be bounded
by
the
x-axis,
the
line
b
z
x
x
=
a,
and the
parabola
?/
2
=
as shown
in
Fig.
194. A
strip
parallel
to
the
?/-axis
will
be
selected as the element
of
area,
the
area
of
the
strip
being
expressed
by
ydx.
The area of the
segment,
then,
is,
A=
CdA=
C
a
ydx
=
-^=
("Vxdx
J
Jo
Vajo
To find
x\
Ax=
CxdA
FIG.
194.
Therefore,
x
=
-
1
1
=
-_|6a
2
fba
2
_3
X
~
A
AREA OF
QUADRANT
OF
AN
ELLIPSE
167
To
find
</,
the
same
elementary strip
will
be
selected,
but
since
each
point
of the element is not
the
same distance
from
the
z-axis,
its
moment
must be
expressed
as
the
product
of the area of
the
strip
and its
centroidal
distance,
K,
from the
x-axis.
Thus,
Therefore,
-K
3,
|ab
8
'
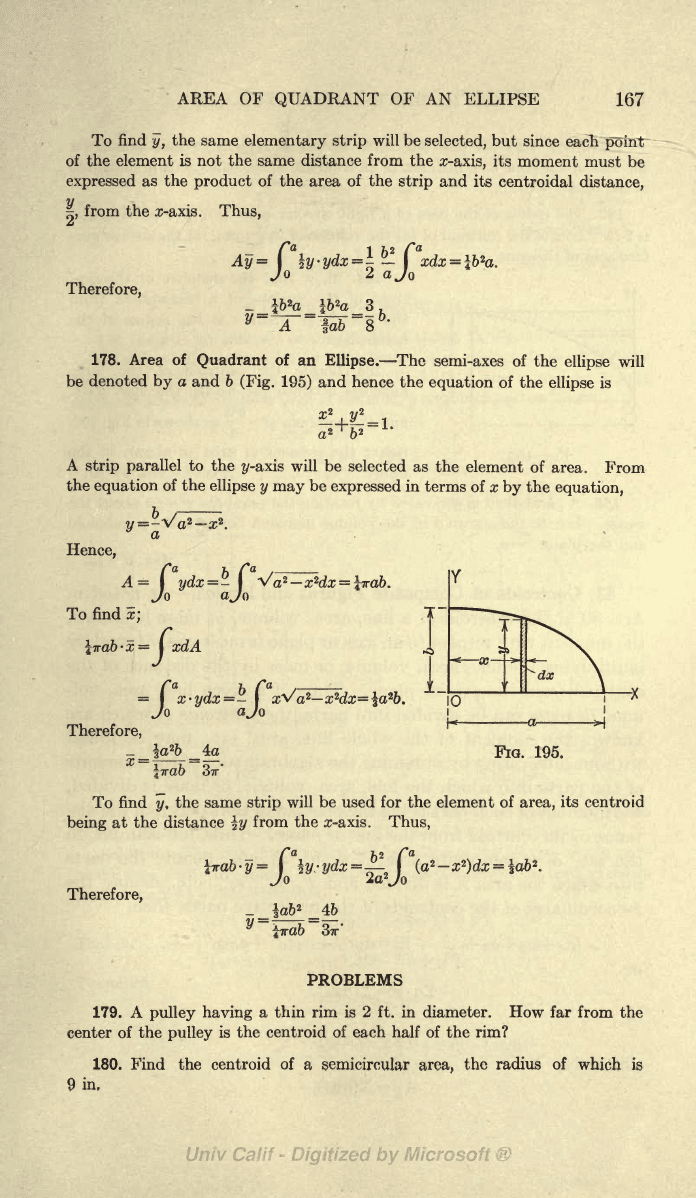
178. Area
of
Quadrant
of an
Ellipse.
The
semi-axes
of the
ellipse
will
be denoted
by
a
and
6
(Fig.
195)
and hence the
equation
of the
ellipse
is
A
strip parallel
to the
y-axis
will be selected
as the
element of
area.
From
the
equation
of
the
ellipse
y may
be
expressed
in
terms
of x
by
the
equation,
a
Hence,
FIG.
195.
\irab
To find
y,
the same
strip
will
be used
for
the
element of
area,
its
centroid
being
at the distance
\y
from the
z-axis.
Thus,
Therefore,
-
Fto-ite-*
(\a*-
Jo
2oJo
PROBLEMS
179.
A
pulley
having
a
thin rim
is
2
ft.
in
diameter. How far
from
the
center
of the
pulley
is
the centroid
of each
half of the
rim?
180.
Find the
centroid
of
a
semicircular
area,
the
radius
of
which
is
9
in,
168
FIRST
MOMENTS
AND
CENTROIDS
181.
Show that the centroid of the
surface of a
right
circular
cone
is
on
the axis of the
cone at a
distance of
|/i
from the
apex,
where
h
is
the
altitude
of the
cone.
182.
The
radius
of the base
of
a
right
circular
cone is 5
in.
and its
height
is
2
ft. Locate
the centroid
of
(a)
the
volume of the
cone;
(6)
the
curved sur-
face
area
of the cone.
183.
Show that the
distance of
the
cen
troid of the
surface of a
hemisphere
from
the
base
is
\r
where
r
is the
radius of
the
hemisphere.
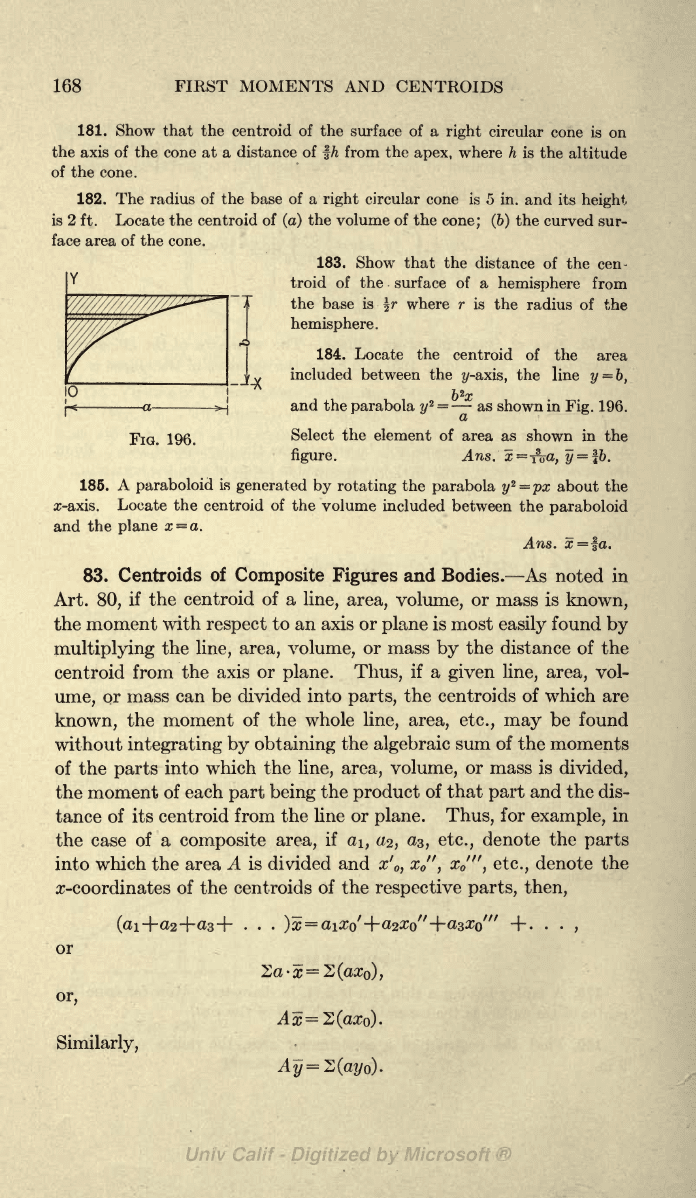
184.
Locate
the
centroid
of the
area
included between the
y-axis,
the
line
y
=
b,
b
2
x
and
the
parabola
y
2
=
as
shown
in
Fig.
196.
FIG. 196.
Select the
element
of area as
shown
in the
figure.
Ans.
x=Tua,y
=
%b.
186.
A
paraboloid
is
generated
by
rotating
the
parabola
y*=px
about the
x-axis.
Locate the
centroid
of
the
volume
included between
the
paraboloid
and
the
plane
x
=
a.
Ans.
x=|a.
83.
Centroids
of
Composite
Figures
and Bodies.
As
noted
in
Art.
80,
if
the
centroid
of
a
line,
area, volume,
or mass
is
known,
the
moment with
respect
to an axis or
plane
is
most
easily
found
by
multiplying
the
line,
area, volume,
or mass
by
the
distance of the
centroid
from the
axis or
plane.
Thus,
if
a
given
line,
area,
vol-
ume,
or
mass
can be
divided
into
parts,
the centroids of which are
known,
the
moment of the whole
line, area, etc., may
be found
without
integrating by
obtaining
the
algebraic
sum of the
moments
of the
parts
into which
the
line,
area,
volume,
or mass is
divided,
the
moment
of
each
part
being
the
product
of that
part
and the dis-
tance of its
centroid
from the line
or
plane.
Thus,
for
example,
in
the case
of a
composite
area,
if
a\,
0,2, as, etc.,
denote the
parts
into which
the area
A is divided and
x'
,
x
",
x
f
",
etc.,
denote the
^-coordinates of the
centroids of the
respective
parts,
then,
(0,1+0,2+0,3
+
)x
=
aixo
f
+a
2
xo"+0,3X0"
+
.
. .
,
or
or,
Similarly,
CENTROIDS OF COMPOSITE
FIGURES 169
From
similar
equations
the
centroid of a
composite
line,
volume,
or
mass
may
be found.
Thus,
Ly=2(ly
),
ILLUSTRATIVE
PROBLEMS
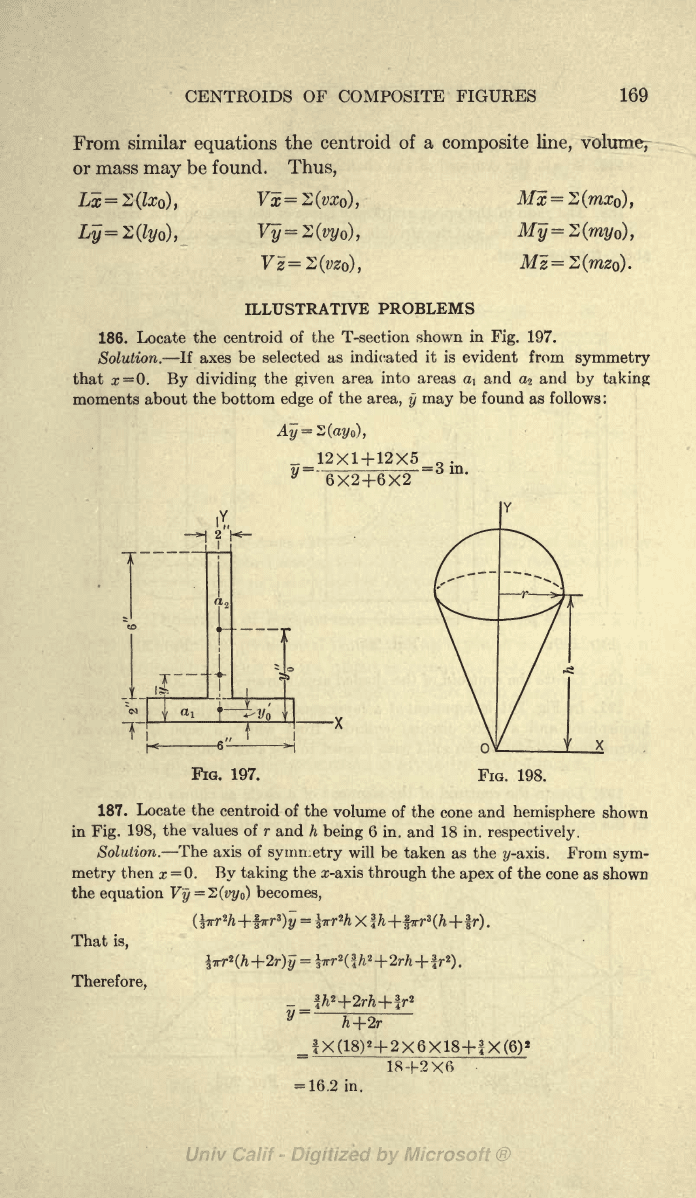
186. Locate
the
centroid
of the T-section
shown
in
Fig.
197.
Solution.
If
axes
be
selected as indicated
it
is evident from
symmetry
that
z=0.
By
dividing
the
given
area
into
areas
a\
and a
2
and
by taking
moments about
the bottom
edge
of
the
area,
y
may
be found
as follows:
=
3
in.
__12X1+12X5
^"^
6X2+6X2
FIG.
197.
FIG. 198.
187.
Locate
the
centroid of
the
volume of the
cone and
hemisphere
shown
in
Fig.
198,
the
values of r
and h
being
6
in. and 18
in.
respectively.
Solution.
The
axis
of
symmetry
will
be taken
as
the
?/-axis.
From
sym-
metry
then
x
=
0.
By taking
the x-axis
through
the
apex
of the
cone
as shown
the
equation
Vy
=
H(vyo)
becomes,
That
is,
Therefore,
h+2r
X(18)
2
+2X6Xl8+|X(6)
18-1-2X6
16.2
in.
170
FIRST MOMENTS
AND
CENTROIDS
PROBLEMS
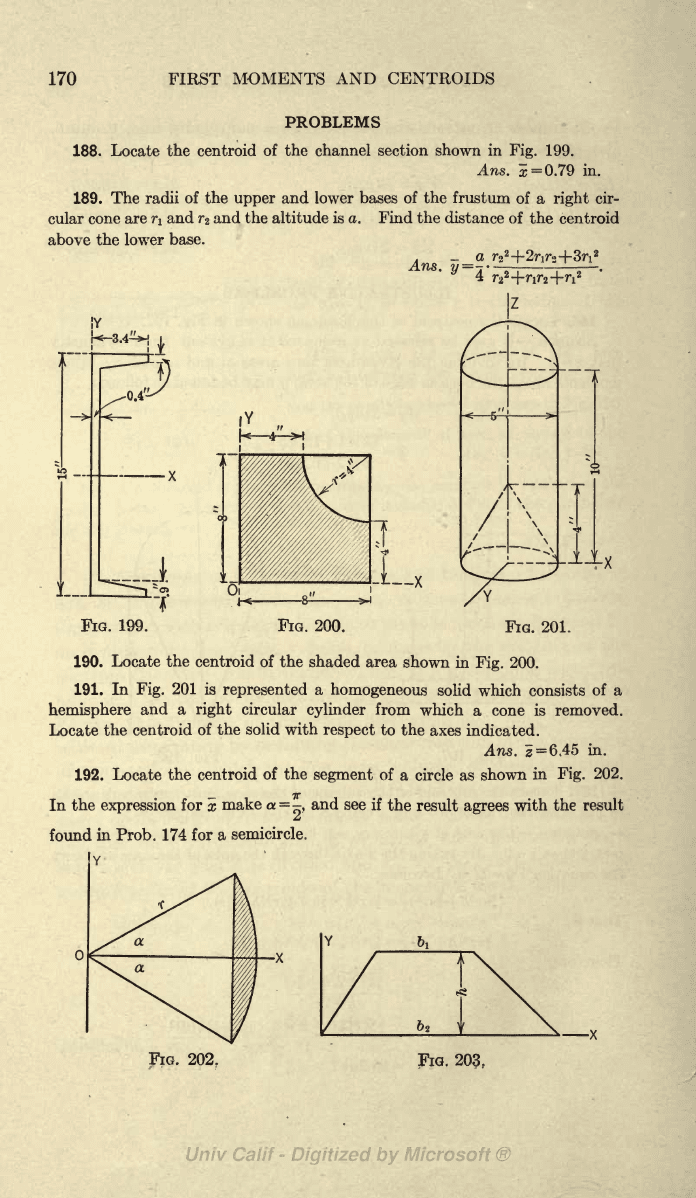
188. Locate
the centroid
of the channel section
shown in
Fig.
199.
Ans.
5=0.79
in.
189. The
radii
of
the
upper
and lower
bases of
the
frustum
of
a
right
cir-
cular
cone
are
n
and
r
2
and the
altitude is a. Find
the
distance of
the
centroid
above
the lower
base.
_
a
r
2
2
+2rir
2
+3r
1
2
FIG.
199.
FIG.
200.
FIG. 201.
190.
Locate
the
centroid
of
the
shaded
area
shown in
Fig.
200.
191. In
Fig.
201 is
represented
a
homogeneous
solid
which consists
of a
hemisphere
and a
right
circular
cylinder
from
which
a cone
is removed.
Locate
the
centroid
of the
solid
with
respect
to
the
axes
indicated.
Ans.
i
=
6,45
in.
192. Locate
the
centroid
of the
segment
of
a circle as
shown
in
Fig.
202.
In the
expression
for
x
make
=-,
and
see if
the result
agrees
with
the
result
z
found
in Prob.
174
for
a
semicircle.
FIG.
202.
FIG.
203,
