Seely F.B. Analytical Mechanics for Engineers
Подождите немного. Документ загружается.

CONICAL
PIVOT
151
the
frictional
moment
for a
solid
flat
pivot and,
hence,
will
not
be
given
in
detail.
The
fFictional
moment
is
found
to
be
:
For
uniform
pressure,
-
o
For
uniform
wear,
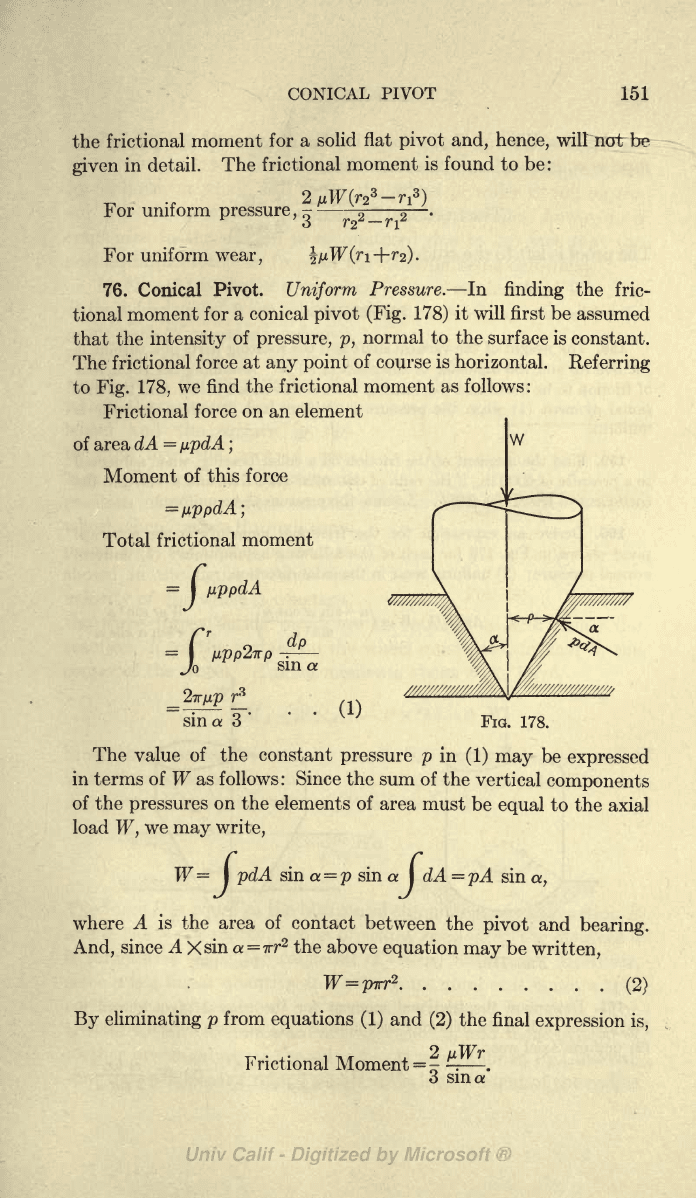
76. Conical
Pivot.
Uniform
Pressure. In
finding
the
fric-
tional
moment
for
a conical
pivot
(Fig.
178)
it will first
be
assumed
that the
intensity
of
pressure,
p,
normal to the
surface is
constant.
The
frictional
force at
any
point
of
course is
horizontal.
Referring
to
Fig.
178,
we
find
the frictional moment as follows:
Frictional
force
on
an element
of area
dA
=
fipdA
;
Moment
of this
force
Total
frictional
moment
dp
sn a
_
2-n-fjip
r*_
sin a
3
'
(1)
FIG.
178.
The
value of
the constant
pressure
p
in
(1)
may
be
expressed
in
terms of W as
follows : Since the
sum
of the
vertical
components
of
the
pressures
on
the
elements of
area
must
be
equal
to
the axial
load
W,
we
may write,
W
=
I
pdA
sin a
=
p
sin a I
dA=pA
sin
a,
where
A
is the area
of
contact
between
the
pivot
and
bearing.
And,
since
A
Xsma
=
irr
2
the
above
equation
may
be
written,
(2)
By eliminating p
from
equations (1)
and
(2)
the final
expression
is,
Frictional
Moment
=
-
-.
-.
3
sin a
152
FRICTION
Uniform
Wear.
If
the wear
in
the
direction
of
the axis
of
the
pivot
is constant it can
be shown
that,
The
f
fictional moment
=
-
M
The
proof
is left to the student.
2
sin a
PROBLEMS
158.
The
weight
of
the vertical shaft and the
rotating
parts
of
a
turbine
is
100,000
Ib. and
the diameter of
the
shaft is
10
in.
Assuming
the
coefficient
of friction to be 0.015
and the
bearing
to be a
flat-ended
pivot,
find the
fric-
tional moment
(1)
when
the
pressure
is uniform
and
(2)
when the
wear is
uniform.
159. Find
the moment of the
friction on a collar
bearing,
when
subjected
to a
pressure
of 6000
Ib.,
if the radii
of the collar are
3.5 in. and
4.5 in. and
the
coefficient
of
friction
is
0.025.
Assume the
pressure
to be
uniform.
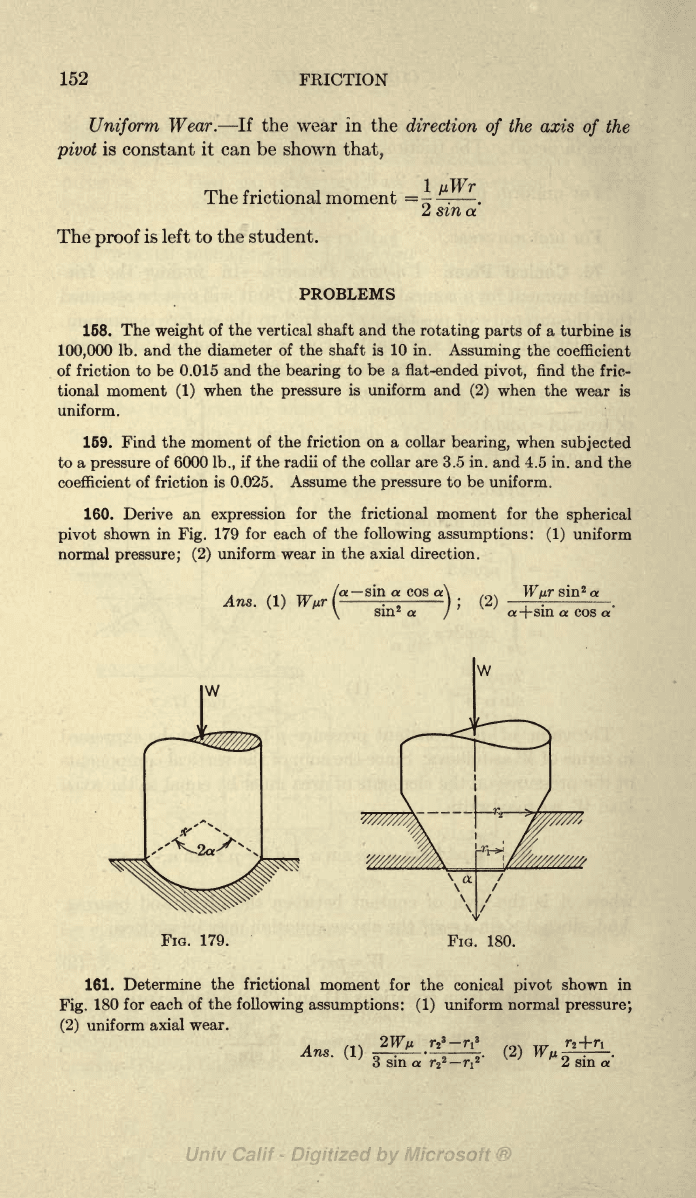
160.
Derive
an
expression
for the frictional moment for
the
spherical
pivot
shown
in
Fig.
179
for each
of the
following assumptions: (1)
uniform
normal
pressure;
(2)
uniform
wear
in the
axial
direction.
Ans.
(1)
Sin a cos a
sin
2
a
(2)
Wur
sin
2
a.
a+sin
a
cos a'
W
FIG.
179.
FIG.
180.
161.
Determine
the frictional
moment for the conical
pivot
shown in
Fig.
180 for
each
of the
following
assumptions:
(1)
uniform normal
pressure;
(2)
uniform axial
wear.
Ans.
(1)
3 sin
2
sin
a'
ROLLING RESISTANCE
153
77.
Rolling
Resistance.
If
a
rigid
wheel
or
roller
which
car-
ries
a vertical
load rests on
a
rigid
horizontal
surface,
a
horizontal
force,
however
small,
will cause
the wheel
or roller to
roll
on the
surface.
If a wheel rolls over
a
yielding
surface,
however,
a
resistance
to the motion is
encountered
due to the fact that the
surface
immediately
in
front of
the
wheel
is
being
deformed.
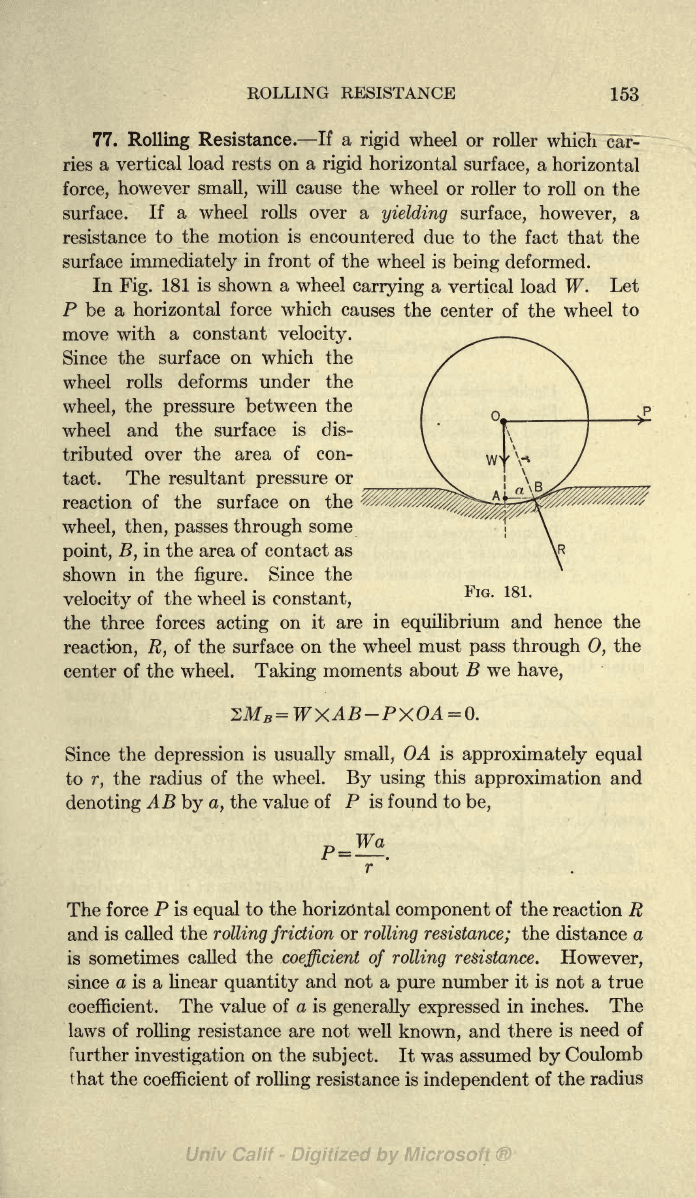
In
Fig.
181
is
shown
a
wheel
carrying
a
vertical load
W.
Let
P
be a
horizontal
force which
causes
the
center
of
the
wheel
to
move with
a
constant
velocity.
Since
the surface
on
which
the
wheel
rolls
deforms
under
the
wheel,
the
pressure
between
the
wheel and the
surface
is
dis-
tributed
over the
area
of con-
tact. The
resultant
pressure
or
reaction
of the
surface on the
wheel,
then, passes through
some
point,
B,
in
the area of contact
as
shown
in the
figure.
Since
the
velocity
of the wheel
is
constant,
the three
forces
acting
on
it are
in
equilibrium
and
hence
the
reaction, R,
of the
surface on
the wheel
must
pass through 0,
the
center of the
wheel.
Taking
moments
about
B
we
have,
Since
the
depression
is
usually
small,
OA is
approximately equal
to
r,
the
radius of the wheel.
By
using
this
approximation
and
denoting
AB
by
a,
the value
of P is
found
to
be,
The
force
P is
equal
to the horizontal
component
of the
reaction
R
and
is called
the
rolling
friction
or
rolling
resistance;
the distance a
is
sometimes
called
the
coefficient
of rolling
resistance.
However,
since
a is a linear
quantity
and
not a
pure
number
it is
not a true
coefficient.
The value of
a
is
generally expressed
in inches.
The
laws
of
rolling
resistance are not
well
known,
and
there
is
need
of
further
investigation
on the
subject.
It was
assumed
by
Coulomb
1
hat
the
coefficient of
rolling
resistance is
independent
of
the
radius
154
FRICTION
of the wheel. Tests
by Dupuit
indicate
that the
coefficient
varies
as the
square
root of
the diameter.
Whether
the
conclusion
of
the
latter
is correct or
not,
it
seems reasonable
to
assume
that
the
value of
the
coefficient
depends
on the
diameter of
the
wheel.
The
values of
the coefficient of
rolling
resistance
given
by
various
investigators
are not
in
close
agreement
and
should
be
used
with
caution.
COEFFICIENTS
OF
ROLLING
RESISTANCE
(Due
to
Coulomb
and
Goodman}
a
(inches)
Lignum
vitse
on oak
0.0195
Elm
on
oak
0327
Steel
on
steel.. 007 to
0.015
Steel
on
wood
06 to
.10
Steel
on macadam
road
05
.20
Steel
on soft
ground
.
.
3.0 to
5 .
Pneumatic
tires
on
good
road . 02
to . 022
Pneumatic
tires
on
mud
road 04
to .06
Solid
rubber tire
on
good
road 04
Solid
rubber tire
on
mud
road
09 to .11
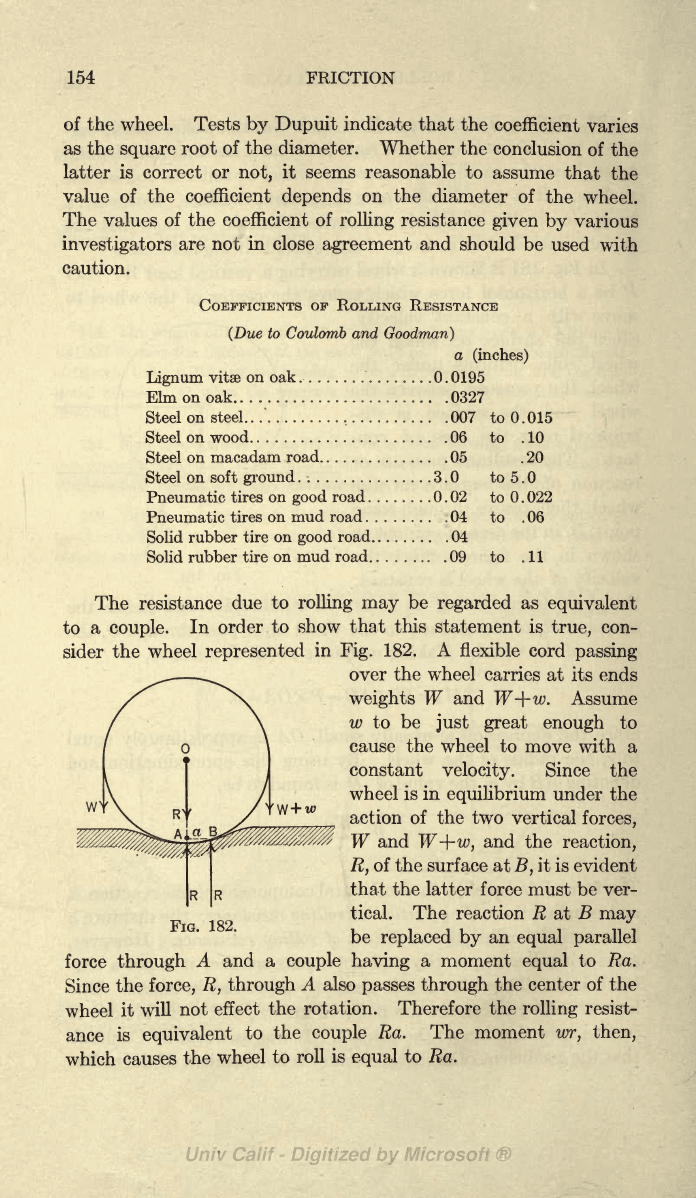
The
resistance
due
to
rolling may
be
regarded
as
equivalent
to
a
couple.
In order
to show that
this
statement is
true,
con-
sider
the wheel
represented
in
Fig.
182.
A
flexible cord
passing
over
the wheel
carries
at its ends
weights
W
and
TF+w.
Assume
w
to
be
just
great
enough
to
cause the
wheel to
move
with
a
constant
velocity.
Since the
wheel
is in
equilibrium
under the
action of the
two
vertical
forces,
W
and
W-\-w,
and
the
reaction,
R,
of the surface
at
B,
it is evident
that the latter force
must
be
ver-
tical.
The reaction
R
at
B
may
be
replaced
by
an
equal
parallel
force
through
A
and
a
couple
having
a moment
equal
to Ra.
Since
the
force, R, through
A
also
passes through
the
center of the
wheel
it will
not
effect
the
rotation.
Therefore
the
rolling
resist-
ance
is
equivalent
to
the
couple
Ra.
The moment
wr, then,
which
causes
the
wheel
to
roll
is
equal
to Ra.
FIG.
182.
BELT FRICTION
155
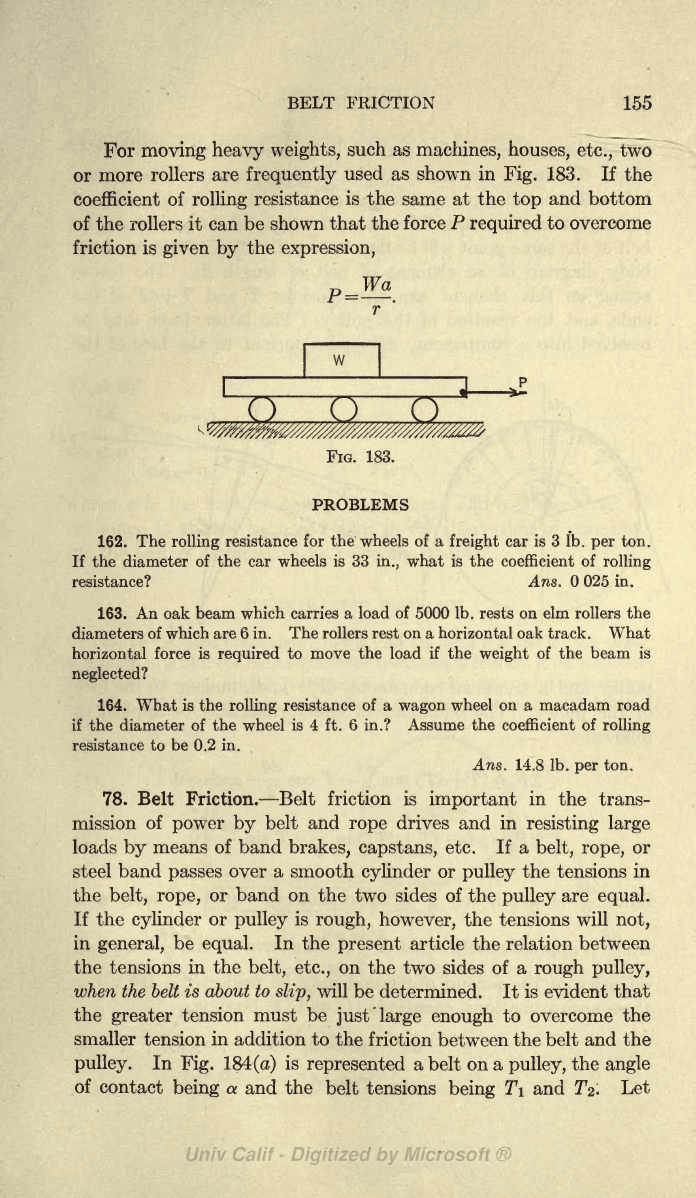
For
moving heavy
weights,
such as
machines, houses,
etc.,
two
or
more
rollers
are
frequently
used as shown
in
Fig.
183.
If the
coefficient
of
rolling
resistance
is
the same at the
top
and
bottom
of the rollers it can
be shown
that the
force
P
required
to overcome
friction is
given
by
the
expression,
p
=
Wa
r
w
() O
0_
'3%%%%^^
FIG.
183.
PROBLEMS
162.
The
rolling
resistance
for the
wheels
of a
freight
car is
3
Ib.
per
ton.
If
the diameter
of the
car wheels
is 33
in.,
what is the coefficient of
rolling
resistance?
Ans. 025
in.
163.
An
oak
beam
which
carries
a load of
5000 Ib. rests on
elm
rollers
the
diameters of which are
6 in.
The
rollers rest
on
a horizontal oak track. What
horizontal force is
required
to move the load
if
the
weight
of the beam is
neglected?
164.
What is
the
rolling
resistance
of
a
wagon
wheel
on a
macadam
road
if the diameter
of the
wheel
is 4 ft. 6 in.?
Assume the
coefficient of
rolling
resistance to
be
0.2 in.
Ans.
14.8 Ib.
per
ton.
78.
Belt Friction. Belt friction is
important
in
the
trans-
mission
of
power
by
belt
and
rope
drives and
in
resisting
large
loads
by
means of band
brakes,
capstans,
etc.
If
a
belt,
rope,
or
steel
band
passes
over
a
smooth
cylinder
or
pulley
the tensions
in
the
belt, rope,
or band
on the
two sides of
the
pulley
are
equal.
If the
cylinder
or
pulley
is
rough,
however,
the tensions will
not,
in
general,
be
equal.
In the
present
article
the
relation between
the tensions
in the
belt, etc.,
on
the
two sides of a
rough
pulley,
when
the belt is about
to
slip,
will be
determined. It
is evident
that
the
greater
tension must be
just
large
enough
to overcome
the
smaller
tension
in addition
to the
friction
between
the
belt and
the
pulley.
In
Fig.
184
(a)
is
represented
a belt on a
pulley,
the
angle
of
contact
being
a
and
the
belt
tensions
being
T\
and
T%.
Let
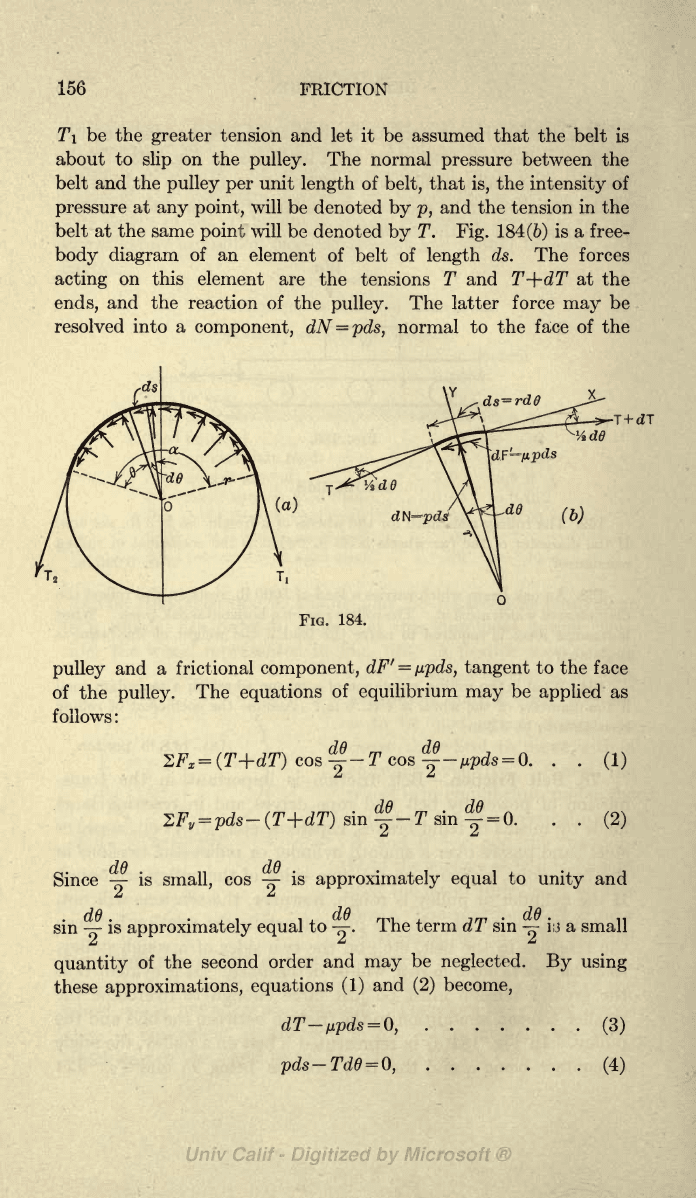
156
FRICTION
TI
be the
greater
tension
and let it be assumed that
the belt
is
about to
slip
on the
pulley.
The normal
pressure
between
the
belt and the
pulley per
unit
length
of
belt,
that
is,
the
intensity
of
pressure
at
any point,
will
be
denoted
by p,
and
the
tension
in
the
belt at the same
point
will be
denoted
by
T.
Fig.
184(6)
is a free-
body diagram
of
an
element
of
belt of
length
ds. The forces
acting
on this
element are
the tensions
T and
T-\-dT
at
the
ends,
and
the reaction of
the
pulley.
The latter force
may
be
resolved into a
component,
dN
=
pds,
normal
to the
face
of
the
FIG.
184.
pulley
and a
frictional
component,
dF
f
=
upds,
tangent
to
the
face
of
the
pulley.
The
equations
of
equilibrium may
be
applied
as
follows :
(1)
=
pds-(T+dT)
sin
-T sin
=
0.
(2)
Since
-jr-
is
small,
cos
-^-
is
approximately equal
to
unity
and
2
sin
is
approximately
equal
to
-^r.
The term
dT
sin
-^
iu a small
2
A
A
quantity
of
the
second
order
and
may
be
neglected.
By
using
these
approximations,
equations
(1)
and
(2)
become,
pds-TdB
=
(3)
(4)
BELT
FRICTION
157
Eliminating pds
from
equations (3)
and
(4)
we
have,
dT
T
(5)
By
integrating
equation
(5)
the relation
between
T\
and
T%
may
be
found as follows
:
That
is,
T
Ti
T~2
r
Jo
M
'
or.
(6)
where
e
is
the
base of
natural
logarithms
and a is
measured
in
radians.
It
should be
noted
that in
the
derivation
of
equation
(6)
the belt
is
assumed to be
perfectly
flexible.
ILLUSTRATIVE PROBLEM
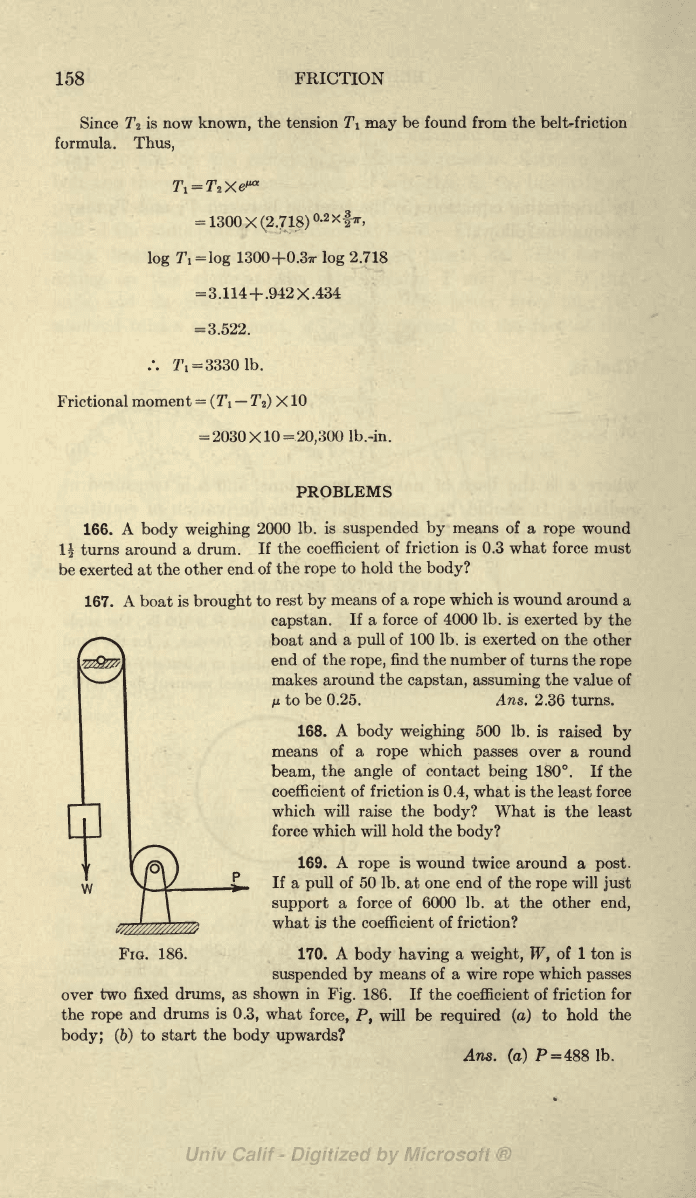
165.
In
the
band
brake
shown in
Fig.
185 the force
P
is
100
lb.,
the
angle
of
contact, a,
is 270
(|TT
radians),
and
the coefficient
of
friction, ju,
for
the band
and
the brake
wheel
is
0.2.
If
the
brake wheel
rotates
in a
counter-clockwise
direction
find
the
tensions
in the band and the
frictional
moment
developed.
FIG.
185.
Solution.
Since
the
operating
lever
ACB is in
equilibrium
the
equation
2M
B
=
may
be
applied,
from
which the band
pull
at
C,
that
is,
the tension
T
2
,
is
found.
Thus,
13001b.
158
FRICTION
Since
Tz
is now
known,
the
tension
T\
may
be found from the belt-friction
formula.
Thus,
=
1300X(2.718)-
2x
f
7r
>
log
Ti
=
log
1300+0.3*-
log
2.718
=
3.114
+
.942X.434
=
3.522.
/.
Ti
=
33301b.
Frictional
moment
=
(T
l
-TJX
10
=
2030X10
=
20,300
Ib.-in.
PROBLEMS
166.
A
body weighing
2000
Ib.
is
suspended by
means
of
a
rope
wound
1
turns around
a drum.
If the
coefficient
of
friction is
0.3
what
force
must
be
exerted at
the
other
end
of
the
rope
to hold the
body?
167.
A
boat is
brought
to
rest
by
means
of a
rope
which
is
wound around
a
capstan.
If a force of 4000 Ib.
is
exerted
by
the
boat
and
a
pull
of 100
Ib.
is exerted on
the
other
end
of the
rope,
find the
number of turns
the
rope
makes around
the
capstan, assuming
the value of
ju
to
be
0.25.
Ans.
2.36
turns.
168.
A
body
weighing
500
Ib. is raised
by
means
of a
rope
which
passes
over
a round
beam,
the
angle
of
contact
being
180.
If the
coefficient of friction
is
0.4,
what
is
the
least
force
which will
raise the
body?
What is
the
least
force
which will
hold the
body?
w
FIG.
186.
169.
A
rope
is
wound twice
around a
post.
If
a
pull
of 50 Ib. at
one end
of
the
rope
will
just
support
a force of 6000 Ib. at the other
end,
what
is
the
coefficient
of
friction?
170. A
body having
a
weight,
W,
of
1
ton
is
suspended by
means
of
a
wire
rope
which
passes
over
two
fixed
drums,
as
shown
in
Fig.
186.
If the
coefficient
of friction
for
the
rope
and
drums is
0.3,
what
force,
P,
will
be
required
(a)
to hold
the
body; (6)
to start the
body upwards?
Ans.
(a)
P
=
4881b,
BELT FRICTION
159
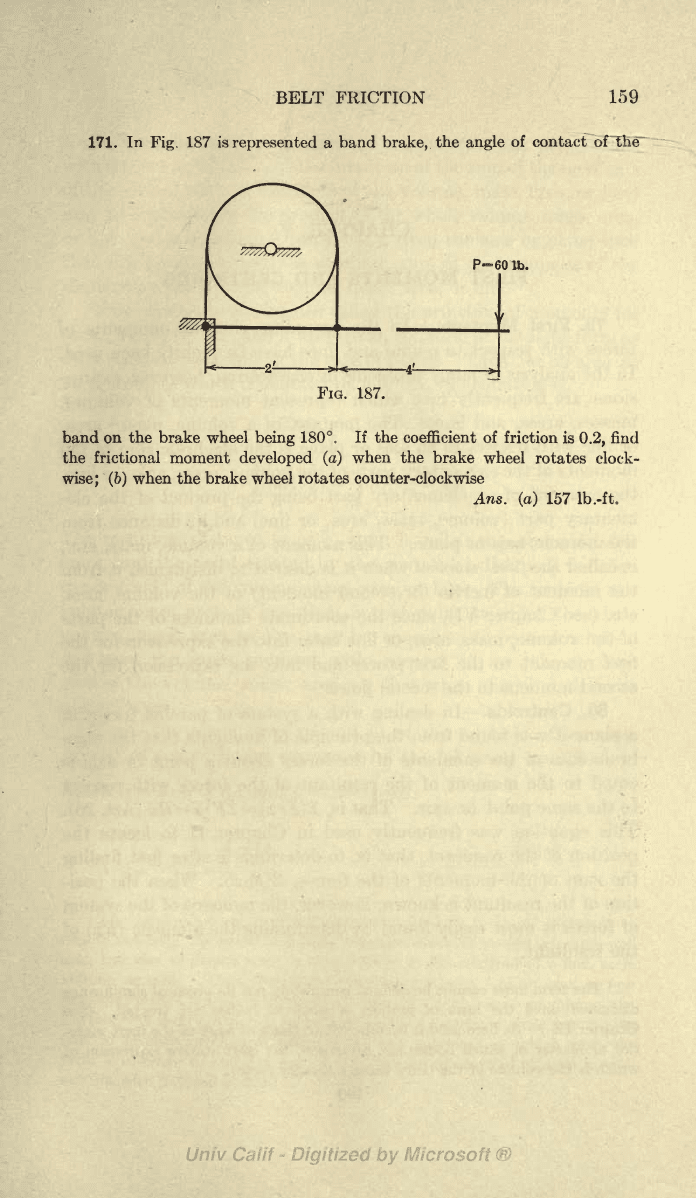
171.
In
Fig.
187 is
represented
a band
brake,
the
angle
of contact ofHie~
P-601b.
FIG. 187.
band on
the brake
wheel
being
180.
If
the coefficient of
friction
is
0.2,
find
the frictional moment
developed
(a)
when
the
brake
wheel
rotates clock-
wise; (b)
when
the brake
wheel
rotates
counter-clockwise
Ans.
(a)
157
Ib.-ft.

CHAPTER
V
FIRST MOMENTS
AND
CENTROIDS
79.
First
Moments. In
the
preceding
chapters
moments
of
forces with
respect
to
points
and lines
have
frequently
been used.
In the
analysis
of
many
problems
in
engineering, however, expres-
sions are
frequently
met which
represent
moments of
volumes,
masses, areas,
and
lines.
The moment of
a
volume, mass,
1
area,
or line with
respect
to
an
axis or
plane
is the
algebraic
sum of the
moments of the
elementary parts
of
the
volume, mass, area,
or
line,
the
moment
of
an
elementary part
being
the
product
of
the ele-
mentary part
(volume,
mass, area,
or
line)
and
its distance from
the moment axis or
plane.
This
moment of a
volume, mass, etc.,
is called the
first
moment when it is
desired to
distinguish
it
from
the moment of inertia
(or
second
moment)
of the
volume,
area,
etc.
(see
Chapter VI),
since the
coordinate
distances
of
the
parts
of
the
volume, mass, area,
or
line
enter into
the
expression
for
the
first moment
to the
first
power
and into
the
expression
for the
second moment to the second
power.
80. Centroids.
In
dealing
with a
system
of
parallel
forces
in
a
plane
it was found from the
principle
of
moments that the
alge-
braic sum of the moments
of
the forces
about a
point
or axis
is
equal
to the moment of the resultant of the forces
with
respect
to the same
point
or axis. That
is,
2(F-x)
=
2F-x
=
Rx
(Art.
26).
This
equation
was
frequently
used in
Chapter
II
to locate
the
position
of the
resultant,
that
is,
to determine
x
after first
finding
the sum of the moments of the
forces,
2(F-x).
When
the
posi-
tion of the resultant is
known, however,
the
moment of
the
system
of
forces is most
easily
found
by
determining
the moment
(Rx)
of
the
resultant.
1
The
term
mass
cannot
be denned
completely
nor
its
physical
significance
discussed
until the
laws
of
motion
of
physical
bodies are
treated.
(See
Chapter
IX.)
As
here used it is sufficient to think of mass as
the
inert
mate-
rial
or matter of which
bodies
are
composed,
the
quantitative
expression
of
which
is
the
volume
of the
body
times a
density
factor.
160
