Principles of Finance with Excel (Основы финансов c Excel)

Подождите немного. Документ загружается.

PFE, Chapter 13: The CAPM and SML page 10

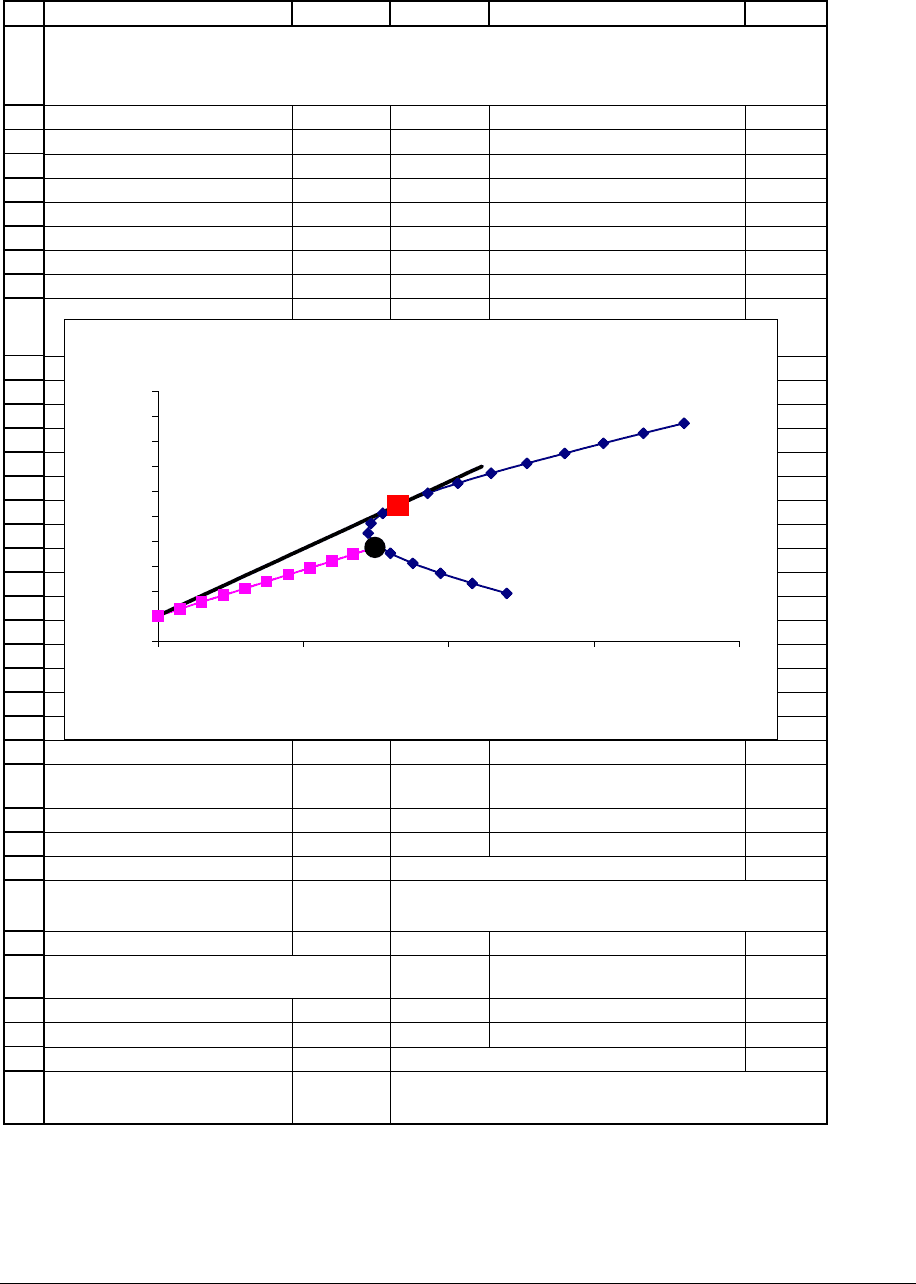
there’s always a point on the red square line which gives a higher return but has the same

portfolio standard deviation

There must be a

best line which starts off from the point 2% on the y-axis. Here it is:

PFE, Chapter 13: The CAPM and SML page 11

ABC DE

Stock A Stock B

Average return 7.00% 15.00%

Variance 0.0064 0.0196

Sigma 8.00% 14.00%

Covariance of returns 0.00

Correlation 0.10

Risk-free rate 2%

Round dot portfolio

•

A 0.9

B 0.1

Mean 7.80% <-- =B29*$B$3+(1-B29)*$C$3

Sigma 7.47%

Best red square portfolio

A 0.5181

B 0.4819

Mean 10.85% <-- =B35*$B$3+(1-B35)*$C$3

Sigma 8.26%

TWO STOCKS AND A RISK-FREE ASSET

the best red square portfolio

<-- =SQRT(B29^2*$B$4+(1-B29)^2*$C$4

+2*B29*(1-B29)*$B$6)

<-- =SQRT(B35^2*$B$4+(1-B35)^2*$C$4

+2*B35*(1-B35)*$B$6)

Expected Return and Standard Deviation of

Portfolio Return

10%

12%

14%

16%

18%

20%

0% 5% 10% 15% 20%

Standard deviation of portfolio return,

Expected portfolio return, E(r

)

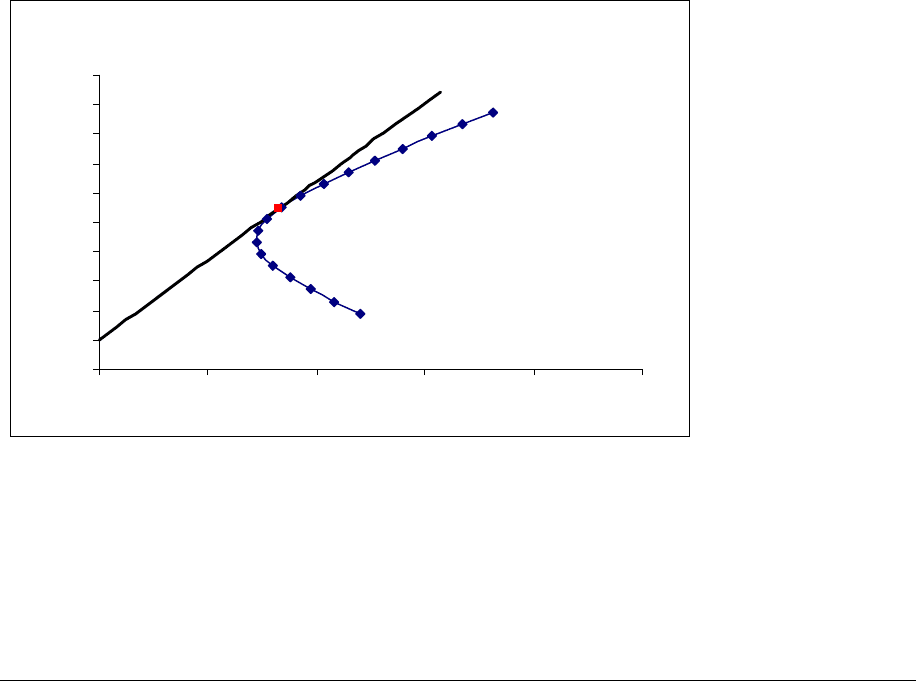
The line as drawn has several properties:

• It starts from the risk-free rate (2%) on the y-axis.

PFE, Chapter 13: The CAPM and SML page 12

• It goes to (and through) a portfolio on the efficient frontier market by the red square. As

you can see in cells B35:B38, this portfolio is composed 51.81% of Stock A and 48.19%

of Stock B. It has expected return 10.85% and standard deviation 8.26%. In Section ???

we’ll describe how we computed this portfolio.

• It is tangent to the efficient frontier—meaning, the line touches the efficient frontier only

at the red square portfolio and nowhere else.

• Finally (and this is the most important point) all the best investment portfolios are on the

red square line. This point is so important that we explore it in a separate subsection.

Optimal portfolios

To emphasize the optimality take another look at the “red point line”:

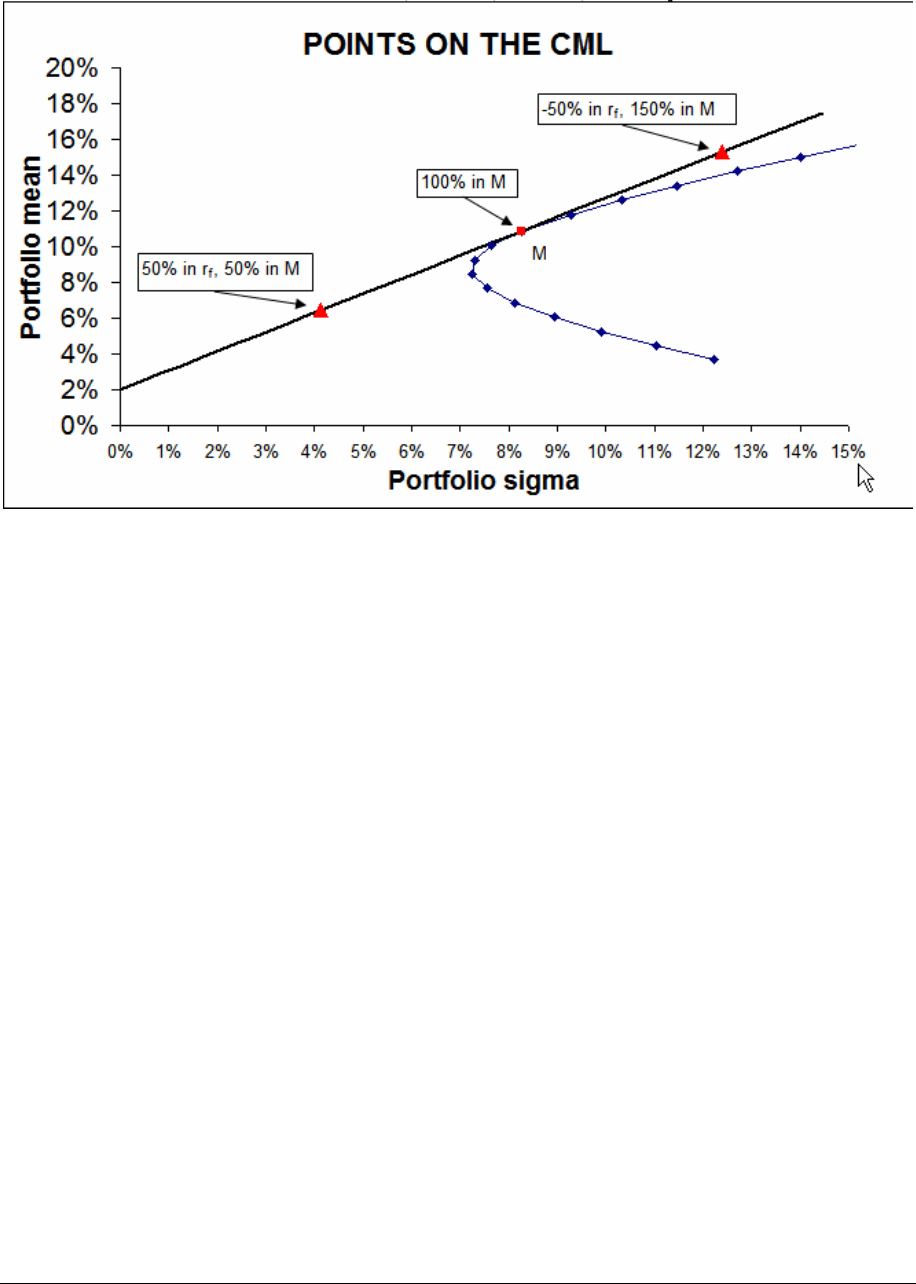
The Capital Market Line (CML)

The red square portfolio is the

Market

portfolio

10%

12%

14%

16%

18%

20%

0% 5% 10% 15% 20% 25%

Standard deviation of portfolio return,

Expected portfolio return, E(r

)

Notice that the line is above the efficient frontier

everywhere (except at point of

tangency, which we now call the

market portfolio M). We call this line the capital market line

(CML):

PFE, Chapter 13: The CAPM and SML page 13

The capital market line is the set of optimal investment portfolios. Each point on the line

is:

•

A combination of some percentage invested in the risk-free asset

•

Another percentage invested in the market portfolio M

In Section ??? we’ll show you how to compute the portfolio M. But first, in the next

section, we explore its meaning.

13.2. Three points on the capital market line (CML)—exploring optimal

investment combinations

What do portfolios on the CML—the line connecting the risk-free rate r

and the market

portfolio

M—look like? To get a feel for this, we explore three portfolios on the CML.

First example

Suppose you have $1000 to invest. You can choose any combination of 3 assets—the

risk-free asset, stock A, or stock B. Suppose you choose to invest $500 in the risk-free asset and

$500 in the market portfolio

M—the portfolio composed of 51.81% stock A and 48.19% stock B.

PFE, Chapter 13: The CAPM and SML page 14

ABCDEF

Stock

Stock B Risk-free

Average 7.00% 15.00% 2.00%

Variance 0.0064 0.0196

Standard deviation (sigma) 8.00% 14.00%

Covariance of returns, Stock A and Stock B 0.0011

Total amount to invest $1,000

Invested in risk-free 50%

Invested in market portfolio M 50%

The % in the market portfolio M is split as follows Percent Dollars

Stock A 51.81% $259.07 <-- =B13*B10*$B$8

Stock B 48.19% $240.93 <-- =B14*B10*$B$8

Expected return of market portfolio M 10.85% <-- =B13*B3+B14*C3

Standard deviation of market portfolio M 8.26% <-- =SQRT(B13^2*B4+B14^2*C4+2*B13*B14*B6)

Portfolio return statistics

Expected portfolio return 6.43% <-- =B9*D3+B10*B15

Portfolio standard deviation 4.13% <-- =B10*B16

PORTFOLIO ON THE CAPITAL MARKET LINE (CML)

The $500 invested in the risky assets are invested in the

market portfolio M. The dollar investment in Stocks A

and B corresponds to their proportions in M: $259.07 in

(51.81%) and $240.93 in B (48.19%).

This looks a little complicated, but it’s really a version of the portfolio calculations we

did in Chapter 12. Our investment is divided 50% into the risk-free asset and another 50% into

portfolio

M which has expected return 10.85% and standard deviation 8.26%. According to the

formula given in Section ???, the expected return and the variance of returns are calculated by:

()

(

)

(

)

pM f

rxEr xr

σσ

=+−

As you can see in cells B19:B20, this gives

(

)

6.43%, 4.13%

. This portfolio is

indicated in the graph below:

PFE, Chapter 13: The CAPM and SML page 15

Second example

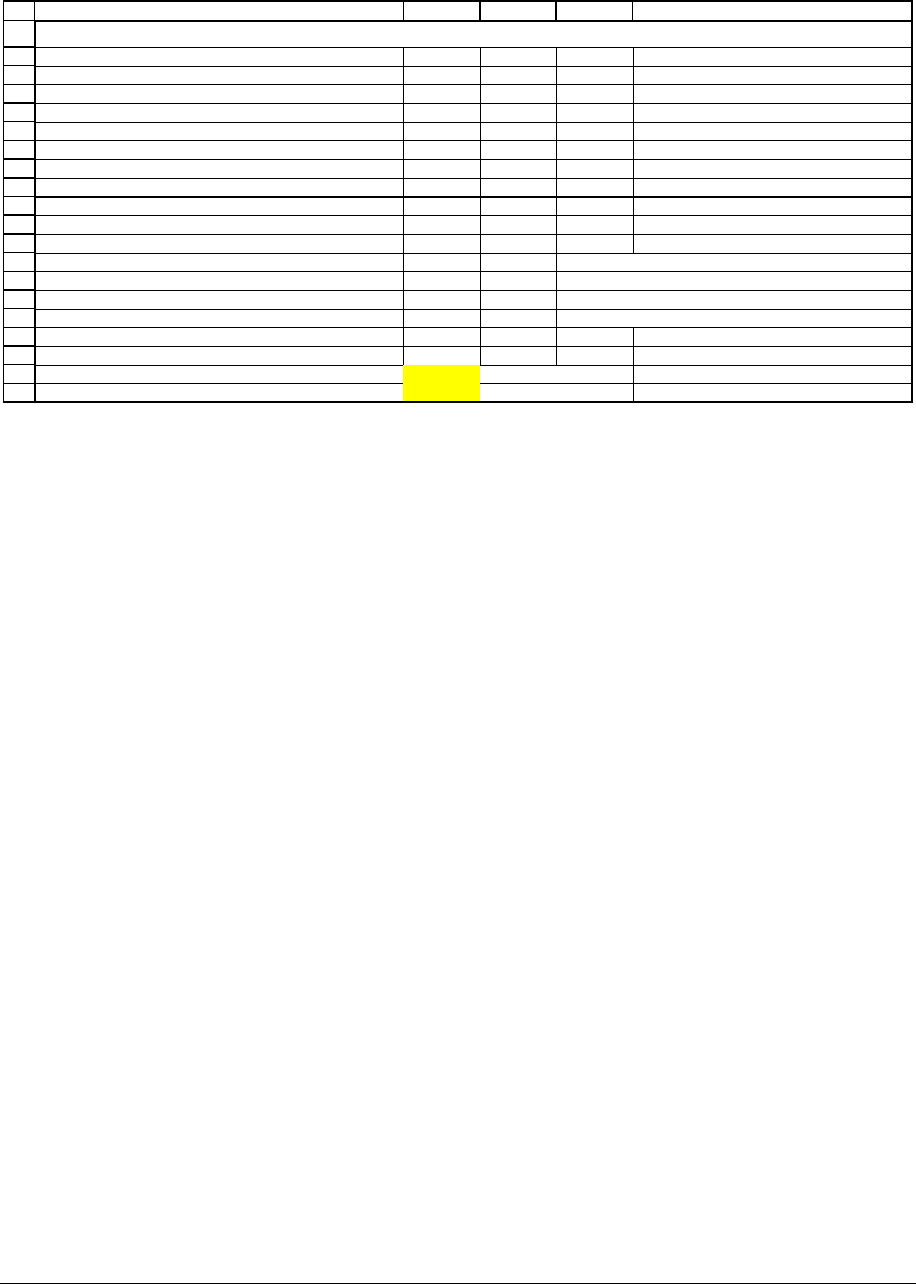
In the previous example, you split your investment of $1000 between the risk-free asset

and the market portfolio M. This time we’ll investigate an investment strategy in which you

borrow money at the risk-free rate and invest more than $1000 in the risky portfolio M. You do

this by using borrowed funds to increase your investment in M.

As before, you have $1000 to invest, and as before you choose to invest some of your

money in the risk-free asset and the rest in the market portfolio M, composed of 51.81% stock A

and 48.19% stock B. However, this time you choose to borrow $500 at the risk-free rate and

invest $1500 in the portfolio of stock A and stock B. As you can see below, this is a riskier

portfolio (it has a standard deviation of 12.40%), but it also has a higher expected return

(15.28%):

PFE, Chapter 13: The CAPM and SML page 16

ABCDE

Stock

Stock B Risk-free

Average 7.00% 15.00% 2.00%

Variance 0.0064 0.0196

Standard deviation (sigma) 8.00% 14.00%

Covariance of returns, Stock A and Stock B 0.0011

Total amount to invest $1,000

Invested in risk-free -50%

Invested in market portfolio M 150%

The % in the market portfolio M is split as follows

Percent Dollars

Stock A 51.81% $777.20 <-- =B13*B10*$B$8

Stock B 48.19% $722.80 <-- =B14*B10*$B$8

Expected return of market portfolio M 10.85% <-- =B13*B3+B14*C3

Standard deviation of market portfolio M 8.26% <-- =SQRT(B13^2*B4+B14^2*C4+2*B13*B14*B6)

Portfolio return statistics

Expected portfolio return 15.28% <-- =B9*D3+B10*B15

Portfolio standard deviation 12.40% <-- =B10*B16

PORTFOLIO ON THE CAPITAL MARKET LINE (CML)

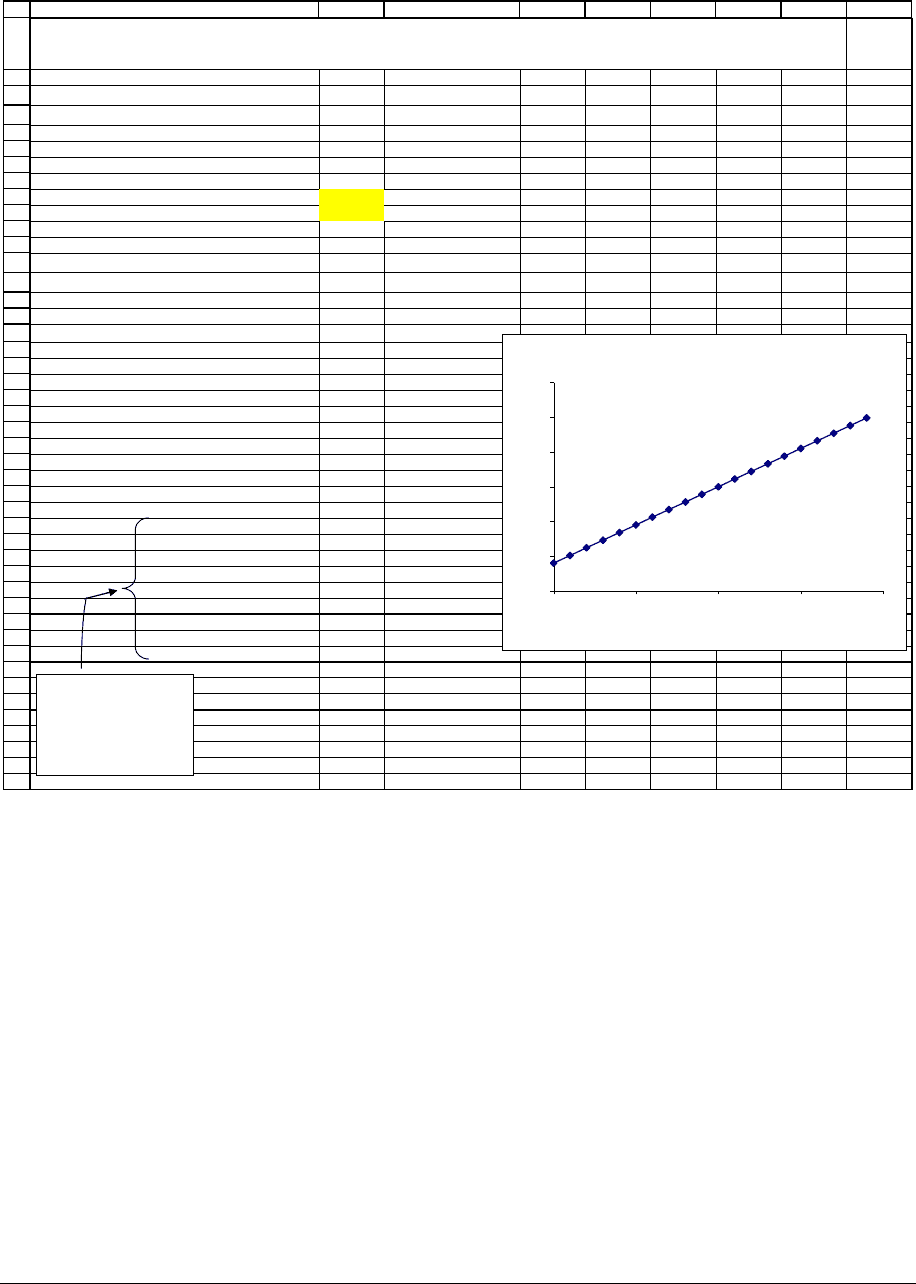
Generalizing

The portfolio calculations we’ve done in the previous two examples don’t really depend

on the $1,000 initial wealth. What’s important is the percentage of the investor’s wealth invested

in the market portfolio M and the percentage of the investor’s wealth invested in the risk-free

asset.

Suppose we denote these percentages by

and 1

− . Then the investor’s

portfolio will have:

•

Expected return

()

(

)

pMM Mf

rxEr xr=+−

•

Standard deviation of return

pMM

Here’s an example which uses statistics which are typical for the S&P 500:

PFE, Chapter 13: The CAPM and SML page 17

ABCDEFGHI

S&P statistics

Expected return, E(r

)

15%

Standard deviation of return,

20%

Risk-free rate of interest 4%

Investor's portfolio

Percentage of wealth invested in S&P 25%

Percentage of wealth invested in risk-free asset 75% <-- =1-B9

Investor's return and standard deviation

Expected portfolio return, E(r

)

6.75% <-- =B9*B3+B10*B6

Standard deviation of portfolio return,

5.00% <-- =B9*B4

Table

Percentage invested in S&P 500

E(r

)

0% 0% 4.00%

10% 2% 5.10%

20% 4% 6.20%

30% 6% 7.30%

40% 8% 8.40%

50% 10% 9.50%

60% 12% 10.60%

70% 14% 11.70%

80% 16% 12.80%

90% 18% 13.90%

100% 20% 15.00%

110% 22% 16.10%

120% 24% 17.20%

130% 26% 18.30%

140% 28% 19.40%

150% 30% 20.50%

160% 32% 21.60%

170% 34% 22.70%

180% 36% 23.80%

190% 38% 24.90%

INVESTING IN A COMBINATION OF THE S&P500 AND THE RISK-FREE

USING TYPICAL S&P500 STATISTICS

The Capital Market Line

Portfolio Expected return E(r

)

versus risk

10%

15%

20%

25%

30%

0% 10% 20% 30% 40%

Portfolio standard deviation

π/

Portfolio expected return E(r

)

These are borrowing

ortfolios --the investor

borrows at the risk-free

rate in order to increase

her investment in the

market portfolio M .

13.3. Computing the market portfolio M: the Sharpe ratio

In this section we’ll show how to compute the market portfolio M. In the process we’ll

introduce a concept called the Sharpe ratio—this is one of the standard reward-return measures

used in capital markets. As you’ll see, the portfolio M is the portfolio which maximizes the

Sharpe ratio.

PFE, Chapter 13: The CAPM and SML page 18

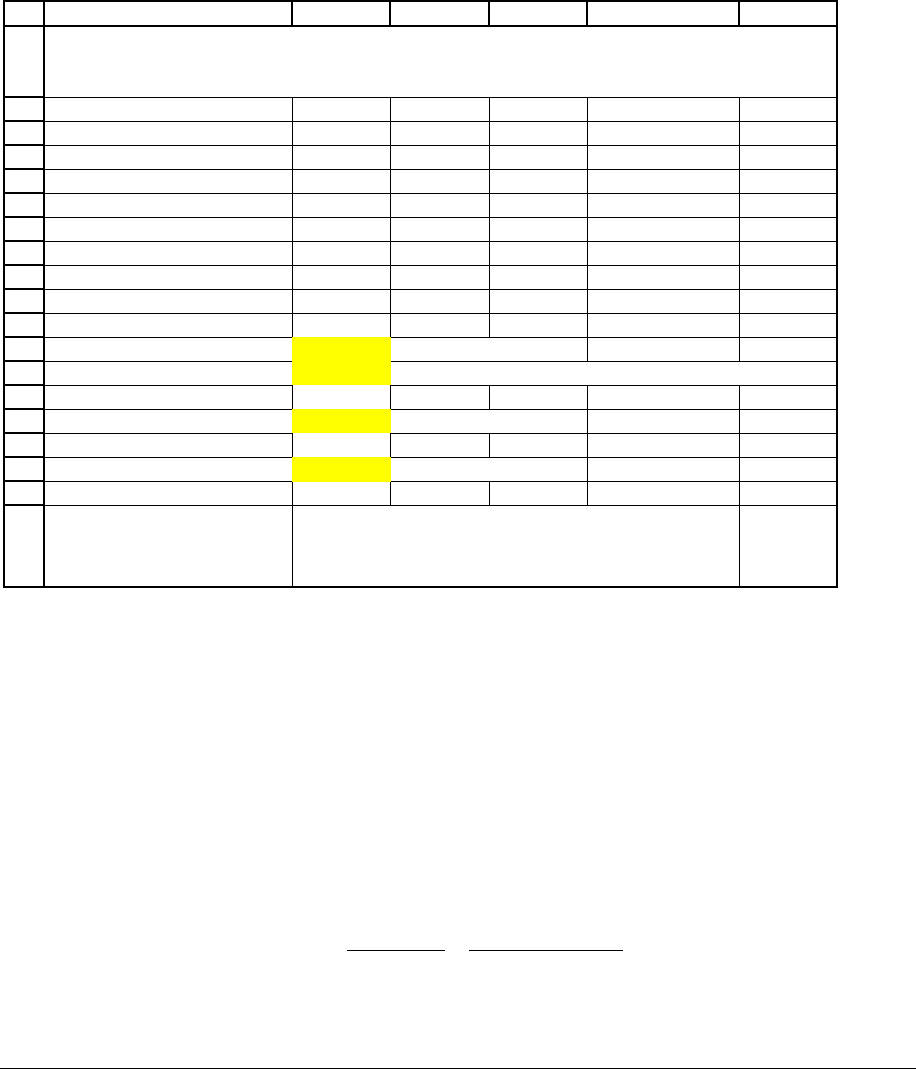
To get some intuitions, look at the spreadsheet below. It continues our example of Stocks

A and B and the risk-free rate of 2%. In cells B9:B10 we’re looking at a portfolio invested 30%

in Stock A and 70% in Stock B. The expected return of this portfolio is 12.60% and its standard

deviation is 10.32% (cells B12:B13):

ABCDEF

Stock A Stock B Risk-free

Average return 7.00% 15.00% 2.00%

Variance of return 0.64% 1.96%

Sigma of return 8.00% 14.00%

Covariance of returns 0.0011

Portfolio return and risk

Percentage in Stock A 30.00%

Percentage in Stock B 70.00%

Expected portfolio return 12.60% <-- =B9*C3+B10*D3

Portfolio standard deviation 10.32% <-- =SQRT(B9^2*C4+B10^2*D4+2*B9*B10*C6)

Excess return 10.60% <-- =B12-E3

Sharpe ratio 1.0271 <-- =(B12-E3)/B13

PORTFOLIO RETURNS WITH A RISK-FREE ASSET

THE SHARPE RATIO

The Sharpe ratio is [E(r

) - r

. It

denotes the ratio of portfolio

excess return to portfolio risk .

The portfolio’s excess return (sometimes called a risk-premium) is defined as the

difference between its expected return and that of the risk-free asset:

()

12.60% 2.00% 10.60%

ort

olio risk premium port

olio expected return risk

ree rate

Er r

−

=−

=−=

The ratio of this risk-premium to the portfolio’s standard deviation is called the Sharpe ratio:

(

)

12.60% 2.00%

1.0271

10.32%

Er r

Sharpe ratio

−

== =.

PFE, Chapter 13: The CAPM and SML page 19

The Sharpe ratio (named after William Sharpe, one of the developers of modern portfolio theory

and winner of the Nobel prize in economics in 1990) is a “reward/risk” ratio: The numerator is

the extra return (over the risk-free rate) you get from your portfolio, and the denominator is the

cost of this extra return—its standard deviation.

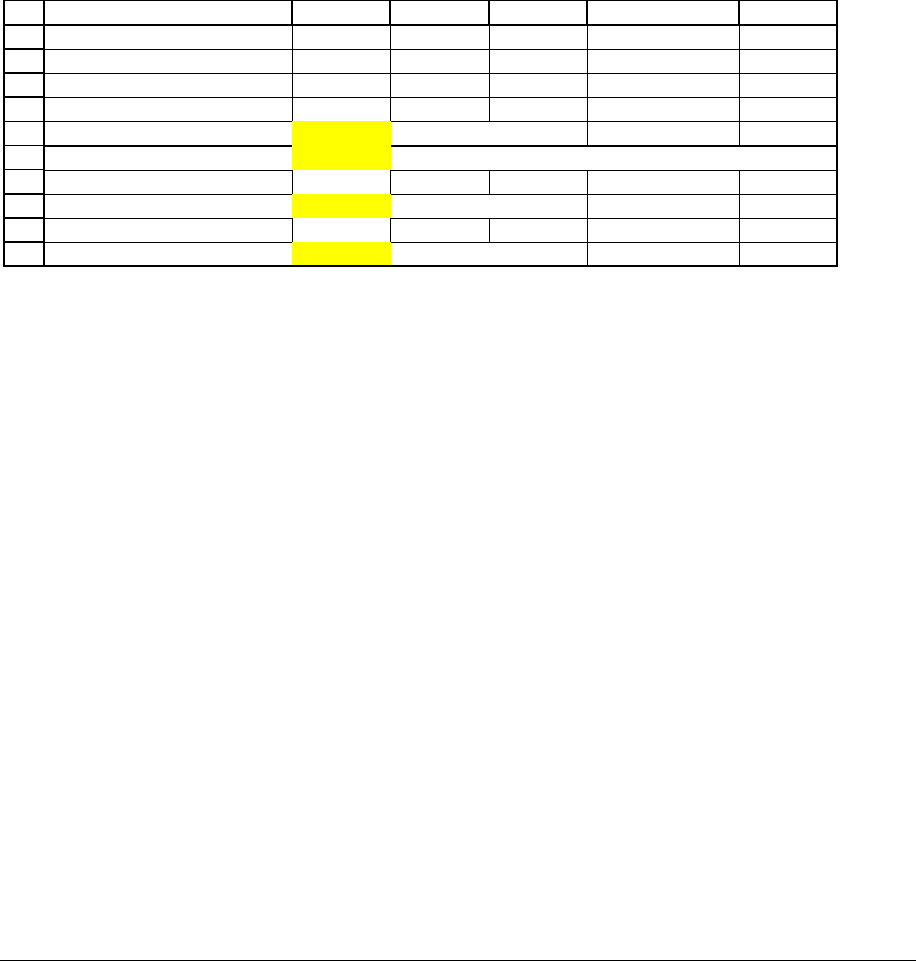
If you play a bit with the spreadsheet, you’ll see that there are other portfolios with higher

Sharpe ratios. Here’s an example:

ABCDEF

Portfolio return and risk

Percentage in Stock A 40.00%

Percentage in Stock B 60.00%

Expected portfolio return 11.80% <-- =B9*C3+B10*D3

Portfolio standard deviation 9.28% <-- =SQRT(B9^2*C4+B10^2*D4+2*B9*B10*C6)

Excess return 9.80% <-- =B12-E3

Sharpe ratio 1.0557 <-- =(B12-E3)/B13

Calculating the market portfolio M—the portfolio with the highest attainable Sharpe

ratio

We can use Excel’s Solver (see Chapter ????) to calculate the portfolio which gives the

highest Sharpe ratio. This portfolio is the market portfolio M.