Powers D.L. Boundary Value Problems and Partial Differential Equations

Подождите немного. Документ загружается.

308 Chapter 5 Higher Dimensions and Other Coordinates

Supposethat,insteadofboundaryconditionsEqs.(2)and(3),wehave

u(x, 0, t) = f

(x), u(x , b, t) = f

(x), 0 < x < a, 0 < t,(2



)

u(0, y, t) = g

(y), u(a, y, t) = g

(y), 0 < y < b, 0 < t.(3



)

Show that the steady-state solution involves the potential equation, and

indicate how to solve it.

7. Solve the two-dimensional heat conduction problem in a rectangle if there

is insulation on all boundaries and the initial condition is

a. u(x, y, 0) =1;

b. u(x, y, 0) = x + y;

c. u(x, y, 0) =xy.

8. Verify the orthogonality relation in Eq. (16) and the formula for a

9. Show that the separation constant −λ

must be negative by showing that

−µ

and −ν

must both be negative.

10. Show that the function

(x, y, t) = sin(µ

x) sin(ν

y) cos(λ

ct),

where µ

,ν

,andλ

areasinthissection,isasolutionofthetwo-

dimensional wave equation on the rectangle 0 < x < a,0< y < b, with

u =0 on the boundary. The function u may be thought of as the displace-

ment of a rectangular membrane (see Section 5.1).

11. The places where u

(x, y, t) = 0 for all t are called nodal lines.Describe

the nodal lines for

(m, n) = (1, 2), (2, 3), (3, 2), (3, 3).

12. Determine the frequencies of vibration for the functions u

of Exer-

cise 10. Are there different pairs (m, n) that have the same frequency if

a =b?

5.4 Problems in Polar Coordinates

We found that the one-dimensional wave and heat problems have a great deal

in common. Namely, the steady-state or time-independent solutions and the

eigenvalue problems that arise are identical in both cases. Also, in solving

problems in a rectangular region, we have seen that those same features are

shared by the heat and wave equations.

5.4 Problems in Polar Coordinates 309

If we consider now the vibrations of a circular membrane or heat conduc-

tion in a circular plate, we shall see common features again. In what follows,

these two problems are given side by side for the region 0 < r < a,0< t.

Wave Heat

∇

v =

∂

∂t

∇

v =

∂v

∂t

v(a,θ,t) = f (θ) v(a,θ,t) = f (θ)

v(r,θ,0) = g(r,θ) v(r,θ,0) = g(r,θ)

∂v

∂t

(r,θ,0) = h(r,θ)

In both problems we require that v be periodic in θ with period 2π:

v(r,θ,t) = v(r,θ +2π,t),

as in Section 4.5.

Although the interpretation of the function v is different in the two cases,

we see that the solution of the problem

∇

v = 0,v(a,θ)=f (θ )

is the rest-state or steady-state solution for both problems, and it will be

needed in both problems to make the boundary condition at r = a homo-

geneous. Let us suppose that the time-independent solution has been found

and subtracted; that is, we will replace f (θ) by zero. Then we have

∇

v =

∂

∂t

∇

v =

∂v

∂t

v(a,θ,t) = 0 v(a,θ,t) = 0

plus the appropriate initial conditions. If we attempt to solve by separation of

variables, setting v(r,θ,t) = φ(r,θ)T(t),inbothcaseswewillﬁndthatφ(r,θ)

must satisfy

∂

∂r



∂φ

∂r



∂

∂θ

=−λ

φ, (1)

φ(a,θ)= 0, (2)

φ(r,θ +2π) = φ(r,π), (3)

φ bounded as r →0. (4)

Now we shall concentrate on the solution of this two-dimensional eigen-

value problem. We can separate variables again by assuming that φ(r,θ) =

310 Chapter 5 Higher Dimensions and Other Coordinates

R(r)Q(θ). After some algebra, we ﬁnd that

(rR



)





=−λ

, (5)

R(a) =0, (6)

Q(θ) = Q(θ +2π), (7)

R(r) bounded as r → 0. (8)

The ratio Q



/Q must be constant; otherwise, λ

could not be constant. Choos-

ing Q



/Q =−µ

, we get a familiar, singular eigenvalue problem:



+µ

Q = 0, (9)

Q(θ +2π) = Q(θ). (10)

We found (in Chapter 4) that the solutions of this problem are

=0, Q

(θ) = 1,

, Q

(θ) = cos(mθ) and sin(mθ),

(11)

where m = 1, 2, 3,....

There remains a problem in R:







−

R +λ

rR =0, 0 < r < a, (12)

R(a) =0, (13)

R(r) bounded as r → 0. (14)

Equation (12) is called Bessel’s equation, and we shall solve it in the next sec-

tion.

EXERCISES

1. State the full initial value–boundary value problems that result from the

problems as originally given when the steady-state or time-independent so-

lution is subtracted from v.

2. Verify the separation of variables that leads to Eqs. (1) and (2).

3. Substitution of v(r,θ,t) in the form of a product led to the problem of

Eqs. (1)–(4) for the factor φ(r,θ). What differential equation is to be satis-

ﬁed by the factor T(t)?

4. Solve Eqs. (9)–(11) and check the solutions given.

5.5 Bessel’s Equation 311

5. Suppose the problems originally stated were to be solved in the half-disk

0 < r < a,0<θ<π, with additional conditions:

v(r, 0, t) = 0, 0 < r < a, 0 < t,

v(r,π,t) = 0, 0 < r < a, 0 < t.

What eigenvalue problem arises in place of Eqs. (9)–(11)? Solve it.

6. Suppose that the boundary condition

∂v

∂r

(a,θ,t) = 0, −π<θ≤ π, 0 < t

were given instead of v(a,θ,t) = f (θ ). Carry out the steps involved in sepa-

ration of variables. Show that the only change is in Eqs. (6) and (13), which

become R



(a) =0.

7. One of the consequences of Green’s theorem is the integral relation





f ∇

g −g∇



dA =





∂g

∂n

−g

∂f

∂n



ds,

where

R is a region in the plane, C is the closed curve that bounds R,and

∂f /∂n is the directional derivative in the direction normal to the curve

Use this relation to show that eigenfunctions of the problem

∇

φ =−λ

φ in R,

φ = 0on

are orthogonal if they correspond to different eigenvalues. (Hint: Use

f =φ

, g =φ

, m = k.)

8. Same problem as Exercise 7, except the boundary condition is

φ +λ

∂φ

∂n

=0on

5.5 Bessel’s Equation

In order to solve the Bessel equation,







−

R +λ

rR =0, (1)

we apply the method of Frobenius. Assume that R(r) has the form of a power

series multiplied by an unknown power of r:

R(r) = r



r +···+c

+···



. (2)

312 Chapter 5 Higher Dimensions and Other Coordinates

When the differentiations in Eq. (1) are carried out and the equation is multi-

plied by r,itbecomes



= α(α −1)c

+(α + 1)αc

α+1

+(α + 2)(α +1)c

α+2

+···

+(α + k)(α +k −1)c

α+k

+···



= αc

+(α + 1)c

α+1

+(α + 2)c

α+2

+···

+(α + k )c

α+k

+···

−µ

R =−µ

−µ

α+1

−µ

α+2

−···

−µ

α+k

+···

R = λ

α+2

+···

+λ

k−2

α+k

+···

The expression for λ

R is jogged to the right to make like powers of r line up

vertically. Note that the lowest power of r present in λ

R is r

α+2

Now we add the tableau vertically. The sum of the left-hand sides is, accord-

ing to the differential equation, equal to zero. Therefore

0 =c



−µ





(α +1)

−µ



α+1





(α +2)

−µ



+λ



α+2

+···+





(α +k)

−µ



+λ

k−2



α+k

+···.

Each term in this power series must be zero in order for the equality to hold.

Therefore, the coefﬁcient of each term must be zero:



−µ



= 0,



(α +1)

−µ



= 0,



(α +k)

−µ



+λ

k−2

= 0, k ≥2.

As a bookkeeping agreement, we take c

=0. Thus α =±µ. Let us study the

case α = µ ≥ 0. The second equation becomes



(µ +1)

−µ



and this implies c

=0. Now, in general the relation

=−

k−2

(µ +k)

−µ

=−λ

k−2

k(2µ +k)

, k ≥ 2, (3)

says that c

can be found from c

k−2

.Inparticular,weﬁnd

=−

2(2µ +2)

=−

4(2µ +4)

2 ·4 ·(2µ +2)(2µ +4)

5.5 Bessel’s Equation 313

and so forth. All c’s with odd index are zero, since they are all multiples of c

The general formula for a coefﬁcient with even index k = 2m is

(−1)

m!(µ +1)(µ +2) ···(µ +m)





. (4)

For integral values of µ, c

is chosen by convention to be





µ!

Then the solution of Eq. (1) that we have found is called the Bessel function of

the ﬁrst kind of order µ:

(λr) =



λr



∞



m=0

(−1)

m!(µ +m)!



λr



. (5)

This series serves us for evaluating the function and for obtaining its proper-

ties. (See the Exercises.) However, from now on, we conside r the Bessel functions

of the ﬁrst kind to be as well known as sines and cosines, although less familiar.

There must be a second independent solution of Bessel’s equation, which

can be found by using variation of parameters. This method yields a solution

in the form

(λr) ·



(λr)

. (6)

In its standard form, the second solution of Bessel’s equation is called the Bessel

function of second kind of order µ and is denoted by Y

(λr).

The most important feature of the second solution is its behavior near r =0.

When r is very small, we can approximate J

(λr) by the ﬁrst term of its series

expansion:

(λr)

∼





µ!

, r 1.

The solution Eq. (6) then can be approximated by

constant × r



1+2µ

=constant ×



ln(r), if µ = 0,

−µ

, if µ>0.

In either case, it is easy to see that



(λr)



→∞ as r → 0.

Both kinds of Bessel functions have an inﬁnite number of zeros. That is,

thereisaninﬁnitenumberofvaluesofα (and β) for which

(α) =0, Y

(β) = 0.

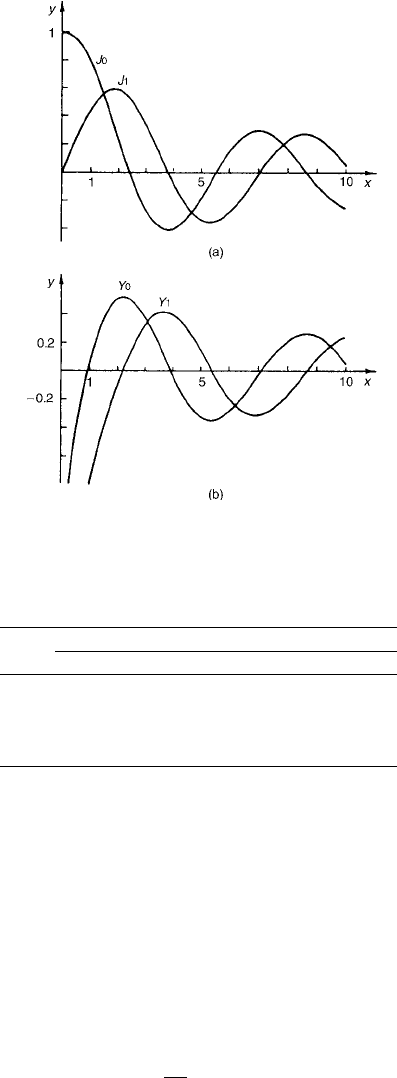
314 Chapter 5 Higher Dimensions and Other Coordinates

Figure 7 Graphs of Bessel functions of the ﬁrst kind. Also see the CD. (a) J

and

,(b)Y

and Y

m 12 3 4

0 2.405 5.520 8.654 11.792

1 3.832 7.016 10.173 13.324

2 5.136 8.417 11.620 14.796

3 6.380 9.761 13.015 16.223

Tab le 1 Zeros of Bessel functions. The values α

satisfy the equation J

(α

) = 0.

Also, as r →∞,bothJ

(λr) and Y

(λr) tend to zero. Figure 7 gives graphs

of several Bessel functions, and Table 1 provides values of their zeros. Further

information can be found in most books of tables.

The modiﬁed Bessel equation differs from the Bessel equation only in the

sign of one term. It is







−

R −λ

rR =0. (7)

5.5 Bessel’s Equation 315

Using the same method as in the preceding, an inﬁnite series can be developed

for the solutions (see Exercise 8). The solution that is bounded at r = 0, in

standard form, is called the modiﬁed Bessel function of the ﬁrst kind of order µ,

designated I

(λr), and its series is

(λr) =



λr



∞



m=0

m!(µ +m)!



λr



Summary

The differential equation





−

R +λ

rR= 0

is called Bessel’s equation. Its general solution is

R(r) = AJ

(λr) +BY

(λr)

(A and B are arbitrary constants). The functions J

and Y

are called

Bessel functions of order µ of the ﬁrst and second kinds, respectively.

The Bessel function of the second kind is unbounded at the origin.

EXERCISES

1. Find the values of the parameter λ for which the following problem has a

nonzero solution:



dφ



+λ

φ = 0, 0 < r < a,

φ(a) = 0,φ(0) bounded.

2. Sketch the ﬁrst few eigenfunctions found in Exercise 1.

3. Show that

(λr) = λJ



(λr),

where the prime denotes differentiation with respect to the argument.

4. Show from the series that

(λr) =−λJ

(λr).

316 Chapter 5 Higher Dimensions and Other Coordinates

By using Exercise 4 and Rolle’s theorem, and knowing that J

(x) = 0 for an

inﬁnite number of values of x, show that J

(x) = 0 has an inﬁnite number

of solutions.

6. Using the inﬁnite series representations for the Bessel functions, verify the

formulas



−µ

(x)



=−x

−µ

µ+1

(x),



(x)



µ−1

(x).

7. Use the second formula in Exercise 6 to derive the integral formula



(x)xdx= x

µ+1

(x).

8. Use the method of Frobenius to obtain a solution of the modiﬁed Bessel

equation (7). Show that the coefﬁcients of the power series are just the

same as those for the Bessel function of the ﬁrst kind, except for signs.

9. Use the modiﬁed Bessel function to solve this problem for the temperature

in a circular plate when the surface is exposed to convection:





−γ

(u −T) = 0, 0 < r < a,

u(a) =T

10. Using the result of Exercise 4, solve the eigenvalue problem



dφ



+λ

φ = 0, 0 < r < a,

dφ

(a) =0,φ(0) bounded.

5.6 Temperature in a Cylinder

In Section 5.4, we observed that both the heat and wave equations have a great

deal in common, especially the equilibrium solution and the eigenvalue prob-

lem. To reinforce that observation, we will solve a heat problem and a wave

problem with analogous conditions so that their similarities may be seen.

These examples illustrate another important point: Problems that would be

two-dimensional in one coordinate system (rectangular) may become one-

dimensional in another system (polar). In order to obtain this simpliﬁcation,

we will assume that the unknown function, v(r,θ,t), is actually independent

5.6 Temperature in a Cylinder 317

of the angular coordinate θ .(Wewritev(r, t) then.) As a consequence of this

assumption, the two-dimensional Laplacian operator becomes

∇

v =

∂

∂r



∂v

∂r



Suppose that the temperature v(r, t) in a large cylinder (radius a)satisﬁes

the problem

∂

∂r



∂v

∂r



∂v

∂t

, 0 < r < a, 0 < t, (1)

v(a, t) = 0, 0 < t, (2)

v(r, 0) = f (r), 0 < r < a. (3)

Because the differential equation (1) and boundary condition (2) are ho-

mogeneous, we may start the separation of variables by assuming v(r, t) =

φ(r)T(t). Using this form for v, we ﬁnd that the partial differential equa-

tion (1) becomes

(rφ



)



T =

φT



After dividing through this equation by φT, we arrive at the equality

(rφ



(r))



rφ(r)



(t)

kT(t)

. (4)

The two members of this equation must both be constant; call their mutual

value −λ

. Then we have two linked ordinary differential equations,



+λ

kT = 0, 0 < t, (5)

(rφ



)



+λ

rφ = 0, 0 < r < a. (6)

The boundary condition, Eq. (2), becomes φ(a)T(t) = 0, 0 < t.Itwillbesat-

isﬁed by requiring that

φ(a) = 0. (7)

We can recognize Eq. (6) as Bessel’s equation with µ = 0. (See Summary,

Section 5.5.) The general solution, therefore, has the form

φ(r) = AJ

(λr) +BY

(λr).

If B = 0, φ(r) must become inﬁnite as r approaches zero. The physical impli-

cations of this possibility are unacceptable, so we require that B = 0. In effect

we have added the boundedness condition



v(r, t)



bounded at r =0, (8)

which we shall employ frequently.