Peube J-L. Fundamentals of fluid mechanics and transport phenomena

Подождите немного. Документ загружается.

Thermodynamics of Continuous Media 53

exterior

interior

dsTfd

(a) (b)

(c)



V













Figure 2.3. Definition and symmetry of the stress tensor

The force density at the point M on the surface

is the

stress

tMT

.),(

Figure 2.3b shows the stresses on the faces of a parallelepiped whose corners are

parallel to the axes. The reader can easily verify that in the absence of volume sources of

torques, the equality of the moments about the axis

of the stresses exerted on the

four faces parallel to the axis

(see Figure 2.3c) leads to

2112

. The same goes

for the other components of the stress tensor (

jiij

2.1.3.3.

Volume source equivalent to the fluxes

The balances in a material domain are clearly always effected

on closed surfaces

which comprise the boundary. By applying the Ostrogradski theorem the flux

the quantity

leaving a closed surface

can be written:

³³³

dvqdivdsnq

[2.6]

This flux can thus be written in the form of a volume integral, which implies

that the total flux of the quantity

on a closed surface is equivalent to the

action of the

volume source

qdiv

of the quantity G.

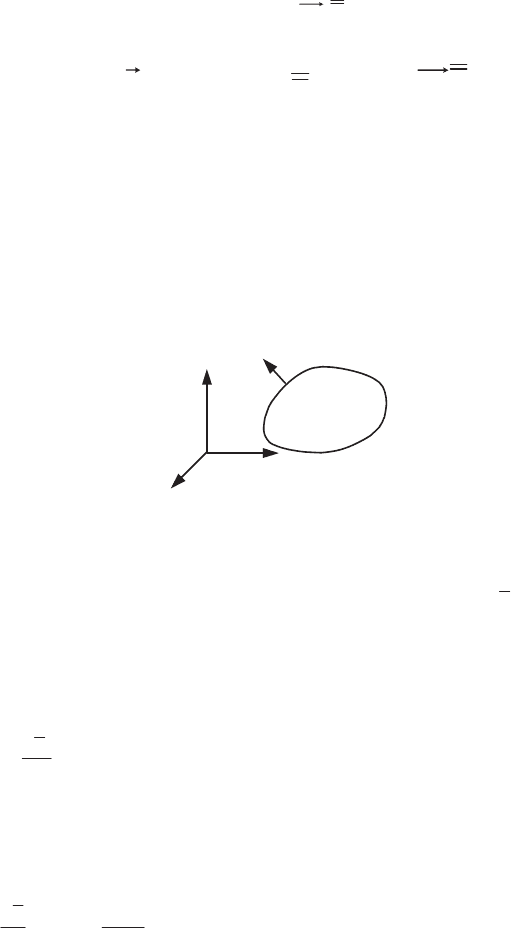
54 Fundamentals of Fluid Mechanics and Transport Phenomena

In the case of stresses in a continuous medium, a force volume source

generated by a stress is equal to the vector

div

³³³

66 D

dvdivdsndstMT

.),(

Reciprocally, all

volume sources

which are mathematically expressed by means of a

divergence operator

(

qdiv

for instance) can be interpreted as a transfer by the flux

across a closed surface of vector flux density

2.1.4. Balance equations in continuous media

2.1.4.1

. Balance equation of an extensive quantity

6

Figure 2.4. Balance of an extensive quantity

The balance equation of a volumetric extensive quantity

consists of

writing that the variation of this quantity in a material domain

is due to

contributions from outside, and which have thus crossed the closed surface

6

which constitutes the boundary of

(the normal is directed outwards), and to

volume sources of density

dsnqdvdv

jGj

³³³



[2.7]

Supposing this relation to be true regardless of the domain

, we can deduce the

local equation:



[2.8]

Thermodynamics of Continuous Media 55

For a fixed medium, which can thus not be deformed, mass

must be strictly

conserved, and we have:

Balance equation [2.8] for the quantity

can also be written, using the mass

quantity



[2.9]

For a fixed continuous medium, the principal extensive quantities are internal

energy (in its thermal form, and in the absence of possible physicochemical

reactions), volume and the number of moles of a chemical species. Electric

quantities may also be manifested; however, we will not deal with such questions in

this work.

For a material of constant

specific heat C

, in the absence of chemical reactions,

the variation of volumetric internal energy

is equal to

U

CdT

, and the balance

equation can be written:



[2.10]

Heat-source volume

is in fact a residuum of another form of energy which is

present in the mass and which is degraded in the form of heat (Joule effect caused by

the passage of an electric current (Ohm’s law), absorption of electromagnetic

radiation, etc.). This result could be obtained in a general way, but such a discussion

is beyond the scope of this work. We will come back to this question in Chapters 3

and 4 when it comes to dealing with mechanical energy.

For a chemical species comprising a number of moles

per unit volume (molar

concentration), the balance equation can be written:

ijn



[2.11]

Excepting diffusion processes, for which we only give volumic balances in this chapter

(section 2.4.4.2.2).

56 Fundamentals of Fluid Mechanics and Transport Phenomena

The molar flux densities q

and the sources

of chemical species are due,

respectively, to diffusion phenomena and chemical reactions.

2.1.4.2. Entropy source

During the natural evolution of a system, entropy is not conserved and it can only

increase. We have already described the entropy-creation mechanism in the case of two

discrete sub-systems that are in contact and have different temperatures (section 1.4.2.3)

and (section 1.4.2.4). The zone where entropy is created was localized in the contact

zone between the two sub-systems. Here the distribution of entropic variables is

continuous, and entropy creation will be diffused and associated with the existence of

gradients with entropic variables.

On account of the local equilibrium hypothesis, relation [1.13] can be written, for the

per mass unit quantities:



[2.12]

By replacing the derivatives

in equation [2.12] by expressions obtained using

balance equations [2.9], the following relation can be obtained:

Gki

Gkik

Gkk

Gki

Gkk





[2.13]

in which the surface flux terms associated with the divergence operator have been

separated from those associated with the volume source as defined in section 2.1.3.3.

The entropy is associated with the extensive quantities and the corresponding entropy

addition are represented by two terms:

–

SGkk

external entropy source associated with the source

of the

extensive quantity

Gk;

–

SGkik

ZqZ

entropy flux associated with the flux of extensive quantities.

Thermodynamics of Continuous Media 57

The thermodynamic imbalance is characterized by the gradients

Zgrad

, which

we designate under the label

thermodynamic forces. The additional term

Gki

of equation [2.13] is the

entropy source associated with the local macroscopic

imbalance

. It is always positive. Its expression is analogous to the expression for the

creation of entropy



2,1



from formula [1.31].

2.1.5. Phenomenological laws

2.1.5.1. Introduction

An irreversible evolution is characterized by flux density fields of the quantity

in a continuous medium. These thermodynamic fluxes are associated with gradients of

intensive entropic quantities

. The transfer of a quantity G

can occur via relatively

different kinds of processes:

Molecular agitation leads to an exchange of extensive quantities between the

particles involved. These intermolecular actions occur from place to place, because it is

these microscopic particles themselves which transport the extensive quantity considered

(mass, momentum, energy, chemical species, etc.). The interaction zone between two

material domains

and D

separated by the surface S (Figure 2.5) is limited to a

thickness in the order of 2d (d: intermolecular distance in liquids or mean free path in

gases). This extremely thin zone is modeled on the macroscopic scale by the surface

on which we can consider

contact actions.

Figure 2.5. Interaction zone between material domains D

and D

2) On the macroscopic scale, and for turbulent flows, we observe chaotic

velocity fluctuations which lead to fluxes of extensive quantities convected by the

fluid. The effective interaction zone between two material domains is no longer

limited to a surface as before. The flux depends on the structure of the whole

turbulent zone. Considerable difficulties result from this situation in which the cause

of the flux of an extensive quantity can no longer be modeled with a general local

phenomenological law ([COU 89], [MAT 00], [TEN 72]).

58 Fundamentals of Fluid Mechanics and Transport Phenomena

3) In other situations, the flux of an extensive quantity (essentially energy) is due

to the presence of an electromagnetic field. This is the case for energy transfer by

thermal radiation in semi-transparent media, which both emit and absorb at all

points and whose local state results from the emission balance in a macroscopic

volume surrounding the point considered. Here again, we can no longer localize the

cause of the extensive quantity flux on a single surface.

2.1.5.2.

Contact actions and thermodynamic forces

The interaction zone between two material domains D

and D

is modeled by the

surface

S. The thermodynamic forces, represented by

Zgrad

, are the cause of

thermodynamic fluxes.

As with discrete systems (section 1.4.2.6)

all causes of the same tensorial nature

act on

all the corresponding effects and we have a coupling of irreversible

phenomena

: for example, a temperature gradient leads to a material flux (thermal

diffusion). Phenomena of different tensorial orders do not interact.

A rudimentary explanation of these facts can be provided from context of kinetic

gas theory. A gas is a set of molecules which are subjected to a thermal agitation.

Irreversible phenomena are the macroscopic result of this

spontaneous action.

Molecules with different properties (mass, type, kinetic energy, etc.) do not respond

in the same way to non-symmetries in the mean properties of the medium. A

molecular concentration of a given species will be progressively diluted in the rest

of the gas; a temperature gradient (gradient of the molecular kinetic energy) will not

act in the same way on different species of molecules and so may create a

concentration gradient. For example, at equal energy, we notice that smaller, and

therefore faster, molecules can slip in a gas comprising larger molecules, hence the

phenomenon of

thermal diffusion. On the other hand, it is difficult to see how the

static scalar properties of a gas which is macroscopically at rest can spontaneously

generate a vector macroscopic momentum (i.e. a bulk movement) in the absence of

an external influence.

There thus exists a relation between thermodynamic forces and thermodynamic

fluxes of the same tensorial rank. Since in the absence of thermodynamic forces, the

thermodynamic fluxes are zero, the general form of this relation can be written as:

 

( , 1,..., ) with: 0,0,... 0

lGk

qFgradZ kl K F 

GJJG JJJJJG JJGGG G

[2.14]

On account of the principle of action and reaction, the function

is odd

(









ZgradFZgradF  ). Relation [2.14] must verify properties of

Thermodynamics of Continuous Media 59

homogeneity and of spatial and material isotropy. In what follows, we will limit our

discussion to cases where the difference from thermodynamic equilibrium is

relatively small, so that we can justify a first order Taylor expansion of the functions





ZgradF





lkll

ZgradLZgradF

For a set of

K extensive quantities,

is a square matrix of order K whose

terms are functions of the values of the

K intensive quantities Z

at the point Z

about which linearization is effected. When the preceding approximation is valid,

we consider that we are dealing with

linear thermodynamics of irreversible

phenomena.

If the medium is considered to be isotropic, the matrix is reduced to a matrix of

dimension

K (see section 2.1.5.3.1).

Thermodynamics does not provide access to any properties associated with the

matrix

. However, by means of statistical reasoning the matrix can be shown to

be symmetric (Onsager’s relations). This

symmetry is only verified if the entropic

variables Z

are used to define this matrix.

In practice, the local imbalance is characterized using simpler variables than the

intensive entropic variables

. For example, we use temperature in place of the

entropic intensive variable

(section 1.3.1.1). Expressions for linearized

thermodynamic force are equivalent to first order, but the matrix coefficients

are

modified, such that the symmetric properties generally disappear. An analogous

situation has been observed in the case of discontinuous media (section 1.4.2.6.2).

2.1.5.3.

Some simplifying laws for irreversible transfer

2.1.5.3.1. Fourier’s law and thermal conduction

Consider firstly a case where the medium comprises a pure body, such that

thermal transfer occurs alone, without any coupling with diffusion or electrical

conduction. The relation between the thermal flux density and the thermal gradient

can be written in the context of linear thermodynamics (Fourier’s law) as:

.or

Ti ij

qgradT q

 

G JJJJJG

ȜȜ

The principal axes of the 3*3 tensor

are the symmetric axes of the medium.

If the medium is homogenous (fluids, non-crystalline solids, etc.), the three

60 Fundamentals of Fluid Mechanics and Transport Phenomena

eigenvalues of the tensor

are equal and this one is spherical (

ijij

); it can

thus be expressed solely as a function of the

thermal conductivity

of the medium.

Fourier’s law can be written as:

Tgradq



[2.15]

The thermal conductivity of the medium

O

is expressed in Watts/meter.K.

Heat is the macroscopic form of mechanical energy related to thermal agitation

of a medium. Its transfer in the medium is due to interactions between the

microscopic entities (molecules, atoms, ions, electrons). The values of the

coefficient

depends on the nature of the medium.

Metallic media are excellent thermal conductors, thermal conduction being

principally assured by electrons which have a high mobility. The values of the

coefficient

lie in a range spanning from a few tens to a few hundred W/m.K.

Solid crystalline media are generally good conductors: as the crystalline structure

presents a reasonably strong coherence on account of its organization, energy can be

transmitted in a vibrational form (phonons). The coefficient values

are in the order

of a few W/m.K.

Solid amorphous media or composites have weaker conductivity: well under 1

W/m.K for fibrous materials.

Liquids, comprising a looser structure, are poorer conductors of heat than solids

(of the order of 0.1 to 0.2 W/m.K): intermolecular forces here assure conduction.

Energy exchange in

gases only occurs by means of molecular collisions in the

gaseous medium; the corresponding values of the coefficient

O are of the order of

0.01 to 0.02 W/m.K. Insulating materials are constituted of a matrix (fibers, wools,

foams, etc.) which is as light as possible, thermal insulation being assured by the

gaseous interstices.

The thermal flux across a surface

S can thus be written as:

³³³

 

SSS

dsnTgraddsnq ...

OOM

GGG

We can also define the

thermal diffusivity a:

Thermodynamics of Continuous Media 61

The reader can easily verify that this quantity

a can be expressed in m

/sec; we

will see the important role that this quantity plays in the heat equation (section

2.3.1).

The thermal diffusivity takes on the following values at 20ºC:

– air: 0.19 × 10

-4

. s

-1

;

– water: 1.4 × 10

-7

. s

-1

;

– metals: ~ 10

-4

. s

-1

2.1.5.3.2. Fick’s law and the diffusion of chemical species

As the extensive variable is here the number of molecules of a chemical species,

the corresponding intensive entropic variable is



(where μ is the chemical

potential (section 1.3.1.1)). In ideal solutions or perfect gases, we replace the

intensive entropic variables with a molar or mass concentration variable. This

simplified approach will suffice here for an exposition and discussion of the

phenomena which we will examine. It will allow us to outline the principal

difficulties associated with fluid movement. The additional complications which

arise when we take account of more complex thermodynamic and chemical

properties are beyond the scope of this work.

The presence of a concentration gradient leads to the existence of a molecular

flux towards regions of weaker concentration. Taking the molecular concentration

(the number of moles per unit volume) of a component, Fick’s law, which

characterizes the diffusion of the species considered, can be written:

ngradDq



[2.16]

where

D is the diffusion coefficient.

Diffusion is a complex phenomenon, as it implies that the material is not

immobile, and in such cases it is not possible to effect a generalized reasoning

using a single constituent. We will come back to this point at a later stage

(section 2.4), and we will specify the conditions under which law [2.16] is valid.

62 Fundamentals of Fluid Mechanics and Transport Phenomena

2.1.5.3.3. Electrical conduction and Ohm’s law

Electric conduction presents certain analogies with thermal conduction and

diffusion; however, the underlying physics is a little different. Here we are dealing

with an electric field derived from an electric potential

V which exerts a force on

mobile electric charge carriers. Ohm’s law has the same form as the preceding laws:

elel

Vgradj



where

is the electric current density and

is the electrical conductivity.

Thermal molecular agitation, which grows with temperature, slows down the

movement of electric charge-carriers because of collisions; thus, electrical

conductivity is a decreasing function of temperature, whereas for other irreversible

phenomena, thermal agitation is the driving factor of thermal and diffusional fluxes.

2.1.5.3.4. General case: coupled transfer between diffusion and thermal

conduction

In general, the flux of a scalar quantity (energy, chemical species) depends on all

the local thermodynamic forces

,,,etc.,

grad T grad n grad p

JJJJJG JJJJJG JJJJJG

associated with these

scalar quantities.

We can schematically write under certain conditions the following relations for

the diffusion of a constituent of binary mixing in the presence of heat transfer:

ngradD

Tgrad

ngradKTgradq



The coefficient

characterizes the thermal diffusion (Soret effect), i.e. the

existence of a flux of chemical species which is associated with a temperature

gradient. The symmetric effect of a heat flux caused by a concentration gradient

(Dufour effect) and characterized by the coefficient

K is generally much weaker.

From a physical point of view, the problem is more complex than this, as each

chemical species introduces its own thermal energy (enthalpy at constant pressure,

etc.). Definitions of the preceding coefficients may vary, and we will not provide a

complete discussion of these phenomena, as this would require lengthy discussions

concerning chemical thermodynamics ([BIR 01], [DEG 62], [PRI 68]).