Peube J-L. Fundamentals of fluid mechanics and transport phenomena

Подождите немного. Документ загружается.

Thermodynamics of Discrete Systems 33

The natural evolution of the system

S from initial temperatures T

and T

leads

to the final temperature

2010



, which is identical for the two blocks, and

to the final entropy S of the system:

2const

SmCLnT

The variation in entropy 'S between the final and initial instants is thus:

 





2010

4 TT

mcLn

mcLnS

It is always positive and independent of the intermediate evolution between the

initial and final instants.

1.4.2.2.2.

Entropy sources

Let dQ

p,q

be the quantity of heat received in time dt by the component p

(

2,1 p

) from the other component (

pq z 2,1

1,22,1

 dQdQ

The heat flow

Tp,q

received by the sub-system p is the quantity of heat

dtdQ

received per unit time by the component p (

2,1 p

) from the other

component. Thus, we have:

 

2,11,2

2,1

1111

TTdt

Tdt

[1.30]

The rate of entropy creation

dtdS is always positive on account of the fact that

the heat transfer naturally occurs from the hot body to the cold body:

> T

 dQ

1,2

< 0 and T

< T

 dQ

1,2

> 0

Let us consider the first situation (T

> T

). The quantity dQ

2,1

is positive; the

entropy (positive) dS

gained by sub-system 2 is greater than the entropy -dS

lost by

sub-system 1. This is in accordance with the fact that for irreversible heat transfer,

the entropy gained by a system is greater than the entropy lost by the body which

has provided the heat.

34 Fundamentals of Fluid Mechanics and Transport Phenomena

The higher the degree of the imbalance, the greater the thermal flux, and the

greater the rate of entropy creation.

1.4.2.3.

The insulated system and entropy creation

Let us consider two bodies, each in equilibrium, and which exchange a quantity

X across a wall permeable for this quantity. We define the intensive entropic

parameters:

During a quasi-static transformation where the quantity dX

1,2

= -dX

exchanged in time dt, we obtain:



2,11,2

2,1

 

[1.31]

As the entropy can only increase, the value of X increases (resp. decreases) in the

sub-system for which the value of Z is greatest (smallest). The transfer of the

quantity X occurs spontaneously from the sub-system with the smallest value of Z to

the sub-system with the greatest value of Z.

As in the preceding section, we see that during an irreversible transfer of an

extensive quantity, the entropy gained by a system is greater than the entropy lost by

the body which has lost this extensive quantity.

Expression [1.31] can be immediately generalized to a system constituted of P

sub-systems characterized by I independent extensive quantities:

11 1

qip

¦¦ ¦

Thermodynamics of Discrete Systems 35

or, after grouping the opposing fluxes (

p < q, so as to only count this component

once):



qip

qipi





¦¦ ¦

11 1

1.4.2.4.

Systems with external exchanges and entropy source

Let us first consider the same thermal systems as above (section 1.4.2.2), but

where each of these receives a quantity of heat

(p = 1,2) in time dt from the

exterior. For the heat exchange we have (Figure 1.6b):

0:with;;

1,22,121,2212,11

  dQdQdQdQdQdQdQdQ

The increase in system entropy is:



212

2,1

TTT

[1.32]

The external entropy supply terms

and

can now take any

sign. The term in [1.32] which corresponds to irreversible internal heat exchange,

which is always positive, constitutes an entropy source. We thus have:

2,1

[1.33]

Suppose, in addition, that the external

sources of heat are in thermostatic

equilibrium

, such that their temperatures T

and T

are defined. These transfers are

natural internal evolutions for any ensemble constituted of a sub-system and a

corresponding external source. They are, as before, entropy generators; we can

therefore write:

2,12,12,1

 

¦¦¦

pSp

TTT

[1.34]

36 Fundamentals of Fluid Mechanics and Transport Phenomena

By then evaluating the entropy lost by the external sources we obtain the

Clausius inequality:

¦¦

2,12,1

[1.35]

Similarly, for internal and external exchanges of an extensive quantity

X, we

obtain, using the same notations as above:



12 12

positive term

dS dX dX

ZZZ

dt dt dt dt

  

The term



2,1

 , which is always positive, is the entropy source

associated with internal exchanges.

The rate of entropy creation is proportional to

the intensity

2,1

of the internal exchanges and to the imbalance



ZZ 

between the two sub-systems.

In general, the internal production of entropy

dtdS

int

(entropy source) is

associated with the evolution of all of the extensive quantities in this system, which

is made up of two sub-systems:



IiZZ

,....2,1

2,1

int



[1.36]

Finally, let us recall that the entropic intensive quantities Zi are related to the

energetic intensive quantities ([1.12]:

TZY  ) and that it is possible to express

the entropy source as a function of any other quantities.

The reasoning used above leads to an expression for entropy sources

int

in a

system made up of

P sub-systems characterized by I independent extensive

quantities:

int

111

()

IPP

ip q

ip ip

ipq

ZZ pq

dt dt



¯

¡°



¡°

¢±



[1.37]

Thermodynamics of Discrete Systems 37

Expression [1.37] only applies to internal entropy creation in the system

considered. Entropy production is also associated with the transfer of extensive

quantities from sources external to the system; this entropy production can be

evaluated in the same way. For thermal transfers between external temperature

sources

(p = 1,…,P) and the system, we have, as above, the Clausius inequality:

¦¦

1.4.2.5.

The average intensive quantity

1.4.2.5.1. Definition

An out-of-equilibrium system is characterized by a collection of intensive

quantities whose values differ according to the sub-systems considered. It may be

useful to characterize the system by a global intensive variable, which is an “average

value” of the intensive variables of the sub-systems. In order to define this average

value, we will refer to an “equivalent” equilibrium state of the system.

Consider an out-of-equilibrium system

S made up of P sub-systems S

each of

which is in instantaneous equilibrium (quasi-static transformations). For each of

these, we can define the intensive entropic quantities

associated with their N

extensive quantities

. The total amount of extensive quantity X

contained in the

system

S is the sum

of the extensive quantities of each sub-system.

It is clear that the system

S cannot be described by any intensive quantity

associated with

. We can however associate system S with an average intensive

quantity

or Z

at any given instant t, defined as the intensive quantity which the

system S would attain following a natural evolution during which values X

should

be constant (without any external contribution). Let us consider as an example the

variables

Suppose that during the transformations undergone by the system, certain

extensive quantities

);,...,1(

pIiX



of the sub-systems are exchanged, while

the other

IN  extensive quantities remain constant in each of the sub-systems (for

example mass, number of moles, volume, etc.). All intensive quantities of all sub-

systems vary during the exchange of extensive quantities. In the final state of the

previously defined system,

intensive quantities Z

corresponding to exchanged

extensive quantities have the uniform value Z

for all sub-systems:

38 Fundamentals of Fluid Mechanics and Transport Phenomena

In each sub-system

p, every intensive quantity Z

corresponding to the extensive

exchanged quantities X

can be expressed as a function of the N extensive quantities

(

I extensive quantities X

exchanged with other sub-systems and N – I other

extensive quantities

which are constant for the sub-system).





),...,1;,...,1;,...,1(, PpINjIiXXZZ

jpipipip



By solving the preceding system of

I equations for each sub-system p, with

respect to the extensive quantities

, we obtain:





),...,1;,...,1;,...,1(, PpINjIiXZXX

jpipipip



The quantities

for the system S can be immediately obtained:



);,...,1;,...,1(,

INjIiXZXX

jpipipi



The equilibrium conditions for the system can be written

);,...,1( pIiZZ

imip



pIZZ

Considering the quantities

to be constant, we obtain a system of I equations

from which we can evaluate the

I average intensive quantities Z

which

characterize the out-of-equilibrium system:





, ( 1,... ; 1,..., 1)

iipimjp

XXZXiIjN







[1.38]

We laid down that certain extensive variables were not exchanged between the

sub-systems. The problem can be easily discussed in the same manner with different

conditions: for example, by fixing the uniform value to certain intensive quantities

in the whole system (section 1.4.2.5.2, example 2), or by choosing different

conditions according to certain ensembles of sub-systems.

1.4.2.5.2.

Examples

We will consider two examples in order to illustrate the preceding procedure,

which we will encounter again in the study of fluid mechanics:

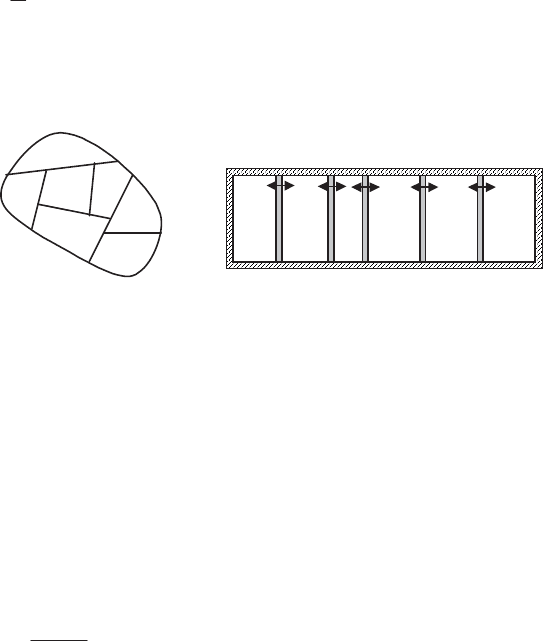
A thermal system – consider a system S

(Figure 1.7a) whose P sub-systems

exchange heat via a constant-volume process, such that the temperature

is the

only variable intensive quantity of any of the sub-systems during the process. The

energy

of each sub-system can be expressed as a function of its temperature and

Thermodynamics of Discrete Systems 39

its specific heat capacity

, which is assumed to be constant for the sake of

simplicity:

ppp

The energy

E of the system and its average temperature T

can be obtained:

¦¦¦

* **

* *

pmm

TTTE

111

:with;

The average temperature of the out-of-equilibrium system can thus be written:

ppm

In this particular case, the average temperature is the average of the temperatures

weighted by the specific heat capacities.

(a) System

(b) System

Figure 1.7.

Examples of out-of-equilibrium system:

(a) incompressible thermal system S

; (b) compressible thermal system S

A thermo-compressible system – consider now a system S

(Figure 1.7b),

which is comprised of sub-systems of variable volumes

, containing a perfect

gas and susceptible to exchange heat between each other. The volumes

are

separated by pistons which may be subject to fluid friction. The equations of

state for a perfect gas (section 1.3.2.5) give the expression sought for the

extensive quantities:

( : heat capacity at constant volume)

ppvppvp

nRT

VET

**

40 Fundamentals of Fluid Mechanics and Transport Phenomena

The global volume and internal energy balances for a natural transformation

allow the average pressure and temperature to be defined:

11 1

;

with: and:

PP P

vp p v m

pp p

pvvp

nRT

VV E TT

 



   



 



We obtain the following expressions for the average temperature and pressure:

¦¦

pvp

nTp

111

The average values of the intensive quantities are no longer simply weighted

arithmetic averages.

1.4.2.5.3.

Some comments

1) The “equivalent” system used to define the average intensive quantities is in

thermostatic equilibrium. It thus behaves according to a general state equation and

may therefore constitute a reduced representation of the out-of-equilibrium system,

which is clearly incomplete for a detailed description of the sub-systems. With the

exception of entropy, this is coherent with the laws of thermostatics (we can also say

that this is a “consistent” representation).

2) The average intensive quantities are only weighted arithmetic averages if the

expressions for the extensive quantities

are linear functions of X

and Y

. The

reader can verify that the state equation for the equivalent complete system

comprises the same linear properties.

3) In the examples of the last section we have considered two systems

constituted of sub-systems with identical properties. The study of more complex

systems, comprising combinations of sub-systems with different structures, can be

effected in the same way.

4) The procedure for definition of average intensive quantities can also be

applied to systems whose intensive quantities may be subjected to certain

constraints. Let us reconsider the example of Figure 1.7a, in which the sub-systems

are all at constant pressure equal to that of the atmosphere, rather than being at

constant volume. We suppose nonetheless that the external walls of the system,

which are in contact with the atmosphere, are adiabatic. The conservation of internal

energy can be written by taking into account the work done by the atmospheric

Thermodynamics of Discrete Systems 41

pressure on the system. The reader can verify that this amounts to writing the

conservation of enthalpy of the sub-systems.

1.4.2.6.

Phenomenological laws

1.4.2.6.1. Introduction

In general, all causes of the same tensor nature act on all of the corresponding

effects: we thus have a

coupling of irreversible phenomena; the example of a

thermocouple is well known. Phenomena of different tensorial order do not mutually

interact (Curie’s principle). The interested reader should refer to the specialized

literature ([DEG 62], [BYU 02], [PRI 68]).

Consider two sub-systems

p and q between which scalar quantities X can be

exchanged. This exchange between the two sub-systems is assumed to be

independent of other sub-systems, which is the case when exchanges only occur via

direct contact, action at a distance not being possible. The irreversible evolution

which occurs takes the form of a flux,

dtdX

qip,

between the two sub-systems, and

is caused by the existence of an imbalance characterized by the different values

and

of all the intensive entropic quantities of the two sub-systems (j = 1,..., I).

We have a

relation between the causes Z

and Z

and the effects (the fluxes)

whose general form can be written as:



IjiZZF

jqjpi

qip

,...,1,,

The principle of action and reaction and the condition of zero flux at thermostatic

equilibrium

)(

jejqjp

ZZZ

can be represented by the relations:













0,;,, 

jejeijpjqijqjpi

ZZFZZFZZF

From this we can deduce a property of the derivatives of

which will be used in

the following section:

 

jpjq

jqjp



1.4.2.6.2.

Linear thermodynamics

Provided the degree of thermodynamic imbalance is reasonably small, it is

possible to perform a limited Taylor expansion of the functions





jqjpi

ZZF ,

in the

42 Fundamentals of Fluid Mechanics and Transport Phenomena

vicinity of the point ,( 1,..., )

Zie I , of thermodynamic equilibrium. Taking into

account the above equality we have:

 



IjiZZoZZ

ZZZZF

jqjpieie

jqjpjqjpi

,...,1,,,, 



In the preceding relations,

 

ieie

iij

ZL ,

is a square matrix of

order

I, the properties of which cannot be obtained via thermodynamic

considerations. Only an experiment or a suitably adapted theory can provide these

laws, which we here qualify as

phenomenological laws.

Statistical reasoning shows that this matrix is often symmetric (Onsager

relations).

This symmetry is no longer maintained if other intensive variables are

used in place of intensive entropic variables.

As long as the preceding approximation is valid, we say that we are dealing with

linear thermodynamics of irreversible phenomena. We thus have, for each

thermodynamic flux of an extensive quantity:



jqjpieij

qip

ZZZL



[1.39]

Internal entropy source [1.36], which exists at the interface of the two sub-

systems considered, becomes:

 



njiZZZZZL

iieij

,....2,1,

2121

int



¦¦

[1.40]

This internal entropy source is thus a

positive quadratic form whose principal

diagonal elements are all positive (



eii

ZL ).

We can also perform a change of variables which involves expressing the

intensive entropic variables as a function of other thermodynamic variables, for

example the

energetic intensive variables. Consider two sub-systems 1 and 2. The

differences



21 ii

ZZ  are generally linear functions (to second order excepted) of

the differences between the new variables which are chosen.

For example, with the intensive entropic variable

T1 which is associated with

energy, we obtain: