Parinov I.A. Microstructure and Properties of High-Temperature Superconductors
Подождите немного. Документ загружается.

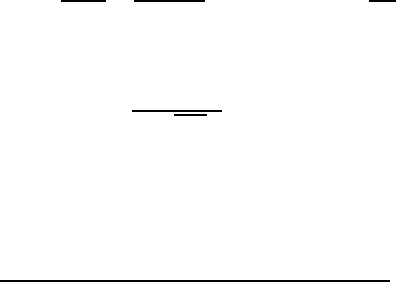
8.2 Twinning Processes in Ferroelastics and Ferroelectrics 381
fracture resistance, which are intrinsic for the material structure, and defines
essentially true strength properties. In the framework of the force approach
by using the SIF for given fracture mode (I, II, III or their combination), the
constitutive relationships, defining equilibrium states of crack growth at the
pointed stages of its propagation, have the form [163]:
dK/dc>dK
c
/dc for unstable fracture ; (8.71)
dK/dc<dK
c
/dc for stable fracture , (8.72)
where c is the length of the growing crack; K
c
is the critical value of SIF,
defining the crack start (i.e., the ceramic fracture toughness). If there are
internal mechanisms, contributing in the change of the crack growth, then
effective driving force, acting in the crack tip (K), can be presented as a sum
of applied external load (K
a
) and internal residual stresses (K
i
):
K = K
a
+
#
i
K
i
. (8.73)
The terms of K
i
in (8.73) can interpret the shielding and anti-shielding
(leading to the crack amplification) influences on transmission of external
stresses to the crack tip depending on their signs (i.e., negative or positive,
respectively). In particular, the microcracking near macrocrack, crack branch-
ing and crack bridging, studied in Sect. 7.1, lead to the shielding effects. At
the same time, the microcrack–macrocrack coalescence causes a decreasing of
fracture resistance (the anti-shielding effect).
Now, consider directly the toughening of HTSC ceramic, caused by the
processes of twinning near growing crack. In this case, the hydrostatic com-
ponent of stress, required to introduce the transformation, is obtained from
the condition ΔΦ ≤ 0 and can be written using (8.67) as
p
A
ΔV ≥−ΔF
0
+
6ΔΓ
i
ηd
+
(η
2
− 1)
η
2
d
Γ
t
+0.14EΔV
2
+
0.4
η
Gγ
2
T
. (8.74)
The hydrostatic stress near the crack tip is subjected to the non-equality
[261]:
p
A
≤
2K(1 + ν)
3
√
2πr
cos(Θ/2) , (8.75)
where {r, Θ} are the polar coordinates connected with the crack tip. Then,
using non-equalities (8.74) and (8.75), we obtain the distance of r
∗
c
from the
crack tip at which single grain will be subjected to the transformation. We
have for ν =0.2:
2πr
∗
c
=
0.8KΔVdη
2
η
2
c
cos(Θ/2)
[2(3ΔΓ
i
+0.2Gγ
2
T
d)ηη
c
− Γ
t
(η
c
+ η)](η
c
− η)
2
. (8.76)
Then, the size of the transformation zone, r
c
, can be calculated, using
(8.76) at K = K
c
and maximum value of the reached toughness (for Θ ≈ π/3)

382 8 Computer Simulation of HTSC Microstructure and Toughening
[262, 668]. The latter suggests that each particle is subjected to sufficient
shear stress, which introduces the martensitic transformation under condition
of thermodynamic limit (8.68) (at p
A
= 0), superimposed on the particle.
Note that the value of the angle Θ ≈ π/3 divides the zones of the toughening
and the crack amplification near the crack tip. The transformed particles at
the crack front increase K
tip
(the stress concentration at the crack tip), while
the particles disposing into the region, |Θ| >π/3, lead to the opposite effect.
Finally, consider the toughening, increasing fracture resistance of HTSC
ceramics due to twinning in the process zone with width 2r
c
, surrounding the
advancing crack (see Fig. 8.26). Taking into account the superconductor be-
havior, caused by the hysteresis curve “σ–ε”, we can estimate the toughening
for the steady-state crack, caused by the twinning as [102, 192]
K
SS
=
(K
0
c
)
2
+ EΔJ
SS
, (8.77)
where ΔJ
SS
=2
r
c
(
0
U(y)dy is the increment of fracture resistance; U is the
square under the curve “σ–ε”,calculated at each value of y-coordinate on
1
23
1
(a)
1.0
0.5
0.0
0
π/3 2π/3 πθ
θ
ΔK
|ΔK*|
(b)
r
c
r
Fig. 8.26. Effect of transformation zone, forming near crack (1), on ceramic tough-
ening: (a) frontal (2) and wake (3) zones of toughening (the pointed parameters
are discussed in text); (b) fracture toughness change, connected with disposition
of transformed particle; ΔK is the SIF difference due to total development of the
transformation zone, that is, at great values of Δc/r
c
[262]

8.2 Twinning Processes in Ferroelastics and Ferroelectrics 383
the basis of the governing law for the material subject to the twinning.
Equation (8.77) is reduced to the following form for one-axis curve “σ–ε” [192]:
K
SS
K
0
c
=
1+
(E − H)
H
3
8π
ln
r
c
d
−
σ
0
K
0
c
2
r
c
1/2
, (8.78)
where K
SS
is the fracture toughness of the superconductor for the steady-
state crack; K
0
c
=(2γ
0
E)
1/2
is the ceramic toughness without the twinning
process; γ
0
is the fracture energy; H is the hardness factor of the ceramic
at indentation; σ
0
is the threshold stress, stating a transition to non-elastic
material behavior due to nucleation of the twinning near the crack tip.
In order to obtain numerical results, we use the known parameters for
YBa
2
Cu
3
O
7−x
,namely:G =41GPa,γ
T
∼ S
0
=0.018,Γ
t
∼ σ
d
=0.01 J/m
2
[261]. In more detail, we discuss a selection of the values for the volumetric
deformation, ΔV , and the threshold stress, σ
0
(∼ P
V
). As has been shown
in [344], the spontaneous (non-elastic) deformation initiates at temperature
T
S
= 920 K, resulting in the structural phase transition from tetragonal phase
(P 4/mmm) to orthorhombic (P mmm). This deformation is defined by the
components C
22
,C
23
and C
55
of the elastic stiffness matrix. Below the tem-
perature of T
S
(into orthorhombic phase), the formed domain structure of
HTSC can re-switch from one spontaneous deformed oriented state to the
other by external shear load, σ. The shear modulus attains minimum value
for σ = σ
c
(where σ
c
is the coercive stress), because the number of twins
is maximum in this case, and the sample is most compliant to mechanical
loading. The number of twins decreases at σ>σ
c
, leading to greater stiffness
of the sample and to increasing of G. Due to this, we select as the thresh-
old value the next one: σ
0
= σ
c
. The presented temperature dependencies of
σ
c
[344] in the vicinity of the phase transition give the value of σ
c
≈ 3MPa.
This is much less than the corresponding value of the threshold stress, causing
the twinning (20 MPa) that is calculated in [578] for BaTiO
3
samples, tested
on impact load at different rates of the loading, and also than the value for
partially stabilized ZrO
2
(4 GPa) [863]. Finally, the spontaneous deformation
jump at the phase transition, we suggest, is equal to ΔV =10
−4
, according
to experimental results of [344]. This is also much less than the corresponding
values for BaTiO
3
(54 × 10
−4
) [849] and for ZrO
2
(57 × 10
−3
) [863].
Substitute the necessary values in (8.68) (at p
A
= 0), calculating the
preliminary values of the twin extent, d, from (8.69) for the observed grain
sizes of YBCO, D = 10–100 μm. The solution (8.68) for the above range of
the grain sizes leads to the value of η
c
→ 0. Then, from (8.76) and (8.77)
an absence of real toughening for HTSC ceramics, caused by the twinning
processes, is found. This is explained by very small magnitudes of ΔV and
σ
0
(∼ P
V
), defining a spontaneous strain in the YBCO, compared to the cor-
responding values for partially stabilized ZrO
2
[863] and ferroelectric ceramic
BaTiO
3
[578, 849], where the twinning processes play the most important
rule in material toughening. Thus, the toughening (or crack amplification)

384 8 Computer Simulation of HTSC Microstructure and Toughening
at the fracture of YBa
2
Cu
3
O
7−x
superconducting ceramic is caused by mi-
crocracking due to deformation or/and thermal anisotropy, and by the pro-
cesses, connected with microcracking (in particular crack branching and crack
bridging), but not transformations of the martensitic type.
8.3 Toughening Mechanisms for Large-Grain YBCO
8.3.1 Model Representations
Two main computer simulation approaches, namely Monte-Carlo simulations
[814, 821] and phase field method [937, 956, 1016], are usually used to study
microstructure transformations, which accompany the YBCO fabrication. The
phase field method has been discussed in Sect. 7.1.6. Here, we consider a modi-
fied Monte-Carlo scheme. The simulation of the YBCO precursor microstruc-
ture will be based on the re-crystallization model for ferroelectric ceramics
[516, 823] and on the study of thermal conductivity in mixes and structure-
heterogeneous solids [234]. Formation of the final YBCO microstructures, us-
ing seeds at existence of dispersed 211 phase, will be found by the Monte-Carlo
techniques [22, 1009, 1010].
A precursor microstructure after YBCO pellet modeling is represented by
discrete lattice with 2000 square cells of characteristic size δ. Each lattice cell
is assigned a number (between 1 and Q), corresponding to the orientation of
the grain in which one is embedded. All grains have different orientations,
defined during the pellet microstructure simulation, in which lesser numbers
correspond to earlier initiated crystallites. This liquidates a neighborhood of
the grain of the same orientation. Then, the cells which have neighbors with
unlike orientation lie at the grain boundary; in the other case, they are placed
in grains. The grain boundary energy is specified, defining an interaction be-
tween nearest neighboring lattice sites as [22, 1009, 1010]
E = −J
#
nn
(δ
S
i
S
j
− 1) , (8.79)
where S
i
is one of the Q orientations on site i(1 ≤ S
i
≤ Q),δ
ij
is the
Kronecker’s delta. The sum is taken over all nearest neighbor sites (nn), J is
a positive constant that sets the energy scale of the simulation. Note that the
orientation dependence of the energy of a straight boundary segment exhibits
a ratio of the maximum to minimum boundary energy of 2/
√
3 for triangular
lattice and
√
2 for square one. Both ratios are close to unity, that is, the grain
boundary energy is nearly isotropic and only weakly lattice dependent [22].
In simulation of boundary motion kinetics by the Monte-Carlo techniques,
a lattice cell is selected at random, and a new trial orientation is randomly
changed to one of the other grainorientations. Then, the energy alteration
8.3 Toughening Mechanisms for Large-Grain YBCO 385
caused by change in orientation (ΔE) is evaluated. The transition probability
(P ) is given by [22]
P =
exp(−ΔE/k
B
T )ΔE>0
1ΔE ≤ 0 ,
(8.80)
where k
B
is Boltzmann constant, T is the temperature. A re-orientation of
a site at a grain boundary corresponds to boundary migration. A boundary
segment moves with rate, related to the local chemical potential difference
(ΔE
i
) as [22]
v
i
= C[1 − exp(−ΔE
i
/k
B
T )] , (8.81)
where factor C is found by the boundary mobility and symmetry of the lattice.
The simulations for the cases of T ≈ 0andT ≈ T
m
,whereT
m
is the melting
point, have shown similar results [1009]; therefore, we use T ≈ 0, below.
The (N −N
p
) attempts of re-orientations, where N is the number of lattice
cells and N
p
is the total particle number, are arbitrarily used as unit of time
and defined as one Monte-Carlo step (MCS) per site. In order to incorporate
particles of 211 phase into the model, corresponding cells are assigned by ori-
entation which are distinct from all grain orientations. It is suggested that
the particle concentration and sites initially arbitrarily selected are fixed dur-
ing simulation and are independent from any attempts of cell re-orientation.
The insertion of the crystalline seed into microstructure is done by replacing
the grains at the center of the microstructure with one large square grain
with characteristic size considerably greater than the mean grain radius of
the YBCO precursor pellet.
In the computer simulations, we consider three cases of YBCO microstruc-
ture evolution after primary re-crystallization (see Fig. 8.27):
(1) particle dispersion of 211 phase into matrix of 123 phase;
(2) insertion of large grain (seed) into matrix of 123 phase;
(3) insertion of large grain (seed) into matrix of 123 phase with dispersed 211
phase.
77 77
77
77
7
64 64
64
47
47
1
1
15 15 15
15
151515
31 31
31
3
23 23
34
48
34
3434
34
77 77 77 9027 27
64 64 64
6464
64
47 47
47 47
47
52 1
111
1
11
115
15
31
23 23
23 23 23
23
77
34
34 34
48
48
4848
77
777777
7
64 64
64
47
47
47
1
1
15 15 15
151515
15 15
31
31 31
(c)(a) (b)
23 23
23
34
34
34
34
34343
48
Fig. 8.27. Three models of YBCO microstructure evolution, used in computer
simulations: (a) dispersion of 211 phase (black) in 123 matrix, (b) large-grain seed
(white) in 123 grain matrix and (c) 211 dispersion and grain-seed in 123 matrix
386 8 Computer Simulation of HTSC Microstructure and Toughening
As a condition of the computation stop in the first and third cases, an exis-
tence of one particle at any intergranular boundary at least (that corresponds
to complete pinning of microstructure with dispersed particles [1009, 1010]) is
selected. In the second case, the computations are finished when some grain
radius attains the seed grain size.
In order to obtain statistically representative results, we use approach
[145]. Then, using comparatively small grain aggregates together with stere-
ological method accelerates computations due to the operation with smaller
arrays of variables and obtains necessary statistics.
8.3.2 Effect of 211 Particles on YBCO Fracture
As has been shown in the previous chapters, a special effect on the 123 ma-
trix fracture may be exerted by dispersed inclusions of the 211 phase. The
platelet structure of 123 superconducting phase covering the 211 single inclu-
sions during solidification from melting via a zipper-like mechanism promotes
the formation at the 123/211 phase interface of the defects-faults and increased
dislocation concentration. Therefore, the existence of secondary phase inclu-
sions causes the alteration of the strength properties indirectly and directly.
In the first case, these inclusions influence formation of certain microstruc-
tures during material fabrication with corresponding fracture resistance. In
the second case, these inclusions define the acting toughening mechanisms,
fracture toughness and strain energy release rate. Therefore, we consider the
possible mechanisms of toughening in the case of the composite structure
YBa
2
Cu
3
O
7−x
/Y
2
BaCuO
5
.
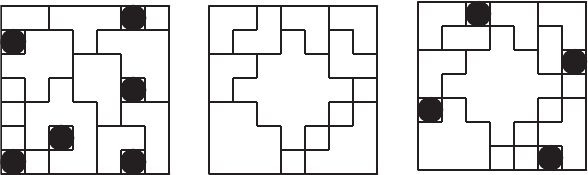
Θ
σ
σ
y
z
x
(a)
y
z
x
Φ
σ
σ
(b)
Fig. 8.28. (a) Tilt and (b) twist of crack at its interaction with inclusion
8.3 Toughening Mechanisms for Large-Grain YBCO 387
Crack Deflection
As the 123/211 interface strength is less than YBCO matrix strength, a crack
deflection around dispersed particles (see Fig. 8.28) in the form of crack tilt
and twist is possible. Then the driving force of the crack, which orients ran-
domly to the main tension vector, is described by the local stress intensity
factors k
1
,k
2
and k
3
, corresponding to the opening, sliding and tearing frac-
ture modes. The crack driving force, governed by the strain energy release
rate, G, is found as [268]
EG = k
2
1
(1 − ν
2
)+k
2
2
(1 − ν
2
)+k
2
3
(1 + ν) , (8.82)
where E and ν are the elastic modules.
In the case of applied SIF of mode I, K
I
,thelocalSIFsofk
t
i
(i =1, 2, 3)
for tilted crack are given as [268]
k
t
1
= F
11
(Θ)K
I
; k
t
2
= F
21
(Θ)K
I
, (8.83)
where
F
11
(Θ)=cos
3
(Θ/2) ; F
21
(Θ)=sin(Θ/2) cos
2
(Θ/2) . (8.84)
For twisted crack the local SIFs of k
T
i
are found by [268]
k
T
1
= F
11
(Φ)k
t
1
+ F
12
(Φ)k
t
2
; k
T
3
= F
31
(Φ)k
t
1
+ F
32
(Φ)k
t
2
, (8.85)
where
F
11
(Φ)=cos
4
(Θ/2)[2ν sin
2
Φ +cos
2
(Θ/2) cos
2
Φ];
F
12
(Φ)=sin
2
(Θ/2) cos
2
(Θ/2)[2ν sin
2
Φ +3cos
2
(Θ/2) cos
2
Φ];
F
31
(Φ)=cos
4
(Θ/2) sin Φ cos Φ[cos
2
(Θ/2) − 2ν];
F
32
(Φ)=sin
2
(Θ/2) cos
2
(Θ/2) sin Φ cos Φ[3 cos
2
(Θ/2) − 2ν] . (8.86)
The computation of toughening due to crack deflection is the statistical
problem, which is connected with averaging of the driving force in all possible
tilt and twist angles. The effect of particle volume fraction, V
f
, which causes
an initial crack path alteration, is equal to [268]
G
c
/G
m
c
=1+0.87V
f
, (8.87)
where G
c
and G
m
c
are the fracture toughness of composite and matrix, respec-
tively. The inclusions with higher characteristic ratio have stronger effects,
that is, spherical particles create a lesser toughening compared with disk-
shaped and rod-shaped ones. Toughening effect attains saturation at V
f
≈ 0.2.
An increased dimension of loading generally causes the increase of crack driv-
ing force due to the complexity of stress state and decrease of toughening
effects.

388 8 Computer Simulation of HTSC Microstructure and Toughening
Crack Pinning by Particles
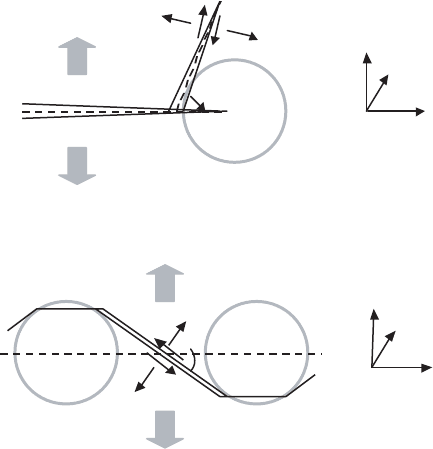
In the case of small-scale crack bridging in which the bridging zone size (L)is
small compared with the crack length, sample sizes and distance from crack
tip to the sample boundaries, the crack pinning by elastic particles with size
2a (see Fig. 8.29) is possible. Then, the toughening factor, Λ, may be found
as [101]
Λ ≡
K
c
/K
cm
ω(1 − c)
=
1+
πS
2
ac(1 − c
1/2
)
2K
2
cm
1/2
; ω =
E(1 − ν
2
m
)
E
m
(1 − ν
2
)
; c =(a/b)
2
,
(8.88)
where K
c
and K
cm
are the critical SIFs for crack growth in composite and in
matrix, respectively; S is the particle strength; b is the radius of penny-shaped
crack with central pinning particle; E and ν are effective elastic modules of
composite (E
m
and ν
m
are the elastic matrix constants). Thus, the toughening
is increased with inclusion size and strength.
Toughening due to Periodically Distributed Inclusions
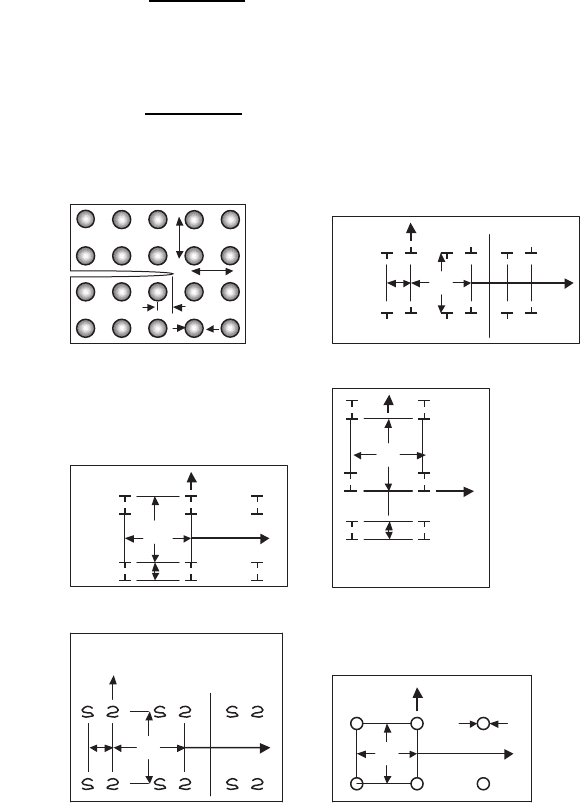
The internal stress state caused by periodically distributed inclusions is al-
most sinusoidal at the mean plains between inclusion layers, where a finite
crack is placed along the x-axis. Dislocation techniques have been applied to
Fig. 8.29. (a) Crack pinning by particles and (b) crack opening; ν is the crack
opening rate; σ
p
is the stress, loading the particle
8.3 Toughening Mechanisms for Large-Grain YBCO 389
inclusions, modeled by centers of shear, compression, anti-plane and extension
(dilatation) (see Fig. 8.30 [621]). These are the following results:
(1) For shear centers:
ΔK
II
=1.6
μV
f
e
T
xy
λ
1/2
(1 − ν)
; a<<λ; b
x
= he
T
xy
. (8.89)
(2) For horizontal compression centers:
ΔK
I
=1.6
μV
f
e
T
xx
λ
1/2
(1 − ν)
; a<<λ; b
x
= he
T
xx
. (8.90)
σ
yy
σ
xy
=
σ
yz
=
0
y
λ
x
2a
y
(d)
λ
x
a
σ
xx
σ
xy
=
0
σ
xz
=
0
λ
y
x
a
σ
xx
=
0
σ
xy
–σ
xy
σ
xz
=
0
σ
yy
=
0
σ
yz
=
0
(b)
x
a
λ
y
σ
xx
=
0
σ
xy
=
0
σ
xz
=
0
σ
yz
(f)
(e)
λ
y
x
a
σ
xy
=
0
σ
yz
=
0
σ
yy
(c)
(a)
x
λ
λ
2a
Fig. 8.30. (a) Crack toughening by periodical array of inclusions; dislocation struc-
tures (with Burgers vector, b), modeling the inclusions: (b)shearcenters;(c)hor-
izontal compression centers; (d) vertical compression centers; (e) anti-plane shear
centers and (f) extension (dilatation) centers

390 8 Computer Simulation of HTSC Microstructure and Toughening
(3) For vertical compression centers:
ΔK
I
=2.2
μV
f
e
T
xx
λ
1/2
(1 − ν)
; a<<λ; b
x
= he
T
xx
. (8.91)
(4) For anti-plane shear centers:
ΔK
III
=0.4μV
f
e
T
yz
λ
1/2
; a<<λ; b
x
= he
T
yz
. (8.92)
(5) For extension (dilatation) centers:
ΔK
I
=0.8
EV
f
e
T
λ
1/2
(1 − 2ν)
; a<<λ; e
T
=ΔV/V . (8.93)
Thus, the SIF difference of corresponding mode for periodically distributed
inclusions is proportional to the elastic modulus, volume fraction of the inclu-
sions, deformation mismatches (e
T
αβ
) and square root of the inclusion spacing
(λ). However, it is independent of the crack length, that is, the dispersion
toughening is more effective at the crack initiation to compare with its growth.
8.3.3 Some Numerical Results
The numerical results presented in Table 8.7 [822] have been found for some
microstructure properties in all possible variants that are found by the 211
particle concentration (f =0.1 and 0.2) and the seed size (S
S
≈ 2S
m
0
and
3S
m
0
,whereS
S
is the seed area and S
m
0
is the maximum grain square after
primary re-crystallization before beginning of the 123 grain growth). The final
microstructures with the dispersed 211 particles demonstrate dependencies
similar to
¯
S ≈ 4a/f,where
¯
S is the mean pinned grain area, a is the particle
square. This corresponds to the topological ideas developed in the computer
simulations of the grain pinning by dispersed particles [1009]. The behavior of
outstripping grain growth around seed coincides well with the computational
results of large grain growth in the microstructure of normal grain growth
[1010] and the growth morphology of YBCO specimens with seed, solidified at
unfavorable temperatures [640]. Moreover, the present computer simulations
may be characteristic to the pushing process of the 211 particles due to 123
front advancing [640]. The numerical results show a correlation between the
grain area,
¯
S, and superconducting field per grain,
¯
S
SR
.
Table 8.7. Numerical results
Para-
meters
f =0.0,
S
S
≈ 2S
m
0
f =0.0,
S
S
≈ 3S
m
0
f =0.1,
S
S
=0
f =0.1,
S
S
≈ 2S
m
0
f =0.1,
S
S
≈ 3S
m
0
f =0.2,
S
S
=0
f =0.2,
S
S
≈ 2S
m
0
f =0.2,
S
S
≈ 3S
m
0
¯
S/δ
2
13.89 23.81 45.45 50.03 62.50 25.01 29.41 31.25
¯
S
SR
/δ
2
13.89 23.81 40.91 45.08 56.25 20.05 23.53 25.00
