Orton C., Tyers P., Vince A. Pottery in archaeology

Подождите немного. Документ загружается.

Seriation

189

Bringing the evidence together

Associations between pottery and other datable artefacts provide another

potential source of evidence. Coins are probably the most common class of

such objects and (when they can be dated reliably) certainly provide a

terminus

post quern for the deposition of an assemblage and hence the final

deposition of any pottery it contains. However, the dating of archaeological

levels from coins is not without difficulty and such data must be used with

great caution.

The key point is the degree of association between a pot and its external

dating evidence. An example of tight association is that of a coin hoard

buried in a pot. Unless an heirloom has been used, or the hoard represents

savings accumulated over a long period in the same pot, there

likely to be a

very close agreement between the date of the pot and that of the hoard, and

such evidence can be very valuable (Bird and Turner 1974). On the other

hand, the casual association of a pot, or perhaps just a sherd, with a coin in a

soil horizon is not likely to give useful information. Either or both may be

residual (p. 168), perhaps by hundreds of years.

Between these two extremes comes the association of pottery with dated

structures. Sometimes a close association can be argued. For example, in the

case of the London waterfronts (p. 168), the stability of the dated timber

structures relied on the infilling dumps behind them, which must therefore be

contemporary. The question that remains is - how long was the pottery

deposited as rubbish (for example in a midden) before that dump was used to

infill

the waterfront? Here comparison of the wares present, together with the

degree of breakage (p. 178) and of abrasion (p. 214) can help us. We can

contrast this situation with one in which the

filling

may have occurred slowly

over a long period. For example, a documented date for the digging of a

castle moat may tell us little (except a

terminus

post

quern)

of the date of the

pottery found in that moat.

Problems with ceramic dates certainly arise when the independently-dated

elements are sparse and greater reliance is placed on chains of associations

where little or no dating evidence is available. The danger of circular argu-

ment becomes acute, perhaps particularly when fine chronological divisions

are being attempted. In addition, assumptions (often unstated) are usually

made about factors such as the residuality of the material, which should more

properly appear on a list of questions to be answered.

Seriation

more formal approach is to try to order assemblages of types of pottery on

the basis of the co-occurrences either of types in assemblages or of character-

istics on types, the former being the more common. Assemblages which are

similar to each other, in terms of the types present in them, may be close

chronologically; conversely, types which commonly co-occur should have

190 Chronology

broadly similar date-ranges. The occurrence of types in assemblages can be

described either in terms of their presence/absence, as used originally by

Petrie (p. 11), or in terms of proportions using an appropriate measure

(p. 168).

The principal technique for simultaneously ordering assemblages and

pottery types is seriation. We have already seen how such techniques devel-

oped out of Petrie's work at the end of the nineteenth century. Whether

performed manually or by a computer program, the aim is to recreate as far

as possible for each type the ideal pattern described above, while using as

much or as little additional information (for example stratigraphy) as may be

available. The basic idea is contained in Petrie's Concentration Principle:'if

the typology is 'chronologically significant', and when the graves have been

correctly ordered, then the 'sequence-date'-rawges for the individual types

will be found to have been individually or in some communal way minimised.'

(Kendall 1971, 217), although it should be remembered that Petrie's work

was on presence/absence, not quantified, data.

The underlying assumption is that the use of any type of pottery follows a

regular pattern of not in use—»increasing use—»steady use—»decreasing

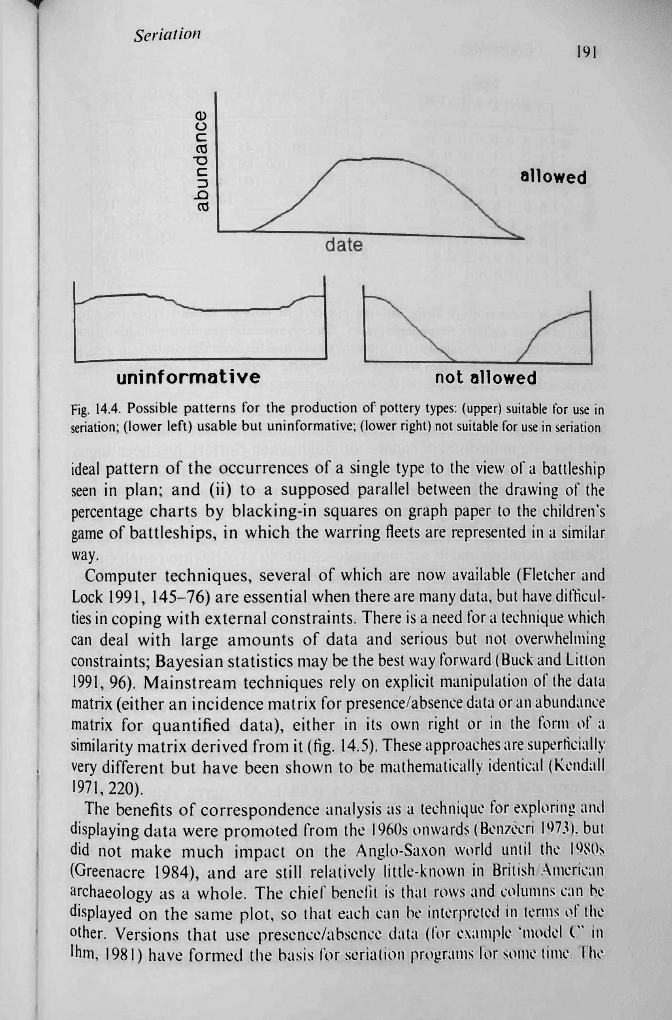
use—»no longer in use. The exact shape of this pattern is not critical, provided

there are no catastrophic collapses in usage. It does not matter if a type is

already in use at the beginning of a period to be studied, or is still in use at the

end, although a type which meets both of these conditions will be of little use

in establishing a chronology and is probably best omitted from such an

analysis (fig. 14.4). It is not necessary to assume that the chronological

distribution of a type follows a normal distribution, although there may be

superficial similarities.

All techniques rely, explicitly or implicitly, on the setting up of a data

matrix in which (usually) the columns represent types and the rows represent

assemblages. This arrangement is purely conventional, and may reflect the

need for some computer programs to work with more rows than columns

(there are usually more assemblages than types). The rows and columns are

then re-ordered (or sorted) until the required pattern emerges (if indeed it was

inherent in the data in the first place, which it may not have been). This is a

trial-and-error approach, and there is no reason why it should have a single

'best' order, or if there is, that we should necessarily find it. The option of

trying every permutation of rows and columns to see if it is the 'best' is not

open to us - the number of possible permutations is so vast that we do not

have 'world enough and time' (Marvell, To his coy mistress).

Manual techniques, such as the 'battleship curve' method (Ford 1962) and

the close-proximity method (Renfrew and Sterud 1969) are best used when

either the amount of data is small or when there are many external constraints

(such as stratigraphy). The term 'battleship curve' has caused some puzzle-

ment as to its origins. We have been told it is due to: (i) the similarity of the

Sedation

191

uninformative not allowed

Fig. 14.4. Possible patterns for the production of pottery types: (upper) suitable for use in

seriation; (lower left) usable but uninformative; (lower right) not suitable for use in seriation

ideal pattern of the occurrences of a single type to the view of a battleship

seen in plan; and (ii) to a supposed parallel between the drawing of the

percentage charts by blacking-in squares on graph paper to the children's

game of battleships, in which the warring fleets are represented in a similar

way.

Computer techniques, several of which are now available (Fletcher and

Lock 1991,145-76) are essential when there are many data, but have

difficul-

ties

in coping with external constraints. There is a need for a technique which

can deal with large amounts of data and serious but not overwhelming

constraints; Bayesian statistics may be the best way forward (Buck and Litton

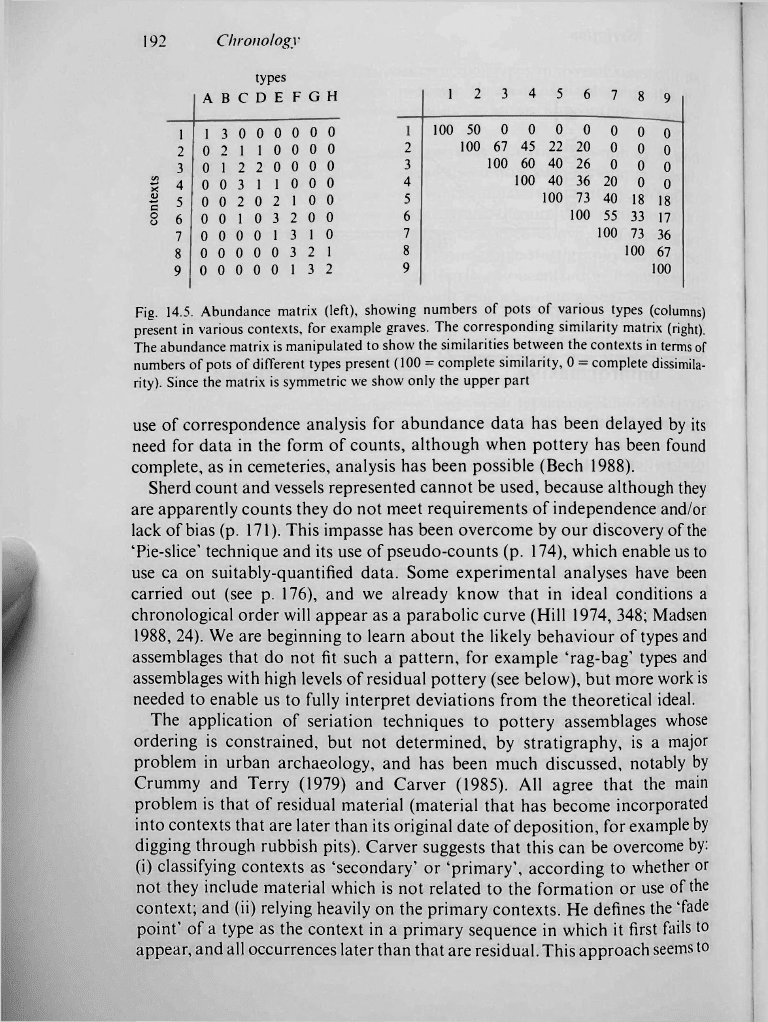
1991, 96). Mainstream techniques rely on explicit manipulation of the data

matrix (either an incidence matrix for presence/absence data or an abundance

matrix for quantified data), either in its own right or in the form of a

similarity matrix derived from it

(fig.

14.5). These approaches are superficially

I very different but have been shown to be mathematically identical (Kendall

1971,, 220).

The benefits of correspondence analysis as a technique for exploring and

displaying data were promoted from the 1960s onwards (Benzecri 1973), but

did not make much impact on the Anglo-Saxon world until the 1980s

(Greenacre 1984), and are still relatively little-known in British/American

archaeology as a whole. The chief benefit is that rows and columns can be

displayed on the same plot, so that each can be interpreted in terms of the

other. Versions that use presence/absence data (for example 'model C in

Ihm, 1981) have formed the basis for seriation programs lor some time. The

allowed

192 Chronology

types

ABCDEFGH 1 2 3 4 5 6 7 8 9

| 5 00202100

8 6 00103200

7 0 0 0 0 1 3 1 0

8 00000321

9 0 0 0 0 0 1 3 2

| 4 00311000

1 1 3 0 0 0 0 0 0

2 0 2 1 1 0 0 0 0

3 0 1 2 2 0 0 0 0

100 50 0 0 0 0 0 0 0

100 67 45 22 20 0 0 Q

100 60 40 26 0 0 0

100 40 36 20 0 0

100 73 40 18 18

100 55 33 17

100 73 36

100 67

100

Fig. 14.5. Abundance matrix (left), showing numbers of pots of various types (columns)

present in various contexts, for example graves. The corresponding similarity matrix (right).

The abundance matrix is manipulated to show the similarities between the contexts in terms of

numbers of pots of different types present (100 = complete similarity, 0 = complete dissimila-

rity). Since the matrix is symmetric we show only the upper part

use of correspondence analysis for abundance data has been delayed by its

need for data in the form of counts, although when pottery has been found

complete, as in cemeteries, analysis has been possible (Bech 1988).

Sherd count and vessels represented cannot be used, because although they

are apparently counts they do not meet requirements of independence and/or

lack of bias (p. 171). This impasse has been overcome by our discovery of the

'Pie-slice' technique and its use of pseudo-counts (p. 174), which enable us to

use ca on suitably-quantified data. Some experimental analyses have been

carried out (see p. 176), and we already know that in ideal conditions a

chronological order will appear as a parabolic curve (Hill 1974, 348; Madsen

1988, 24). We are beginning to learn about the likely behaviour of types and

assemblages that do not fit such a pattern, for example 'rag-bag' types and

assemblages with high levels of residual pottery (see below), but more work is

needed to enable us to fully interpret deviations from the theoretical ideal.

The application of seriation techniques to pottery assemblages whose

ordering is constrained, but not determined, by stratigraphy, is a major

problem in urban archaeology, and has been much discussed, notably by

Crummy and Terry (1979) and Carver (1985). All agree that the main

problem is that of residual material (material that has become incorporated

into contexts that are later than its original date of deposition, for example by

digging through rubbish pits). Carver suggests that this can be overcome by:

(i) classifying contexts as 'secondary' or 'primary', according to whether or

not they include material which is not related to the formation or use of the

context; and (ii) relying heavily on the primary contexts. He defines the 'fade

point' of a type as the context in a primary sequence in which it first fails to

appear, and all occurrences later than that are residual. This approach seems to

s ТгТ

Я os

їй о

а —

2-а

йО

U -О

СЯ

!•»

I s

00 _c

s »

'та

о о

с л

8 g

'S g

jö й

a «s

Й s

a»

-sr" ,S5

Чиарроу iBJauaj)

jatïnoa S

194 Chronology

be at risk of circular argument, unless one can be sure which contexts are

primary, and it fails to tackle the problem of sample size and the functional

variation between assemblages from primary contexts. Crummy and Terry

(1979, 54-5) make some suggestions for what they call 'class V contexts* but

say that they will be few on an urban site, while Millett and Graham (1986,9)

admit that they could not distinguish such contexts. We prefer an approach

based on comparison of the parameters of different types in an assemblage,

for example their brokenness and state of abrasion (p. 214).

Carver (1985, 360) goes on to discuss how the shape of the seriation

diagram (or

seriography

fig. 14.6; see Perrin 1990) can be interpreted in terms

of the site's development, with characteristics corresponding to steady and

intense occupation, levelling (removal of deposits) and dumping (addition of

deposits). While valuable, this seems to contain implicit assumptions about

the regularity of the introduction of new types, and should be used with

caution.

The ordering generated by automatic seriation need not necessarily be

chronological; other factors such as geographical proximity, function or

status may generate a similar pattern in the data. As always, an archaeo-

logical interpretation of the analysis must be made. Even if a chronological

pattern is suspected, neither the direction nor the rate of passing of time can

be deduced from the table alone. Chronological markets (such as the intro-

duction of new types) cannot be assumed to occur at regular intervals. In

either case, the formal study needs to be combined with understanding of the

structure of pottery supply, which in turn relies on the combination of

provenance studies and quantified data.

Meanwhile, research continues on more analytical approaches to seriation

(Laxton 1976; 1987; Laxton and Restorick 1989). It seems likely that a

combination of the two approaches, the exploratory and the more analytical,

will be used in future.

A case study

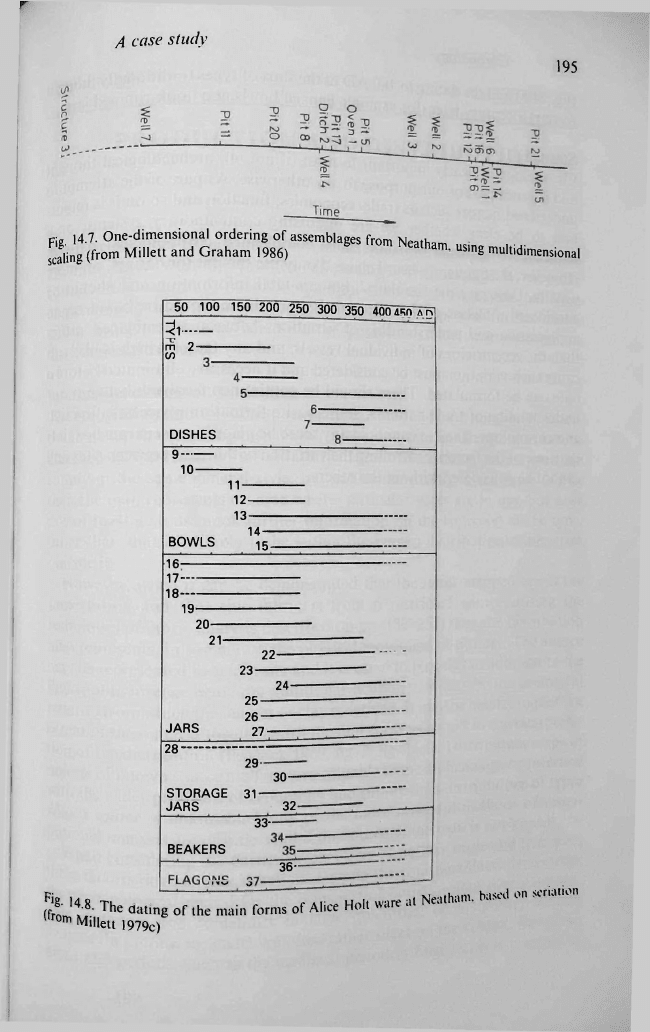
We use Millett's (1979c) study of twenty-two stratified pottery assemblages

dating from c. AD 75 to the fifth century, from the Romano-British small

town of Neatham, Hampshire (Millett and Graham 1986). Using counts of

rim sherds, he defined similarity coefficients between pairs of assemblages

and used multidimensional scaling (mdscal; see Doran and Hodson 1975,

213-16) to produce a one-dimensional ordering (fig. 14.7). The problem of

residual pottery was noted, with the comment that it may have made some

early types appear to have continued later than they really did. In our view,

the converse is also possible: the presence of a late type in an assemblage of

mainly residual material could make it appear to start earlier than it really

did. This might account for the apparent paucity of new types after

200 AD

A case study

ig. 14 7 One-dimensional ordering of assemblages from Neatham

scaling (from Millett and Graham 1986) Neatham,

195

using multidimensional

50 100 150 2Off 250 300 3b0 400dsn at?

m 2-

DISHES

9——

11-

12-

13-

BOWLS

14-

15-

16—

1 7

1 8

19"-

20-

21-

22-

23-

24-

JARS

25-

26-

27-

29-

30-

STORAGE 31-

JARS

32-

33-

BEAKERS

36-

fa'

The

of,he S

forms

Alice

Ho.,

ware a,

|gg —

wrom Vfjii , _

Millett 1979c)

196 Chronology

(fig. 14.8) and the dating to 180 AD of the start of types traditionally thought

to start a century later (for example flanged bowls and hook-rimmed jars).

Summary

Chronology is clearly important to most (if not all) archaeological thought

and it is not part of our purpose to say otherwise. As part of the attempt to

understand factors such as trade, economics, function and so on it is impor-

tant to be clear whether we are discussing contemporary systems, or a

sequence of systems, or some more complicated chronological pattern.

However, the frequently-heard claim 'I only use the pot for dating', or, more

usually, 'Just give me the dates', ignores vital information and should be

considered a misuse of the material. Pottery dates should be based on an

appreciation and understanding of variations between assemblages, rather

than the eccentricities of individual vessels, and any factor which is likely to

cause such variation must be considered and if necessary eliminated before a

date can be formulated. There should be continuous feedback between our

understanding of trade-patterns, sources, site formation processes, function

and chronology. The last cannot in any sense be placed in a separate box; it

only one of the factors controlling the variation within and between sites and

cannot be considered without the others.

PRODUCTION AND DISTRIBUTION

When we consider the significance of an assemblage from a particular site we

are then faced with the problem of placing the assemblage in its wider

context. It is natural to try to compare the site's assemblage with that from

contemporary sites in the immediate area, and perhaps also further afield,

which brings us face-to-face with the problems of the interpretation of

distributional evidence.

Information content of distribution

The recognition that the same pottery types are found on a number of

sites

the first step in compiling distribution maps, but the significance that can be

attached to the result is not always the same. When the types mapped are

simply in the same general style, but not demonstrably in the same fabric,

then the map represents an area where particular types are in use, but does

not of itself give us much further information on the source(s) of the type,

other than that it is likely to be within the known distribution rather than

outside it.

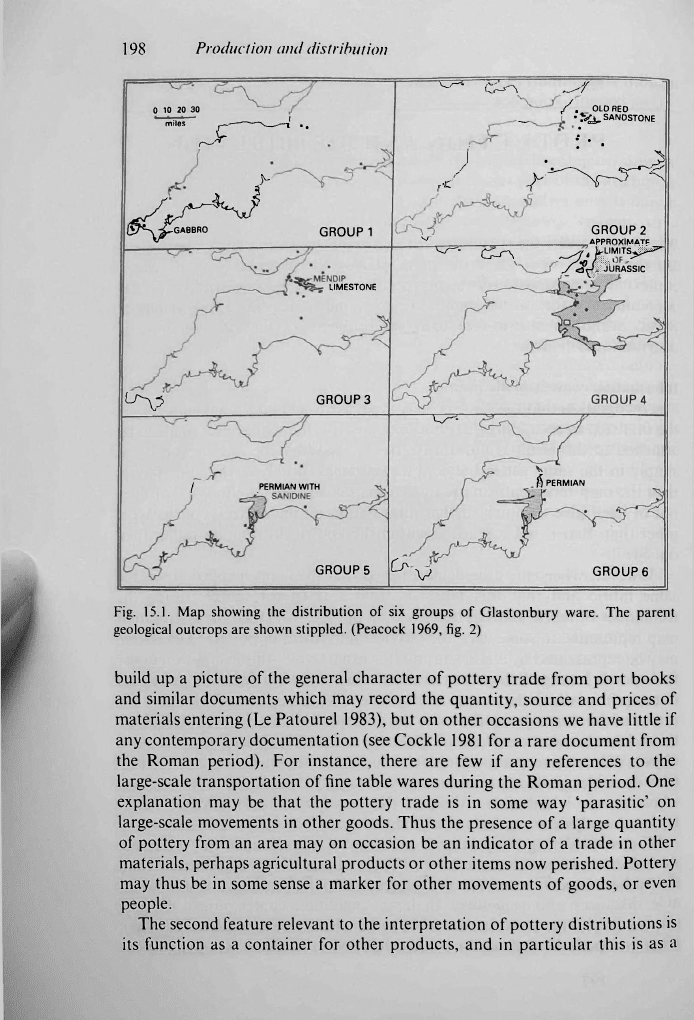
However, when it can be demonstrated that the items mapped are in the

same fabric and that this fabric is from a restricted source, (using the

techniques of fabric analysis described on pp. 132-151) then the distribution

map represents, in some way, the physical movement of

pottery.

The source

may be represented by a kiln site and it is usual to plot this in addition to the

find spot (a lozenge being the traditional symbol) or it may be the geological

outcrop from which the temper or clay is derived. It may be

helpful

to plot the

extent of the outcrop together with the find spots as an aid to the interpreta-

tion of the distribution (Peacock 1969, fig. 2; fig. 15.1). Distribution maps of

objects of known source are far more valuable to the archaeologist concerned

with the wider problems of economics and supply than distributions of types

whose source is unknown. Many of the more powerful methods of distri-

butional analysis are of little avail if the source of the item is not known.

When considering the particular problems of pottery trade and transport

three factors deserve consideration. Despite the considerable evidence from

the archaeological record for the transport of pottery, often over consider-

able distances and sometimes in large quantities, contemporary written

sources (in literate societies) are often rather silent on the subject. For some

areas and periods, such as the medieval period in England, it is possible to

197

198 Production and distribution

0 10 20 30

miles j

rJf

ypf^S

P^aii GROUP 1

^ С^л - -j

/. OLD RED

—'^SANDSTONE

f • .

J -J^^

GROUP 2

Ш APPRnVIMATB

UMESTONE

УЩф GROUP 3

JgSg ^STl ^t'MITS^^T

y^P^&RASSIC

PERMIAN WITH

GROUP 5

V*-

PERMIAN

^V GROUP 6

Fig. 15.1. Map showing the distribution of six groups of Glastonbury ware. The parent

geological outcrops are shown stippled. (Peacock 1969, fig. 2)

build up a picture of the general character of pottery trade from port books

and similar documents which may record the quantity, source and prices of

materials entering (Le Patourel 1983), but on other occasions we have little if

any contemporary documentation (see Cockle 1981 for a rare document from

the Roman period). For instance, there are few if any references to the

large-scale transportation of fine table wares during the Roman period. One

explanation may be that the pottery trade is in some way 'parasitic' on

large-scale movements in other goods. Thus the presence of a large quantity

of pottery from an area may on occasion be an indicator of a trade in other

materials, perhaps agricultural products or other items now perished. Pottery

may thus be in some sense a marker for other movements of goods, or even

people.

The second feature relevant to the interpretation of pottery distributions is

its function as a container for other products, and in particular this is as a