Nakahara M. Geometry, Topology and Physics
Подождите немного. Документ загружается.

Exercise 14.3. Show that δγ
2
γ
and δX
2
γ
are invariant under Diff(
g
) but not
under Weyl(
g
). This is the possible origin of conformal anomalies, see (14.84).
Before we proceed further, we need to clarify the overlap between Diff
0
(
g
)
and Weyl(
g
). Suppose δv ∈ ker P
1
,thatis,
P
1
δv =∇
α
δv
β
+∇
β
δv
α
− γ
αβ
(∇
γ
δv
γ
) = 0. (14.57)
We find, for such δv,thatδ
D
γ
αβ
= (∇
γ
δv
γ
)γ
αβ
. A vector δv ∈ ker P
1
is identified with the conformal Killing vector (CKV), see section 7.7. It is
important to note that δ
D
and δ
W
yield the same metric deformations if δφ is taken
to be ∇
γ
δv
γ
. Thus, the set of the CKVs is identified with the overlap between
Diff
0
(
g
) and Weyl(
g
). Let there be k independent CKVs on
g
and denote
these by
α
s
(1 ≤ s ≤ k). It is known from the theory of Riemann surfaces that
k =
6 g = 0
2 g = 1
0 g ≥ 2.
(14.58)
We separate δv into a part generated by the CKV, and its orthogonal complement,
which we write as
δv
α
= δ ˜v
α
+ δa
s
α
s
. (14.59)
The tangent vector δ X is also decomposed as
δ X = δ
˜
X + δ ˜v
α
∂
α
˜
X
µ
+ δa
s
α
s
∂
α
˜
X
µ
. (14.60)
The functional measures now become
δγ δ X → J d
n
δ t δφ δ ˜v d
k
δ a δ
˜
X (14.61)
where we noted that the t-anda-parameters are finite dimensional.
Let Diff
⊥
0
(
g
) be the subspace of Diff
0
(
g
), which is orthogonal to the
CKV. We have
V (Diff
0
) = V (Diff
⊥
0
) · V (CKV) (14.62)
V (Diff
0
∗ Weyl) = V (Diff
⊥
0
)V (Wey l)
= V (Diff
0
)V (Weyl)/V (CKV). (14.63)
Takeaslice ˆγ(t) of
g
. The slice is parametrized by n Teichm ¨uller
parameters. Any metric ˜γ related to ˆγ by G = Diff(
g
) ∗Weyl(
g
) is written as
˜γ = f
∗
(e
φ
ˆγ) f ∈ Diff(
g
), e
φ
∈ Weyl(
g
). (14.64)
We express a small deformation δ ˜γ at ˜γ as a pullback of a deformation δγ at
γ ≡ e
δφ
ˆγ : δ ˜γ = f
∗
(δγ ). Note that δγ is a small diffeomorphism at the origin

of Diff
0
(
g
) and, hence, can be described by a vector field δv.Aswasshown
in exercise 14.3, Diff(
g
) is the isometry of the relevant vector spaces. It then
follows that
δ ˜γ
2
˜γ
=f
∗
(δγ )
2
f
∗
γ
=δγ
2
γ
γ = e
φ
ˆγ. (14.65)
At the point γ , we decompose δγ as
δγ
αβ
= δφγ
αβ
+ (P
1
δ ˜v)
αβ
+ δt
i
T
iαβ
(14.66)
where δφ has been redefined so that it includes the trace parts of the Teichm¨uller
deformation and ∇
α
δv
β
+∇
β
δv
α
, see (14.51).
Exercise 14.4. Show that T
iαβ
at γ is related to
ˆ
T
iαβ
at ˆγ as
T
iαβ
= e
φ
ˆ
T
iαβ
. (14.67)
Now we are ready to give the explicit form of the measure. We first find the
Jacobian associated with the change
δv → δ ˜vd
k
δa.Wehave
1 =
δv exp(−
1
2
δv
2
γ
)
= J
δ ˜v d
k
δ a exp(−
1
2
δ ˜v
2
γ
−
1
2
δa
s
s
2
γ
)
= J [det(
s
,
r
)]
−1/2
(14.68a)
where
(
s
,
r
) =
d
2
ξ
√
γγ
αβ
α
s
β
r
.(14.68b)
[Remark: Although the matrix element (14.68b) is defined for γ = e
φ
ˆγ , we can
show that it is independent of e
φ
. To see this, let us take a CKV
ˆ
α
s
of the metric
ˆγ ;
ˆ
∇
α
ˆ
sβ
+
ˆ
∇
β
ˆ
sα
=ˆγ
αβ
ˆ
∇
ˆ
γ
s
,where
ˆ
∇ is the covariant derivative with respect
to ˆγ and
ˆ
sα
≡ˆγ
αβ
ˆ
β
s
. A simple calculation shows that
sα
= γ
αβ
ˆ
β
s
= e
φ
ˆ
sα
satisfies
∇
α
sβ
+∇
β
sα
= e
φ
(
ˆ
∇
α
ˆ
sβ
+
ˆ
∇
β
ˆ
sα
+ˆγ
αβ
γ
s
∂
γ
φ)
= e
φ
ˆγ
αβ
(
ˆ
∇
γ
γ
s
+
γ
s
∂
γ
φ) = γ
αβ
∇
γ
γ
s
∇ being the covariant derivative with respect to γ . Thus,
α
s
=
ˆ
α
s
is a CKV
of the metric γ = e
φ
ˆγ andtheCKVaretakentobeφ independent.] Equation
(14.68a) shows that
δv =[det(
r
,
s
)]
1/2
δ ˜v d
k
δ a. (14.69)
Now the total measure is written as
J [det(
r
,
s
)]
1/2
d
n
t δφ δ ˜v d
k
δ a δ
˜
X (14.70)
where J takes care of the rest of the variable changes.
The Jacobian J is now obtained from (14.60), (14.66), (14.70) and the
definition of the measures (14.56). We have
1 =
δγ δ X exp(−
1
2
||δγ ||
2
γ
−
1
2
||δ X ||
2
γ
)
= J det
1/2
(, )
d
n
δ t δ ˜v δφ d
k
δ a δ
˜
X
× exp
−
1
2
H
H
H
H
δφγ
αβ
+ (P
1
δ ˜v)
αβ
+ δt
i
∂γ
αβ
∂t
i
H
H
H
H
2
−
1
2
δ
˜
X + δ ˜v
α
∂
α
˜
X + δa
s
α
s
∂
α
˜
X
2
= J det
1/2
(, )
d
n
δ t δ ˜v...exp(−
1
2
MV
2
) (14.71)
where
V =
δt
δφ
δ ˜v
δa
δ
˜
X
M =
∂γ/∂t γ P
1
00
00∂
˜
X · ∂
˜
X 1
≡
A 0
CB
.
(14.72)
The matrix in the exponent of (14.71) is
M
†
M =
A
†
C
†
0 B
†
A 0
CB
=
A
†
A + C
†
CC
†
B
B
†
CB
†
B
=
I ∗
0 B
†
B
A
†
A 0
∗∗ I
(14.73)
where ∗ and ∗∗ are irrelevant. The last expression has been obtained from the
identity,
AB
CD
=
IB
0 D
A − BD
−1
C 0
D
−1
CI
.
The Gaussian integrals in (14.71) are readily evaluated to yield
1 = J det
1/2
(, ) det
−1/2
(M
†
M)
= J det
1/2
(, )[det(A
†
A) det(B
†
B)]
−1/2
. (14.74)
To compute det
1/2
(A
†
A), we need to evaluate δγ
2
γ
.Wehave
||δγ ||
2
γ
=
d
2
ξ
√
γ(G
αβγ δ
+ uγ
αβ
γ
γδ
)
×[δφγ
αβ
+ (P
1
δ ˜v)
αβ
+ δt
i
T
iαβ
][δφγ
γδ
+ (P
1
δ ˜v)
γδ
+ δt
j
T
j γδ
]
= 4uδφ
2
γ
+P
1
δ ˜v
2
+ δt
i
δt
j
(T
i
, T
j
) + 2δt
i
(P
1
δ ˜v, T
i
).
(14.75)
In general, T
i
is not orthogonal to P
1
δv. To separate T
i
into parts orthogonal to
P
1
δv and parallel to P
1
δv, we need to define the adjoint P
†
1
of P
1
. P
1
is an elliptic
operator which takes a vector field into a traceless symmetric tensor field. Thus,
P
†
1
maps symmetric traceless tensors to vectors. For a symmetric traceless tensor
δh,wehave
(P
1
δv, δh) =
d
2
ξ
√
γ G
αβγ δ
(P
1
δv)
αβ
δh
γδ
=
d
2
ξ
√
γ(∇
α
δv
β
+∇
β
δv
α
)δh
αβ
=
d
2
ξ
√
γδv
α
(−2∇
β
)δh
αβ
≡ (δv, P
†
1
δh)
where the inner product in the last expression is defined by (14.54c). Thus, it
follows that
(P
†
1
δh)
α
=−2∇
β
δh
αβ
. (14.76)
Suppose δh is orthogonal to P
1
δv. From the previous discussion, we have
(P
1
δv, δh) = (δv, P
†
1
δh) = 0. Since δv is arbitrary, δh must be an element
of ker P
†
1
, see figure 14.4. Now T
i
may be separated as
T
i
=
0
T
i
+
⊥
T
i
(14.77a)
where the projection operators
0
and
⊥
are defined by
0
≡ 1 − P
1
1
P
†
1
P
1
P
†
1
⊥
≡ P
1
1
P
†
1
P
1
P
†
1
.(14.77b)
It is easy to verify that
0
+
1
= 1,
0 ⊥
= 0, P
†
1
0
= 0, P
†
1
⊥
=
P
†
1
,
0
T
i
= T
i
and
⊥
T
i
= 0forT
i
∈ kerP
†
1
etc. Thus (14.77a) is an orthogonal
decomposition of T
i
. We write
⊥
T
i
= P
1
u
i
,where
u
i
=
1
P
†
1
P
1
P
†
1
T
i
.
Let {ψ
r
} (1 ≤ r ≤ n) be a real basis of ker P
†
1
, which is not necessarily
orthonormal. Then T
i
can be expanded as (figure 14.5)
T
i
=
r
ψ
r
Q
ri
+ P
1
u
i
. (14.78)
Taking an inner product between T
i
and ψ
r
,wefindthat
Q
ri
=
s
[(ψ, ψ)
−1
]
rs
(ψ
s
, T
i
). (14.79)
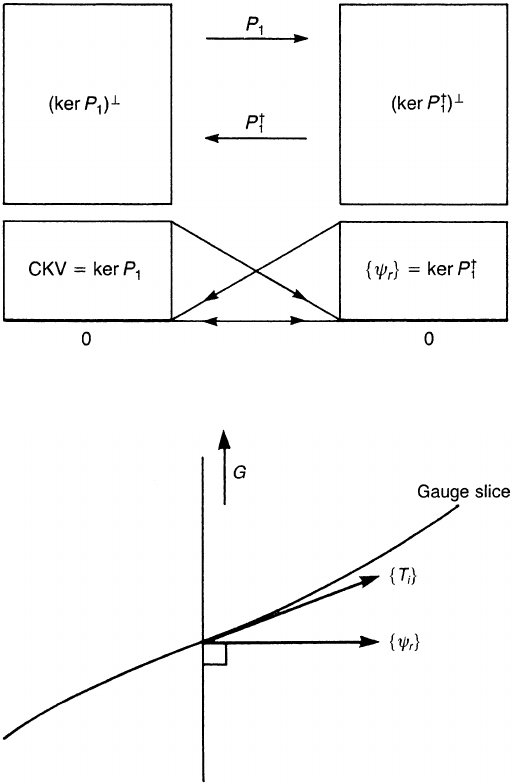
Figure 14.4. The map P
1
and its adjoint P
†
1
.
Figure 14.5. {T
i
}spans the deformation tangent to the gauge slice while {ψ
r
}spans ker P
†
1
.
Finally, δγ is decomposed into mutually orthogonal pieces as
δγ = δφγ + P
1
(δ ˜v + δt
i
u
i
) + δt
i
ψ
r
Q
ri
. (14.80a)
Correspondingly, the space of the metric deformation {δγ } separates into the
direct sum
{δγ }={conf}⊕{im P
1
}⊕{ker P
†
1
}.(14.80b)
Substituting (14.80a) into (14.75), we obtain
||δγ ||
2
= 4u||δφ||
2
+||P
1
δ ¯v||
2
+ δt
i
δt
j
(T
i
,ψ
r
)
γ
[(ψ, ψ)
−1
γ
]
rs
(ψ
s
, T
j
)
γ
(14.81)
where δ ¯v ≡ δ ˜v + δt
i
u
i
and the inverse in the last term refers to the inverse of the
matrix (a
rs
) = ((ψ
r
,ψ
s
)). If we put
t
1
= (δt,δφ,δ¯v),wefindthat
det
−1/2
(A
†
A) =
d
n
δ t δφ δ ¯v exp(−
1
2
t
1
A
†
A
1
)
=
δφ exp(−2u||δφ||
2
)
δ ¯v exp(−
1
2
||P
1
¯v||
2
)
×
d
n
δ t exp{−
1
2
δt
i
(T
i
,ψ
r
)[(ψ, ψ)
−1
]
rs
(ψ
s
, T
j
)δt
j
}
∝ (det P
†
1
P
1
)
−1/2
det(T ,ψ)
2
det(ψ, ψ)
−1/2
. (14.82)
Collecting the results (14.71) and (14.82), we have
1 = J det
1/2
(, ) det
−1/2
B
†
B det
−1/2
P
†
1
P
1
det(T ,ψ)
2
det(ψ, ψ)
−1/2
.
The g-loop partition function is then given by
Z
g
=
d
n
t ¯v φ det
˜
X
V (Diff ∗ Wey l)
det
1/2
B
†
B det
−1/2
(, )
×
det P
†
1
P
1
det(T,ψ)
2
det(ψ, ψ)
1/2
e
−S
. (14.83)
The integral over a (the CKV) has been omitted since it is already included in the
φ-integration. Naively, the integral over ¯v yields V (Diff
⊥
0
) and that over φ yields
V (Weyl). However, as exercise 14.3 shows, the measures
X and γ depend
on the conformal factor. Polyakov (1981) has shown that, under the conformal
transformation γ → e
2φ
γ , the measures transform as
X → exp
D
24π
2
d
2
ξ
√
γ(γ
αβ
∂
α
φ∂
β
φ + φ)
X (14.84a)
γ → exp
−26
24π
2
d
2
ξ
√
γ(γ
αβ
∂
α
φ∂
β
φ + φ)
γ. (14.84b)
Thus, the measure
X γ is conformally invariant if and only if D = 26. This
number 26 is called the critical dimension. Henceforth, we always assume that
D = 26. Now (14.83) simplifies as
Z
g
=
1
|MCG|
d
n
t
˜
X det
1/2
B
†
B det
−1/2
(, )
×
det P
†
1
P
1
det(T,ψ)
2
det(ψ, ψ)
1/2
e
−S
. (14.85)
We perform the X -integration to eliminate det
1/2
B
†
B.Wehave
1 =
δ X exp(−
1
2
||δ X ||
2
)
= J
δ
˜
X d
k
δ a exp(−
1
2
||δ
˜
X + δa
s
α
s
∂
α
˜
X||
2
)
= J
δ
˜
X exp(−
1
2
||δ
˜
X ||
2
)
d
k
δ a exp(−
1
2
||δa
s
α
s
∂
α
˜
X ||
2
)
= J det
−1/2
(B
†
B)
and hence det
1/2
(B
†
B) is identified with the Jacobian of the transformation
X → (
˜
X , a). Thus, it follows that
˜
X det
1/2
B
†
Be
−S
=
X
V (CKV)
e
−S
(14.86)
where V (CKV) =
d
k
a is the volume of the CKV.
The integration over X is readily carried out. Let us write
Xe
−S
=
X exp[−
1
2
(X,X)] (14.87a)
where
=−
1
√
γ
∂
α
√
γγ
αβ
∂
β
(14.87b)
is the Laplacian acting on 0-forms, see (7.188). We write down the explicit form
of the path integral (14.87a). Let ψ
n
be the eigenfunction of ,
ψ
n
= λ
n
ψ
n
λ
n
∈[0, ∞) (14.88)
where ψ
n
are normalized as
(ψ
n
,ψ
m
) =
d
2
ξ
√
γψ
n
ψ
m
= δ
nm
.
The eigenvalue λ is non-negative since is positive definite. Let us expand X
µ
in ψ
n
as
X
µ
=
∞
n=0
a
µ
n
ψ
n
= X
µ
0
+ X
µ
a
µ
n
∈ R (14.89)
where X
µ
0
= a
µ
0
ψ
0
is the zero eigenfunction of and X
µ
are the remaining
degrees of freedom. Correspondingly, the path integral (14.87a) is written as
X exp[−
1
2
(X,X)]=
n,µ
da
µ
n
exp
−
1
2
n,µ
λ
n
(a
µ
n
)
2
=
µ
da
µ
0
n=0
µ
da
µ
n
exp
−
1
2
n,µ
λ
n
(a
µ
n
)
2
=
µ
da
µ
0
(det
)
−13
(14.90)
where the prime indicates that the zero mode is omitted. To integrate over the
zero mode, we note that the normalized eigenvector ψ
0
is given by
6
ψ
0
=
1
d
2
ξ
√
γ
1/2
. (14.91)
From X
µ
0
= a
µ
0
ψ
0
,wehave
µ
da
µ
0
=
µ
dX
µ
0
(ψ
0
)
−26
= V
1
d
2
ξ
√
γ
−13
(14.92)
where V =
dX
µ
0
is the spacetime volume. Collecting the results (14.90) and
(14.92), we find that
Xe
−S
=
det
d
2
ξ
√
γ
−13
(14.93)
where we have dropped V and other irrelevant constants.
Finally, we have obtained the expression for the g-loop partition function
Z
g
=
Mod
d
n
t
V (CKV)
det(T,ψ)
det
1/2
(ψ, ψ) det
1/2
(, )
×[det
P
†
1
P
1
]
1/2
det
d
2
ξ
√
γ
−13
(14.94)
where we have noted that
1
|MCG|
Teich
d
n
t =
Mod
d
n
t. (14.95)
If g ≥ 2, the Riemann surfaces have no CKV and (14.95) reduces to
Z
g
=
Mod
d
n
t
det(T,ψ)
det
1/2
(ψ, ψ)
(det
P
†
1
P
1
)
1/2
det
d
2
ξ
√
γ
−13
. (14.96)
6
Since ψ
0
satisfies ψ
0
= 0, it is a harmonic function. Any harmonic function on a Riemann
surface must be a constant by the maximum principle.

14.2.3 Complex tensor calculus and string measure
Since any Riemann surface admits complex structures, we may take advantage of
this fact to compute string amplitudes. Many beautiful aspects of string theory
are revealed only when these complex structures are explicitly taken into account.
Here we rewrite the partition function in the language of complex differential
geometry.
We first fix the gauge in
g
by choosing the isothermal coordinate system
γ =
1
2
e
2σ
[dz ⊗ d¯z + d¯z ⊗ dz]
where γ
z ¯z
= γ
¯zz
=
1
2
exp 2σ .
7
Then the deformation of γ under a
diffeomorphism generated by δv is (cf (14.45))
δ
D
γ
zz
= 2∇
(−1)
z
δv
z
δ
D
γ
z ¯z
=∇
z
δv
¯z
+∇
¯z
δv
z
= γ
¯zz
(∇
(1)
z
δv
z
+∇
z
(−1)
δv
z
).
(14.97)
Similarly, δ
W
γ generated by an infinitesimal conformal change is (cf (14.46))
δ
W
γ
z ¯z
= δφγ
z ¯z
δ
W
γ
zz
= 0. (14.98)
To see the action of the operator P
1
on vectors, we take δv
z
∈
1
and
δv
z
∈
−1
. From (14.50), we find that
(P
1
δv)
zz
= 2∇
z
(1)
δv
z
∈
2
(14.99a)
(P
1
δv)
zz
= 2∇
(−1)
z
δv
z
∈
−2
. (14.99b)
This shows that P
1
is a map:
P
1
=
∇
z
(1)
0
0 ∇
(−1)
z
:
1
⊕
−1
→
2
⊕
−2
. (14.100)
Similarly, P
†
1
maps traceless symmetric tensors to vectors. For δh
zz
∈
2
and
δh
zz
∈
−2
,wehave
(P
†
1
δh)
z
=∇
(2)
z
δh
zz
∈
1
(14.101a)
(P
†
1
δh)
z
=∇
z
(−2)
δh
zz
∈
−1
. (14.101b)
Thus, P
†
1
is a map:
P
†
1
=
∇
(2)
z
0
0 ∇
z
(−2)
:
2
⊕
−2
→
1
⊕
−1
. (14.102)
7
In fact, the gauge is not uniquely fixed with this choice. We will invoke the uniformization theorem
later to fix the gauge completely.
The product P
†
1
P
1
is
P
†
1
P
1
=
∇
(2)
z
∇
z
(1)
0
0 ∇
z
(−2)
∇
(−1)
z
:
1
⊕
−1
→
1
⊕
−1
. (14.103)
Accordingly, the determinant in (14.96) becomes
(det
P
†
1
P
1
)
1/2
= (det
∇
(2)
z
∇
z
(1)
det
∇
z
(−2)
∇
(−1)
z
)
1/2
= (det
+
(1)
−
(−1)
)
1/2
(14.104)
where
±
(n)
are the Laplacians. We show that the spectrum of
+
(1)
is the same as
that of
−
(−1)
. Take an eigenfunction δv
z
of
+
(1)
,
+
(1)
δv
z
=−2e
−4σ
∂
z
e
2σ
∂
¯z
δv
z
= λδz
z
(14.105)
where (14.21a) has been used. The eigenvalue λ is a non-negative real number
(note
±
(n)
are positive-definite Hermitian operators). Then we find
−
(−1)
(γ
z ¯z
δv
z
) =−e
−2σ
∂
¯z
e
2σ
∂
z
δv
z
=−e
−2σ
∂
z
e
2σ
∂
¯z
δv
z
=−γ
z ¯z
2e
−4σ
∂
z
e
2σ
∂
¯z
δv
z
= λγ
z ¯z
δv
z
(14.106)
which shows that γ
z ¯z
δv
z
is an eigenfunction of
−
(−1)
with the same eigenvalue
λ. It is easy to see that the converse is also true, see exercise 14.5. Thus,
+
(1)
and
−
(−1)
share the same eigenvalues and det
+
(1)
= det
−
(−1)
. Now (14.104)
becomes
(det
P
†
1
P
1
)
1/2
= det
−
(−1)
= det
+
(1)
. (14.107)
Exercise 14.5. Let δv
z
be an eigenvector of
−
(−1)
with an eigenvalue λ.Show
that γ
z ¯z
δv
z
is an eigenvector of
+
(1)
with the same eigenvalue.
The physical change of the metric is the Teichm¨uller deformation δτ
i
µ
i
,
where τ
i
(µ
i
) is the complex counterpart of t
i
(T
i
). From our experience, we
know that the relevant part of the Teichm¨uller deformation is symmetric and
traceless in the real basis. In the complex basis, this amounts to µ
iz¯z
= µ
i ¯zz
= 0.
Accordingly, the general variation of the metric is given by
δγ
zz
=∇
(−1)
z
δ ˜v
z
+ δτ
i
µ
izz
(14.108a)
δγ
z ¯z
= δφγ
z ¯z
(14.108b)
where we have redefined δφ so that it includes the variation of δγ
z ¯z
due to δv
(note that δ
D
γ
z ¯z
∝ γ
z ¯z
). In (14.108a), δ ˜v does not contain the CKV, that is,
δ ˜v ∈ (ker ∇
(−1)
z
)
⊥
.
