Mourachkine A. Room-Temperature Superconductivity

Подождите немного. Документ загружается.

174 ROOM-TEMPERATURE SUPERCONDUCTIVITY

= 0.19

> 0.3

0.27 <

< 0.3

CuO

plane

= 0

0 <

< 0.05

0.05 <

< 0.13

0.14 <

< 0.18

0.2 <

< 0.27

~150

150 Å

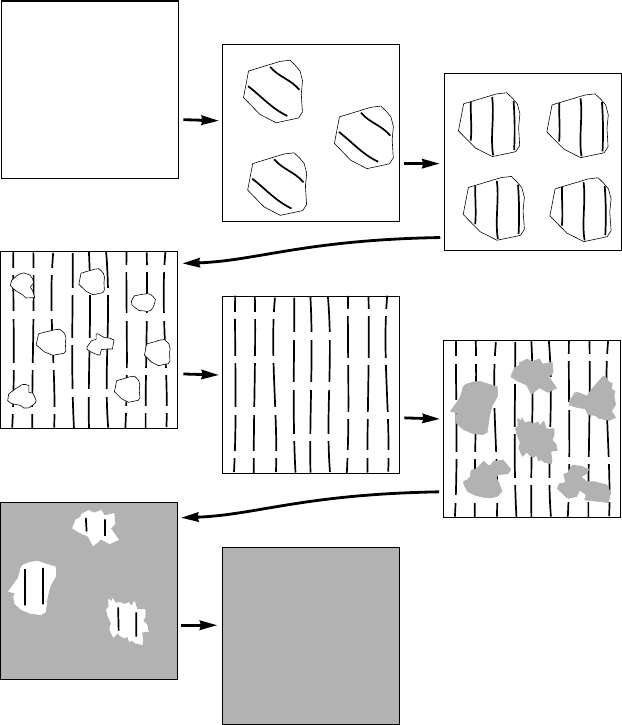
Figure 6.6. Sketch of charge distribution into a CuO

plane as a function of doping. Anti-

ferromagnetic and metallic phases are shown in white and grey, respectively. The lines show

charge stripes (see text for more details).

structures, the average distance between vertical charge stripes in the CuO

planes above p  0.125 remains constant, d

 4a, in correspondence with

that shown in Fig. 6.2. The quasi-two-dimensional spin stripes that separate

the dynamic charge stripes can be considered as a local memory effect of the

antiferromagnetic insulating phase at low doping.

The vertical charge stripes are dynamic in the clusters: they meander and

can move in the transverse direction. Therefore, they are not strictly one-

dimensional but quasi-one-dimensional. The mean length of a separate vertical

Third group of superconductors: Mechanism of superconductivity 175

1/8

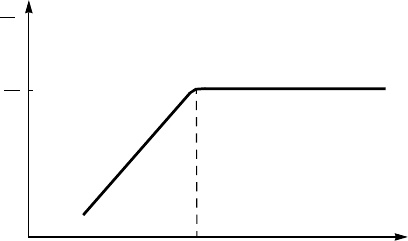
Figure 6.7. Doping dependence of incommensurability 2δ (∝ 1/d

) of magnetic ordering,

where d

is the distance between charge stripes (see references in [19]).

charge stripe is about 30–40 A

◦

, i.e. ∼ 10a. The striped phase will be discussed

in detail in the following subsection.

In the optimally doped (p ∼ 0.16) and overdoped (0.2 ≤ p ≤ 0.27) regions,

the average distance between charge stripes remains unchanged, as shown in

Fig. 6.7. Therefore, as the doping level increases, new doped holes take over

the virginal antiferromagnetic matrix. In the optimally doped region, the pic-

ture is diametrically opposed to that in the underdoped region: now clusters

with intact antiferromagnetic order are embedded in a matrix with vertical

charge stripes, as illustrated in Fig. 6.6. The clusters with intact antiferro-

magnetic order disappears completely at p = 0.19, as shown in Fig. 6.8a. The

point p = 0.19 is a quantum critical point in the cuprates. At p = 0.19, the

CuO

planes, in a ﬁrst approximation, consist only of the striped phase shown

in Fig. 6.2. The fraction of this phase as a function of doping level is sketched

in Fig. 6.8b. Since the Cooper pairs in the cuprates originate from the striped

phase, it is the most robust at p = 0.19, and not at p = 0.16 where T

is a

maximum for most of the cuprates.

Finally, let us consider the overdoped region (0.2 ≤ p ≤ 0.27). Above p =

0.19, new doped holes gather between stripes, forming clusters with the Fermi

sea in which the hole distribution is more or less homogeneous, as schemati-

cally depicted in Fig. 6.6. The Fermi-sea clusters are embedded in a matrix

with vertical charge stripes. As p → 0.27, the Fermi-sea clusters grow in size

and start coalescing. The fraction of the Fermi-see clusters (matrix) in the

CuO

planes is depicted in Fig. 6.8a. At low temperature, superconductivity

in the cuprates collapses when the doping level reaches p  0.27. However,

the hole distribution in the CuO

planes, apparently, is not yet homogeneous:

it is most likely that in the interval 0.27 <p<0.3, there are clusters with

vertical charge stripes imbedded in a Fermi-see matrix, as shown in Fig. 6.6.

176 ROOM-TEMPERATURE SUPERCONDUCTIVITY

0.16

0.05

0.27

100

0.1

0.2

100

0.3

(a)

(b)

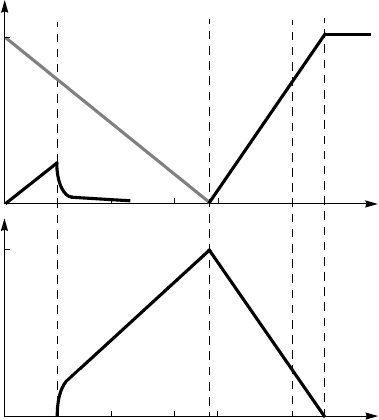
Figure 6.8. Fraction of a certain matrix or clusters in the CuO

planes of cuprates at T  T

as a function of doping: (a) antiferromagnetic matrix or clusters (AF), Fermi-sea clusters or

matrix (M), and spin-glass clusters (SG); and (b) clusters or matrix with vertical charge stripes

(VS). Both plots are shown schematically.

The hole distribution in the CuO

planes becomes homogeneous only above

p = 0.3. The question of hole distribution in the interval 0.27 <p<0.3 is

not very important for the understanding of the mechanism of unconventional

superconductivity in the cuprates.

As was noted above, in undoped cuprates, unconventional spin glass occurs

at low temperatures in the clusters with diagonal charge stripes which are em-

bedded into an antiferromagnetic matrix. The fraction of this spin glass as a

function of doping level is sketched in Fig. 6.8a. At p  0.05, the charge-

stripe orientation in most of the nanoscale clusters becomes vertical (or hor-

izontal). However, in a very small fraction of the clusters, the charge stripes

remain diagonally oriented. Therefore, this unconventional spin glass can still

be observed in the cuprates at very low temperatures and p>0.05, for exam-

ple, by muon spin relaxation/rotation (µSR) measurements. The clusters with

diagonal charge stripes in the CuO

planes disappear completely somewhere

between 0.16 and 0.19.

In Fig. 6.8a, the disappearance of the matrix (clusters) with local antiferro-

magnetic order at p = 0.19 does not imply the disappearance of magnetic cor-

relations above this doping level. The insulating quasi-two-dimensional stripes

that are located between vertical charge stripes remain magnetically ordered at

Third group of superconductors: Mechanism of superconductivity 177

low temperature. Experimentally, even in the highly overdoped region, mag-

netic relaxation is still dominant.

3.2.1 Nickelates and manganites

A similar stripe order is observed in nickelates with the NiO

planes [13].

In the nickelates, however, the charge orientation is always diagonal as that

in the undoped cuprates. Doped holes in the nickelates are less destructive to

the antiferromagnetic background than those in the isostructural cuprates. The

phase diagram of La

2−x

NiO

(LSNO) is very similar to the phase diagram

of the isostructural LSCO, having a series of phases which are closely related

to the HTT, LTO and LTT phases of LSCO. Thus, there are many similarities

between the striped phases of the cuprates and nickelates.

The stripe order is also observed in manganites with the MnO

planes. De-

pending on the doping level, the manganites can exhibit either antiferromag-

netic or ferromagnetic ordering. A mesoscopic phase separation into the MnO

planes of the manganites is an experimental fact [41].

Charge stripes in the nickelates and manganites ﬂuctuate slowly in time and

space, while in the cuprates very quickly. Therefore, it is much easier to ob-

serve a charge inhomogeneity in the nickelates and manganites than that in the

cuprates. As a consequence, there is ample evidence in the literature for charge

stripes in the nickelates and manganites (see references in [19]), and much less

for the cuprates. Nevertheless, there is direct evidence for dynamic charge

stripes in superconducting cuprates as well, for example, in LSCO, YBCO

(see references in [19]) and Bi2212 [42].

3.3 The striped phase

In the cuprates, the length of a separate charge stripe can vary. The mean

length is about 30–40 A

◦

,or∼ 10a. The charge stripes in the cuprates are dy-

namic: they can meander and move in the transverse direction. In the cuprates,

the charge stripes are quoter-ﬁlled: that is one positive electron charge per two

sites along the stripes (in the nickelates, the charge density along the

stripes is one hole per each Ni

site). The pattern of charge stripes in real

space shown in Fig. 6.2, in fact, is not correct for the CuO

planes. In Fig.

6.2, such an alternating order along the stripes has a periodicity of 4k

in mo-

mentum space, where k

is the wave number at the Fermi surface. Analysis of

INS data obtained in some cuprates show that, in the cuprates, the periodicity

of charge stripes in momentum space is not 4k

but 2k

. A real-space sketch

of a 2k

quoter-ﬁlled charge stripe is shown in Fig. 6.9. One can see that this

pattern is different from that in Fig. 6.2. The chains in YBCO have also the

charge modulation observed by tunneling spectroscopy. It is worth noting

that traditional charge-ordered states such as sinusoidal CDWs, for example,

in Peierls compounds have also a 2k

charge modulation; however, they are

178 ROOM-TEMPERATURE SUPERCONDUCTIVITY

...

charge ordering

Figure 6.9. Schematic ordering pattern on a 2k

quoter-ﬁlled charge stripe. In plot, • (◦)

denotes the presence (absence) of a hole.

quasi-static, contrary to the charge stripes in the cuprates. Thus, the charge

order on the stripes in the cuprates is similar to usual CDWs.

In discussing the charge distribution of the CuO

planes in the previous sub-

section, we assumed that the vertical charge stripes run above the Cu sites. In

principle, the stripes can alternatively be centered on the Cu–O bonds. Simula-

tions of angle-resolved photoemission (ARPES) data obtained in Bi2212 show

that the stripes in Bi2212 seem to be site-centered, not bond-centered.

Why do holes doped in the CuO

planes prefer to form stripes rather than

to be distributed homogeneously? The combination of the electron-phonon,

Coulomb and magnetic interactions results in the appearance of the charge

stripes. The charge-stripe ordering in the cuprates, nickelates and manganites

is evidence for a strong, nonlinear electron-lattice coupling. The holes in these

compounds are self-trapped.

In the cuprates as in any system with strongly-correlated electrons, the elect-

ron-electron interactions are unscreened and, as a consequence, very strong.

At low temperature, the magnetic order in the cuprates, frustrated by doped

holes, does everything to expel them from the magnetic phase. In the presence

of a strong and nonlinear electron-phonon interaction, doped holes become

self-trapped, that is, in exchange of interaction with the lattice, a doped hole

locally deforms the lattice in a way that it is attracted by the deformation. In its

turn, this local deformation created by the ﬁrst hole attracts another one, and

so on. Of course, without the Coulomb repulsion, the holes attracted by the

lattice deformation would rather gather in a cluster, not in a one-dimensional

stripe. Thus, the charge stripes in the CuO

planes are a “product” of the

electron-phonon, Coulomb and magnetic interactions. In addition, there are

other factors favoring the charge-stripe formation; for example, gathering in

one-dimensional stripes, the holes lower their kinetic energy in the transverse

direction.

The parallel arrangement of the charge stripes minimizes the Coulomb re-

pulsion between the neighboring stripes. What is the characteristic length

of the charge-stripe order in the cuprates? Experimentally, the characteristic

length of the charge-stripe order is about 80–110 A

◦

, i.e. ∼ 21–28a [42, 43].

The striped phase in Fig. 6.2 which is nearly one-dimensional will cause

the appearance of two incommensurate Bragg peaks symmetric about the fun-

damental lattice Bragg peaks for an ideal square CuO

plane. Experimentally

Third group of superconductors: Mechanism of superconductivity 179

however, upon doping, the fundamental lattice Bragg peaks are replaced by

four new incommensurate peaks displaced by ±2δ from the fundamental lat-

tice peaks (see Fig. 6.33a). It was initially proposed that the appearance of

four incommensurate peaks is the consequence of the stripe orientation in the

adjacent CuO

layers: the stripe orientation is alternately rotated by 90

◦

layer

by layer, as schematically shown in Fig. 6.10a. Later, another explanation

for the appearance of four incommensurate Bragg peaks was proposed [19].

Since the charge-stripe ordering is not very long (∼ 25a), it is possible that, in

each CuO

plane, the charge-stripe orientation in neighboring nanoscale clus-

ters with vertical charge stripes may differ by 90

◦

, as schematically depicted

in Fig. 6.10b. Thus, the charge stripes with vertical and horizontal orientations

may coexist in the same CuO

plane, causing the appearance of four incom-

mensurate Bragg peaks. In this case it is possible that at high doping level thus

in the overdoped region, the stripes in the neighboring clusters with perpen-

dicular stripe orientations may merge, forming structures with the “L” and “”

shapes.

In the cuprates, nickelates and manganites, the charge stripes are insulating

on the nanoscale scale. This means that there is a charge gap on the stripes. On

a macroscopic scale, however, the charge stripes are conducting due to soliton-

like excitations present on the stripes. Therefore, the in-plane charge transport

in the cuprates is quasi-one-dimensional, as well as that in the nickelates and

manganites.

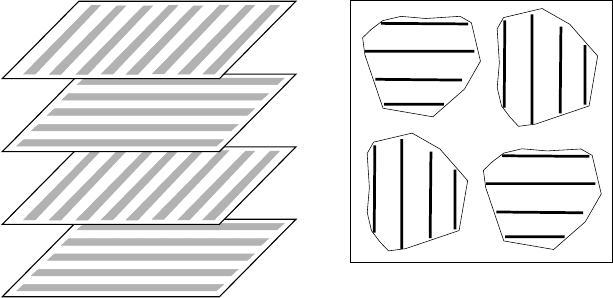
(a)

(b)

CuO

plane

Figure 6.10. (a) Stripe orientation in adjacent CuO

layers is assumed to be alternately rotated

by 90

◦

. (b) Sketch of charge-stripe clusters in a CuO

plane having two possible orientations in

the same CuO

plane.

180 ROOM-TEMPERATURE SUPERCONDUCTIVITY

3.4 Phase diagram

Let us now discuss the phase diagram of hole-doped cuprates. The prob-

lem, however, is that the phase diagram for each superconducting cuprate, in

a sense, is unique. Of course, the differences among all these phase diagrams

are not drastic; nevertheless, the phase diagram for each cuprate has its spe-

ciﬁc features. As an example, comparing the phase diagrams for LSCO and

YBCO, shown respectively in Figs. 3.8 and 3.10, one can notice that, in these

two cuprates, the width of the antiferromagnetic phase at low doping level is

different. In addition, there are some other features in each plot. In such a situ-

ation, we have no alternative than to consider in detail a phase diagram of one

superconducting cuprate which reﬂects main features of the physics involved

in all cuprates.

Figure 6.11 shows an idealized phase diagram of Bi2212. In the plot, the

commensurate antiferromagnetic phase at low doping level is not shown be-

cause it was not yet observed in Bi2212: There is a technical problem to

synthesize large-size good-quality undoped single crystals of Bi2212 which

are necessary for INS and µSR measurements. Without the antiferromagnetic

scale at low doping level, Figure 6.11 depicts six temperature/energy scales for

Bi2212.

In Fig. 6.11, the spin-glass T

temperature scale was recently observed in

Bi2212 by µSR measurements [45]. As was discussed above,this unconvention-

100

200

300

400

500

0 0.05 0.1 0.15 0.2 0.25 0.3

hole concentration, p

Bi2212

pair

QCP

Temperature (K)

Figure 6.11. Phase diagram of Bi2212: T

is the critical temperature; T

pair

is the pairing

temperature; T

is the magnetic-transition temperature; T

is the magnetic-ordering tem-

perature; and T

is the charge-ordering temperature (see text for more details) [19, 44]. The

commensurate antiferromagnetic phase at low doping is not shown. The spin-glass temperature

scale T

is shown schematically (QCP = quantum critical point).

Third group of superconductors: Mechanism of superconductivity 181

CuO

plane

Figure 6.12. Schematic representation of four types of clusters (phases) existing in the CuO

planes at different dopings. Cluster A includes the intact antiferromagnetic phase. Cluster B

includes diagonal charge stripes and a magnetic ordering between the stripes. The stripes are

schematically shown by the lines. Cluster C includes vertical charge stripes and a magnetic

ordering between the stripes. In cluster D, holes are distributed homogeneously (Fermi liquid).

The magnetic orderings in clusters A, B and C occur at T

, T

and T

, respectively.

al spin glass occurs in nanoscale clusters with diagonal charge stripes, shown

schematically in Fig. 6.12. The T

temperature scale ﬁrst rises approximately

linearly as the doping level starts to increase from zero, reaches a maximum

when it crosses the Ne



el temperature scale T

(p), and then falls to zero as the

doping increases. In Bi2212, T

 8Katp = 0.05, and T

= 0 somewhere at

p  0.16 [45].

In Bi2212, the doping level of p = 0.19 is a quantum critical point which

is located at absolute zero. In general, in a quantum critical point, a magnetic

order is about to form or to disappear. At low temperature, superconductivity

in the cuprates is the most robust at this doping level p = 0.19, and not at p =

0.16 where T

is a maximum.

In Fig. 6.11, the T

temperature scale starts/ends in the quantum criti-

cal point (MT = Magnetic Transition). Then, it is more or less obvious that

this temperature scale has the magnetic origin. The T

temperature scale is

analogous to a magnetic transition temperature of a long-range antiferromag-

netic phase in heavy fermions [19]. The doping dependence T

(p) can be

expressed as

(p)  T

MT,0



1 −

0.19



, (6.1)

where T

MT,0

= 970–990 K (see references in [19]). This temperature scale

can be observed in resistivity, nuclear magnetic resonance (NMR) and speciﬁc-

182 ROOM-TEMPERATURE SUPERCONDUCTIVITY

heat measurements, and will be discussed separately. Along the transition tem-

perature T

(p), magnetic ﬂuctuations are very strong. The T

temperature

scale originates from a local antiferromagnetic ordering in hole-poor clusters

(matrix) shown in Fig. 6.12. This type of clusters disappears completely at

p = 0.19.

In Fig. 6.11, the three temperature scales, T

, T

and T

pair

, originate

from nanoscale clusters with vertical charge stripes, shown schematically in

Fig. 6.12. The T

temperature scale corresponds to a charge-stripe order-

ing (CO = charge ordering). We already know that the striped phase in the

cuprates is charge driven, and a structural phase transition precedes the charge

ordering (see Fig. 6.5). The doping dependence T

(p) can approximately be

expressed as

(p)  980 ×



1 −

0.3



(in K). (6.2)

The corresponding charge gap ∆

, observed in tunneling and ARPES mea-

surements, depends on hole concentration as

∆

(p)  251 ×



1 −

0.3



(in meV). (6.3)

In the striped phase of cuprates shown in Fig. 6.2, the charge ordering is

always followed by a magnetic ordering. In Fig. 6.11, the T

temperature

scale corresponds to a magnetic ordering (MO) occurring in insulating spin

stripes located between the charge stripes. This is shown in Fig. 6.12. The

doping dependence T

(p) can be expressed as follows

(p)  566 ×



1 −

0.3



(in K). (6.4)

Since the charge stripes in the CuO

planes ﬂuctuate very rapidly, this mag-

netic ordering must also rearrange itself quickly. The dynamic quasi-two-

dimensional spin stripes can be considered as a local memory effect of the

commensurate antiferromagnetic phase located at low dopings. In the striped

phase, the magnetic order occurring at T

helps to stabilize the charge order.

In Fig. 6.11, the T

pair

temperature scale corresponds to the formation of

Cooper pairs, the doping dependence of which can be expressed as

pair

(p) 

(p)

980



1 −

0.3



 326



1 −

0.3



(in K). (6.5)

The corresponding pairing energy scale ∆

depends on the doping level as

follows

∆

(p) 

∆

(p)

251



1 −

0.3



 83.6



1 −

0.3



(in meV). (6.6)

Third group of superconductors: Mechanism of superconductivity 183

The pairing gap manifests itself in tunneling and ARPES measurements. It

is important to emphasize that the ratio between T

pair

and ∆

, obtained ex-

perimentally, clearly indicates a strong-coupling regime of electron pairing in

Bi2212:

2∆

 6 k

pair

. (6.7)

The superconducting phase appears at a critical temperature T

shown in

Fig. 6.11. In Bi2212 and in most of the cuprates, the doping dependence T

(p)

can be expressed as

(p)  T

c,max

[1 − 82.6(p − 0.16)

]. (6.8)

For Bi2212, T

c,max

= 95 K. The superconducting phase is approximately lo-

cated between p = 0.05 and 0.27, having the maximum critical temperature

c,max

in the middle, thus at p  0.16. The corresponding phase-coherence

energy scale ∆

is proportional to T

2∆

=Λk

. (6.9)

In different cuprates, the coefﬁcient Λ is slightly different: Λ  5.45 in

Bi2212; Λ  5.1 in YBCO; and Λ  5.9 in Tl2201. The phase-coherence

gap ∆

manifests itself in Andreev-reﬂection, penetration-depth and tunneling

measurements.

Let us consider a few issues directly related to the phase diagram in Fig.

6.11. First, in Bi2212, the extensions of three temperature scales T

(p),

(p) and T

pair

(p) cut the concentration axis approximately in one point, at

p = 0.3. Superconductivity however collapses at about p  0.27. Assuming

that this collapse is due to a lack of long-range phase coherence, one can then

understand why in Fig. 6.6, the distributionof doped holes in the CuO

plane at

0.27 <p<0.3 is shown inhomogeneous. Second, the two temperature scales

(p) and T

(p) in Fig. 6.11 intersect the vertical axis approximately in

one point, at T ≈ 980 K. This seems logic. When, in the CuO

planes (at p →

0), doped holes gather in nanoscale clusters at T

to form charge stripes, the

hole-poor matrix gets an opportunity to order itself magnetically, at least, lo-

cally. It is obvious that the latter process depends on the former one. At p = 0

they occur simultaneously (or almost simultaneously) at T ∼ 980 K. In reality

however, this point is not accessible because the melting point of Bi2212 is

about 850 K. Third, in the phase diagram of Bi2212 shown in Fig. 6.11, one

can see that three temperature scales have the magnetic origin, namely, T

(p),

(p) and T

(p). This is not odd because these three temperature scales

originate from magnetic orderings that occur in different clusters (or matrix)

sketched in Fig. 6.12. The spin glass occurs at T

in the nanoscale clusters

with diagonal charge stripes. The T

temperature scale originates from lo-

cal antiferromagnetic ordering in the hole-poor matrix which becomes divided

into clusters as p → 0.19. The magnetic ordering occurring in insulating spin