Moran M.J., Shapiro H.N. Fundamentals of Engineering Thermodynamics

Подождите немного. Документ загружается.

equilibrium composition even though it is not involved in the chemical reaction. The form

of Eq. 14.24 suggests that at equilibrium the tendency of A and B to form C and D is just

balanced by the tendency of C and D to form A and B.

The stoichiometric coefficients 

, 

, and 

do not correspond to the respective

number of moles of the components present. The amounts of the components present are

designated n

, n

,and n

. However, changes in the amounts of the components

present do bear a definite relationship to the values of the stoichiometric coefficients.

That is

(14.25a)

where the minus signs indicate that A and B would be consumed when C and D are pro-

duced. Since E is inert, the amount of this component remains constant, so dn

 0.

Introducing a proportionality factor d Eqs. 14.25a take the form

from which the following expressions are obtained:

(14.25b)

The parameter is sometimes referred to as the extent of reaction.

For the system under present consideration, Eq. 14.10 takes the form

Introducing Eqs. 14.25b and noting that dn

 0, this becomes

At equilibrium, dG]

T,p

 0, so the term in parentheses must be zero. That is

or when written in a form resembling Eq. 14.24

(14.26)

For the present case, Eq. 14.26 is the equation of reaction equilibrium. In principle, the

composition that would be present at equilibrium for a given temperature and pressure can

be determined by solving this equation. The solution procedure is simplified through the

equilibrium constant concept introduced in the next section.

 n

 n

 n

n

 n

 n

 0

dG4

T, p

 1n

 n

 n

2 de

dG4

T, p

 m

 m

 n

de,

 n

n

de,

n

dn



dn



 de

dn



dn



686 Chapter 14 Chemical and Phase Equilibrium

extent of reaction

equation of reaction

equilibrium



14.3 Calculating Equilibrium Compositions

The objective of the present section is to show how the equilibrium composition of a system

at a specified temperature and pressure can be determined by solving the equation of reac-

tion equilibrium. An important part is played in this by the equilibrium constant.

14.3 Calculating Equilibrium Compositions 687

 14.3.1 Equilibrium Constant for Ideal Gas Mixtures

The first step in solving the equation of reaction equilibrium, Eq. 14.26, for the equilibrium

composition is to introduce expressions for the chemical potentials in terms of temperature,

pressure, and composition. For an ideal gas mixture, Eq. 14.17 can be used for this purpose.

When this expression is introduced for each of the components A, B, C, and D, Eq. 14.26

becomes

(14.27)

where is the Gibbs function of component i evaluated at temperature T and the pressure

ref

 1 atm. Equation 14.27 is the basic working relation for chemical equilibrium in a mix-

ture of ideal gases. However, subsequent calculations are facilitated if it is written in an

alternative form, as follows.

Collect like terms and rearrange Eq. 14.27 as

(14.28)

The term on the left side of Eq. 14.28 can be expressed concisely as G°. That is

(14.29a)

which is the change in the Gibbs function for the reaction given by Eq. 14.24 if each reac-

tant and product were separate at temperature T and a pressure of 1 atm. This expression can

be written alternatively in terms of specific enthalpies and entropies as

(14.29b)

Since the enthalpy of an ideal gas depends on temperature only, the of Eq. 14.29b are

evaluated at temperature T. As indicated by the superscript , each of the entropies is evalu-

ated at temperature T and a pressure of 1 atm.

Introducing Eq. 14.29a into Eq. 14.28 and combining the terms involving logarithms into

a single expression gives

(14.30)

Equation 14.30 is simply the form taken by the equation of reaction equilibrium, Eq. 14.26,

for an ideal gas mixture subject to the reaction Eq. 14.24. As illustrated by subsequent ex-

amples, similar expressions can be written for other reactions.

Equation 14.30 can be expressed concisely as

(14.31)

¢G°

 ln K1T 2



¢G°

 ln c

ref

 n

 n

’s

 1n

 n

 n

2 T 1n

s °

 n

s °

 n

s °

 n

s °

¢G°  n

 T s °

2 n

 T s °

2 n

 T s °

2 n

 T s °

¢G°  n

g °

 n

g °

 n

g °

 n

g °

 RT an

ref

 n

ref

 n

ref

 n

ref

g °

 n

g °

 n

g °

 n

g °

 n

ag °

 RT ln

ref

b n

ag °

 RT ln

ref

ag °

 RT ln

ref

b n

ag °

 RT ln

ref

where K is the equilibrium constant defined by

(14.32)

Given the values of the stoichiometric coefficients, 

, 

, and 

and the temperature

T, the left side of Eq. 14.31 can be evaluated using either of Eqs. 14.29 together with the ap-

propriate property data. The equation can then be solved for the value of the equilibrium con-

stant K. Accordingly, for selected reactions K can be evaluated and tabulated against temper-

ature. It is common to tabulate log

K or ln K versus temperature, however. A tabulation of

log

K values over a range of temperatures for several reactions is provided in Table A-27,

which is extracted from a more extensive compilation.

The terms in the numerator and denominator of Eq. 14.32 correspond, respectively, to the

products and reactants of the reaction given by Eq. 14.24 as it proceeds from left to right as

written. For the inverse reaction the equilibrium constant takes

the form

(14.33)

Comparing Eqs. 14.32 and 14.33, it follows that the value of K* is just the reciprocal of K:

K*  1K. Accordingly,

(14.34)

Hence, Table A-27 can be used both to evaluate K for the reactions listed proceeding in the

direction left to right and to evaluate K* for the inverse reactions proceeding in the direction

right to left.

Example 14.1 illustrates how the log

K values of Table A-27 are determined. Subsequent

examples show how the log

K values can be used to evaluate equilibrium compositions.

log

K* log

K* 

ref

 n

n

 n

C  n

A  n

K1T2

ref

 n

 n

n

688 Chapter 14 Chemical and Phase Equilibrium

equilibrium constant

EXAMPLE 14.1 Evaluating the Equilibrium Constant at a Specified Temperature

Evaluate the equilibrium constant, expressed as log

K, for the reaction at (a) 298 K and (b) 2000 K. Com-

pare with the value obtained from Table A-27.

SOLUTION

Known: The reaction is

Find: Determine the equilibrium constant for T  298 K (25C) and T  2000 K.

Assumption: The ideal gas model applies.

Analysis: The equilibrium constant requires the evaluation of G for the reaction. Invoking Eq. 14.29b for this purpose,

we have

where the enthalpies are evaluated at temperature T and the absolute entropies are evaluated at temperature T and a pressure

of 1 atm. Using Eq. 13.13, the enthalpies are evaluated in terms of the respective enthalpies of formation, giving

where the terms account for the change in specific enthalpy from T

ref

 298 K to the specified temperature T. The en-

thalpy of formation of oxygen is zero by definition.

¢h

¢G°  31h°

 1h°



1h°

4 31¢h2

 1¢h2



1¢h2

4 T 1s °

 s °



s°

¢G°  1h

 h



2 T 1s °

 s °



s°

CO 

14.3 Calculating Equilibrium Compositions 689

 14.3.2 Illustrations of the Calculation of Equilibrium

Compositions for Reacting Ideal Gas Mixtures

It is often convenient to express Eq. 14.32 explicitly in terms of the number of moles that

would be present at equilibrium. Each mole fraction appearing in the equation has the form

 n

n, where n

is the amount of component i in the equilibrium mixture and n is the

total number of moles of mixture. Hence, Eq. 14.32 can be rewritten as

(14.35)

The value of n must include not only the reacting components A, B, C, and D but also all

inert components present. Since inert component E has been assumed present, we would

write n  n

 n

Equation 14.35 provides a relationship among the temperature, pressure, and composition

of an ideal gas mixture at equilibrium. Accordingly, if any two of temperature, pressure, and

composition are known, the third can be found by solving this equation.  for

example. . .

suppose that the temperature T and pressure p are known and the object is

the equilibrium composition. With temperature known, the value of K can be obtained from

Table A-27. The n’s of the reacting components A, B, C, and D can be expressed in terms

of a single unknown variable through application of the conservation of mass principle to

K 



ref

 n

n

(a) When T  298 K, the terms of the above expression for vanish. The required enthalpy of formation and absolute

entropy values can be read from Table A-25, giving

With this value for , Eq. 14.31 gives

which corresponds to log

K  45.093.

Table A-27 gives the logarithm to the base 10 of the equilibrium constant for the inverse reaction: That

is, log

K* 45.066. Thus, with Eq. 14.34, log

K  45.066, which agrees closely with the calculated value.

(b) When T  2000 K, the and terms for O

, CO, and CO

required by the above expression for G are evaluated

from Tables A-23. The enthalpy of formation values are the same as in part (a). Thus

With this value, Eq. 14.31 gives

which corresponds to log

K  2.885.

At 2000 K, Table A-27 gives log

K* 2.884. With Eq. 14.34, log

K  2.884, which is in agreement with the calculated

value.

Using the procedures described above, it is straightforward to determine log

K versus temperature for each of several specified

reactions and tabulate the results as in Table A-27.

ln K 

1110,4532

18.3142 120002

 6.643

282,990  5102  167,435 110,453 kJ/kmol



167,881  868224 20003309.210  258.600 

1268.65524

¢G°  31393,5202 1110,5302

1024 31100,804  93642 165408  86692

°¢h

CO 

ln K 

1257,253 kJ/kmol2

18.314 kJ/kmol

K2 1298 K2

 103.83

¢G°

257,253 kJ/kmol

¢G°  31393,5202 1110,5302

1024 2983213.69  197.54 

1205.0324

¢G°¢h



the various chemical species present. Then, since the pressure is known, Eq. 14.35 consti-

tutes a single equation in a single unknown, which can be solved using an equation solver

or iteratively with a hand calculator. 

In Example 14.2, we apply Eq. 14.35 to study the effect of pressure on the equilibrium

composition of a mixture of CO

, CO, and O

690 Chapter 14 Chemical and Phase Equilibrium

EXAMPLE 14.2 Determining Equilibrium Composition Given Temperature and Pressure

One kilomole of carbon monoxide, CO, reacts with kmol of oxygen, O

, to form an equilibrium mixture of CO

, CO, and

at 2500 K and (a) 1 atm, (b) 10 atm. Determine the equilibrium composition in terms of mole fractions.

SOLUTION

Known: A system initially consisting of 1 kmol of CO and kmol of O

reacts to form an equilibrium mixture of CO

, CO,

and O

. The temperature of the mixture is 2500 K and the pressure is (a) 1 atm, (b) 10 atm.

Find: Determine the equilibrium composition in terms of mole fractions.

Assumption: The equilibrium mixture is modeled as an ideal gas mixture.

Analysis: Equation 14.35 relates temperature, pressure, and composition for an ideal gas mixture at equilibrium. If any two

are known, the third can be determined using this equation. In the present case, T and p are known, and the composition is

unknown.

Applying conservation of mass, the overall balanced chemical reaction equation is

where z is the amount of CO, in kmol, present in the equilibrium mixture. Note that

The total number of moles n in the equilibrium mixture is

Accordingly, the molar analysis of the equilibrium mixture is

At equilibrium, the tendency of CO and O

to form CO

is just balanced by the tendency of CO

to form CO and O

,so

we have Accordingly, Eq. 14.35 takes the form

At 2500 K, Table A-27 gives log

K 1.44. Thus, K  0.0363. Inserting this value into the last expression

(a)

(a) When p  1 atm, Eq. (a) becomes

Using an equation solver or iteration with a hand calculator, z  0.129. The equilibrium composition in terms of mole fractions

is then



210.1292

2.129

 0.121,



0.129

2.129

 0.061,



211  0.1292

2.129

 0.818

0.0363 

1  z

2  z



0.0363 

1  z

2  z



ref



K 

z1z



11  z2



ref

12  z2



11



21



1  z

2  z



ref



CO 



2  z



2  z



211  z2

2  z

n  z 

 11  z2

2  z

0  z  1.

1CO 

S zCO 

 11  z2CO

14.3 Calculating Equilibrium Compositions 691

In Example 14.3, we determine the temperature of an equilibrium mixture when the pres-

sure and composition are known.

(b) When p  10 atm, Eq. (a) becomes

Solving, z  0.062. The corresponding equilibrium composition in terms of mole fractions is y

 0.06,  0.03,

Comparing the results of parts (a) and (b) we conclude that the extent to which the reaction proceeds toward completion

(the extent to which CO

is formed) is increased by increasing the pressure.

It is left as an exercise to show that if the mole fraction of CO

in the equilibrium mixture at 2500 K were 0.93, the cor-

responding pressure would be closely 22.4 atm.

 0.91.

0.0363 

1  z

2  z



1102



❶

❷

❶

❷

EXAMPLE 14.3 Determining Equilibrium Temperature Given Pressure and Composition

Measurements show that at a temperature T and a pressure of 1 atm, the equilibrium mixture for the system of Example 14.2

has the composition y

 0.298, Determine the temperature T of the mixture, in K.

SOLUTION

Known: The pressure and composition of an equilibrium mixture of CO, O

, and CO

are specified.

Find: Determine the temperature of the mixture, in K.

Assumption: The mixture can be modeled as an ideal gas mixture.

Analysis: Equation 14.35 relates temperature, pressure, and composition for an ideal gas mixture at equilibrium. If any two

are known, the third can be found using this equation. In the present case, composition and pressure are known, and the tem-

perature is the unknown.

Equation 14.35 takes the same form here as in Example 14.2. Thus, when p  1 atm, we have

where z is the amount of CO, in kmol, present in the equilibrium mixture and T is the temperature of the mixture.

The solution to Example 14.2 gives the following expression for the mole fraction of the CO in the mixture: y



2z(2  z). Since y

 0.298, z  0.35.

Inserting this value for z into the expression for the equilibrium constant gives K  0.2078. Thus, log

K 0.6824.

Interpolation in Table A-27 then gives T  2881 K.

Comparing this example with part (a) of Example 14.2, we conclude that the extent to which the reaction proceeds to

completion (the extent to which CO

is formed) is decreased by increasing the temperature.

1T2

1  z

2  z



 0.553.y

 0.149,

❶

In Example 14.4, we consider the effect of an inert component on the equilibrium

composition.

692 Chapter 14 Chemical and Phase Equilibrium

EXAMPLE 14.4 Considering the Effect of an Inert Component

One kilomole of carbon monoxide reacts with the theoretical amount of air to form an equilibrium mixture of CO

, CO, O

and N

at 2500 K and 1 atm. Determine the equilibrium composition in terms of mole fractions, and compare with the result

of Example 14.2.

SOLUTION

Known: A system initially consisting of 1 kmol of CO and the theoretical amount of air reacts to form an equilibrium mix-

ture of CO

, CO, O

, and N

. The temperature and pressure of the mixture are 2500 K and 1 atm.

Find: Determine the equilibrium composition in terms of mole fractions, and compare with the result of Example 14.2.

Assumption: The equilibrium mixture can be modeled as an ideal gas mixture.

Analysis: For a complete reaction of CO with the theoretical amount of air

Accordingly, the reaction of CO with the theoretical amount of air to form CO

, CO, O

, and N

where z is the amount of CO, in kmol, present in the equilibrium mixture.

The total number of moles n in the equilibrium mixture is

The composition of the equilibrium mixture in terms of mole fractions is

At equilibrium we have So Eq. 14.35 takes the form

The value of K is the same as in the solution to Example 14.2, K  0.0363. Thus, since p  1 atm, we have

Solving, z  0.175. The corresponding equilibrium composition is y

 0.059,

Comparing this example with Example 14.2, we conclude that the presence of the inert component nitrogen reduces the

extent to which the reaction proceeds toward completion at the specified temperature and pressure (reduces the extent to which

is formed).

 0.278, y

 0.029, y

 0.634.

0.0363 

1  z

5.76  z



K 

z1z



11  z2



ref

15.76  z2



CO 



5.76  z



5.76  z



211  z2

5.76  z



3.76

5.76  z

n  z 

 11  z2 1.88 

5.76  z

CO 

 1.88N

S zCO 

 11  z2CO

 1.88N

CO 

 1.88N

S CO

 1.88N

In the next example, the equilibrium concepts of this chapter are applied together with

the energy balance for reacting systems developed in Chap. 13.

EXAMPLE 14.5 Using Equilibrium Concepts and the Energy Balance

Carbon dioxide at 25C, 1 atm enters a reactor operating at steady state and dissociates, giving an equilibrium mixture of CO

CO, and O

that exits at 3200 K, 1 atm. Determine the heat transfer to the reactor, in kJ per kmol of CO

entering. The effects

of kinetic and potential energy can be ignored and W

 0.

14.3 Calculating Equilibrium Compositions 693

SOLUTION

Known: Carbon dioxide at 25C, 1 atm enters a reactor at steady state. An equilibrium mixture of CO

, CO, and O

exits at

3200 K, 1 atm.

Find: Determine the heat transfer to the reactor, in kJ per kmol of CO

entering.

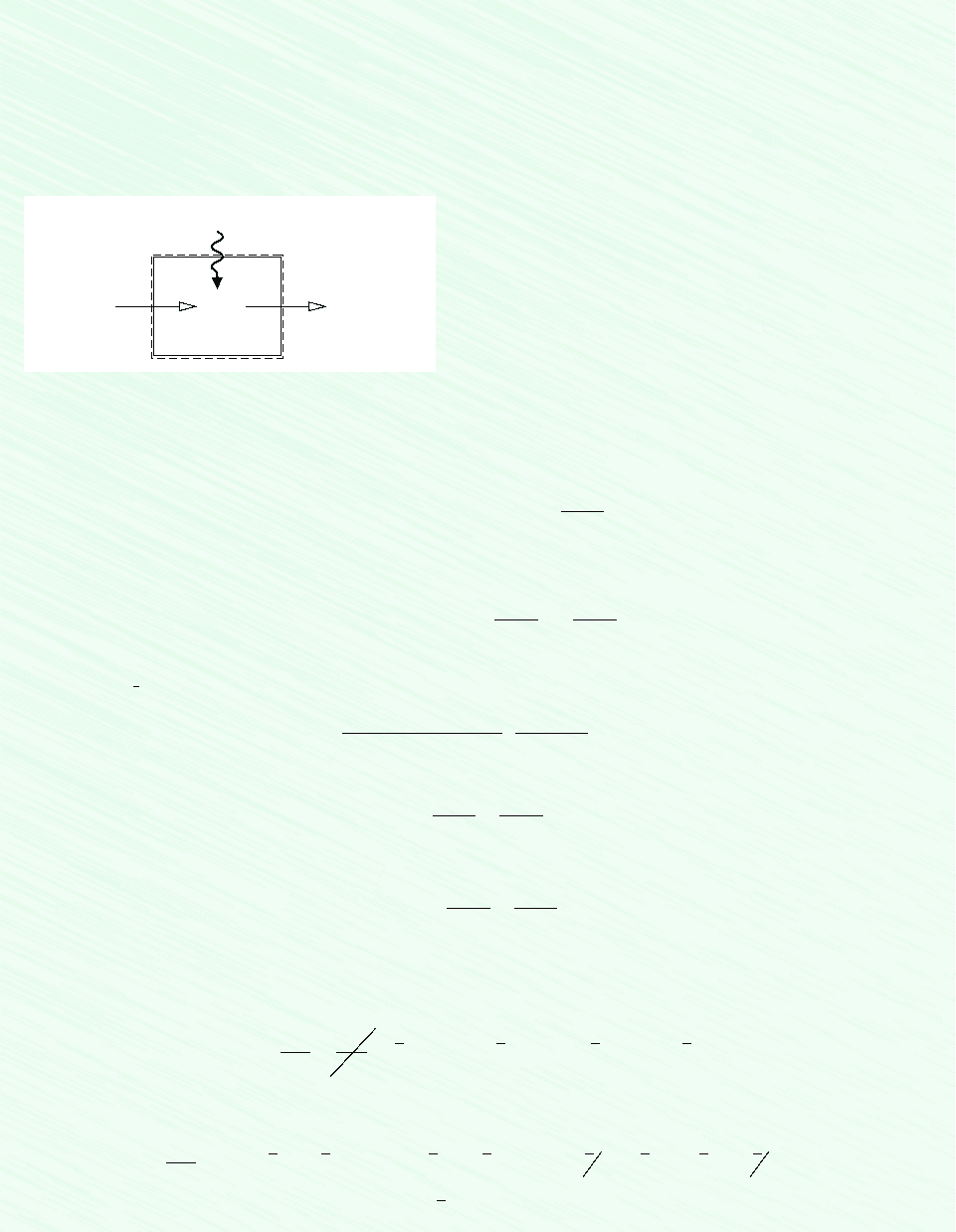
Schematic and Given Data:

25°C, 1 atm

(CO

, CO, O

)

3200 K, 1 atm

 Figure E14.5

Assumptions:

1. The control volume shown on the accompanying sketch by a

dashed line operates at steady state with Kinetic energy

and potential energy effects can be ignored.

2. The entering CO

is modeled as an ideal gas.

3. The exiting mixture of CO

, CO, and O

is an equilibrium ideal

gas mixture.

 0.

Analysis: The required heat transfer can be determined from an energy rate balance for the control volume, but first the

composition of the exiting equilibrium mixture must be determined.

Applying the conservation of mass principle, the overall dissociation reaction is described by

where z is the amount of CO

, in kmol, present in the mixture exiting the control volume, per kmol of CO

entering. The total

number of moles n in the mixture is then

The exiting mixture is assumed to be an equilibrium mixture (assumption 3). Thus, for the mixture we have

Equation 14.35 takes the form

Rearranging and noting that p  1 atm

At 3200 K, Table A-27 gives log

K 0.189. Thus, K  0.647, and the equilibrium constant expression becomes

Solving, z  0.422. The composition of the exiting equilibrium mixture, in kmol per kmol of CO

entering, is then (0.422CO

0.578CO, 0.289O

When expressed per kmol of CO

entering the control volume, the energy rate balance reduces by assumption 1 to

Solving for the heat transfer per kmol of CO

entering, and evaluating each enthalpy in terms of the respective enthalpy

of formation

The enthalpy of formation of O

is zero by definition; for the CO

at the inlet vanishes because CO

enters at 25C.¢h

 0.4221h°

 ¢h2

 0.5781h°

 ¢h2

 0.2891h°

 ¢h2

 1h°

 ¢h2

0 



 h

 10.422h

 0.578h

 0.289h

0.647  a

1  z

b a

1  z

3  z



K  a

1  z

b a

1  z

3  z



K 

11  z2

311  z2



ref

13  z2



11



21

CO 

n  z  11  z2 a

1  z

b

3  z

S zCO

 11  z2 CO  a

1  z

b O

 14.3.3 Equilibrium Constant for Mixtures and Solutions

The procedures that led to the equilibrium constant for reacting ideal gas mixtures can be

followed for the general case of reacting mixtures by using the fugacity and activity con-

cepts introduced in Sec. 11.9. In principle, equilibrium compositions of such mixtures can

be determined with an approach paralleling the one for ideal gas mixtures.

Equation 11.141 can be used to evaluate the chemical potentials appearing in the equa-

tion of reaction equilibrium (Eq. 14.26). The result is

(14.36)

where is the Gibbs function of pure component i at temperature T and the pressure p

ref



1 atm, and a

is the activity of that component.

Collecting terms and employing Eq. 14.29a, Eq. 14.36 becomes

(14.37)

This equation can be expressed in the same form as Eq. 14.31 by defining the equilibrium

constant as

(14.38)

Since Table A-27 and similar compilations are constructed simply by evaluating

for specified reactions at several temperatures, such tables can be employed to evaluate the

more general equilibrium constant given by Eq. 14.38. However, before Eq. 14.38 can be

used to determine the equilibrium composition for a known value of K, it is necessary to

evaluate the activity of the various mixture components. Let us illustrate this for the case of

mixtures that can be modeled as ideal solutions.

¢G°



K 



¢G°

 ln a

1g °

 RT ln a

2 n

1g °

 RT ln a

2 n

1g °

 RT ln a

2 n

1g °

 RT ln a

694 Chapter 14 Chemical and Phase Equilibrium

With enthalpy of formation values from Tables A-25 and values for O

, CO, and CO

, from Table A-23

For comparison, let us determine the heat transfer if we assume no dissociation—namely, when CO

alone exits the re-

actor. With data from Table A-23, the heat transfer is

The value is much less than the value obtained in the solution above because the dissociation of CO

requires more energy

input (an endothermic reaction).

 174,695  9364  165,331 kJ/kmol

1CO

 h

13200 K2 h

1298 K2

 322,385 kJ/kmol 1CO

 0.2891114,809  86822 1393,5202

 0.4223393,520  1174,695  936424 0.5783110,530  1109,667  866924

¢h

❶

This section requires study of Sec. 11.9.

14.4 Further Examples of the Use of the Equilibrium Constant 695

IDEAL SOLUTIONS. For an ideal solution, the activity of component i is given by

(11.142)

where f

is the fugacity of pure i at the temperature T and pressure p of the mixture, and

is the fugacity of pure i at temperature T and the pressure p

ref

. Using this expression to eval-

uate a

, a

, and a

, Eq. 14.38 becomes

(14.39a)

which can be expressed alternatively as

(14.39b)

The ratios of fugacity to pressure in this equation can be evaluated, in principle, from

Eq. 11.124 or the generalized fugacity chart, Fig. A-6, developed from it. In the special case

when each component behaves as an ideal gas at both T, p and T, p

ref

, these ratios equal unity

and Eq. 14.39b reduces to the underlined term, which is just Eq. 14.32.

K  c



1 f



1 f



1 f



d c

f °



ref

1 f °



ref

1 f °



ref

1 f °



ref

d c

ref

 n

 n

K 



f °



f °



f °



f °



f °

Some additional aspects of the use of the equilibrium constant are introduced in this section:

the equilibrium flame temperature, the van’t Hoff equation, and chemical equilibrium for

ionization reactions and simultaneous reactions. To keep the presentation at an introductory

level, only the case of ideal gas mixtures is considered.

 14.4.1 Determining Equilibrium Flame Temperature

In this section, the effect of incomplete combustion on the adiabatic flame temperature, intro-

duced in Sec. 13.3, is considered using concepts developed in the present chapter. We begin with

a review of some ideas related to the adiabatic flame temperature by considering a reactor

operating at steady state for which no significant heat transfer with the surroundings takes place.

Let carbon monoxide gas entering at one location react completely with the theoretical

amount of air entering at another location as follows:

As discussed in Sec. 13.3, the products would exit the reactor at a temperature we have

designated the maximum adiabatic flame temperature. This temperature can be determined

by solving a single equation, the energy equation. At such an elevated temperature, however,

there would be a tendency for CO

to dissociate

Since dissociation requires energy (an endothermic reaction), the temperature of the prod-

ucts would be less than the maximum adiabatic temperature found under the assumption of

complete combustion.

When dissociation takes place, the gaseous products exiting the reactor would not be CO

and

, but a mixture of CO

,CO,O

,and N

. The balanced chemical reaction equation would read

(14.40)

CO 

 1.88N

S z CO  11  z2CO



 1.88N

S CO 

CO 

 1.88N

S CO

 1.88N



14.4 Further Examples of the Use of the

Equilibrium Constant