Moller K.D. Optics. Learning by Computing, with Examples Using Mathcad, Matlab, Mathematica, and Maple

Подождите немного. Документ загружается.

2.7. RANDOM ARRANGEMENT OF SOURCE POINTS 127

The result for the intensity in the case where the array is not periodic is

 A

N. (2.123)

We compare this result to Eq. (2.117), that is, for the periodic array. For maxima

we obtained

 A

. (2.124)

This is an important result for the discussion of phenomena having their origin in

periodic and non periodic appearance. In our case of interference, one has for the

non periodic case an “incoherent” addition of the waves. The result is an average

distribution I ∝ N, and there is no interference pattern. In the periodic case, the

waves add coherently and an interference pattern is observed. The light appears

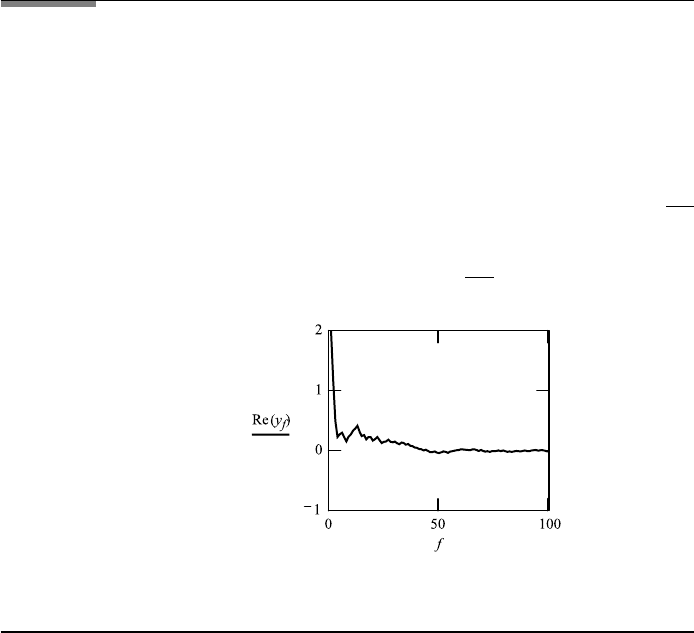
as maxima and minima. In FileFig 2.21 we show the incoherent addition of the

waves for the non periodic case. The sum of Eq. (2.122) is plotted depending

on the number N

of randomly positioned openings and approaches zero when

choosing large numbers of N

FileFig 2.21 (I21RANDS)

Incoherent addition of N phase factors.

I21RANDS

Addition of Exponential Functions with Random Angles

The real part of the sum of exp iθ is plotted.

f : 1 ...100 N

: fk: 1, 2,...1000 i :

√

−1

: rnd(2 · π) y

:



k0

i·(θ

)

Application 2.21. Change f to a small value and then increase it and observe

the changes in the average.

128 2. INTERFERENCE

See also on CD

PI1. Cos-Waves depending on Space and Time (see p. 80).

PI2. Superposition of two cos-waves with ﬁxed Optical path Difference (see

p. 82).

PI3. 3-D Graph of Maxima and Minima (see p. 84).

PI4. Average( see p. 85).

PI5. Fresnel’s Mirrors (see p. 93).

PI6. Young’s and Lloyd Experiment (see p. 93).

PI7. Plane parallel Plate in two Beam Interferometry with different Refractive

Indices (see p. 95).

PI9. Wedge shaped Film (see p. 99).

PI10. Newton’s Rings (see p. 101).

PI11. Ring pattern and Michelson interferometer (see p. 107).

PI12. Plane parallel plate (see p.108-111).

PI13. Interference of white light on a thin ﬁlm (see p. 113).

PI14. Reﬂection and Transmission coefﬁcients (p. 111–112).

PI15. Plane parallel plate, graphs depending on wavelength (see p. 113).

PI16. Dependence on angle of the Finesse (p. 117).

PI17. Plane parallel plate with mirror surface.

PI18. Fabry-Perot (see p. 115).

PI19. Interference with an array of source points (see p. 122).

PI20. Summation of random phase angles (see p. 125).

CHAPTER

Diffraction

3.1 INTRODUCTION

We know Huygens’Principle from introductory physics. It tells us that a “new”

wavefront of a traveling wave may be constructed at a later time by the envelope

of many wavelets generated at the “old” wavefront. One assumes that a primary

wave generates ﬁctitious spherical waves at each point of the “old” wavefront.

The ﬁctitious spherical wave is called Huygens’wavelet and the superposition of

all these wavelets results in the “new” wavefront. This is schematically shown in

Figure 3.1. The distance between the generating source points is inﬁnitely small

and therefore, integration has to be applied for their superposition.

We discussed in Chapter 2 the superposition of light waves and the resulting

interference patterns. In the division process of the incident wave into parts, we

neglected the effect of diffraction. In this chapter we take into account interfer-

ence and diffraction of the wave incident on an aperture. Optical path differences,

FIGURE 3.1 Schematic of wavefront construction using Huygens’ Principle.

129

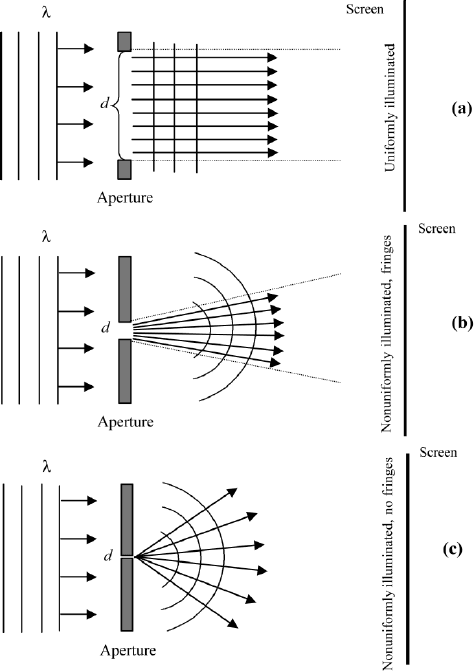
130 3. DIFFRACTION

FIGURE 3.2 Conditions for diffraction on a single slit: (a) d  λ, no appreciable diffraction; (b)

d of the same order of magnitude of λ, diffraction is observed (fringes); (c) d  λ, nonuniformly

illuminated observation screen, but no fringes.

generated between adjacent light waves, are ﬁnite for the superposition process

of interference and inﬁnitely small for diffraction.

If we apply this division process to an open aperture, the incident wave gen-

erates new waves in the plane of the aperture, and these newly generated waves

have ﬁxed phase relations with the incident wave and with one another. We as-

sume that all waves generated by the incident wave propagate only in the forward

direction, and not backward to the source of light. Let us consider the diffraction

on a slit (Figure 3.2). The observed pattern depends on the wavelength and the

size of the opening. A slit of a width of several orders of magnitude larger than

the wavelength of the incident light will give us almost the geometrical shadow

(Figure 3.2a). A slit of width of an order or two larger than the wavelength

will bend the light and fringes will occur; see Figure 3.2b. A slit smaller than the

wavelengthwill showan intensity pattern with no fringes and decreasing intensity

3.2. KIRCHHOFF–FRESNEL INTEGRAL 131

for larger angles; see Figure 3.2c. All openings will show small deformations of

the wavefront close to the edges of the slit (not shown in Figure 3.2).

The model we are using for the description of diffraction is called scalar wave

diffraction theory and uses the Kirchhoff–Fresnel integral. All the waves we

consider are solutions of the scalar wave equation, as used for the discussion of

the interference phenomena in Chapter 2. Here we use spherical waves of the

type Ae

ikr

/r, where A is the magnitude of the wave, r the distance from the

origin, and k  2π/λ. These spherical waves are solutions of the scalar wave

equation

∇

u + k

u  0. (3.1)

Written in spherical coordinates r, θ , and φ one has

∇

 (1/r

){∂/∂r(r

∂/∂r)}+ (terms in θ and φ), (3.2)

where we have not explicitly given the terms in θ and φ because we only use

spherical symmetric solutions and they do not depend on the angular terms.

There is the question of why we should use a summation process based on

the idea of Huygen’s Principle to describe diffraction theory. Why not solve

Maxwell’s equations with the appropriate boundary conditions? The mathemat-

ical formulation of Huygens’ Principle was performed by Gustav Kirchhoff and

Augustin Jean Fresnel before Maxwell’s theory was developed. It turned out

that the use of the Kirchhoff–Fresnel integral for many applications is so much

easier than solving Maxwell’s equations and applying boundary conditions, that

one just continues to use the scalar wave diffraction theory. The wavelength is

assumed to be smaller than the aperture opening under consideration.

3.2 KIRCHHOFF–FRESNEL INTEGRAL

3.2.1 The Integral

We assume for the summation process of the Huygens’wavelets, that the primary

wave from the source S has amplitude A and travels distance R in the direction

of the aperture (Figure 3.3). We disregard the time factor for all waves consid-

ered in this chapter. We recall that in Chapter 2 the time factor disappeared when

calculating the intensity. At each point of the aperture a Huygens’wavelet is gen-

erated, [(1/ρ) exp(ikρ)], and travels only in the “forward” direction (Figure 3.3).

It has the amplitude of the incident wave, that is, {(A/R) exp(ikR)}.Wehavefor

a newly generated wavelet

[(A/R) exp(ikR)](1/ρ) exp(ikρ) exp(iα), (3.3)

where exp(iα) is a phase factor related to the generation process. However, it is

set to 1.

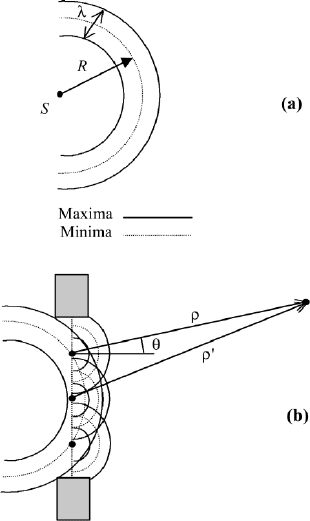
132 3. DIFFRACTION

FIGURE 3.3 (a) Maxima and minima of incident wave; (b) three newly generated Huygen’s

wavelets are shown at the aperture.

From experiments we know that there is an angular dependence of the in-

tensity in the direction of propagation. Therefore we multiply by cos θ , where

θ is the angle to the normal of the aperture, pointing into the forward direc-

tion. Integration over all points of the aperture results in the Kirchhoff–Fresnel

integral,



(A/R) exp(ikR)(1/ρ) exp(ikρ) cos θdσ, (3.4)

opening of aperture

where dσ is the surface element for integration over the opening of the aperture.

This integralmay be derived from the scalar wave equation and Green’s Theorem.

The derivation yields the factor cos θ and shows that the diffracted light is only

traveling in the forward direction. However, there are some problems with the

boundary conditions. A formulation using Green’s function avoids this problem,

but is not necessarily better. For more information, see Goodman, 1988, p. 42.

In the following two sections we apply Eq. (3.4) to a special symmetric ar-

rangement of source and observation points, both at large distances from the

aperture. We calculate the diffracted intensity only at one observation point on

3.2. KIRCHHOFF–FRESNEL INTEGRAL 133

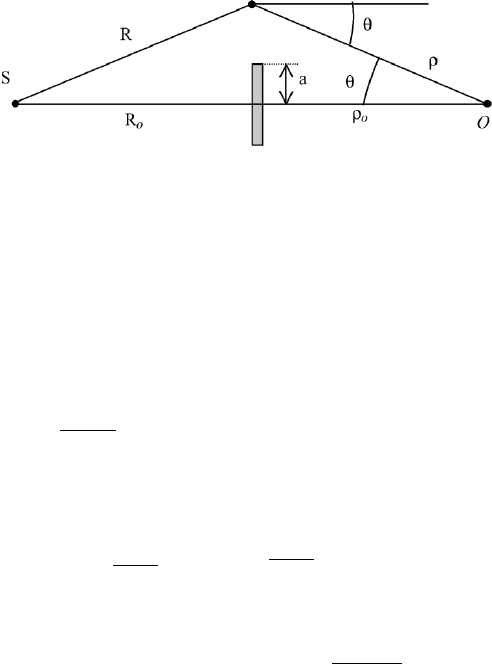
FIGURE 3.4 Coordinates for the circular opening. The source point and the observation point

have the same distance from the aperture.

the axis of the system for the diffraction on a round aperture and a round stop.

These calculations are taken from Sommerfeld’s book on theoretical physics.

3.2.2 On Axis Observation for the Circular Opening

The diffraction on a round opening is important since most lenses, spherical

mirrors, and optical instruments have circular symmetry. We consider a round

aperture of radius a with equal distance to the aperture of the source point S and

the observationpoint O. In Figure 3.4 we showthe coordinates and have R

 ρ

R  ρ, cos θ  ρ

/ρ, and for the surface element dσ  2πrdr. The amplitude

at the observation screen is then obtained by the integral over the opening

u  A



{(1/Rρ) exp(ik(R + ρ))}{ρ

/ρ} 2πrdr. (3.5)

opening

The integration limits are from ρ



+ ρ

). We get from r

+ ρ

 ρ

that rdr  ρdρ and have

u  A2πρ



√

+ρ

)

(1/ρ

) exp(ik2ρ)dρ. (3.6)

Integration by parts with u  1/ρ

, dv  e

ik2ρ

, and v  (1/2ik)e

ik2ρ

results in

(1/ρ

)(1/2ik)e

ik2ρ

√

+ρ

)



+(1/ik)



√

+ρ

)

(1/ρ

ik2ρ

dρ.

Since ρ is large, we retain only the ﬁrst term with the power of 1/ρ

and have

u  (2πAρ

/2ik)



i2k

√

+ρ



/(a

+ ρ

) − (exp{i2kρ

})/ρ



. (3.7)

Vorlesungen uber theoretische Physik, Band IV, by A. Sommerfeld. Dieterich’sche Verlagsbuchhandlung,

Wiesbaden, 1950.

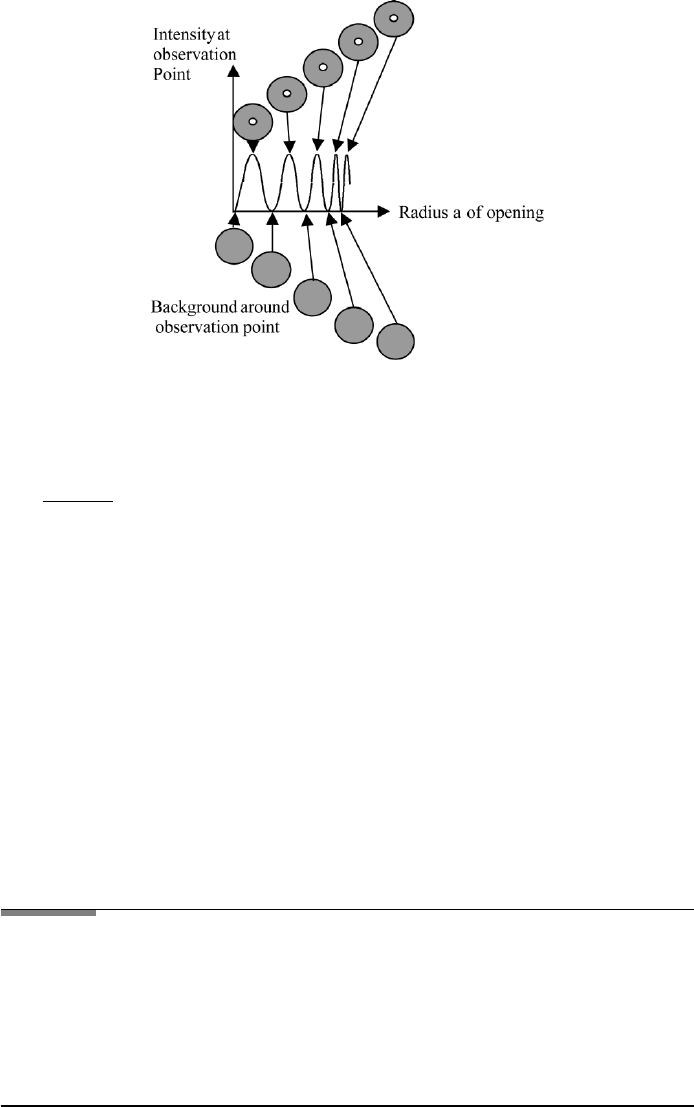
134 3. DIFFRACTION

FIGURE 3.5 Diffraction pattern for a circular aperture at the observation point. The intensity has

a maximum for certain values of the radius a, shown as a white spot on the gray background. For

other values of a the intensity is zero; only the gray background is shown.

Further simpliﬁcation is obtained by assuming ρ

 a,



+ ρ

≈ ρ

(1 + a

/2ρ

), (3.8)

and obtaining for the amplitude

u  (2πAρ

/2ik)(exp{i2kρ

})(1/ρ

)[exp{ik(a

/ρ

)}−1]. (3.9)

The intensity uu

∗

is obtained after normalization as

I  I

sin

(ka

/2ρ

). (3.10)

In Figure 3.5 we show maxima and minima for different radii a of the circular

opening, at the center. Our calculation refers to the center spot only and the radius

of the corresponding maxima or minima may be read from the graph in FileFig

3.1.

FileFig 3.1 (D1CIRS)

In FileFig 3.1 we show a graph of the intensity at the center. We use λ 

0.0005 mm, ρ

 4000 mm, and radius a of 0.1 to 5 mm. With increasing

diameter of the aperture, that is, with increasing a, we have at the center a

change from maxima to minima to maxima and so on.

D1CIR is only on the CD.

3.2. KIRCHHOFF–FRESNEL INTEGRAL 135

FIGURE 3.6 Coordinates for the circular stop. The source point and the observation point have

the same distance from the aperture.

3.2.3 On Axis Observation for Circular Stop

In optics, it is often of interest to study complementary screens or arrays. We

apply the Kirchhoff–Fresnel integral, Eq. (3.4), to a circular stop, as shown in

Figure 3.4. Similar to the “opening,” we must now evaluate

u  A2πρ



∞

√

+ρ

)

(1/ρ

) exp(ik2ρ)dρ. (3.11)

Integration by parts yields

(1/ρ

)(1/2ik)e

ik2ρ

∞



√

+ρ

+(1/ik)



∞

√

+ρ

(1/ρ

ik2ρ

dρ. (3.12)

Neglecting the last integral we get

u  (2πAρ

/2ik)[−{1/(a

+ ρ

)}]{exp(i2k



+ ρ

)}. (3.13)

Multiplication of u in Eq. (3.13) by u

∗

yields the intensity, and taking for the

normalization I

 A

/(a

+ ρ

), we have

I  I

/4. (3.14)

The intensity of Eq. (3.14) depends only on the wavelength, not on the diameter

of the aperture or the distance from it. We have the result that at any point in the

shadow of an aperture stop, we will observe a bright spot.

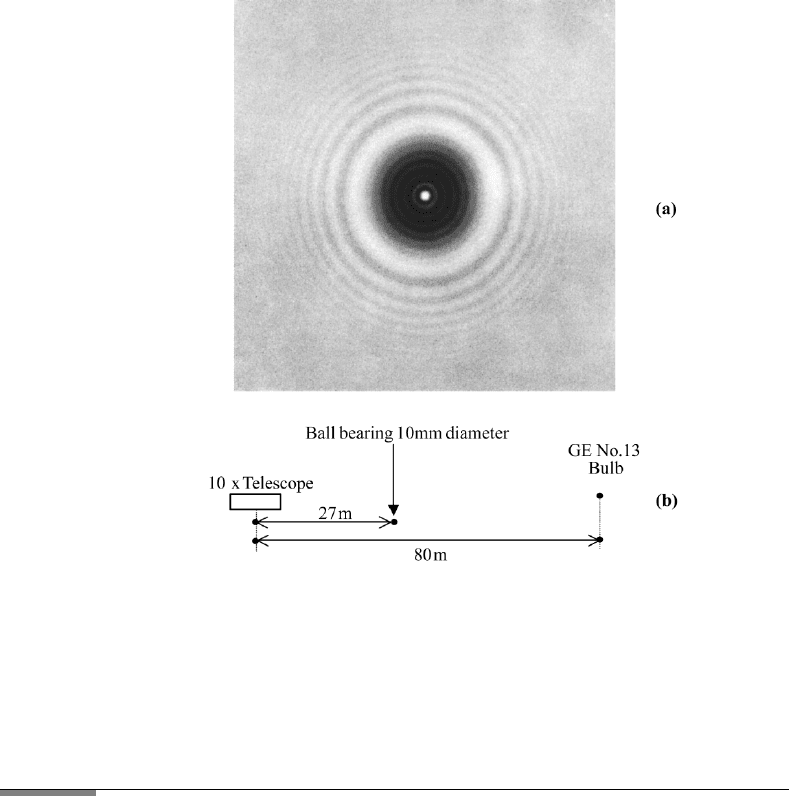
There is the story that Fresnel presented his wave theory of light to the French

Academy of Sciences. The famous Poisson questioned the validity and argued

that there should be a light spot in the shadow of an illuminated sphere, for

example, a steel ball. Another scientist of the Academy, Arago, made the exper-

iment, observed the spot and presented his ﬁnding to the Academy in support of

Fresnel’s theory, but the spot remains the “Poisson spot.” A photograph of the

Poisson spot and an experimental setup for observation are shown in Figure 3.7.

136 3. DIFFRACTION

FIGURE 3.7 (a) Photograph of the diffraction pattern produced by a round stop. The Poisson

spot appears in the middle (from Cagnet, Francon, Thrierr, Atlas of Optical Phenomena, Springer-

Verlag, Heidelberg, 1962); (b) parameters for the observation of the Poisson spot (after R. Pohl.

Einf¨uhrung in die Optic, R.W. Pohl, Springer-Verlag, Heidelberg, 1948).

3.3 FRESNEL DIFFRACTION, FAR FIELD

APPROXIMATION, AND FRAUNHOFER

OBSERVATION

In the ﬁrst two applications of the Kirchhoff–Fresnel integral, we have assumed

that the source of light and the observation point are at large distances from

the aperture. How large was not speciﬁed. When assuming that the distance is

“inﬁnitely large,” so large that we essentially have plane waves incident on the

aperture, we are at the approximation used in Chapter 2. When observing at

a screen similarly far away from the aperture, the waves arriving there are also

considered plane waves and are also parallel for their superposition. This is called

far ﬁeld approximation.When we use a lens and observe the diffraction pattern in

the focal plane, we have Fraunhofer diffraction. The mathematical presentation

of far ﬁeld approximation and Fraunhofer diffraction is the same. In contrast,

when the distance from the aperture to the observation screen is large but ﬁnite,