Moller K.D. Optics. Learning by Computing, with Examples Using Mathcad, Matlab, Mathematica, and Maple
Подождите немного. Документ загружается.

76 1. GEOMETRICAL OPTICS
matrix, the light may pass through many round trips and no light will escape.
One calls such a resonator stable, and the condition for stability is where the
magnitudes of the eigenvalues are equal to 1.
|λ
1
||λ
2
|1. (1.116)
We may write for Eq. (1.114),
λ
1
(2g
1
g
2
− 1) + [(2g
1
g
2
− 1)
2
− 1]
1/2
(1.117)
or
λ
1
(2g
1
g
2
− 1) + i[1 − (2g
1
g
2
− 1)
2
]
1/2
. (1.118)
The real and imaginary parts of Eq. (1.118) must be on a circle of radius 1; that is,
|(2g
1
g
2
− 1)|≤1, or 0 ≤ g
1
g
2
≤ 1. (1.119)
in agreement with the imaginary part and plotted in FileFig 1.32.
In FileFig 1.33 we show a repetition of the calculations, starting from the
five matrices of the cavity in Eq. (1.111), but now in terms of r
1
, r
2
, and d.In
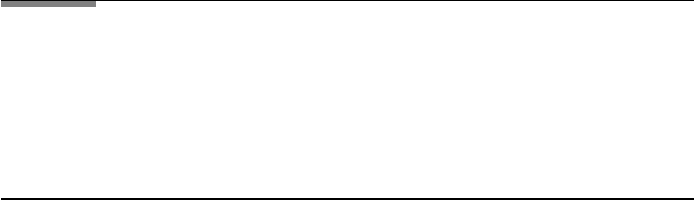
Figure 1.33, we show schematics of the Fabry–Perot, a focal, a confocal and a
spherical cavity for values of the parameters r
1
, r
2
, and d, and also of g
1
and g
2
.
For both representations one finds that the absolute values of the eigenvalues λ
1
and λ
2
are always 1.
FileFig 1.33 (G33RESCY)
Calculation of the eigenvalues of the cavity with two reflecting mirrors using r
1
,
r
2
, and d. Numerical calculation with r
1
1, r
2
1, and d 2.
G33RESCY is only on the CD.
Application 1.33. Use the values of the parameters r
1
, r
2
, and d for the Fabry–
Perot, focal, confocal, and spherical cavities, and find that all are stable cavities.
1.10. MATRICES FOR A REFLECTING CAVITY AND THE EIGENVALUE PROBLEM 77
FIGURE 1.33 Schematic of light path for four cavities with different values of radii of curvature
and length of cavity. The corresponding valuesof g
1
and g
2
are indicated: (a) Fabry–Perot; (b) focal;
(c) confocal; (d) concentric.

2
2
CHAPTER
Interference
2.1 INTRODUCTION
In Chapter 1 we described image formation by light, using our model which states
that light propagates along straight lines and utilizes the laws of reflection and
refraction. We now consider the wave nature of light. In the famous experiment
by Thomas Young, one observes on a screen an interference pattern, consisting
of bright and not so bright stripes of light. The interpretation of an interference
pattern was done by using an analogy to water waves. However, the water wave
pattern is observed as an amplitude interference pattern whereas the superpo-
sition of light waves, also generated as an amplitude pattern, is observed as an
intensity pattern. Historically, Newton associated the light beams of geometrical
optics with a stream of particles and some scientists attacked Young in his time,
saying that he was diminishing Newton’s work. Today we know that light is an
electromagnetic wave but, in a complementary way, light is also described by
quantum mechanics as an assembly of particles.
In this chapter we use a model for the description of interference phenomena.
We assume that there is always one incident wave when two- or more beam in-
terferometry is discussed. After one has taken into account what happens in the
experimental setup, the waves leaving the setup appear superimposed. The inter-
ference pattern is produced with finite optical path differences. The calculation
of the optical path difference and the interpretation of the resulting interference
pattern are the main subjects of this chapter. The process of splitting the inci-
dent wave into parts involves diffraction, which we neglect in this chapter as a
secondary effect and discuss in detail in Chapter 3.
In this chapter we use for the incident wave one harmonic wave, a solution of
the scalar wave equation, which is written in Cartesian coordinates as
∂
2
u/∂x
2
+ ∂
2
u/∂y
2
+ ∂
2
u/∂z
2
(1/v)
2
∂
2
u/∂t
2
, (2.1)
79

80 2. INTERFERENCE
where v is the phase velocity of light in the medium with refractive index n,
related to the speed of light c in vacuum as v c/n. The scalar wave equation
follows from Maxwell’s theory. It may also be written in spherical coordinates
∇
2
u + k
2
u 0, (2.2)
where ∇ is the differential operator in spherical coordinates, k 2π/λ, and λ
is the wavelength of the light. A simple solution of this equation is a spherical
wave of the type (e
ikr
)/r, where r is the distance from the origin to the ob-
servation point. The spherical wave propagates from its origin in all directions
and its intensity is attenuated by 1/r
2
. We consider such spherical waves only
conceptually and approximate them at a large distance by plane waves.
The differential equation of the scalar wave equation is linear and superposi-
tion of solutions of the differential equation will again result in a solution. This
is part of the superposition principle. In this chapter we only need the superpo-
sition of a number of monochromatic waves, each of frequency ν, to result in a
monochromatic wave having the same frequency ν.
For our model description we use some results from Maxwell’s theory for
quantitative expressions of the reflection and transmission coefficients of mate-
rials contained in Fresnel’s formulas. In particular, we use the results that waves
pick up a phase jump of π, when reflected at an optically denser medium, and
that they travel in the optically denser medium with wavelength λ/n, where n is
the index of refraction. The intensity is calculated either as the time average of
the square of the amplitude or the square of the absolute value of the complex
representation and may be normalized with an arbitrary constant.
2.2 HARMONIC WAVES
The solution of the scalar wave equation, (Eq. (2.1)), is a function, depending on
the space coordinates x, y, z and the time t . In addition, there may be an arbitrary
phase factor. We consider harmonic waves in vacuum and in an isotropic and
nonconducting medium of index n. However, in most cases, we only need waves
depending on one space coordinate and time. We describe the transverse waves
by vibrating in the u direction and moving in the x direction, having wavelength
λ and time period T .
u A cos[2π(x/λ − t/T +φ)]. (2.3)
The amplitude u of the wave varies in the x direction, A is the magnitude of
the wave, and φ is a phase constant. The first graph of FileFig 2.1 shows the
amplitude u, depending on the space coordinate x for three time instances t and
three phase constants. The second graph shows the dependence on time for three
points in space and three phase constants. The magnitudes A
1
to A
3
and B
1
to
2.2. HARMONIC WAVES 81
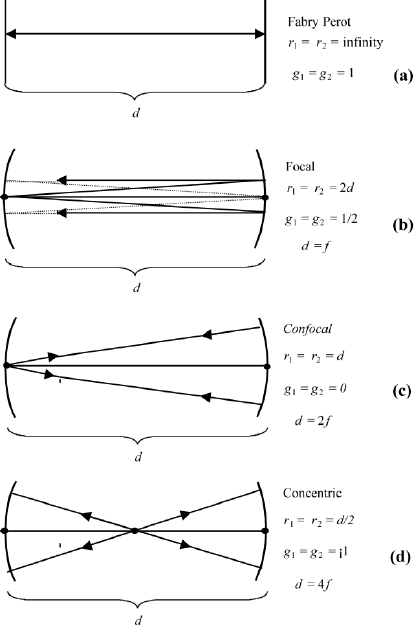
λ
1
λ
2
λ
1
FIGURE 2.1 Change of wavelength as the wave enters and leaves a dielectric medium.
B
3
have been assumed to have the same value, and the three phase constants
φ
1
to φ
3
and
1
to
3
are assumed to be different. Comparing the graphs, one
observes equivalence of the dependence of the cosine function on x/λ and t/T.
Changing the range of variable from x to t, the family of curves depending on x
is similar to the one depending on t.
We may modify x/λ and t/T in such a way that they contain phase constants.
Then, in the “net” expression cos[2π (x/λ−t/T +φ)] we can not distinguish if φ
belongs to the space part or the time part. We show below that for our discussions
on interference we do not need the time dependence and it is eliminated.
The product of the frequency ν and wavelength λ/n is equal to the phase
velocity v ω/k of the wave propagating in the medium of refractive index n.
The angular frequency ω 2πν, and the wave vector k 2πn/λ, where λ is
the wavelength in vacuum. We may then write Eq. (2.3) as
u A cos(kx − ωt) (2.4)
or
u A cos k(x − (ω/k)t) A cos k(x − vt).
The phase velocity in vacuum is c, and in an isotropic medium with refractive
index n it is c/n. The wavelength of “free space” λ is reduced in the medium to
λ/n (see Figure 2.1).
FileFig 2.1 (I1COSWS)
Cosine functions depending on space and time coordinate and one additional
phase constant. Graphs are shown for cosine functions depending on the space
coordinates for three time instances. This may be interpreted as graphs of the

82 2. INTERFERENCE
same wave at threeconsecutive snapshots. Graphs are shown for cosine functions
depending on the time coordinates for three points in space.
I1COSWS is only on the CD.
Application 2.1.
1. One may change the phase φ and the space coordinate and choose both so
there is no resulting change in the graph. Choose φ 2,4,6.
2. One may change the phase φ and the time coordinate and choose both so
there is no resulting change in the graph. Choose φ 2,4,6.
3. Change φ in such a way that there is a shift to smaller values of the position
coordinate.
4. Change φ in such a way that there is a shift to larger values of the time
coordinate.
2.3 SUPERPOSITION OF HARMONIC WAVES
2.3.1 Superposition of Two Waves Depending on Space and
Time Coordinates
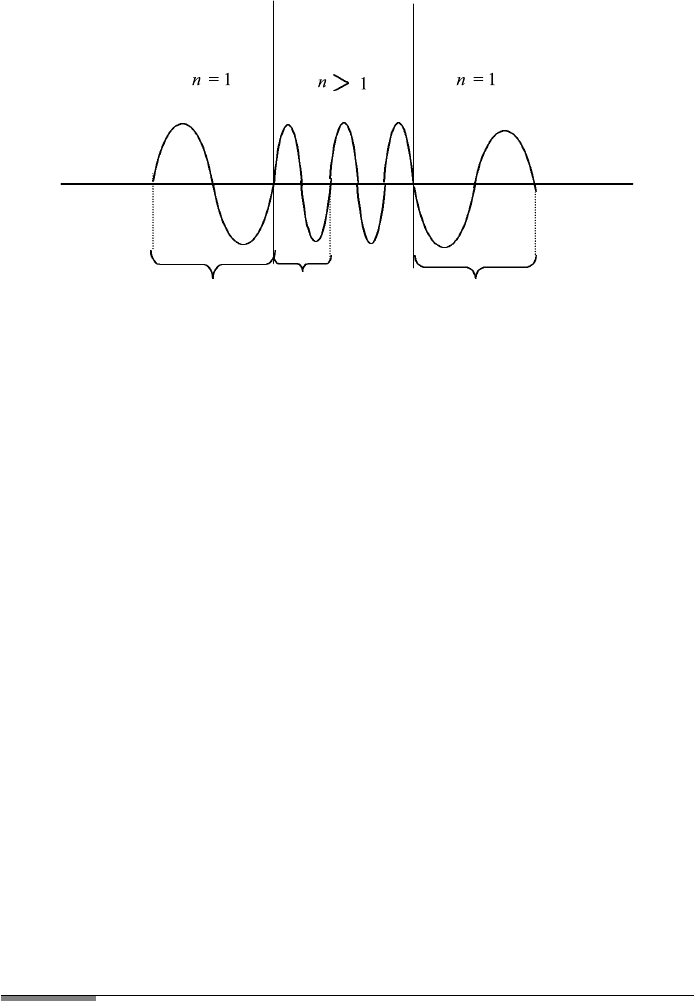
We describe the interference of two waves in a simple way, using the superpo-
sition of two harmonic waves u1 and u2. Both waves will propagate in the x
direction and vibrate in the y direction.
u
1
A cos 2π[x/λ − t/T] u
2
A cos 2π[(x − δ)/λ − t/T]. (2.5)
We assume that the two waves have an optical path difference δ.At time instance
t 0, the wave u
1
has its first maximum at x 0, and u
2
at x δ (Figure 2.2).
Adding u
1
and u
2
we have
u u
1
+ u
2
A cos 2π[x/λ − t/T] + A cos 2π[(x −δ)/λ − t/T]. (2.6)
Using
cos(α) +cos(β) 2 cos{(α − β)/2}cos (α + β)/2 (2.7)
we get
u [2A cos{2π(δ/2)/λ}][cos{2π(x/λ − t/T) − 2π(δ/2)/λ}]. (2.8)
In FileFig 2.2 we show graphs of the square of Eq. (2.8) for the same time instant
t
1
and wavelength λ. We choose a number of optical path differences δ
1
0,
δ
2
0.1, δ
3
0.2, δ
4
0.3, δ
5
0.4, δ
6
0.5, corresponding to the ratios
of the optical path difference to the wavelength between 0 and
1
2
. One observes
that the height of the maxima decreases with increasing δ
1
to δ
6
, and shifts to
larger values of x.
2.3. SUPERPOSITION OF HARMONIC WAVES 83
FIGURE 2.2 Two waves with magnitude A and wavelength λ.Wehaveu
1
A for x 0 and
u
2
A for x δ.
We nowdiscuss the twofactors of Eq. (2.8). The first factor 2A cos{2π (δ/2)/λ}
depends on δ and λ, but not on x and t. One obtains for δ equal to 0 or a multiple
integer of the wavelength
[2A cos{2π(δ/2)/λ}]
2
is 4A
2
(2.9)
and for δ equal to a multiple of half a wavelength
[2A cos{2π(δ/2)/λ}]
2
is 0. (2.10)
The first factor in Eq. (2.8) may be called the amplitude factor and is used for
characterization of the interference maxima and minima.
One has
maxima for δ mλ, where m is 0 or an integer (2.11)
minima for δ mλ, where m is
1
2
plus an integer (2.12)
and m is called the order of interference.
The second factor is a time-dependent cosine wave with a phase constant
depending on δ and λ. For the description of the interference pattern this factor
is averaged over time and results in a constant, which may be factored out and
included in the normalization constant (see below).
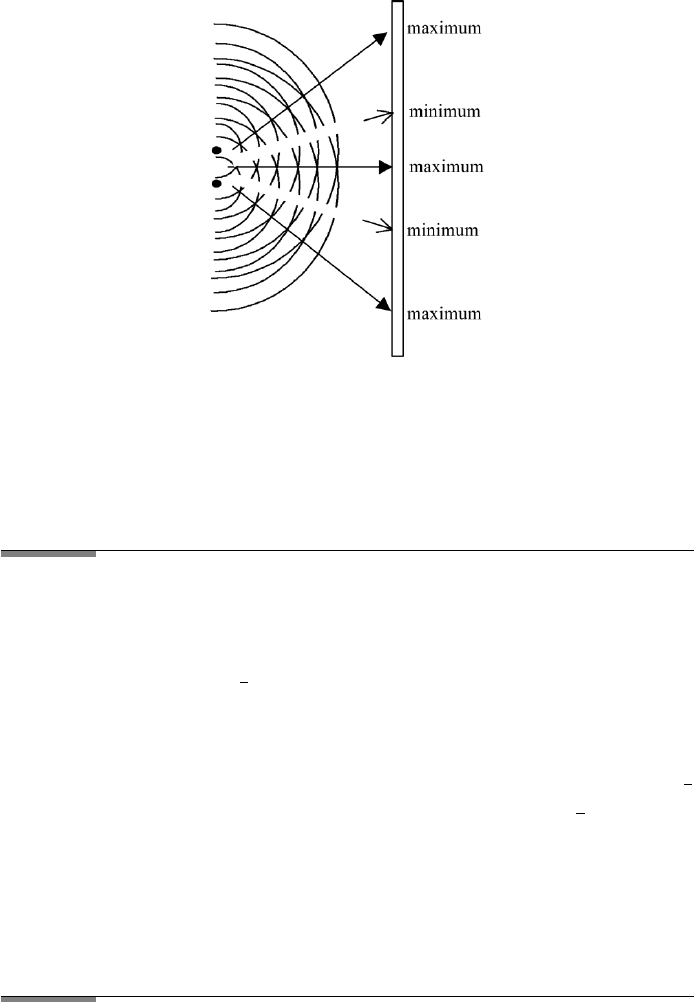
In Figure 2.3 we show schematically the interference of two water waves
with a fixed phase relation. When the interference factor is zero one has minima,
indicated by white strips. They do not depend on time. The maxima oscillate and
appear and disappear along the line in the observable direction.
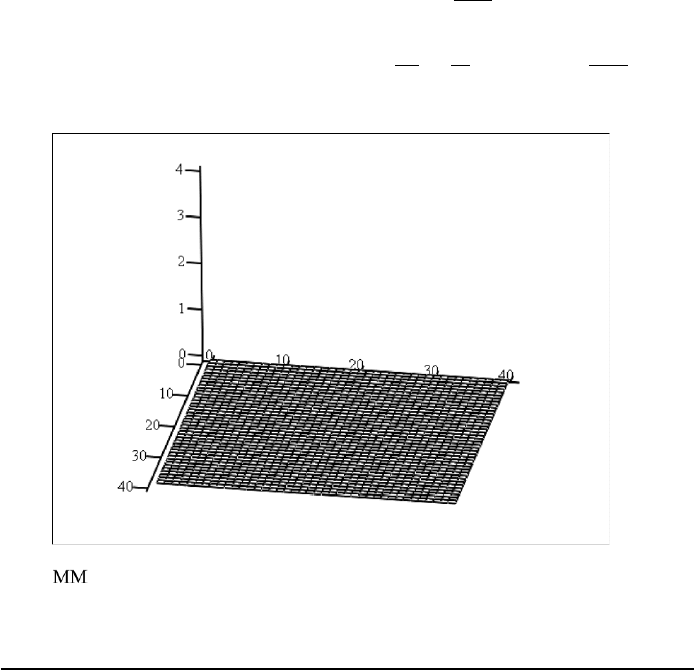
Maxima and minima are shown in FileFig 2.3 as 3-D graphs. The maxima
are shown for δ λ, and in the second graph, for δ λ/2, there is just one
minimum. The maxima show the time dependence of the second factor for each
of the space coordinates. One can estimate that a time average will result in half
the maximum value. The minimum is zero. It is zero for all time.
84 2. INTERFERENCE
FIGURE 2.3 Schematic of the interference pattern produced by two sources vibrating in phase. At
the crossing of the lines, the amplitudes of the waves of both sources are the same and adding. Taking
the time dependence into account, the magnitude changes between maximum and minimum.
These are the maxima when considering light. Between the maxima we indicate the two lines
corresponding to the minima. Along these lines the amplitude of the two waves compensate each
other; their sum is zero for all times.
FileFig 2.2 (I2COSSUPS)
Graphs of the superposition of two cosine waves with wavelength λ 1, for
a number of optical path differences δ
1
0, δ
2
0.1, δ
3
0.2, δ
4
0.3,
δ
5
0.4, δ
6
0.5 corresponding to ratios of the optical path difference to the
wavelength between 0 and
1
2
.
I2COSSUPS is only on the CD.
Application 2.2.
1. Extend the range of the optical path differences of the six graphs from
1
2
wavelength to 1 wavelength, and then from 1 wavelength to
3
2
wavelength
and indicate in a list when there is repetition.
2. Make a graph of y cos{2π(δ/2)/λ}for fixed λ as function of δ and make a
list of the δ values for minima and maxima. Compare with a list of δ/λ values.
FileFig 2.3 (I3COSGRA)
3-D demonstration of the superposition of two waves for δ/λ 1 corresponding
to a maximum, and δ/λ 0.5 corresponding to a minimum. In the graph of the
maximum, the amplitude changes in time for a specific spot in space between
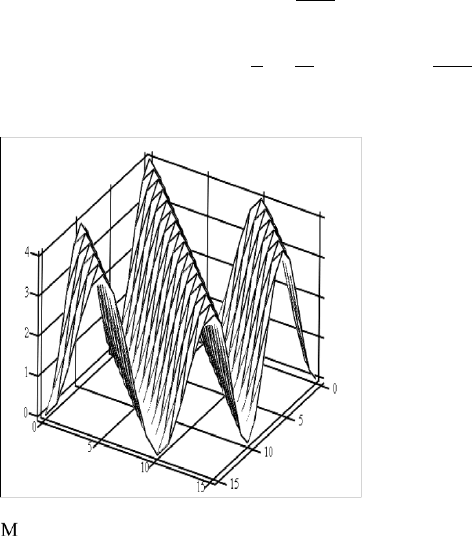
2.3. SUPERPOSITION OF HARMONIC WAVES 85
0 and (2A)
2
, and one can estimate that the time average will be half of it. The
graph of the minimum is zero for all time and space values.
I3COSGRA
Superposition of Two Cosine Waves
One wave has optical path difference δ with respect to the other. The sum is
squared to result in the intensity. We are looking at them time dependence; the
graphs are plots in space x and time t. Period T , path difference δ, wavelength λ.
1. Graph for optical path difference corresponding to a maximum
λ : 1 A : 1
N : 40 i : 0 ...N j : 0 ...N
x
i
:−.2 + .05 · it1
j
:−.2 + .05 · j
uc(x, t1) :
2 ·A · cos
2 ·π ·
δ1
2 ·λ
·
cos
2 ·π ·
x
λ
−
t1
T
− 2 ·π ·
δ1
2 ·λ
2
M
i,j
: uc
x
i
,t1
j
δ1 ≡ 1 T ≡ 1 t1 ≡ .1.
2. Graph for optical path difference corresponding to a minimum
N : 40
i : 0 ...N j : 0 ...N
xx
i
:−.2 + .04 · it1
j
:−.2 + .02 · jδ2 ≡ .5
86 2. INTERFERENCE
ud(xx, t1) :
2 ·A · cos
2 ·π ·
δ2
2 ·λ
·
cos
2 ·π ·
xx
λ
−
t1
T
− 2 ·π ·
δ2
2 ·λ
2
M
i,j
: ud
xx
i
,t1
j
t1 ≡ .1 T ≡ 1.
Application 2.3. One may change the wavelength λ such that for δ1/λ one gets
minima, and for δ2/λ one gets maxima.
2.3.2 Intensities
The interference pattern of water waves is an amplitude pattern. We may observe
minima and maxima with respect to the level of the undisturbed water surface.
The interference pattern of light shows intensity minima as dark spots in space
and maxima as bright spots. An amplitude pattern shows negative amplitudes,
but an intensity pattern has only positive or zero values. The amplitude pattern
has to be considered first; it produces interference. Then we have to obtain the
intensity pattern. We compare the intensity pattern to observations.
For the square of the amplitude of equation (2.8) we have
u
2
[2A cos{2π(δ/2)/λ}]
2
[cos{2π(x/λ − t/T) − 2π(δ/2)/λ}]
2
(2.13)
In Section 2.1 we mentioned that for the intensity we use either the time average
of the square of the amplitude or the square of the absolute value, when using
complex notation. In this section we compare these two calculations.
