Mindlin R.D., Yang J. An Introduction to the Mathematical Theory of Vibrations of Elastic Plates
Подождите немного. Документ загружается.

First-Order Approximation
151
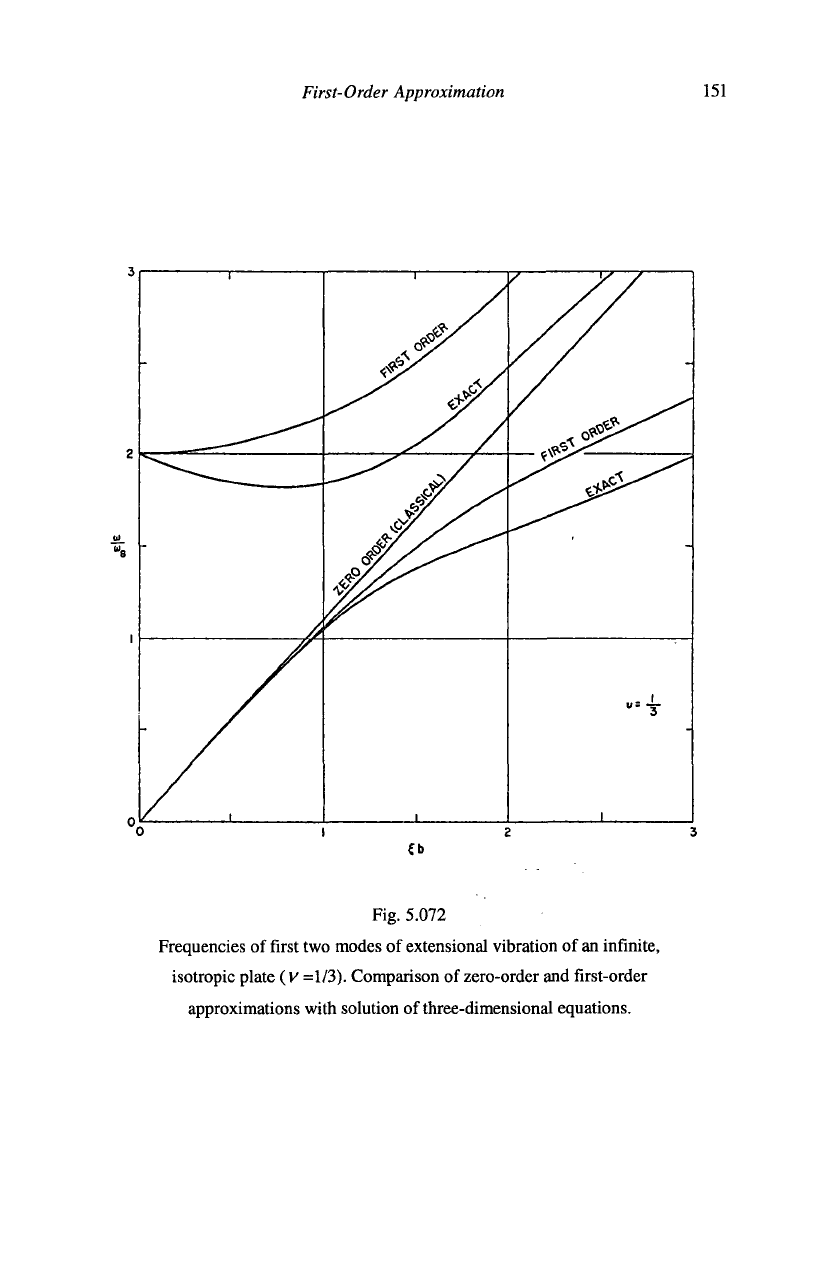
Fig.
5.072
Frequencies of first two modes of extensional vibration of an infinite,
isotropic plate (V =1/3). Comparison of zero-order and first-order
approximations with solution of three-dimensional equations.

Chapter 6
Intermediate Approximations
6.01 Introductory
The equations of the first-order approximation take account of four
types of motion of an infinite plate. In order of decreasing frequency they
are:
thickness-stretch, thickness-shear, face-extension and
thickness-flexure. In an isotropic plate, thickness-shear is coupled with
thickness-flexure and thickness-stretch is coupled with face-extension. In
a crystal plate, all four motions are coupled. There are many applications
in which it is not necessary to take the full coupling into account. For
example, if the terms accommodating the thickness-stretch mode are
dropped, the remaining equations are useful up to frequencies somewhat
above that of the thickness-shear mode. Further simplification, by
neglect of the rotatory inertia, leads to equations which accommodate
face-extension and moderately high-frequency thickness-flexure. Finally,
suppression of the thickness-shear deformation leaves equations suitable
for low-frequency thickness-flexure. At any stage of the process of
reduction, the coupling between extension and flexure, in a crystal, may
be eliminated with resulting simplification of the equations and with,
nevertheless, some remaining useful ranges of application. Also, at any
stage, another expansion may be made in a series of powers of a
face-coordinate. This restricts the useful range to modes having a limited
number of phase reversals along that coordinate in addition to the
limitation with respect to the thickness-coordinate.
153
154 Mathematical Theory
of
Vibrations
of
Elastic Plates
6.02 Thickness-Shear, Thickness-Flexure and Face-Extension
The thickness-stretch vibrations
may be
eliminated from
the
first-order approximation
by
setting
«£>
=
F«
=
7f
= T™ =
7f>
=0
(6.021)
These conditions, rather than
the
condition u!p
=
0,
are
adopted
in
order
to permit free development
of
the strains illustrated
in
Fig.
4.011.
As a
result
of
(6.021)
the
fifth
of the six
equations
of
motion (5.0117)
disappears.
The
extensional motions that
are
left
are
those
of the
zero-order approximation while the thickness-shear
and
flexure remain
at
the first-order approximation.
With r
2
(0)
=0,the zero-order stress-strain relations
are
obtained
in
the usual manner
by
using
the
second
of
(5.041)
to
eliminate
the
strain
S^ • hi the
case
of the
first-order stress-strain relations, three
components
of
stress
(
r
2
(1)
, r
4
(1)
,
r
6
(1)
) are
zero. When
so
many
components
of
stress
are
absent, simpler expressions
for
stress
in
terms
of strain
are
obtained
by
employing elastic compliances
s
pq
instead
of
stiffnesses c
pq
.
In
the present case
we
have
|i
3
5«=5
11
r«
+Jl3
7',«
+
,
15
r«
-b'Sl
l)
=
s
3i
T
{
m
+ s
33
T
3
m
+
s
35
T
5
m
(6.022)
l/,3o(i)
_
r
(i) ,
r
(D , .
r
(i)
— O
^
5
-S
sl
l
l
+^53
i
3
+5
55-
Z
5

Intermediate Approximations
155
When these are solved for the components of stress, there result
?f
=
f^M
0
^^*^)
(6-023)
where
Yu =(s33
J
S5-4)
/A
Y35 =r53=(
S
n
S
l5-
S
U
S
3s)
/A
r
33
=(y
S
5
J
n--
s
5i)
/A
ftl =
^15
=
(^35^31
-
^33^51
)/
A
{6.Q24)
Y55
=
\
s
u
s
33~
s
n)'^
Yl3 = r31
=
(
J
51^3-^55%)
/A
A — S
U
S
3i
S
S5
+
^
,S
3S
S
5l
S
li 5
U
5
35
S33S51
S
55
S
n
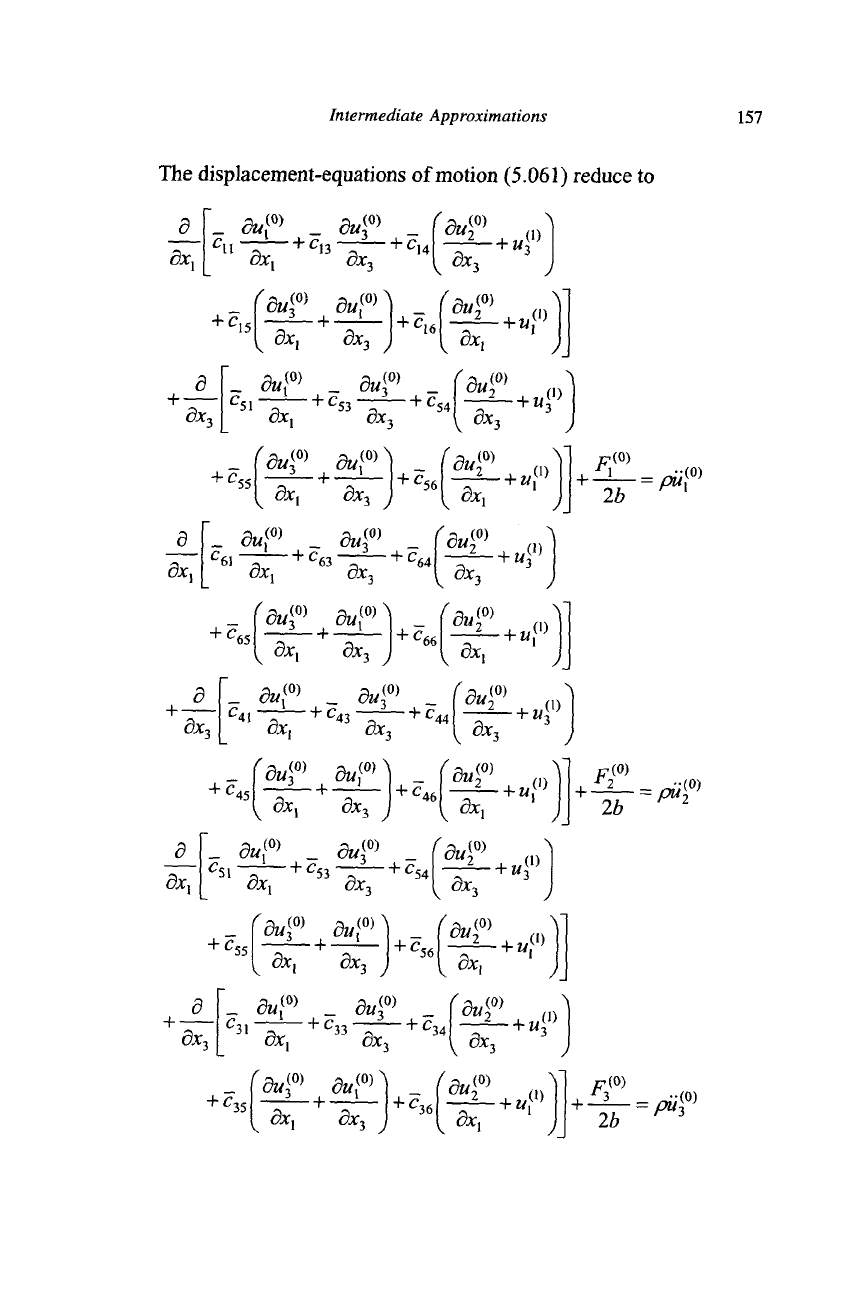
Hence, for the triclinic crystal, the stress-displacement relations (5.051)
reduce to
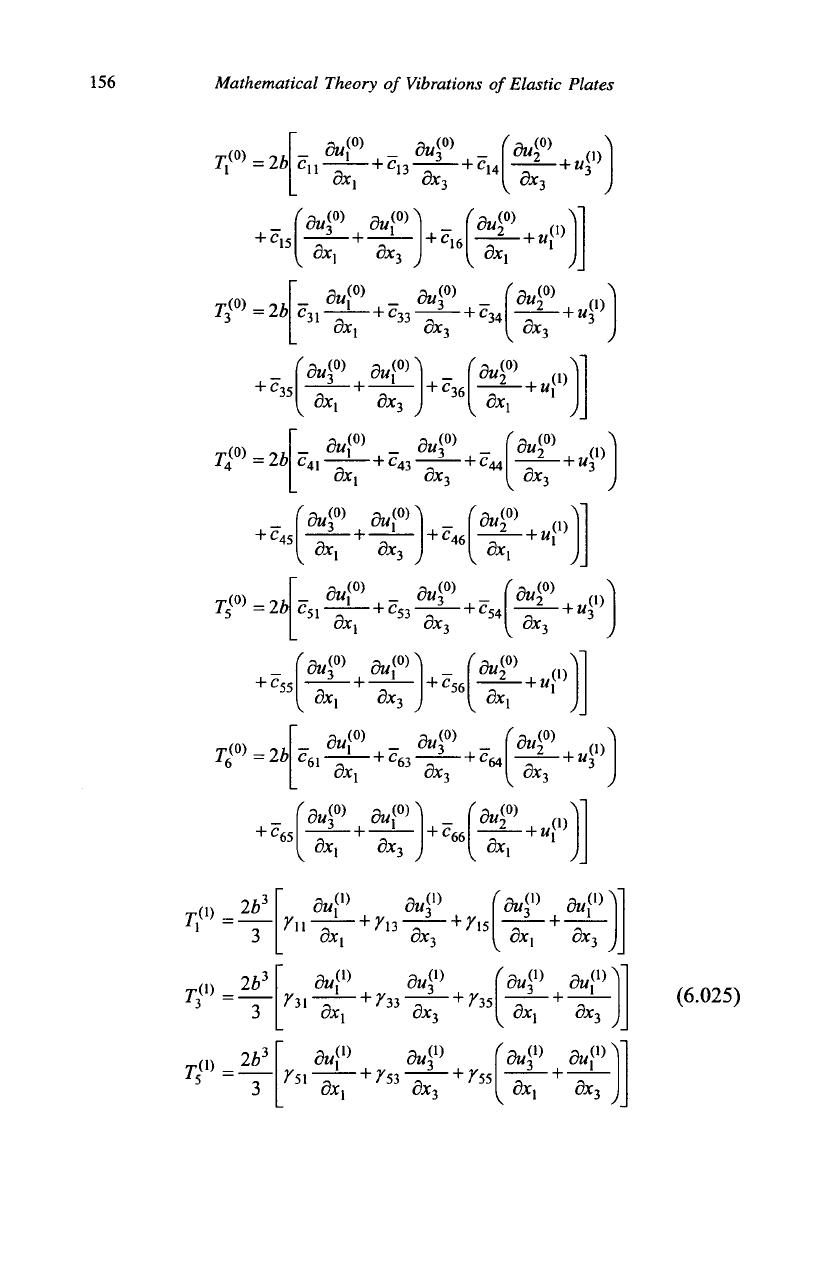
Mathematical Theory
of
Vibrations
of
Elastic Plates
T.
w
=2b
_
du\v _ a,(o) _ ^(0) •
c
ll
+
c
13
+
c
14 — +
U\'
dx
x
dx
3
I
fit
3
I
SXTJ
3x
3
I I 3x,
,.(0
J_
a
Ml
(0
>
_
a,<°>
_ (du^ ,'
rr=2b c
31
—
L
-+c
3
3-^-+c
34
^
1
-+«
1)
ax,
ox
3
1 ax
3
+C35 ^
i
-
+
^
i
- +
c
36 -r-+«i
^
3x, dx
}
) [dXi )
r
4
(0)
=26
C41
_i_
+C4
3-JL-
+C44
-^-+
M
1}
ax,
ax
3
1 0x3
+ c
45
fie,
A
/
ax,
3x
;
:
3;
+
c
46
Sxi
^J
T^=2b
8u{
0)
_ &,<»> _
~^-
+c
»^x7
+c
»
ax,
,(0)
f
ft
(0)
8x3
+ c
55
T^=2b
(a^
a*}
0
*'
[
0)
1
-
N
0)
(1)
'
•l
) {SX
{
dx
x
dx
3
J I ax,
"_
a
M
,
(0)
_
a*<
0)
_
c
61~^
+C
63"T
hc
64
ax, cbc
3
dx
2*1
3
2*1
3
r
(0 _
2
*
3
y(l)
_
1
" 3
73
" 3
a«f
a^M _ (a«f ,„
ax,
ax
3
1 1 ax,
'a,™
a»,
(l
—£_+—1.
a«,
(1)
a,™
i^—+rn-r-
+
ri5
fix,
ox
3
a*,
,(')\
ax,
a*
3
11
a«,
(1)
a
w
«
fa,?*
a«,
(1
>
ctc,
ax
3
'
du[
l)
du^
r
5
i^
L
-+r53^—+r55
ax,
ax
3
ax,
ax
3
a»f
,
__
(du? du^
ax
3
3x,
dx
•3
7
(6.025)
Intermediate Approximations
157
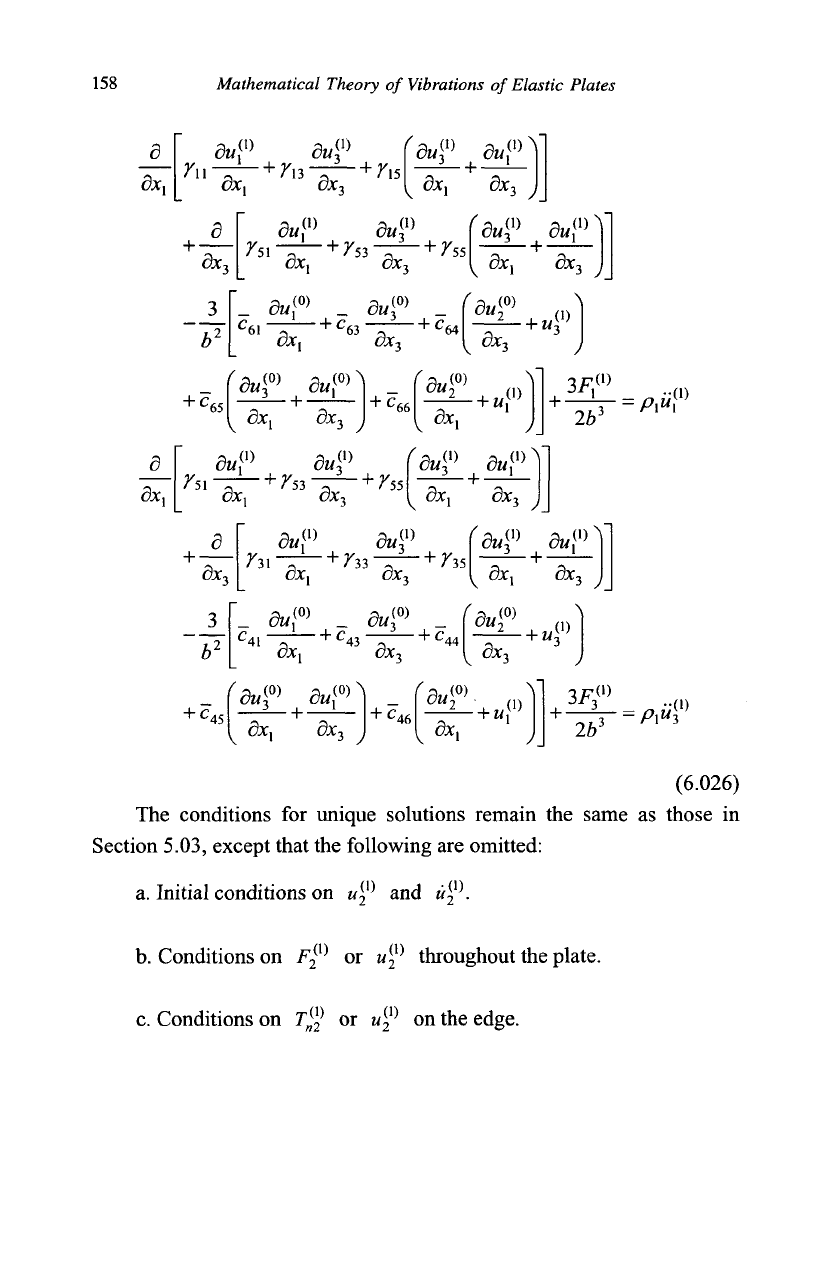
The displacement-equations of motion (5.061) reduce to
fix,
_ du
(0)
dx
{
•
+ c,
duf_
fix,
+
c,
14
fix,
J
+ c
15
et/f du™
fix,
fix
+ c,
16
3 ;
fix,
fix,
_
anj
0
>
_
a«<
0)
_
-51
fix,
•
+ c
53
Sx,
•
+ c.
54
8»f
fix,
+
M
(1)
+
c.
55
fix,
du
W\
fix
+ c,
56
3 J
(du™
fix,
+
W
(1)
fix,
_
fi
M
,
(0)
_ du™
-61
fix,
•
+ c,
63
fix,
-
+
c,
64
fix,
+ u
(1)
+ c,
65
du™ 5
M
[°M
fix,
fix
+
c,
66
3 y
a*4
0)
fix,
+ w
(1)
+
-
fix,
fix,
_ fiw
(0)
+ c,
43
fix,
•
+ c,
44
du™
fix,
+
w
(1)
_ du™ du™
+
C
4
5-
J
—«"
fix
fix,
_
5
W1
(0)
C
51
—
;
+c
53
fix-
+
c
46
3 j
fix,
+
W
(1)
26 ^
2
fix,
_ 6wf^
fix:,
+
c,
54
du™
fix,
+ M
(1)
+
C
55
fiw<
0)
fi
M
,
(
— + '
fix,
(0)^
fix
+
c.
56
3 >/
(du™
fix,
+
W,
(1)
/
fix,
_
fi
Ml
(0)
_ du™ _
/
fix,
•
+ c
33
fix,
+
c
34
*£.•.?>
fix
+
c
35
'e
M
<
0)
,
fi
Ml
(0
M
fix,
-
+
-
dx
+
a
36
3 J
du™
dx,
+
u
(i)
158 Mathematical Theory of Vibrations of Elastic Plates
dx,
Y\
du\
l)
dx,
+ rn-^-+ri5
fix,
——+- '
fix, dx
3 )
+
-
fix
3
b
1
du\
x)
dul_
7i\
a
+
^53 , + /55
fiXj
OX
3
_
fi
W
<
0)
_ du™
dx,
•
+ c,
+
c,
(du™
65
8u
63
(0)^
fix,
+
c,
64
fi
M
<
0)
fiw
(D^
fix
3 J
\
dx-,
+
14
(1)
J
dx
x
fix
3
+ c,
66
(duT
fix,
•
+ u
(i)
3F
(1)
2b
dx,
rsi-^+r
5
3-^—+r55
fdu
m
dx dx
v
8x
i
3 Ju?
fix,
+
-
fix
3
_3_
b
2
dul
l)
dui
l)
r3i-^—+Yx-r-
+
r35
fix,
c ^
dx,
fix
3
_ dui
0)
dx-,
•3 j
du[
n
du
m~\
•
+ c
43
•
+ c
V
dx
\
44
V
8X
3
•
+ u
3 )
\
+
C
45
5
+
•
fix- fix,
+ c
46
•
+
w
(i)
3
F
(D
(6.026)
The conditions for unique solutions remain the same as those in
Section 5.03, except that the following are omitted:
a. Initial conditions on u^
}
and it^.
b.
Conditions on F
2
(1)
or u^ throughout the plate.
c. Conditions on r
n
y or u\' on the edge.
Intermediate Approximations
159
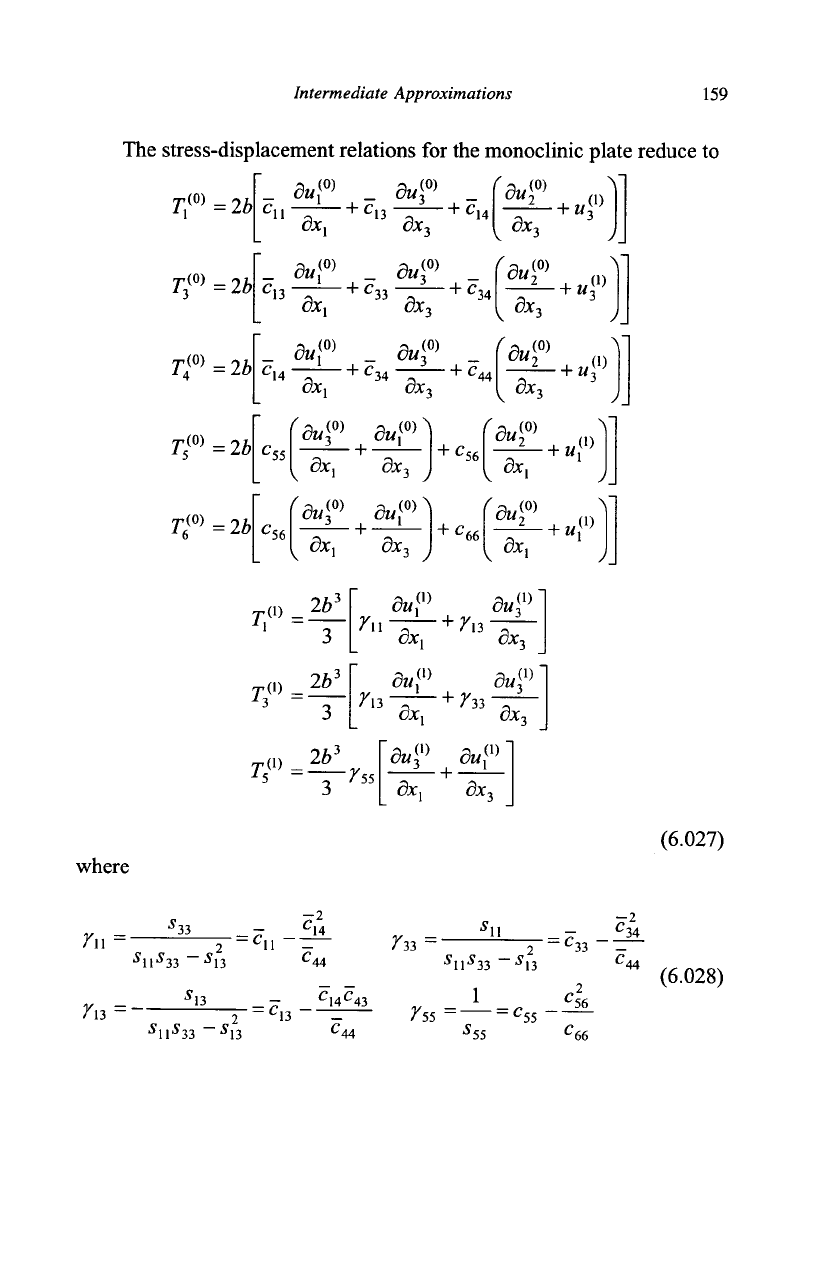
The stress-displacement relations
for
the monoclinic plate reduce
to
T^=2b
Tj
0)
=2b\
du\
0)
dx,
da™
dx,
+
c
13
duj
0)
dX-,
+
c
14
+
C
_
a«<
0)
33
Sx,
+
c
34
,(0)
••2b
'14
du{
0)
dx,
+
c
34
du^_
dx?
du^
dx-,
+
14
(1)
+
c
44
dx
3
dx,
+
u
(i)
+
u
(1)
f
)
=2A
;
(0)
=2Z>
/
C
55
V
/
C
56
V
a
M
<
0)
a^
dx,
+
•
3w
(0)A
&o
+
c
56
+
•
5M
(0)^
dx-,
dul_
dx.
+
u
(i)
+
c,
66
a«<
0)
5x,
+
W
(1)
7-0)
-
r(')
-
J
3
_
J
5
_
26
3
3
2b
3
Y\\
Yn
2b
3
•Y55
du[
l)
du?
—
+
Y\i——
dx
{
dx
3
dul
l)
du?~
dx
x
dx
3
—— + ——
8x
i
dx
i
where
(6.027)
Yn
Yn
5
33
•
= c
11
-
C
44
_-
C
14
C
43
' — C17
S,,S
11
J
33 '44
^33=-
c
2
J
n
5
3
3
5
13
Y55=
=
C
;
55
3
55
C
33-"
C
66
34
'44
(6.028)
160
Mathematical Theory of Vibrations of Elastic Plates
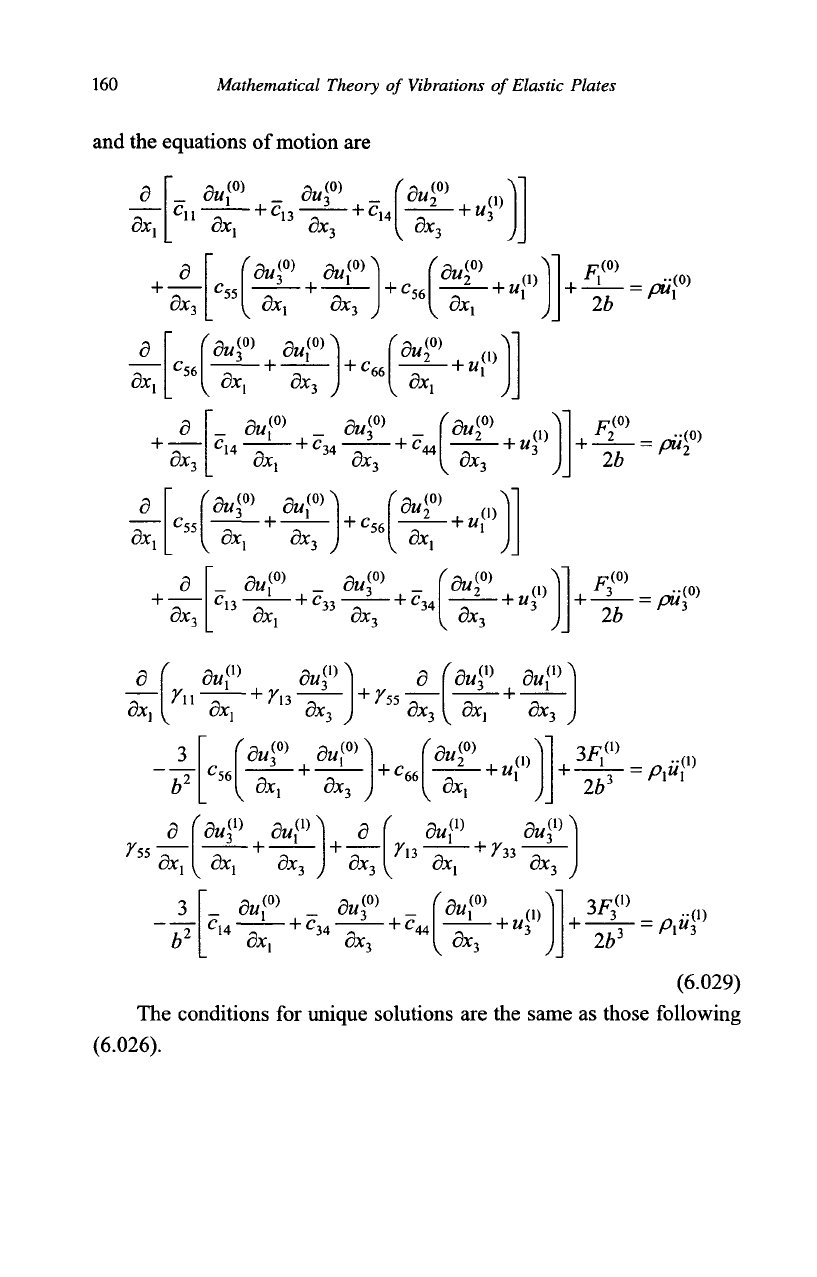
and the equations of motion are
dx,
du\
(0)
dx,
•
+ c,
duj
0)
dx?
+ c
14
V
&
3
•
+ u
(1)
dx,
'55
(du™ du{
0)
)
—
+
!
^ &c, dx
3
)
+
c
56
dx,
•
+ u
(i)
/r«»
5x,
'du™ du?A (du™
'56
V
dx
\
'3
.
+
-1
dx
+
c,
66
3 ;
V
dx
i
+ U
(1)
+
-
fix,
_
a«
(0
>
. - *r -
14
„
+C
34 -
+C
44
(duf
dx dx,
V
dx
i
+
u
(i)
C-(O)
dx,
'55
a«<
0)
e
Ml
(0)>
l
v
dx
\
dx
+ c
(<**>
56
3
)
\
dx
\
+ U
(1)
+
-
dx,
du™ _ du™
fix,
•
+ c
33
fix,
•
+ c
34
V
&
3
+ U
(1)
+
dx,
Vu
du™
dx,
+ ri3-
du
w\
dx
3 ;
fa,.m
+
755
dx,
du
(
,
l>
du,
i
+ '
(n\
y dx, dx
3
j
fa..V»
'56
(oA
—— +——
dx
x
dx
3
+ c,
66
(du™
v
&
i
•
+
W
(1)
r
55
^
f
duf du\
dx
QA
• +
•
v
&, 5x
3
,
dx,
r.
:
e
M
,
(1)
V
dx,
+ riy
du
0>^
Sx
3 ;
_ du™
c
, _ 3K<
0)
_
14
^ +
C
34 ~ +
C
44
dx,
du
V
5
*3
+ W
(1)
2fe
3
-A»3
Sx,
^
3
(6.029)
The conditions for unique solutions are the same as those following
(6.026).
