McMahon D. String Theory Demystified

Подождите немного. Документ загружается.

136

String Theory Demystiﬁ ed

The leftover term multiplying the inﬁ nitesimal

∂

is our conserved current.

Being that we started with a translation of space-time coordinates, we identify this

as the momentum:

PTX

=∂

With Example 7.3 in mind, we can easily ﬁ nd the conserved supercurrent, which

is the conserved current associated with the supersymmetry transformation. Let’s

just grind it out. Starting with

LT XXi=− ∂ ∂ − ∂/( )2

µα

αµ

ψρ ψ

, we have

δδψρψψρδψ

µα

αµ

µα

αµ

XXi i

=−

∂∂− ∂−∂

2( ) ( ) (

))

() ( )

⎡

⎣

⎤

⎦

=− ∂ ∂ + ∂ ∂ −

µα

αµ

µα

εψ ερ ρ ψ ψ ρ

∂∂∂

⎡

⎣

⎤

⎦

=− ∂ ∂ −∂ ∂

µα

ρε

εψ ερ

()

() (

))()

()

ρψ ψ ρ ρ ε

εψ

µα

βµ

µα

−∂∂

⎡

⎣

⎤

⎦

=− ∂ ∂ −∂

(()

() (

ερ ρ ψ

εψ ε ρ

µα

∂

⎡

⎣

⎤

⎦

=− ∂ ∂ −∂ ∂

TX X

))()

()

ρψ ερρ ψ

εψ εψ

βα

αβ

µα

−∂∂

⎡

⎣

⎤

⎦

=− ∂ ∂ −

µµ

µα

βα µ

βµ α

ερρψ ε ψ

∂∂ −∂ ∂ + ∂∂

⎡

⎣

⎤

⎦

=−

XXX

()()

∂∂∂−∂ ∂

⎡

⎣

⎤

⎦

µα

βα µ

βµ

εψ ε ρ ρ ψ

()( )XX

The ﬁ rst term is a total derivative, so it does not contribute to the variation of the

action. So we identify the conserved current with the second term. It is taken to be

µβ

βµ

ρρψ

=∂

(7.13)

The Energy-Momentum Tensor

The next item of interest in our description of strings with worldsheet supersymmetry

is the derivation of the energy-momentum tensor. The energy-momentum tensor is

associated with translation symmetry on the worldsheet. Consider an inﬁ nitesimal

translation

which is used to vary the worldsheet coordinates as

σσε

ααα

→+

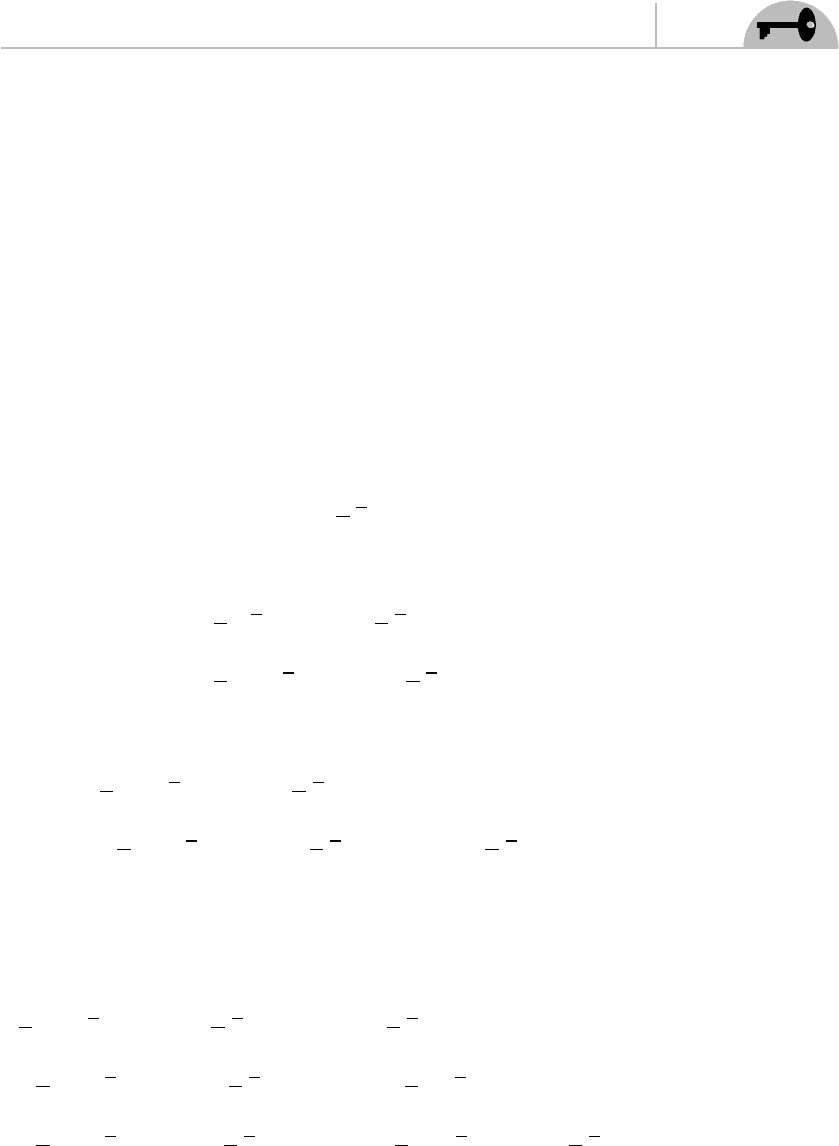
We can write the change of the bosonic ﬁ elds

by basically writing down their

Taylor expansion:

XX X

µµα

→+∂

(7.14)

A similar relation holds for the fermionic ﬁ elds:

ψψεψ

µµα

→+∂

(7.15)

With this in mind, we again follow the Noether procedure. Vary the action as if

depended on the worldsheet coordinates, and look for terms multiplied by

∂

. At

the end we consider

to be constant so that term vanishes from the action—the

term which multiplies

∂

will be the energy-momentum tensor that we seek.

We proceed in two parts. Let’s take a look at the fermionic part of the lagrangian

ﬁ rst. We have

=− ∂

ψρ ψ

µα

αµ

Using Eq. (7.15), we vary this term as follows:

δδψρψψρδψ

εψ

µα

αµ

µα

αµ

=− ∂ − ∂

=− ∂

() ()

(

))()

ρψ ψρ εψ

αµ

µα

βµ

∂− ∂∂

Let’s apply the product rule and carry out the derivative on the second term:

−∂ ∂− ∂∂

=− ∂

() ()

(

εψρψ ψρ εψ

µα

αµ

µα

βµ

ψψρψψρεψψρεψ

µα

αµ

µα

βµ

µαβ

αβ µ

) ∂− ∂∂− ∂∂

Now, the variation actually takes place as a variation of the action S, so we can

integrate by parts. We do this on the last term to move one of the derivatives off

∂∂

αβ

. Integration by parts introduces a sign change, so we get

−∂ ∂− ∂∂− ∂

iii

222

()

εψρψ ψρεψ ψρε

µα

αµ

µα

βµ

µαβ

∂∂

=− ∂ ∂ − ∂ ∂ + ∂

βµ

µα

αµ

µα

βµ β

εψρψ ψρεψ

iii

222

()

(()

()

ψρε ψ

εψρψ ψρε

µαβ

αµ

µα

αµ

µα

∂

=− ∂ ∂ − ∂ ∂

ββµ

µα

αµ

µα

αµ

ψεψρψψρεψ

+∂ ∂+ ∂∂

CHAPTER 7 RNS Superstrings

137

138

String Theory Demystiﬁ ed

The divergence term

∂

is not going to contribute anything, so we drop it. The

ﬁ rst and third terms cancel, leaving us with

δεψρψ

βµα

βµ

=∂ − ∂

⎛

⎝

⎜

⎞

⎠

⎟

This is what we want, because terms that multiply

∂

are going to be terms that

make up the energy-momentum tensor

. This isn’t quite right, because we want

it to be symmetric. So, we take

δεψρψψρψ

βµα

βµ

µβ

αµ

=∂ − ∂ − ∂

⎛

⎝

⎜

⎞

⎠

⎟

(7.16)

In the Chapter Quiz, you will derive an expression for the bosonic part of the

energy-momentum tensor. When all is said and done

TXX

αβ α

βµ

αβ µ

βα µ

ψρ ψ ψρ ψ

=∂ ∂ + ∂ + ∂ −

()Trace

(7.17)

The “Trace” is explicitly removed to ensure that

αβ

remains traceless as required

for scale invariance.

The energy-momentum tensor and supercurrent can be written compactly using

worldsheet light-cone coordinates. The energy-momentum tensor has two nonzero

components given by

TXX

++ + + + + + −− − − − −

=∂ ∂ + ∂ =∂ ∂ + ∂

µµ

ψψ ψ

ψψ

−

(7.18)

The components of the supercurrent are

JXJX

+++ −−−

=∂ =∂

ψψ

(7.19)

The equations of motion for the fermion ﬁ elds are

∂=∂=

+− −+

ψψ

µµ

0 (7.20)

Together with the equations of motion of the boson ﬁ elds

∂∂ =

+−

(7.21)

CHAPTER 7 RNS Superstrings

139

We obtain conservation laws for the energy-momentum tensor:

∂=∂=

−++ +−−

TT0

(7.22)

EXAMPLE 7.4

Show that the equations of motion for the fermion and boson ﬁ elds lead to

conservation of the supercurrent.

SOLUTION

We start with

and consider the derivative

∂

−+

. We have:

∂=∂ ∂

=∂ ∂ + ∂∂

−+ − + +

−+ + + −+

()

() ( )

ψψ

The result was readily obtained using Eqs. (7.20) and (7.21). Now taking

−

, we

obtain a second conservation equation by calculating

∂

+−

which gives

∂=∂ ∂

=∂

()

∂+∂∂

()

+− + − −

+− − − +−

−

()

ψψ

µµ

()∂∂ =

−+

X 0

EXAMPLE 7.5

Show that

∂=

−++

T 0

SOLUTION

Using

TXXi

++ + + + + +

=∂ ∂ + ∂

µµ

ψψ

, we ﬁ nd

∂=∂∂∂+ ∂

⎛

⎝

⎜

⎞

⎠

⎟

=∂∂ ∂

−++ − + + + + +

−+

TXX

µµ

ψψ

()

++ + −+ −+ ++ +−+

+∂ ∂ ∂ + ∂

()

∂+∂∂XX X

µµ

ψψ ψ ψ

()

+−++ ++−+

=∂∂=∂∂=

ψψ ψψ

To obtain this result, we applied Eqs. (7.20) and (7.21) together with the

commutativity of partial derivatives.

140

String Theory Demystiﬁ ed

Mode Expansions and Boundary Conditions

The ﬁ nal step in putting together the classical physics of the RNS superstring

follows the program used in the bosonic case—we need to apply boundary conditions

and write down the mode expansions. Speciﬁ cally, we need to apply boundary

conditions for the fermionic ﬁ elds. It is simplest to continue working in light-cone

coordinates and vary the fermionic part of the action. Before doing this, it can be

helpful to review some elementary calculus.

Recall integration by parts:

dx fg

gxdx

() ()=−

∫∫

The product

is called the boundary term. When we vary the fermionic action, we

are going to obtain boundary terms for the ﬁ elds

, so we need to specify boundary

conditions so that the variation in the action vanishes. The fermionic part of the

action in light-cone coordinates, modulo a few constants and ignoring the space-

time index is

=∂+∂

∫

−+ − +− +

σψ ψ ψ ψ

()

(7.23)

For simplicity, let’s consider one piece of this expression and vary it. We obtain

δ σψψ σδψψψ δψ

∫∫

+− + +− + +− +

∂= ∂+∂[()]

Following the usual procedure applied in ﬁ eld theory, we want to move the derivative

off the

δψ

term. This can be done using integration by parts. When this is done, we

pick up a boundary term:

ddd

σ ψ δψ τ ψ δψ σ ψ δψ

σπ

∫∫∫

+− +

−∞

∞

++=

−+ +

∂= −∂()

A similar expression arises from the variation of the other term. All together, the

boundary terms obtained by varying the action are

δ τ ψδψ ψδψ ψδψ ψδψ

σπ

=−−−

−∞

∞

++ −−= ++ −−

∫

()()

σσ

{}

(7.24)

CHAPTER 7 RNS Superstrings

141

OPEN STRING BOUNDARY CONDITIONS

When varying the action, the boundary terms must vanish in order to maintain

Lorentz invariance. In the case of open string, the boundary terms

= 0

and

must both vanish independently. We can obtain

ψδψ ψδψ

++ −−

−=0

= 0

if we take

ψτψτ

µµ

+−

=(,) (,)00

(7.25)

Now in general,

ψψ

+−

=±

will make the boundary terms vanish, but typical convention

is to ﬁ x the boundary condition at

= 0

using Eq. (7.25). This leaves the choice of

sign at

σπ

ambiguous. Depending on the sign we choose, we obtain two different

boundary conditions. Ramond or R boundary conditions are given by the choice

ψπτ ψπτ

µµ

+−

=( , ) ( , ) (Ramond)

(7.26)

The other choice we can make is known as Neveau-Schwarz or NS boundary

conditions:

ψπτ ψπτ

µµ

+−

=−( , ) ( , ) (Neveau-Schwarz)

(7.27)

We often refer to the boundary conditions chosen as the sector. The choice of

boundary conditions has dramatic consequences. In particular

• The R sector gives rise to string states that are space-time fermions.

• The NS sector gives rise to string states that are space-time bosons.

OPEN STRING MODE EXPANSIONS

We consider the R sector ﬁ rst. The mode expansions are

ψστ

µµτσ

µµ

−

−−

−

∑

(, )

()

iin

()

τσ

∑

(7.28)

142

String Theory Demystiﬁ ed

The Majorana condition is the requirement that the fermionic ﬁ elds are real. This

forces us to take

nn−

()

µµ

†

(7.29)

Here, the summation index is an integer and so runs

=±±012,, ,…

The NS sector results in different mode expansions, as you might guess, since

this gives rise to different string states. The expansions are

ψστ

µµτσ

µµ

−

−−

−

∑

(, )

()

iir

()

τσ

∑

(7.30)

This is more than simple notational gymnastics. The summations in the NS sector

are quite different than those for the R sector, because here we take

r =±±±

,,,…

(7.31)

CLOSED STRING BOUNDARY CONDITIONS

In the case of closed strings, we can apply periodic or antiperiodic boundary

conditions. These are given by

ψστ ψσπτ

±±

=+( , ) ( , ) (periodic boundary conditiion)

(antiperiodic bound

ψστ ψσπτ

±±

=− +(, ) ( ,) aary condition)

(7.32)

CLOSED STRING MODE EXPANSIONS

The boundary conditions in Eq. (7.32) can be applied separately to left and right

movers. The mode expansions are

ψστ

µµτσ

µµ

−+

−

∑

(, )

()



2 rr

()

τσ

−

∑

(7.33)

CHAPTER 7 RNS Superstrings

143

If we choose the R sector, then following the open string case

=±±012,, ,… (7.34)

On the other hand, if we choose the NS sector, then

r =± ±

,,$

(7.35)

We can choose either sector for left and right movers independently. If the

sectors match for left and right movers, we obtain space-time bosons. If the

sectors are different for left and right movers, then we obtain space-time fermions.

That is,

• Choosing the NS sector for left movers and the NS sector for right movers

gives space-time bosons.

• Choosing the R sector for left movers and the R sector for right movers

gives space-time bosons.

• Choosing the NS sector for left movers and the R sector for right movers

gives space-time fermions.

• Choosing the R sector for left movers and the NS sector for right movers

gives space-time fermions.

Super-Virasoro Generators

When we quantize the theory we will need super-Virasoro operators. These are

generalizations of what we have already worked out for bosonic string theory. We

extend the idea in this case to include a fermionic operator. That is,

LLL

→+

() ()

It can be shown that the following deﬁ nition will work:

Lrmbb

mmnn

rmr

=⋅+−

−

=−∞

∞

−+

∑∑

αα

()

(7.36)

144

String Theory Demystiﬁ ed

In addition, in superstring theory we have a second generator that arises from the

supercurrent

GdeJ b

mrm

==⋅

−

=−∞

∞

∫

∑

σα

(7.37)

for the NS sector, while we take

mnmn

=⋅

−+

∑

(7.38)

for the R sector. Here, m and n are integers while

r =± ±12 32/, /,…

Canonical Quantization

Now we are ready to quantize the theory, and canonical quantization is not so bad

because fermions are simple to deal with. The condition on the modes for the

bosonic string was the commutator:

αα δ η

µν µν

mn mn

⎡

⎣

⎤

⎦

+ 0

(7.39)

This relation is supplemented by a similar commutator for the

′

in the case of

closed strings. For the supersymmetric theory, we need to supplement Eq. (7.39)

with relations for the fermionic modes. You will recall from your studies of quantum

ﬁ eld theory that fermionic ﬁ elds satisfy anticommutation relations. In our case the

Majorana ﬁ elds will satisfy the equal time anticommutation relation:

{ (,), ( ,)} ( )

ψστψστ πηδδσσ

µν µν

AB AB

′

=−

′

(7.40)

In terms of the modes, we will have the following sets of anticommutation relations

depending on the sector used:

rs rs

mn mn

µν µν

ηδ

{}

(7.41)

The presence of the Minkowski metric in these equations mean that the theory will

still be plagued by negative norm states that we will have to remove.

CHAPTER 7 RNS Superstrings

145

The Virasoro operators generate what is known as a super-Virasoro algebra. There

are some differences for the R sector and the NS sector, so we consider each

independently.

NS SECTOR ALGEBRA

For the NS sector, the following relations are satisﬁ ed:

[, ]( ) ( )

LL n mL

n m nm nm

=− + −

(7.42)

[, ] ( )LG n rG

nr nr

=−

(7.43)

{,} ( )

GG L

r s rs rs

=+ −

(7.44)

The central charge is related to the space-time dimension by

cDD=+/2

. Let

be a physical state in the NS sector. The NS sector super-Virasoro constraints are

()La

NS0

0−=

(7.45)

=>00

(7.46)

=>00

(7.47)

Here, following the quantization of the bosonic string,

is a normal-ordering

constant. The open string mass formula is taken by setting

NS0

which gives

mNa

′

−

()

(7.48)

Where the number operator is

Nrbb

=⋅+ ⋅

−

∞

−

∞

∑∑

αα

112/

(7.49)

The Super-Virasoro Algebra