McMahon D. String Theory Demystified

Подождите немного. Документ загружается.

146

String Theory Demystiﬁ ed

R SECTOR ALGEBRA

In the R sector, the commutation and anticommutation relations are

[,]( )

LL mnL

m n mn mn

=− +

(7.50)

[,]LF

mn mn

=−

⎛

⎝

⎜

⎞

⎠

⎟

(7.51)

{,}

FF L

m n mn mn

(7.52)

The conditions on the physical states are

()La

0−=

(7.53)

=>00

(7.54)

=≥00

(7.55)

Here,

is the normal-ordering constant for the R sector.

EXAMPLE 7.6

Deduce that

SOLUTION

We start with the anticommutation relation satisﬁ ed by the

{,}

FF L

m n mn mn

Notice that if

mn==

we obtain

{,}F F FF FF F L

0 0 00 00 0

22=+==

⇒=

The

annihilate physical states

. Therefore,

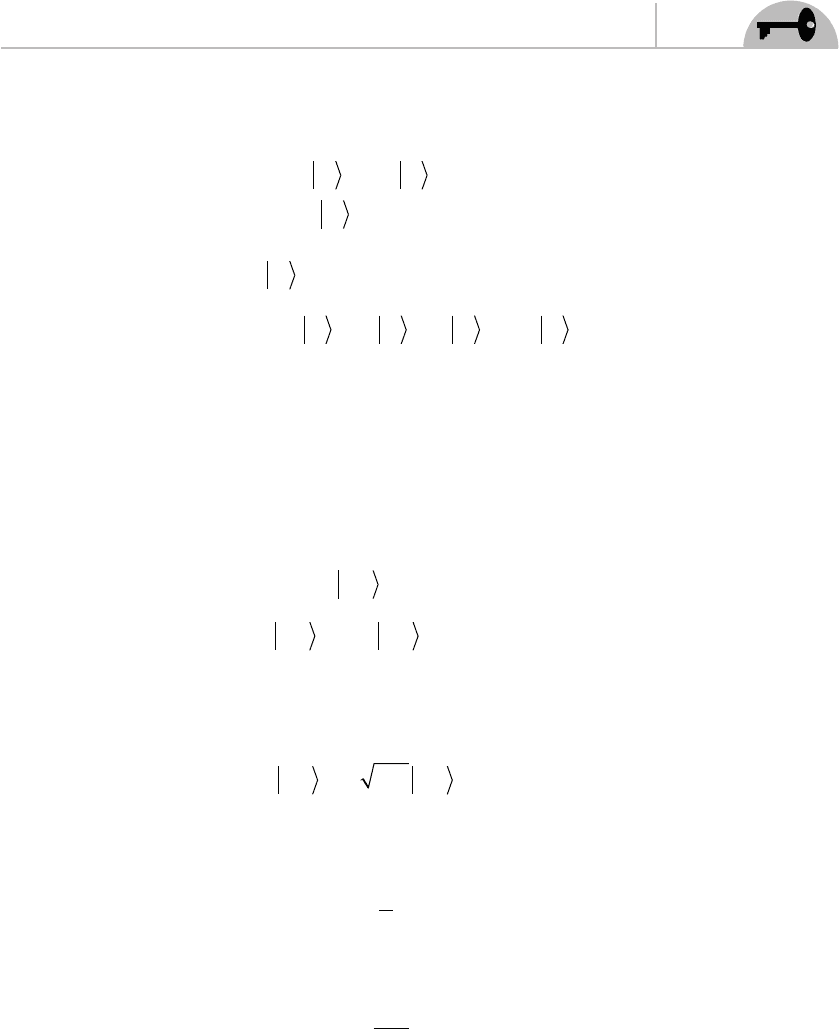
CHAPTER 7 RNS Superstrings

147

From this we obtain, by acting on the equation with

, the relation

FF F

00 0

ψψ

()

⇒=

But we know that

()La

0−=

. Hence,

=− = − =−

⇒=

()La L a a

RRR

ψψψ ψ

The Open String Spectrum

Now let’s examine the states of the string. We will look at states of the open string

in this chapter. We must consider the NS and R sectors independently. Working in

the NS sector ﬁ rst, the ground state is

0,k

and it is annihilated by the modes

kbk000,,==

(7.56)

where

nr, > 0

. The zero mode

as discussed in the bosonic string case is a

momentum operator:

αα

020,,kk

NS NS

′

(7.57)

It can be shown that the normal-ordering constant in the NS sector is

(7.58)

Using this we can ﬁ nd the mass of the ground state, which is

=−

′

(7.59)

Once again, we have a state with

so the theory still contains a tachyon state.

We will see later that we can get rid of the tachyon state in the superstring theory.

The ground state in the NS sector is a unique spin-0 state. To ﬁ nd massive states, we

progressively act on the state with negative mode oscillators.

148

String Theory Demystiﬁ ed

Next we consider the R sector, which describes space-time fermions in the open

string case. The ground state is annihilated by

µµ

kdk000,,==

(7.60)

for

m > 0

. The zero mode

is actually a Dirac operator. That is,

µµ

=Γ

(7.61)

We will see below that the critical space-time dimension is 10, so the states in the

R sector are 10-dimensional spinors. The ground state satisﬁ es the massless Dirac

wave equation. In our notation, this is written in the following way, recalling that

the momentum operator is

00⋅=dk

(7.62)

From Eq. (7.61), we deduce that the ground state in the R sector is a massless Dirac

spinor in 10 dimensions.

GSO Projection

In the previous section, we saw that the theory still has a major problem—it admits

an imaginary mass or tachyon state. This indicates that the vacuum is unstable. We

can rid the theory of the tachyon state, however, giving superstring theory a major

advantage over bosonic string theory (aside from bringing fermions into the picture).

This is done using GSO projection.

GSO projection reduces the number of states in the theory, and rids it of unwanted

problems like the tachyon state. In the NS sector, we keep states with an odd number

of fermion excitations and reject states with an even number of fermion excitations.

This is done by deﬁ ning a fermion number operator:

Fbb

=⋅

−

∑

(7.63)

Then we deﬁ ne a parity operator given by

=−−

11[()]

(7.64)

CHAPTER 7 RNS Superstrings

149

The parity operator determines the states that we can have in the theory.

Notice that if

=⇒ =00,

. Only half integer values of the number operator

Nrbbrbb

=⋅+ ⋅→ ⋅

−

∞

−

∞

−

∞

∑∑ ∑

αα

112 12//

are allowed, giving a mass spectrum for

the NS sector:

′′

,,,

αα

(7.65)

This means that the spin-0 ground state of the NS sector is now massless. The

tachyon state has been removed from the theory.

In the R sector, we deﬁ ne the Klein operator which is given by

()−=±1

11F

(7.66)

Here,

ΓΓΓΓ

11 0 1 9

= $

(7.67)

is a 10-dimensional chirality operator. It acts on spinors

according to

ψψ

=±

(7.68)

That is, states have positive or negative chirality. Weyl spinors are states with

deﬁ nite chirality, and states can be projected into spinors with opposite space-time

chirality using the operator

=±

()Γ

(7.69)

Critical Dimension

We will not pursue light-cone quantization in this chapter, but if that procedure is

used the number of space-time dimensions is easily extracted. One obtains a relation

for the Lorentz generators

[, ]

()

()(MM

−−

∞

=− − ∆ −

∑

αα αα

nn)

(7.70)

150

String Theory Demystiﬁ ed

where

∆=

−

⎛

⎝

⎜

⎞

⎠

⎟

+−

−

⎛

⎝

⎜

⎞

⎠

⎟

nNS

(7.71)

In order to maintain Lorentz invariance, we must have

[, ]MM

ij−−

= 0

. This can

only be true if the ﬁ rst term on the right-hand side of Eq. (7.71) is n and the second

term vanishes. This implies that

−

⇒=

(7.72)

So, we see that

• Lorentz invariance requires us to take the critical space-time dimension to

be 10 (9 space and 1 time dimension) in superstring theory.

Using Eq. (7.72), we can deduce the value of the normal-ordering constant:

010

NS NS

−

==⇒=

Summary

In this chapter, we made the ﬁ rst attempt to introduce fermions to string theory. This

was done by adding supersymmetry as a global symmetry on the worldsheet. The

conserved current and supercurrent was derived. Next, we wrote down the super-

Virasoro algebra and determined how physical states behave in the theory, and the

spectrum of the open string was described including the two sectors, the NS and R

sectors which give rise to bosonic and fermionic states, respectively. Using GSO

projection, one can remove unwanted states like the Tachyon from the theory. Finally,

we showed how Lorentz invariance forces us to take the critical dimension to be 10.

Quiz

1. Compute

to arrive at the equations of motion [Eq. (7.8)].

2. Using

STd XXi=− ∂ ∂ − ∂

∫

/( )2

σψρψ

µα

αµ

, consider an inﬁ nitesimal

Lorentz transformation

µν

→

. Find the conserved current associated

with the fermionic part of the lagrangian. (Hint: The Majorana spinors used

here transform as vectors under Lorentz transformations.)

CHAPTER 7 RNS Superstrings

151

3. A continuation of Prob. 2. What is the total conserved current? (Hint:

µν

antisymmetric.)

4. For the worldsheet supercurrent

µβ

βµ

ρρψ

=∂12/

, calculate

5. Using Eq. (7.14), ﬁ nd

given STd XXi=− ∂ ∂ − ∂

∫

/( )2

σψρψ

µα

αµ

6. Calculate

∂

+−−

7. Find

(),−

{}

8. Find

{, }ΓΓ

9. Calculate

()Γ

11 2

10. Characterize the states

µµ

−−11

NS R

dand

This page intentionally left blank

CHAPTER 8

Compactiﬁ cation

and T-Duality

In this chapter we introduce two important concepts. The ﬁ rst, compactiﬁ cation,

involves compactifying one of the extra spatial dimensions into a circle of radius R.

The second, T-duality, allows us to relate a theory with compactiﬁ ed extra dimension

of radius R to one with compactiﬁ ed extra dimension of radius

′

Compactiﬁ cation of the 25th Dimension

For simplicity, we consider the theory of the bosonic string which means we are

back to working with 26 space-time dimensions for the moment. The dimension

is timelike while

, ...,

are spatial dimensions. We imagine that one of those

spatial dimensions, typically chosen to be X

is curled up into a circle of radius R.

We wish to study how this compactiﬁ cation affects closed strings. As we will see,

it has some interesting consequences.

154

String Theory Demystiﬁ ed

Previously a closed string was constrained by the following periodic boundary

condition:

µµ

στ σ πτ

(,) ( ,)=+2

(8.1)

This boundary condition was stated with the implicit assumption where the string was

moving in a space-time with noncompact dimensions. Now let’s modify the situation.

As stated above, we are going to let the 25th dimension be a circle with radius R. This

changes the boundary condition in Eq. (8.1) as follows, but only for

XXnR

25 25

22(,)(,)

σπτ στ π

+= +

(8.2)

The interesting thing about Eq. (8.2) is that now the string will have winding states.

Simply put, the string can wind around the compactiﬁ ed dimension any number of

times. For all other dimensions

≠ 25

, Eq. (8.1) still holds.

The number n in Eq. (8.2) is called the winding number. Using the winding

number we can deﬁ ne the winding w as

′

(8.3)

We are going to see in a moment that the winding is actually a type of momentum. The

periodic boundary condition in Eq. (8.2) can be written in terms of the winding as

XXw

25 25

22(,)(,)

σπτ στ πα

+= +

′

(8.4)

Now let’s take a closer look at the boundary condition by seeing how it affects left-

moving and right-moving modes. This will demonstrate the fact that the winding is

a kind of momentum. First recall that

XXX

µµµ

στ στ στ

(,) (,) (,

)

. The left- and

right-moving modes can be written as follows:

Xxpi

LLL

25 25 25

22 2

(,) ( )

στ

τσ

′

≠

−

∑

iin()

τσ

(8.5)

Xxpi

RRR

25 25 25

22 2

(,) ( )

στ

τσ

′

−+

′

≠

−

∑

iin()

τσ

−

(8.6)

where we have made the identiﬁ cations

αα αα

25 1 2 25

22=

′

(/) (/)

and

Adding Eqs. (8.5) and (8.6) together (while ignoring the oscillator contributions) we get

X x pp pp

LR LR

25 25 25 25 25 25

(,)

στ

′

(

)

′

−

(

)

σσ

+ modes

(8.7)

CHAPTER 8 Compactiﬁ cation and T-Duality

155

The total center of mass momentum of the string is

ppp

25 25 25

(8.8)

Along the compactiﬁ ed dimension, the string acts like a particle moving on a circle.

The momentum is quantized according to

(8.9)

where K is an integer called the Kaluza-Klein excitation number. This is an important

result—without the compactiﬁ ed dimension, the center of mass momentum of the

string is continuous. Compactifying a dimension quantizes the center of mass

momentum along that dimension.

Looking at Eq. (8.7) then, the ﬁ rst term involving the momenta is the total center

of mass momentum of the string. We call this the momentum mode. The second

term, however, also involves momentum. In fact this term is the winding mode of

the string, which satisﬁ es

′

−

()

25 25

pp nR

(8.10)

Looking at Eq. (8.3), we see that the winding w can be deﬁ ned in terms of the

momentum of the left- and right-moving modes as

′

−

()

αα

25 25

(8.11)

Modiﬁ ed Mass Spectrum

Compactifying a dimension will lead to a modiﬁ ed mass spectrum. To obtain the

mass spectrum for the state with a compactiﬁ ed dimension, let us begin with the

Virasoro operators. Recall that

Lpp

RR n n

′

+⋅

−

∞

∑

αα

(8.12)

Note the repeated index which is an upper and lower index on the ﬁ rst term in

the right—so we have an implied sum. Here the index

ranges over the entire