Luo A.C.J. (Ed.) Dynamical Systems: Discontinuity, Stochasticity and Time-Delay

Подождите немного. Документ загружается.

194 A.C.J. Luo and Y. Wang

In addition, (16.25) gives the following mapping relations

kC1

D P

) P

W x

! x

kC1

D P

kC1

) P

W x

kC1

! x

kC1

kC2

D P

kC1

) P

W x

kC1

! x

kC2

kC1

D P

kC2

) P

W x

kC2

! x

kC2

kCn

D P

kCn1

) P

W x

kCn1

! x

kCn

nC1

kCn

D P

nC1

kCn

) P

nC1

W x

kCn

! x

nC1

kCn

;

: (16.25)

Mapping relations in (16.20) yields a set of algebraic equations as



kC1

; x

;˛



D 0; g



kC1

; x

kC1



D 0;



kCn

; x

kCn1

;˛

kCn



D 0; g

nC1



kCn

; x

nC1

kCn



D 0:

;

(16.26)

If there is a periodic motion, the periodicity for t

kCn

D T C t

nC1

kCn

D x

for l

nC1

D l

or x

nC1

1;kCn

D x

1;k

;:::;x

nC1

m;kCn

DPx

m;k

; (16.27)

where T is time period. The resultant periodic solution of the switching system is

for i D 1; 2; ;n

.t/ D

.t/

t 2 Œt

kCnsCi 1

kCnsCi

 for s D 0; 1; 2; : : :



;

kCnsCi

D x

mod .i;n/C1

kCnsCi

for s D 0; 1; 2; : : :

)

(16.28)

From (16.23)and(16.24), the corresponding switching points for the periodic

motion can be determined. From the time difference parameter, the time interval

parameter is deﬁned as

kCm

and

mD1

kCm

D 1: (16.29)

If a set of the time interval parameters for switching subsystems during the next

period is the same as during the current period, the periodic ﬂow is called the

equi-time-interval periodic ﬂow. The pattern of the resultant ﬂow for the switching

system during the next period will repeat the pattern of the ﬂow during the current

period. If a set of the time interval parameters for the second period is different

from the ﬁrst period, the periodic motion is called the non-equi-time-interval peri-

odic motion. For this ﬂow, the switching pattern during the next period is different

16 On Periodic Flows of a 3-D Switching System with Many Subsystems 195

from the current one. For a general case, during two periods, only one pattern to

make (16.28) can be satisﬁed. Hence, this switching pattern can be treated as a pe-

riodic ﬂow with two periods.

To determine the stability of such a periodic motion, the Jacobian matrix can be

computed, i.e.,

DP D DP







; (16.30)

where for j D 1;2;:::;n,

kCs

kCs1

mm



1.kCs/

2.kCs/

;:::;x

m.kCs/





1.kCs1/

2.kCs1/

;:::;x

m.kCs1/



mm

D

kCs

1

mm

kCs1

mm

(16.31)

because from (16.15) one obtains

kCs1

mm

kCs1

mm

kCs

kCs1

mm

D 0: (16.32)

Similarly, from the transport law, one obtains

j C1

kCs

mm

j C1

kCs

mm

j C1

kCs

mm

D 0; (16.33)

j C1

kCs

mm



j C1

1.kCs/

j C1

2.kCs/

;:::;x

j C1

m.kCs/





1.kCs/

2.kCs/

;:::;x

m.kCs/



mm

D

j C1

kCs

1

mm

j C1

kCs

mm

(16.34)

If the magnitudes of two eigenvalues of the total Jacobian matrix DP are less than

1(i.e.,



<1, ˛ D 1;2;:::;m:/, the periodic motion is stable. If the magnitude

of one of two eigenvalues is greater than 1 (



>1,˛ 2f1;2;:::;mg/, the peri-

odic motion is unstable. If one of eigenvalues is positive one (C1)andtherestof

eigenvalues are in the unit cycle, the periodic ﬂow experiences a saddle-node bifur-

cation. If one of eigenvalues is negative one (1) and the rest of eigenvalues are in

the unit cycle, the periodic ﬂow possesses a period-doubling bifurcation. If a pair of

complex eigenvalues is on the unit cycle and the rest of eigenvalues are in the unit

cycle, a Neimark bifurcation of the periodic ﬂow occurs.

196 A.C.J. Luo and Y. Wang

16.3 Analytical Predictions

For switching system, periodic motions can be predicted by constructing certain

mapping structure and the corresponding stability can be determined by evaluat-

ing eigenvalues of Jacobian matrix. To illustrate the stability of the 3-D switching

system, consider a linear switching system as an example that includes two 3-D

subsystems with two matrices for j D 0; 1; 2; : : :

.1/

for t 2 Œt

kC2j

kC2j C1

;

.2/

for t 2 Œt

kC2j C1

kC2j C2

; (16.35)

.i/



.i/

tt

.i/

 .t  t

/; A

.i/



for i D 1; 2:

The linear switching system is expressed by

.i/

D A

.i/

C Q

.i/

(16.36)

The two subsystems are continuously connected at the switching points. Without

losing generality, the parameters for a dynamical system are ﬁxed and the parame-

ters for another subsystem are varied. For instance, select the parameters as

D a

D b

D1I

D a

D 1I a

D a

D2I a

D 3Ia

D3I b

D1:5: (16.37)

To understand the time intervals of the two subsystems for periodic motion,

introduce a new parameter as

.i/

2kCi 1

2kCi

 t

2kCi 1

for i D 1; 2 and

1 D

iD1

kD1

.i/

2kCi 1

for k D 1;2;:::;n: (16.38)

If q

.i/

2kCi 1

D q

.i/

,theith subsystem possesses the equi-time interval. Otherwise,

the i th subsystem possesses the non-equi-time interval. Consider a simple periodic

motion with a mapping structure as P D P

 P

D P

, and the corresponding

time interval parameter can be set as q

.1/

and q

.2/

.Forq

.1/

D 0 (q

.2/

D 1/,the

switching system is formed by the second subsystem only. For q

.1/

D 1 (q

.2/

D 0/,

the switching system is formed by the ﬁrst subsystem only. Switching points are

recorded in Fig. 16.3 for a periodic ﬂow with P D P

,whereP

is given as a

mapping from an initial state x

.1/

to the ﬁnal state x

.1/

kC1

in the ﬁrst subsystem of

16 On Periodic Flows of a 3-D Switching System with Many Subsystems 197

Parameter, b

1000

Switching, x

1(k)

Switching, x

2(k)

Switching, x

3(k)

−100

−50

100

1(k+1)

2(k+1)

3(k+1)

3(k)

1(k)

2(k)

−100

−50

100

200 400 600 800

Parameter, b

1000

200 400 600 800

Parameter, b

1000

200 400 600 800

−400

−200

200

400

Fig. 16.3 Periodic motion scenario with P

for switching 3-D systems (a) switching x

1.k/

(b) switching x

2.k/

and (c) switching x

3.k/

. q

.1/

D 0:25I a

D a

D b

D1I a

D a

D b

D 1Ia

D a

D2I a

D 3I a

D3I b

D1:5I b

0:5I b

D 2I b

D2:0I A

.1/

D A

.1/

D A

.1/

D A

.2/

D A

.2/

D 1I A

.2/

D1I T D 4

the switching system, and P

represents the mapping between an initial state x

.2/

kC1

and the ﬁnal state x

.2/

kC2

for the second subsystem. The solid line stands for the

stable periodic response in shaded area and the dashed line represents the unsta-

ble response in white area with the parameter (b

D2:0; q

.1/

D 0:25), where

.1/

is the time interval of ﬁrst subsystem as deﬁned in (16.38). The similarity of

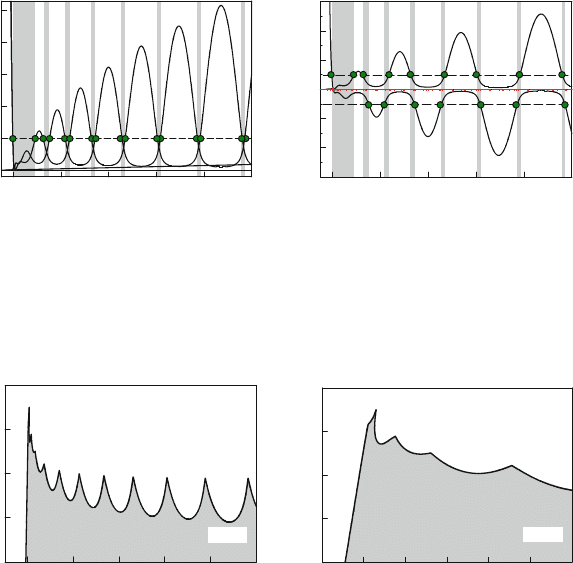
198 A.C.J. Luo and Y. Wang

the switching points and periodic motion can be observed as parameter b

vary-

ing from 0 to 1,000. The corresponding eigenvalues are given in Fig. 16.4.Once

there is amplitude of eigenvalues of Jocabian matrix greater than one, the switching

system is unstable. Only if all amplitudes of eigenvalues are less than one, switching

system is stable. It is consistent with the results of switching points and eigenval-

ues. When one real eigenvalue approaches to positive one (C1), switching points of

periodic ﬂow goes to inﬁnite and turns to unstable inﬁnite. Meanwhile, if one real

eigenvalue is negative one (1), switching points are ﬁnite numbers shown as solid

points. As long as parameter b

is increasing, the stable region vanished to zero and

values of switching points are expanding. Parameter map of stability region is given

in Fig. 16.5 with respect to b

and b

. For the boundary of stable and unstable

region, b

and b

goes to negative inﬁnity in the left-hand plane, and cusps can be

found along the boundary in right-hand plane.

Parameter, b

Magnitude of Eigenvalues

0.0

1.0

2.0

3.0

4.0

5.0

Parameter, b

200 400 600 800

1000

200 400 600 800 1000

Eigenvalues

−6.0

−4.0

−2.0

0.0

2.0

4.0

6.0

Fig. 16.4 Eigenvalue analysis for periodic motions with P

for switching 3-D systems (a)mag-

nitudes and (b) real and imaginary parts of eigenvalues. q

.1/

D 0:25I a

D a

D2I a

D 3I a

D3I a

D a

D b

D1I a

D a

D b

D 1Ib

1:5I b

D 0:5I b

D 2Ib

D2:0I A

.1/

D A

.1/

D A

.1/

D A

.2/

D A

.2/

D 1I A

.2/

1I T D 4

Parameter, b

Unstable

Stable

Parameter, b

0 200 400 600 800 1000

−20 0 20 40 60 80 100

Parameter, b

−8.0

−4.0

0.0

4.0

8.0

Unstable

Stable

−8.0

−4.0

0.0

4.0

8.0

Fig. 16.5 Parameter map for switching 3-D systems, (a) stable and unstable motion regions

(b) zoomed view. q

.1/

D 0:25; a

D a

D b

D1; a

D a

D 1; a

D 3; T D 4; a

D a

D2; a

D3; b

D1:5; b

D 0:5; b

D 2;

.1/

D A

.1/

D A

.1/

D A

.2/

D A

.2/

D 1; A

.2/

D1; b

D 0:5; b

D 2; b

D1

16 On Periodic Flows of a 3-D Switching System with Many Subsystems 199

time, t

State, x

0.0 2.5 5.0 7.5 10.0

−10.0

−5.0

0.0

5.0

10.0

time, t

State, x

−12.0

−8.5

−5.0

−1.5

2.0

0.0

5.0 7.5 10.0

State, x

time, t

0.0 2.5 5.0 7.5 10.0

−10.0

−6.5

−3.0

5.0

4.0

−10.0

−6.0

−2.0

2.0

−5.0

0.0

5.0

10.0

−10.0

−2.0

State, x

time, t

0.0 2.5 5.0 7.5 10.0

−

100.0

−

50.0

0.0

50.0

State, x

time, t

0.0 2.5 5.0 7.5 10.0

−

50.0

−

25.0

0.0

25.0

50.0

State, x

time, t

0.0 2.5 5.0 7.5 10.0

−

400.0

−

200.0

0.0

200.0

400.0

−3.0

1.0

5.0

9.0

−4.0

−2.0

0.0

2.0

4.0

−38.0

−23.0

−8.0

7.0

22.0

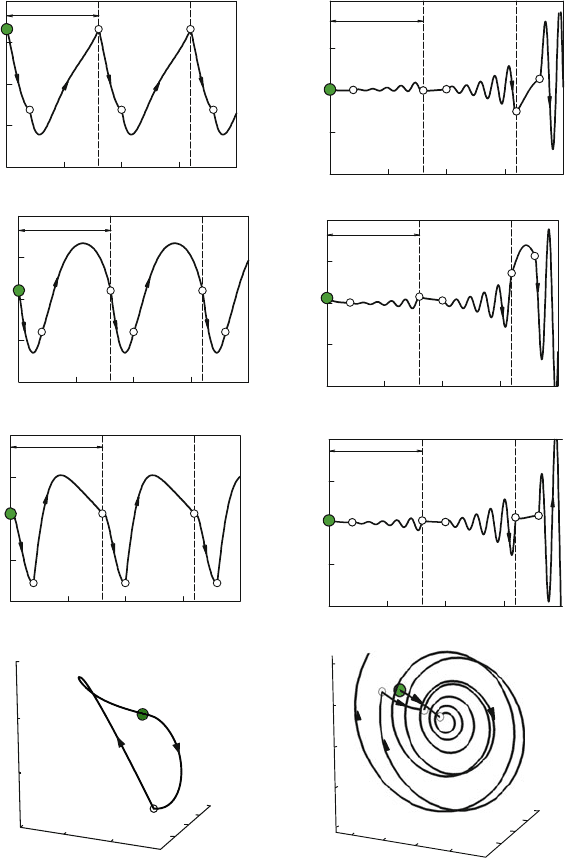
Fig. 16.6 Periodic ﬂows of P

for switching 3-D systems. q

.1/

D 0:25; a

D a

2; b

D 2; b

D 0:5; a

D a

D b

D1; a

D b

D 1; a

D 3; a

D3; b

D2:0; b

D1:5; A

.2/

D1; T D

4; A

.1/

D A

.1/

D A

.1/

D A

.2/

D A

.2/

D 1: t

D 0; (a–d) stable periodic ﬂow

/  6:6304; x

/ 4:3082; x

/  2:6031; b

D 0; (e–h) unstable ﬂow x

/ 

0:7232; x

/  3:0431; x

/  10:1835; b

D 100

200 A.C.J. Luo and Y. Wang

16.4 Numerical Illustrations

From the analytical prediction, numerical illustrations of periodic ﬂows can provide

a comprehensive understanding of the switching systems. Consider the switching

system as deﬁned in (16.37) and parameters in (16.36), a stable periodic ﬂow is

given in Fig. 16.6(a)–(d) with b

D 0; q

.1/

D 0:25; A

.2/

D1; T D 4; and

.1/

.2/

D1. From analytical prediction, the initial condition

for this periodic ﬂow is x

.0/ D 6:6304, x

.0/ D4:3082, x

.0/ D 2:6031.

The time histories for three state variables (x

, i D 1; 2; 3/ in the periodic ﬂow of

the 3-D linear switching system are presented in Fig. 16.6(a)–(c). The solid point

represents the initial points and hollow points are switching points when subsystem

is switching from one to another. It is observed that all the switching points are

continuous but nonsmooth. An unstable ﬂow of P

is given in Fig. 16.6(e)–(h) with

D 100. Even the initial condition is chosen as x

.0/ D 0:7232, x

.0/ D 3:0431,

.0/ D 10:1835 from analytical prediction, the periodic ﬂow is easily destroyed

by a disturbance and the values of each state (x

,i D 1; 2; 3) will goes to inﬁnity as

showninFig.16.6(e)–(g) in time history. The numerical simulations are consistent

with analytical predictions.

16.5 Conclusions

In this chapter, a switching system of multiple subsystems with transport laws at

switching points is discussed. A frame work for periodic ﬂows of such a switch-

ing system is presented. To show applications, periodic ﬂows and stability for linear

switching systems are discussed as an example. Analytical prediction of periodic

ﬂows in such linear switching systems is carried out, and parameter maps for pe-

riodic motion stability are developed. Numerical simulations are demonstrated for

illustration of stable and unstable motions. For linear switching systems, bifurca-

tions of the periodic ﬂows cannot be observed. This framework can be applied to

the nonlinear switching systems. The further results on stability and bifurcation of

periodic ﬂows in nonlinear switching systems will be presented in sequel.

References

1. Morse AS (1997) Control using logic-based switching. Lecture notes in control and information

sciences, vol 222. Springer, London

2. Sachdev MS, Hakal PD, Sidhu TS (1997) Automated design of substation switching systems.

Developments in power system protection, 6th international conference, pp 369–372

3. Liberzon D, Morse AS (1999) Basic problems in stability and design of switched systems. IEEE

Control Syst 19(5):59–70

4. Danca MF (2008) Numerical approximations of a class of switch dynamical systems. Chaos

Solitons Fractals 38:184–191

16 On Periodic Flows of a 3-D Switching System with Many Subsystems 201

5. Grune L, Kloeden PE (2006) High order numerical approximation of switching system. Syst

Control Lett 55:746–754

6. Gokcek C (2004) Stability analysis of periodically switched linear system using Floquet theory.

Math Probl Eng 2004(1):1–10

7. Luo ACJ (2005) A theory for non-smooth dynamic systems on the connectable domains. Com-

mun Nonlinear Sci Numer Simul 10:1–55

8. Luo ACJ (2006) Singularity and dynamics on discontinuous vector ﬁelds. Elsevier, Amsterdam

9. Luo ACJ, Wang Y (2009) Switching dynamics of multiple linear oscillators. Commun Nonlinear

Sci Numer Simul 14:3472–3485

Chapter 17

Impulsive Control Induced Effects on Dynamics

of Complex Networks

Xiuping Han and Xilin Fu

Abstract Control and synchronization of complex networks have been extensively

investigated in many research and application ﬁelds. Previous works focused upon

realizing synchronization by varied methods. There has been little research on the

dynamics of synchronization manifold of complex networks by now. It was known

that dynamics of the single system can be changed very obviously after inputting

particular impulse signals. For the ﬁrst time, above impulsive control of complex

networks is considered in this chapter. Complex networks can realize to synchronize

with such impulsive control. And dynamics of the synchronous state of complex

networks can be induced to different orbit. The orbit may be an equilibrium point,

a periodic orbit,or a chaotic orbit, which is determined by a parameter in the outer

impulse signal. Strict theories are given.

17.1 Introduction

Recently, complex networks have received rapidly increasing attentions from

different ﬁelds. Such as from internet to world wide web, from communication

networks to social organizations, from food webs to ecological communities, etc.

They widely exist in our life and are presently prominent candidates to describe the

sophisticated collaborative dynamics in many sciences [1,3, 5,8,19,21]. So far, the

dynamics of complex networks has been extensively investigated. Control and syn-

chronization are typical topics that have attracted lots of interests [11,13,17, 19–21].

Synchronization is a fundamental phenomenon that enables coherent behavior in

networks as a result of interactions. And several different approaches including

adaptive synchronization [26], robust synchronization [16], and impulsive control

[9] have been introduced to solve the above problem. Among these approaches,

X. Fu (



)

School of Mathematical Sciences, Shandong Normal University, Jinan 250014,

People’s Republic of China

e-mail: xilinfu@gmail.com

A.C.J. Luo (ed.), Dynamical Systems: Discontinuity, Stochasticity and Time-Delay,

DOI 10.1007/978-1-4419-5754-2

17,

 Springer Science+Business Media, LLC 2010

203

204 X. Han and X. Fu

studies show that impulsive control strategy [2, 10,15, 22–24] is very effective and

robust while with low cost. In past decades, it has been widely applied in many

ﬁelds, such as space techniques, information science, control system, dynamical

nerve cell networks, and communication security etc. It allows stability of a com-

plex network only by small impulses being sent to the receiving systems at the

discrete impulsive instances, which can reduce the information redundancy in the

transmitted signal and increase robustness against the disturbances. In this sense,

impulsive control schemes have been applied to numerous chaos-based communi-

cation systems for cryptographically secure purposes and detailed experiments have

been carried out [6,7, 12].

Previous work on impulsive control and synchronization of complex networks

are focused on normal impulsive effects. The impulsive input only contains state

variable. Little work has been done for the synchronization of networks with special

impulsive control, which contains outer signals. Control of chaotic systems to peri-

odic motions has been discussed using proper impulsive input [25]. It is presented

in [18] that chaos exists in a class of impulsive differential equation. Two chaotic

models for single impulsive differential systems have been discussed in [14]. Impul-

sive control induced effects on single and coupled systems with two systems have

been discussed in [4].

In this chapter, impulsive control induced effects on dynamics of complex

networks and the stability of complex networks with different dynamical nodes

by such impulsive control is discussed, where the impulse effects have outer in-

put signals. It can be seen that the complex networks realize synchronization with

such special impulsive control. The dynamics of complex networks are affected by

changes of outer input signals. Complex networks can realize synchronization and

its synchronization manifold can be changed with such impulsive control signals.

17.2 Main Results

Consider a network consisting of N nodes, in which each node is an n-dimensional

dynamical system. The state equations are

D f

/ C

j D1;:::;N

x

;iD 1;2;:::;N; (17.1)

where x

2 R

, B D .b

N N

denote the coupling conﬁguration matrix. Then

D b

D 1 if there is a connection between node i and j (i ¤ j ); otherwise,

D b

D 0. In this model, it is required that the coupling coefﬁcients satisfy

D

j D1;j ¤i

.And 2 R

nn

is the inner connecting matrix in each node. It

is called complex networks with identical dynamical nodes if f

D f

DDf

otherwise it is with different dynamical nodes.The impulsive control with special