Luo A.C.J. (Ed.) Dynamical Systems: Discontinuity, Stochasticity and Time-Delay

Подождите немного. Документ загружается.

128 Z. Shang et al.

11.4.1 Stability Domains in the Plane of ˝

In this case, the mass ratio and the stiffness ratio are ﬁxed to M

D 0:2; ˇ

D 2:0.

The stability domains of the synchronous full annular rub solutions in the parameter

plane of ˝   at different cross-coupling coefﬁcients are studied.

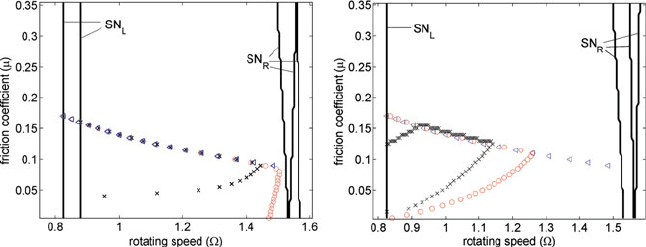
Figure 11.2 demonstrates the inﬂuence of the cross-coupling stiffness on the

stability domains. In Fig. 11.2 (left), it is found that with the increase of the cross-

coupling stiffness of the rotor, 

, from 0.0 to 0.4, both the lower and the upper

existence boundaries of the solution move rightward. So the existence region of

the solution has almost no change with the increase of 

while the stable domain

shrinks. In fact, the increase of the stable domain may be observed when 

is tuned

between 0.0 and 0.2. As pointed out in [3], the dry friction and the cross-coupling

stiffness of the rotor play contrary roles in stabilizing the synchronous full annular

rub solution and both can destabilize the solution. So when the two parameters are

well balanced for the given system parameters, the synchronous full annular solu-

tion is stable. As either of the two parameters is too large to be compensated by the

other one, the solution will become unstable. In Fig. 11.2 (left), the lower branches

of the stability boundary at 

D 0:2 and 

D 0:4 are due to the too large cross-

coupling stiffness of the rotor. In Fig. 11.2 (right), it is easily seen that the increase

of the cross-coupling stiffness of the stator has no inﬂuence on the lower boundary

of the existence region of the synchronous full annular solution, but will shift the

upper boundary to the right. So the increase of 

will enlarge the existence region

of the solution. Meanwhile it will also monotonically reduce the stability domain

of the solution, indicating the disadvantage for the appearance of the cross-coupling

stiffness of the stator in the rotor-to-stator contact systems.

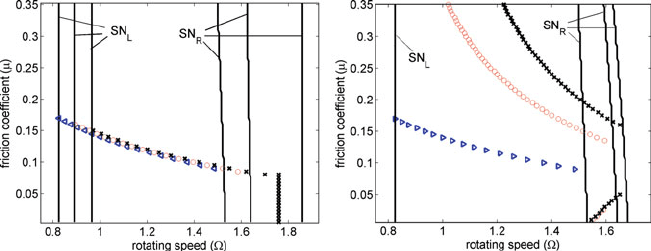
The inﬂuence of the cross-coupling damping on the stability of the synchronous

full annular rub solution is demonstrated in Fig. 11.3. It is found from Fig. 11.3 (left)

Fig. 11.2 Stability chart of the synchronous full annular solution in the parameter plane of ˝ 

at different cross-coupling stiffness coefﬁcients. Left: in the case that 

D 

D 0,where

(inverted open triangle) stands for the stability boundary at 

D 0,(open circle)at

D 0:2,

(cross)at

D 0:4. Right: in the case that 

D 

D 0,where(inverted open triangle)

stands for the stability boundary at 

D 0,(open circle)at

D 0:2,(cross)at

D 0:4

11 Cross-Coupling Effects on the Dynamics of Rotor/Stator Rubbing 129

Fig. 11.3 Stability chart of the synchronous full annular solution in the parameter plane of ˝ 

at different cross-coupling damping coefﬁcients. Left: in the case that 

D 

D 

D 0,where

(inverted open triangle) stands for the stability boundary at 

D 0,(open circle)at

D 0:2,

(cross)at

D 0:4. Right: in the case that 

D 

D 

D 0,where(inverted open triangle)

stands for the stability boundary at

D 0,Oat

D 0:2,(cross)at

D 0:4

that the cross-coupling damping of the rotor, 

, has a little inﬂuence on the size of

the existence region of the synchronous full annular rub solution when 

changes

from 0.0 to 0.2 then to 0.4. But it has almost no effect on the stability domain of

the solution. The effect of the cross-coupling damping of the stator is signiﬁcant in

comparison. First, it enlarges the existence region of the full annular rub solution

by keeping the lower boundary unchanged and moving the upper boundary right-

ward. Secondly, it increases the stability domain of the solution remarkably. So the

cross-coupling damping of the stator will beneﬁt the synchronous full annular rub

response by avoiding the contact severity between the rotor and the stator through a

synchronous full annular rub.

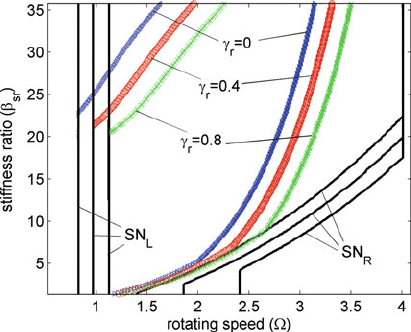
11.4.2 Stability Domains in the Plane of ˝ˇ

Below the mass ratio and the friction coefﬁcient are ﬁxed to M

D 0:2;  D

0:10. The cross-coupling damping of the rotor, 

, on the stability domains of the

synchronous full annular rub solution in the parameter plane of ˝ ˇ

are explored.

It is noticed from Fig. 11.4 that the speed range by which the synchronous full

annular rub solution exists increases signiﬁcantly with the increase of the stiffness

ratio between the stator and the rotor. Meanwhile, the speed range of the stable

synchronous full annular rub solution becomes large when the stiffness ratio ˇ

reduced, indicating that small stiffness ratio between the stator and the rotor may

beneﬁt the system to have a relatively large range of mild rubbing in the case that

rubbing between the rotor and the stator is unavoidable.

The inﬂuence of the cross-coupling damping of the rotor on both the existence

region and the stable domain of the full annular rub solution is seen to be very little.

130 Z. Shang et al.

Fig. 11.4 Stability chart of the synchronous full annular solution in the parameter plane of ˝ ˇ

at different cross-coupling damping of the rotor in the case that 

D 

D 

D 0,where(inverted

open triangle) stands for the stability boundary at 

D 0:0,(open circle)at

D 0:4,(cross)at



D 0:8

With the increase of 

, the lower and the upper boundaries of the solution move

simultaneously to the right, while the stability boundaries of the solution shift in the

similar manner to produce seemingly unaltered stable domains.

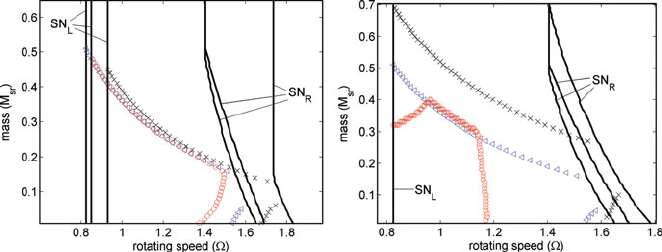
11.4.3 Stability Domains in the Plane of ˝M

To study the stability domains of the synchronous full annular rub solution in the

parameter plane of ˝M

, the stiffness ratio and the friction coefﬁcient are ﬁxed

to ˇ

D 2:0;  D 0:10.

From Fig. 11.5 it is seen that the inﬂuence of the mass ratio on the existence re-

gion of the synchronous full annular rub solution is small. It is, however, observable

that the speed range of the stable synchronous full annular rub solution will take its

maximal value around M

D 0:2. In order to get an overall view on the inﬂuence of

the cross-coupling effects on the stability of the synchronous full annular rub solu-

tion, the stability domains of the solution under the inﬂuence of the cross-coupling

effects of the rotor and of the stator are drawn in Fig. 11.5 (left) and (right), respec-

tively. It is found that the cross-coupling stiffness of the rotor slightly enlarges the

stability domain in this case and the cross-coupling damping of the rotor reduces

it as mentioned above (see Fig. 11.5 (left)). On the other hand, the increase of the

cross-coupling damping of the stator signiﬁcantly enlarges the stable domain of the

synchronous full annular rub solution, while the increase of the cross-coupling stiff-

ness of the stator may largely reduce the stable domain of the solution, as shown in

Fig. 11.5 (right).

11 Cross-Coupling Effects on the Dynamics of Rotor/Stator Rubbing 131

Fig. 11.5 Stability chart of the synchronous full annular solution in the parameter plane of

˝M

. Left: under the cross-coupling effects of the rotor with 

D 

D 0,where(inverted open

triangle) stands for the stability boundary at 

D 

D 0:0;(open circle)at

D 0:0; 

D 0:3;

(cross)at

D 0:3; 

D 0:0. Right: under the cross-coupling effects of the stator with



D 

D 0,where(inverted open triangle) stands for the stability boundary at 

D 

D 0:0;

(open circle)at

D 0:3 

D 0:0;(cross)at

D 0:0; 

D 0:3

11.5 Conclusions

With the goal to understand the inﬂuence of cross-coupling effects on the rubbing-

related dynamics of rotor/stator systems, a model for a rotor/stator system including

both the dynamics of the stator and the deformation on the contact surface as well as

the cross-coupling damping and stiffness is set up in this chapter. After solving the

synchronous full annular rub solution of the model, the stability analysis of the so-

lution is then carried out. Our results show that except the cross-coupling damping

of the rotor, the other three cross-coupling terms all have inﬂuence on the stability

domains of the synchronous full annular rub solution. While the cross-coupling stiff-

ness of the stator can monotonically reduce the stability domain, the cross-coupling

stiffness of the rotor has some optimal value that may achieve a maximal stability

domain for the given system parameters. The cross-coupling damping of the stator

will beneﬁt the rotor-to-stator contact system through signiﬁcantly enlarging the sta-

ble domain of the synchronous full annular rub solution. Since the synchronous full

annular rub is a mild rub in comparing with the heavy rub, such as the quasiperiodic

partial rubs and the destructive self-excited dry friction backward whirls. So an ap-

propriate adaptation of the cross-coupling effects that can stabilize the synchronous

full annular rubs in the rotor/stator system may prevent system from quick damages

under rubbing.

Acknowledgments The authors are grateful for the ﬁnancial support by the National Natural

Science Foundation of China (NSFC) under the grant No. 10872155, 10472086 and 10772140.

132 Z. Shang et al.

References

1. Muszynska A (1989) Rotor-to-stationary element rub-related vibration phenomena in rotating

machinery – literature survey. Sound Vib Dig 21:3–11

2. Black HF (1968) Interaction of a whirling rotor with a vibrating stator across a clearance an-

nulus. Int J Mech Eng Sci 10:1–12

3. Jiang J, Ulbrich H (2001) Stability analysis of sliding whirl in a nonlinear jeffcott rotor with

cross-coupling stiffness coefﬁcients. Nonlinear Dyn 24:269–283

4. Yu JJ, Goldman P, Bently DE, Muszynska A (2002) Rotor/seal experimental and analytical

study on full annular rub. ASME J Gas Turbine Power, 124:340–350

5. Childs DW (1979) Rub-induced parametric excitation in rotors. J Mech Design 101:640–644

6. Ehrich FF (1988) High order sub-harmonic response of high speed rotors in bearing clearance.

ASME J Vib Acoust Stress Reliab 110:9–16

7. Day WB (1987) Asymptotic expansions in nonlinear rotor-dynamics. Quart Appl Math

44:779–792

8. YB Kim, Noah ST (1996) Quasi-periodic response and stability analysis for a nonlinear jeffcott

rotor. J Sound Vib 190:239–253

9. Choi SK, Noah ST (1994) Mode-locking and chaos in a Jeffcott rotor with bearing clearances.

ASME J Appl Mech 61:131–138

10. Abdul Azeez MF, Vakakis AF (1999) Numerical and experimental analysis of a continuous

overhung rotor undergoing vibro-impacts. Int J Nonlinear Mech 34:415–435

11. Choi YS (2002) Investigation on the whirling motion of full annular rotor rub. J Sound Vib

258:191–198

12. Jiang J, Ulbrich H (2005) The physical reason and the analytical condition for the onset of dry

whip in rotor-to-stator contact systems. ASME J Acoust Vib 127:594–603

13. Sun L, Krodkiewski JM (1998) Self-tuning adaptive control of forced vibration in rotor systems

using an active journal bearing. J Sound Vib 213:1–14

14. Knospe C, Hope R, Tamer S, Fedigan S (1996) Robustness of adaptive unba-lance control of

rotors with magnetic bearings. J Vib Control 2:33–52

15. Qiu JH, Tani J, Kwon T (2003) Control of self-excited vibration of a rotor system with active

gas bearings. ASME J Vib Acoust 125:328–334

16. Jiang J, Ulbrich H, Chavz A (2006) Improvement of rotor performance under rubbing condi-

tions through active auxiliary bearings. Int J Non-Linear Mech 41/8:949–957

Part II

Time-delay Systems

Chapter 12

Some Control Studies of Dynamical Systems

with Time Delay

Bo Song and Jian-Qiao Sun

Abstract This chapter presents a summary of recent studies of controlling

dynamical systems with time delay. The time delay can be uncertain and time-

varying with known lower and upper bounds. Two methods for approximate

solutions of the system with time delay are discussed, namely the method of

semi discretization and the method of continuous time approximation. The spec-

tral properties of mapping based methods are discussed. We also demonstrate the

supervisory control to handle uncertainties in time delay. Several control examples

are presented in the chapter.

12.1 Introduction

Time-delayed systems have been studied using discretization techniques with an

extended state vector. Pinto and Goncalves [1] fully discretized a nonlinear SDOF

system to study control problems with time delay. Klein and Ramirez [2] studied

MDOF delayed optimal regulator controllers with a hybrid discretization technique,

where the state equation was partitioned into discrete and continuous portions. Yang

andWu[3] and Stepan [4] have studied structural systems with time delay. A study

on stability and performance of feedback controls with multiple time delays is re-

portedin[5] by considering the roots of the closed-loop characteristic equation.

A method using Chebyshev polynomials to approximate general nonlinear functions

of time has been developed to handle linear and nonlinear time-delayed dynamical

systems with periodic coefﬁcients [6–9]. The method has also been applied to study

optimal control problems. A temporal ﬁnite element method has been proposed in

[10] to study the stability of time-delayed systems with parametric excitations. The

work reported in [11] makes use of the piece-wise exact solution of linear differen-

tial equations with a single time delay to create a map in order to study the stability

J.-Q. Sun (



)

School of Engineering, University of California, Merced CA 95344, USA

e-mail: jqsun@ucmerced.edu

A.C.J. Luo (ed.), Dynamical Systems: Discontinuity, Stochasticity and Time-Delay,

DOI 10.1007/978-1-4419-5754-2

12,

 Springer Science+Business Media, LLC 2010

135

136 B. Song and J.-Q. Sun

of the system. A series of papers in [12–14] have studied optimal feedback gain

designs based on the mapping of an extended state vector. For deterministic delayed

linear systems, a survey of methods for stability analysis is presented in [15]. An ex-

cellent survey of stability and control of time-delayed systems can be found in [16].

There have also been many studies of control systems with unknown and time-

varying time delays. Chen et al. derived sufﬁcient conditions for the existence of

the guaranteed cost output-feedback controller in terms of matrix inequalities for

uncertain dynamical systems with time delay [17]. The Lyapunov method is used

in [18] for the stability analysis of systems with time-varying delay with known

lower and upper bounds. The Lyapunov function dependent on the known upper

bound of uncertain state-delays is derived in the study of model predictive controls

(MPC) for a constrained linear digital systems with uncertain state-delays [19]. A

class of iterative learning control systems with uncertain state delay and control

delay is studied in [20]. Robust stability of uncertain linear systems with interval

time-varying delay is studied in [21]. Stability of systems with bounded uncertain

time-varying bounded delays in the feedback loop is studied in [22]. The stabil-

ity problem is treated in the integral quadratic constraint (IQC) framework. Kwon,

Park and Lee [23] investigated delay-dependent robust stability for neutral systems

with the help of the Lyapunov method. The system has time-varying structured un-

certainties and interval time-varying delays. A compensation scheme that consists

of a fuzzy-PID controller and a neural network compensator is proposed for real-

time control over the network is studied in [24]. This scheme reduces the inﬂuence

of time delays on stability while maintaining the system performance. According

to [25], given a ﬁnite-dimensional linear time invariant (LTI) plant and an upper

bound on the admissible time delay, there is no general theory for designing a con-

troller to handle an arbitrarily large uncertain delay. The authors show that given

a ﬁnite-dimensional LTI plant and an upper bound on the admissible time delay,

there exists a linear periodic controller which robustly stabilizes the plant. Robust

stability for systems with random time-varying delay with a known probability dis-

tribution is studied in [26]. The resulting system model has stochastic parameters.

Sufﬁcient conditions for the exponential mean square stability of the system are

derived by using the Lyapunov functional method and the linear matrix inequal-

ity (LMI) technique.

When the uncertain time delay is bounded with known lower and upper bounds,

we can consider the supervisory control [27–30]. The supervisory control proposes

to use several estimates of uncertain parameters for the system model. For each

estimate of the parameter, a control is designed to achieve the desired performance.

A supervisor monitors the real-time response of the system, selects a plant model

according to a switching criterion, and implements the corresponding control.

In this chapter, we review some recent control studies of dynamical systems

with time delay. We ﬁrst review two approximate methods for computing the

response of time-delayed systems, discuss their properties and applications to feed-

back controls. We also introduce the supervisory control of systems with unknown

time delay. The inﬂuence of the range of the unknowntime delays on the supervisory

control is discussed. A number of examples are included to demonstrate the theo-

retical discussions.

12 Control of Systems with Time Delay 137

12.2 Methods of Solution

12.2.1 Semi-Discretization

Consider a linear periodic system with time delay

x.t/ D A.t/x.t/CA

.t/x.t  / C B.t/u.t/; (12.1)

where x 2 R

and u 2 R

. A.t/ 2 R

nn

, A

.t/ 2 R

nn

and B.t/ 2 R

nm

are pe-

riodic matrices with period T . We shall consider a feedback control with or without

time delay in the following forms

u.t/ DKx.t/ or u.t/ DKx.t  /; (12.2)

where K 2 R

mn

is the gain matrix. In the closed loop system, the control simply

modiﬁes the matrix A.t/ or A

.t/.

When we introduce the method of semi-discretization, we can focus on the fol-

lowing system without loss of generality,

x.t/ D A.t/x.t/CA

.t/x.t  /: (12.3)

Because of the time delay, the state vector of the system is no longer just x.t/,

but .x

.t/; x

.t  

for all 0<

 , which has an inﬁnite dimension. The

time delay signiﬁcantly complicates the solution process of the system.

Let us discretize the period T into an integer k intervals of length t such that

T D kt. For the sake of simplicity, we assume that the time delay  D Nt

where N is an integer. When an integer N cannot be found, discretization of the

time delay  will be approximate [31], or a continuous time approximation can be

adopted as discussed in Sect.12.2.2.

Consider (12.3) in a time interval t 2 Œt

iC1

,wheret

D it, i D

0;1;2;:::;k. In each small time interval Œt

iC1

, the delayed responses x.t  /

and the time dependent coefﬁcients are assumed to be constant. We denote

x.t

 / D x..i  N/t/ D x

iN

;

A.t

/ D A

; A

/ D A

: (12.4)

Equation (12.3) becomes

x.t/  A

x.t/ D A

.t/x.t / t 2 Œt

iC1

; i D 0;1;2;:::;k: (12.5)

The general solution of the equation is

x.t/ D e

.tt



t/x.

t  /d

t 2 Œt

iC1

; i D 0;1;2;:::;k: (12.6)

138 B. Song and J.-Q. Sun

The integration on the RHS of the above equation can be computed by assuming

that A

.t/x.t  / is a constant or a linear function of time over the small interval

Œt

iC1

.Theworkin[32] has studied the accuracy of these approximation schemes.

As an example, we show the case when A

.t/x.t  / is assumed to be constant

over Œt

iC1

: The response x

iC1

D x.t

iC1

/ at time t

iC1

can then be expressed in

the following mapping

iC1

D Q

C P

iN

: (12.7)

where

iC1

.t/

d; Q

t

; (12.8)

Deﬁne an .N C1/n dimensional state vector as



i1

i2

::: x

iN



: (12.9)

A mapping of the state vector over the interval Œt

iC1

 can be found as

iC1

D H

; (12.10)

where the transition matrix from time t

to t

iC1

n.N 1/n

nn

n.N 1/n

nn

.N 1/nn

.N 1/n.N 1/n

.N 1/nn

: (12.11)

The mapping of the state vector over one period T D kt is therefore

j C1

D ˆy

; (12.12)

where the mapping matrix ˆ is given by

ˆ D H

k1

k2

H

: (12.13)

Note that the index j (j D 0; 1; :::) refers to the number of periods, i.e. y

is the

state vector at the beginning of the j th period.

The stability of the control system is determined by the eigenvalues of ˆ.Let

jj

max

denote the largest absolute value of eigenvalues of the matrix ˆ. Then,

j C1

jjj

max

j: (12.14)

When jj

max

<1, ˆ is a contraction, and the control system is asymptotically

stable. The stability boundary is given by jj

max

D 1. Equation (12.14) indicates

that the smaller jj

max

is, the faster the system converges to zero. jj

max

therefore

also provides a measure of the control performance.