Litton C.W., . Reynolds D.C., Collins T.C. Zinc Oxide Materials for Electronic and Optoelectronic Device Applications

Подождите немного. Документ загружается.

Furthermore, observation of the atomic force microscopy (AFM) images revealed that the

surface of an MQW is composed of well-deﬁned atomically ﬂat terraces and steps

corresponding to the charge neutral unit of ZnO. In other words, the interface roughness

in the heterostructure cannot be larger than 0.26 nm. It can be said that ZnO and MgZnO alloy

layers grow in a two-dimensional growth mode on this substrate, resulting in the formation of

a sharp hetero-interface between them.

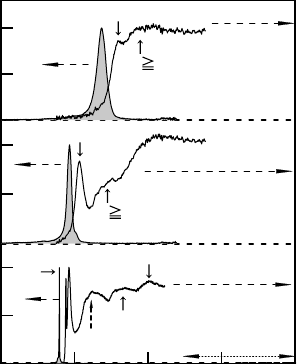
We show, in Figure 12.1, luminescence (PL) and absorption spectra in ZnO/

0.12

0.88

O MQWs on SCAM substrates measured at 5 K with well widths ( L

)of

17.5 and 6.9 A



. Also shown for comparison in the bottom panel of the ﬁgure is PL and

absorption spectra in a 500-A



-thick ZnO epilayer on the lattice-matched SCAM substrate.

Both the PL and absorption peaks shifted towards the higher energy side as L

decreased.

This shift resulted from a quantum conﬁnement effect. For ref erence, we note that the

exciton Bohr radius is 18 A



[29]

The absorption peaks (n ¼ 1) arise from the lowest

excitonic states of well layers. The peak energies of PL were constantly located on the

lower energy side of those of absorption peaks.

The well width dependences of the peak energies of PL (closed circles) and absorption

(open squares) are shown in Figure 12.2(b) and (c), respectively.

[28]

The lowest transition

energy of excitons (open triangles) formed with conﬁned electrons and holes was

calculated using a model of one-dimensional, ﬁnite periodic square-well potential

proposed by Gol’dman and Krivchnokov.

[30]

The exciton binding energy (60 meV)

[31]

Absor

tion

4.03.83.63.43.2

Photon Ener

(eV)

Normalized PL Intensity (arb. units)

ZnO(500A)

barrier abs.

17.5A

(3.5L)

He-Cd

5.0 K

= 6.91A

(1.5L)

n =1

n 2

n = 1

A, B

B+LO

B+2LO

B+3LO

Figure 12.1 PL and absorption spectra obtained from ZnO/Mg

0.12

0.88

O MQWs measured

at 5 K for L

of 17.5 and 6.9 A



. The absorption energy of the barrier layers is shown by a

horizontal arrow. Spectra obtained from a 500-A



-thick ZnO ﬁlm are also shown. “B þLO,

B þ2LO, and B þ3LO” correspond to exciton–phonon complex transitions, “n ¼ 1” shows the

lowest excitonic absorption of the well layers, and “n  2” means the excited states of the

exciton or higher interband transitions. Reprinted from Makino, T., et al., Room-temperature

luminescence of excitons in ZnO/(Mg,Zn)Omulti-quantum wells on lattice-matched substrates,

App. Phys. Lett., 77,975. Copyright (2000) with permission from American Institute of Physics

334 Room-Temperature Stimulated Emission from ZnO Multiple Quantum Wells

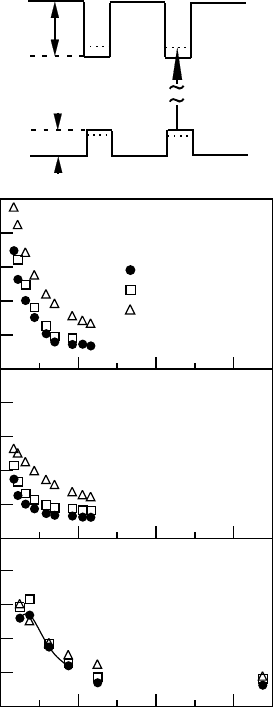
3.7

3.6

3.5

3.4

3.3

12080400

Well width (A)

(d)

ZnO(L

)/Mg

0.15

0.85

on sapphire

3.7

3.6

3.5

3.4

3.3

(c)

ZnO(L

)/Mg

0.12

0.88

on SCAM

Exciton Resonant Energy (eV)

3.8

3.7

3.6

3.5

3.4

3.3

(b)

ZnO(L

)/Mg

0.27

0.73

on SCAM

Abs

Calc

(a) ZnO/Mg

0.12

0.88

O MQW

189 meV

21 meV

Figure 12.2 (a) Diagram of conduction and valence bands between barrier and well layers in

a ZnO/Mg

0.12

0.88

O MQW.

[9]

The upward arrow shows the lowest interband transition. (b)

Peak energies of PL (circles) and absorption (squares) are plotted against L

in [ZnO/

0.27

0.73

on SCAM substrates. Results of calculation (triangles) of the interband

transition energy that include the excitonic effect are also shown. (c) Similar except that the

Mg content was 12%. (d) Similar except that the substrate was sapphire. The Mg content was

15%. Energies of PL excitation spectra (squares) are plotted instead of those of absorption

spectra, due to the presence of 100-nm-thick ZnO buffer layers. Note that the peak energies of

PL excitation spectra coincide with those of the absorption spectra. The curve is a visual

guide.

[28]

Reprinted from Makino, T., et al., Room-temperature luminescence of excitons in

ZnO/(Mg,Zn)Omulti-quantum wells on lattice-matched substrates, App. Phys. Lett., 77,975.

Quantum Conﬁnement Effect of Excitons in QWs 335

is assumed to be independent of L

here. The optical transition process on a ZnO/

0.12

0.88

O MQW is shown in Figure 12.2(a). This tendency of the L

dependence of

the exciton transition energy was consistent with the calculation results. As reported by

Coli and Bajaj,

[32]

incorporation of the effects of exciton–phonon interaction and the

quantum conﬁnement in calculation, leads to values of the excitonic transitions that are in

good agreement with our experimental data. Figure 12.2(d) shows the corresponding peak

energy plot for MQWs grown on sapphire substrates. As shown in Figure 12.2(d), both

peak energies have a maximum at L

of 15 A



when sapphire substrates were employed.

This indicates a critical thickness that prevents quantum conﬁnement with respect to the

exciton energy. It is speculated that the existence of the critical thickness corresponds to

the poor controllability of layer thickness due to the lattice mismatching.

12.4 Exciton–Phonon Interaction in QWs

Despite the viable technological applications and remarkable achievements of ZnO, the

fundamental properties, such as electron–phonon interaction are less understood. The

intrinsic properties cannot be controlled but must be understood since they play an

essential role in the device operations. The coupling constant between excitons and

phonons in ZnO QWs (MQWs) has not been evaluated quantitatively so far. We quantify

the coupling constant by estimating the temperature dependence of the absorption spectra.

Figure 12.3 shows the temperature depend ence of the full width at half-maximum

(FWHM) of the excitonic absorption peaks for a ZnO epilayer [Figure 12.3(a)] and for

a typical MQW sample with a QW width of 17.5 A



[Figure 12.3(b)]. The solid line

represents the ﬁtted results based on Equation (12.1). The temperature dependence of the

FWHM can be approximately described by:

[33]

GðTÞ¼G

þg

T þG

=½expðhv

TÞ1ð12:1Þ

where G

, hv

(72 meV), g

, G

and k

are the inhomogeneous linewidth at

temperature (T) of 0 K, longitudinal optical (LO)-phonon energy, strengths of the

exciton-acoustic-phonon and the exciton-LO-phonon couplings and the Bolzmann con-

stant, respectively. It was experimentally found that hv

of the MQWs is not different

from the bulk value.

Figure 12.4 (closed circles, left axis) shows the values of G

obtained for the epilayer

and its well width (L

) dependence obtained for ZnO/Mg

0.12

0.88

O MQWs. The values

of G

of the MQWs are smaller than those for the epilayers and monotonically decrease

with decrease in L

. Here we try to explain this result by the enhancement of exciton

binding energy induced by the quantum conﬁnement effect. Figure 12.4 (open circles,

right axis) shows the L

dependence of exciton binding energy. This depend ence was

determined by studying spectra of stimulated emiss ion. As is well known, the major

process that contributes to broadening of the exciton linewidth is scattering of 1S excitons

into the free-electron–hole continuum or into excited excitonic states by absorbing LO

phonons. If exciton binding energy exceeds hv

(72 meV), dissociation efﬁciency into

the continuum states is greatly suppressed compared with the case of exciton binding

energy smaller than hv

. In such case, G

is reduced. Indeed, exciton binding energy

336 Room-Temperature Stimulated Emission from ZnO Multiple Quantum Wells

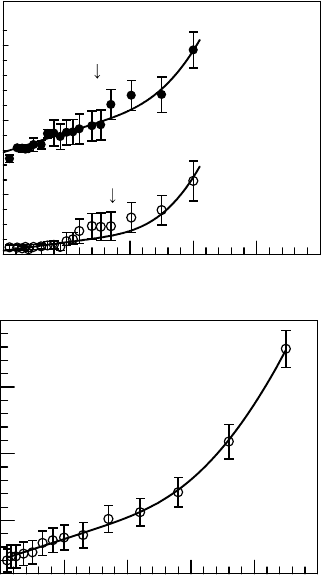
exceeds hv

in the case of MQWs. A similar effect has also been observed in other QW

systems.

[37]

A schematic explanation is described in the literature.

[35,38]

12.5 The Localization Mechanism of the Exciton in a QW

It was found that the excitonic luminescence in the ZnO MQWs under investigation is

due to radiative recombination from excitons localized by the potentials form ed by the

ﬂuctuation s of L

and barrier height. Our spectral as signments are based on (1) the well

width dependence of Stokes shift (difference between the energies of absorption and

luminescence bands), (2) the temperature dependence of PL spectra, and (3) the

spectral distribution (luminescence energy dependence) of decay time constants of

FWHM (meV)

250200150100500

Tem

erature (K)

(b) ZnO quantum well

(well width = 50 Å)

FWHM (meV)

250200150100500

Temperature (K)

A-exciton

B-exciton

(a) ZnO epilayer

Figure 12.3 (a) Width (full width at half-maximum, FWHM, circles) of A- and B-exciton

absorption bands plotted as a function of temperature. Open circles are data of the A-excitons

and the closed circles are data of the B-excitons. The solid curves represent the ﬁtting results.

(b) Similar plot for the MQW with Mg concentration of 0.12 and L

of 46.5 A



[34,35]

Reprinted

from Sun, H. D., et al., Temperature dependence of the exciton linewidth in ZnO/(Mg,Zn)O

multi-quantum wells grown on lattice matched substrates. Appl. Phys. Lett. 78, 2464. Copyright

(2001) with permission from American Institute of Physics

The Localization Mechanism of the Exciton in a QW 337

luminescence.

[28,35,39,40]

A typical example of the spectr al distri bution of decay time

constants is shown in Figure 12.5(b). Her e, the temperature dependence of the PL

spectrum in a QW in the case of magnesium composition of 0.27 is described in detail.

Figure 12.5(a) shows the temperature dependence of PL (solid line) and that of

absorption (broken line) spectra in ZnO(17.5 A



)/Mg

0.27

0.73

O MQWs over a temperature

range of 5–300 K. It should be noted that spectra obtained at temperatures between 95 K

and 200 K had two peaks, both of which originated from a recombination of localized

excitons. The separation of these peaks was 12–20 meV. Figure 12.5(b) shows PL decay

time as a function of monitored photon energy at 5 K in the same MQW. The solid curve is

the results of theoretic al calculation based on the model of the excitonic localization.

[28,41]

Figure 12.6(a) summarizes peak energies of the PL spectra (E

) (open circles and

triangles) and the excitonic absorption energy (open squares) as functions of temperatures.

It should be noted that the higher PL peak position does not coincide with that of

absorption spectra even at temperatures near room temperature. We also examined, for

comparison, the temperature dependence of PL peak energy in an MQW having a lower

barrier height: a ZnO/Mg

0.12

0.88

O MQW with a well width of 27.9 A



. Figure 12.6(b)

shows the peak energies of PL (circles ) and absorption (squares) spectra in this sample. In

this case, the energies of luminescence and absorption are the almost the same as each

other at temperatures near room temperature. Two kinds of MQWs having different barrier

heights showed signiﬁcantly different temperature dependences of PL spectra.

Following a temperature rise, the PL energy of ZnO(17.5 A



)/Mg

0.27

0.73

O MQWs

exhibited low energy shifts between 5 K and 50 K, blue-shifts between 50 K and 200 K,

and again shifts to a low energy side at temperatures higher than 200 K. Furthermore, at

temperatures between 95 K and 200 K, the spectra had two peaks, both of which originated

Exciton bindin

ener

y (meV)

54321

Well width (nm)

900

800

700

600

500

400

300

200

Exciton-LO-phonon coupling Γ

(meV)

ZnO/Zn

0.88

0.12

O MQW

Bulk ZnO

hω

Figure 12.4 Strengths of coupling between excitons and LO phonons G

(closed circles) and

exciton binding energies (open circles) in bulk ZnO and MQWs with different L

[36]

The solid

curve is a visual guide

338 Room-Temperature Stimulated Emission from ZnO Multiple Quantum Wells

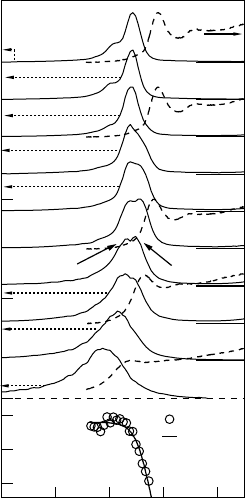
from a recombination of localized excitons. The absorption peak energies both in ZnO

epilayers and in MQWs are monotonically decreasing functions of temperature as was

revealed in previous studies.

[34,35]

This can be explained in terms of narrowing of the

fundamental energy gap induced by a temperature rise.

In general, when a dominant PL peak is assigned to a radiative recombination of localized

excitons, its peak energy blue-shifts with increase in temperature in a range of low

temperatures and red-shifts at higher temperatures. The E

blue-shifts and continuously

connects to that of free excitons due to thermal activation of localized excitons. The E

of the

free-excitonic emission is a monotonically decreasing function of temperature due to the

band gap shrinkage. The temperature dependence shown in Figure 12.5(a) is, however,

different from the abovementioned typical behavior. The temperature dependence of the

recombination mechanism for localized excitons is thought to be closely related to the

temperature variation in the decay time constant of their PL. Thus temperature dependence of

PL decay times (t

) in an MQW with a L

of 27.9 A



is shown in Figure 12.7(c). The t

PL intensity (arb. units)

3.63.53.43.33.2

Photon ener

(eV)

200

150

100

Decay (ps)

(a) L

=17.5 Å

= 0.27

5 K

25 K

50 K

80 K

95 K

120 K

175 K

200 K

240 K

300 K

Absor

tion (arb. units)

(b) 5 K

exp.

calc.

Figure 12.5 (a) PL (solid line) and absorption (broken line) spectra in a ZnO (17.5 A



0.27

0.73

O MQW over the temperature range of 5–300 K. All of the spectra have been

normalized and shifted in the vertical direction for clarity. (b) PL decay times as a function of

monitored photon energy at 5 K in the same MQW. The solid curve is results of the theoretical

calculation based on the model of the excitonic localization. Reprinted from Makino, T., et al.,

Temperature dependence of near ultraviolet photoluminescence in ZnO/(Mg,Zn)O multi-

quantum wells, Appl. Phys. Lett. 78, 1979. Copyright (2001) with permission from American

Institute of Physics

The Localization Mechanism of the Exciton in a QW 339

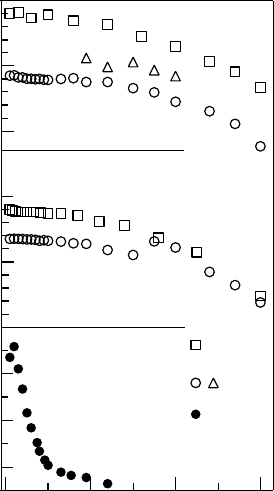
values exhibit nonmonotonical behavior with respect to temperature; the value increased in a

low temperature range and decreased above a certain critical temperature.

The temper ature dependence of the recombination mechanism for localized excitons

can be explained

[42]

as follows: (i) For 5 K G T G 50 K, the relatively long relaxation time

of excitons gives the excitons more opportunity to relax down into lower energy tail states

caused by the inhomogeneous potential ﬂuctuations before recombining. This is because

radiative recombination processes dominate in this temperature range. This behavior

produces a red-shift in the peak energy position with increasing temperature. (ii) For

50 K G T G 95 K, the exciton lifetimes decrease with increasing temperature. Thus, these

excitons recombine before reaching the lower energy tail states. This behavior enhances a

broadening of the higher-energy side emission and leads to a blue-shift in the peak energy.

(iii) For 95 K G T G 200 K, further enhancement of high-energy emission components

produces a new peak, as seen in Figur e 12.6(a ) (triangles). (iv) At temperatures above

200 K, since the excitons are less affected by the temperature-induced rapid change in their

lifetimes and since the relaxation rate of the excitons increased due to an increase in the

3.50

3.45

3.40

Peak energies (eV)

3002001000

Tem

erature (K)

3.40

3.35

3.30

Peak energies (eV)

300

200

100

Decay time (ps)

abs

(b) L

=27.9 Å

= 0.12

(a) L

= 17.5 Å

= 0.27

= 27.9 Å

x =

0.27

Figure 12.6 PL (open circles and triangles) and absorption (open squares) peak positions as a

function of temperature in ZnO(17.5 A



)/Mg

0.27

0.73

O (a) and ZnO(27.9A



)/Mg

0.12

0.88

O(b)

MQWs. (c) Temperature dependence of PL decay times, t

, in ZnO(27.9 A



)/Mg

0.27

0.73

MQWs at temperatures of 5–120 K.

[28]

Reprinted from Makino, T., et al., Temperature depen-

dence of near ultraviolet photoluminescence in ZnO/(Mg,Zn)O multi-quantum wells, Appl.

Phys. Lett. 78, 1979. Copyright (2001) with permission from American Institute of Physics

340 Room-Temperature Stimulated Emission from ZnO Multiple Quantum Wells

phonon population, blue-shift behavior becomes less pronou nced. Since the energy of

blue-shift is les s than the temperature -induced band gap shrinkage, the peak position

again exhibits a red-shift behavior. As mentioned above, the features of excitonic

spontaneous emission in the well layers are sensitively affected by the dynamics of

recombination of localized exciton states, which signiﬁcantly vary with temperature.

Readers should refer to the literature for details.

[39]

12.6 Time-Resolved Luminescence in ZnO QWs

Time-resolved PL (TRPL) is a nondestructive and powerful technique that has been

commonly used for the optical characterization of direct-ga p semiconductors. One can

measure by this time-domain spectroscopy the exciton recombination time, an important

parameter related to material quality and device performance. The exciton lifetimes will

vary with crystal quality, becoming longer as the quality improves or localization of the

excitons become deeper.

Reynolds et al.

[43]

measured the recombination lifetime of the free excitons at 2 K in a

single-crystal ZnO grown by the hydrothermal method.

In addition to the time-integrated luminescence, time-resolved luminescence of ZnO

MQW were measured in order to investigate the exciton dynamics in this hetero-structure.

Chia et al.

[44]

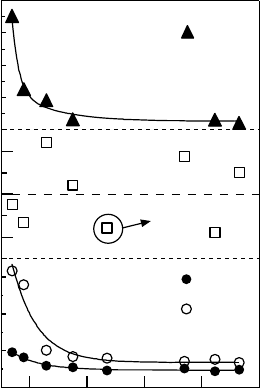
presented results of TRPL measurements for the ZnO QWs. Figure 12.7 shows

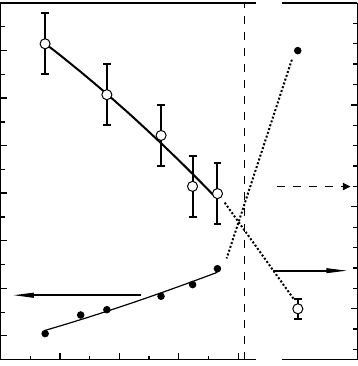
600

400

200

Decay Time Constant (ps)

5040302010

Well Width (Å)

400

300

200

7.0

6.0

5.0

4.0

3.0

Depth (meV)

rad

nonrad

ZnO MQWs

Figure 12.7 Well-width dependences of localization depth (E

, closed triangles) and recom-

bination times, t

(closed circles), t

rad

(open circles), and t

nonrad

(open squares). The solid

curves are drawn as a guide to the eye. Reprinted from Makino, T., et al., Temperature

dependence of near ultraviolet photoluminescence in ZnO/(Mg,Zn)O multi-quantum wells,

Appl. Phys. Lett. 78, 1979. Copyright (2001) with permission from American Institute of Physics

Time-Resolved Luminescence in ZnO QWs 341

the TRPL data for the QWs as a function of well layer thickness. The time-resolved

luminescence data ﬁtted well with a single exponential decay curve function with a several

hundreds of picoseconds decay component. Evaluated PL decay time is a monotonically

decreasing function of the emission energy. In other words, decay time constants in the low

energy side are longer than those in the higher counnterparts. This suggests that not only

radiative recombination but also the transfer process to the tail state should contribute to the

decay of the localized excitons in the QW system. Figure 12.7 shows the decay time constant

of localized excitons (t

, closed circles) and E

(closed triangles) as a function of the well

width. When the well width becomes thin, it is found that both the t

and E

become longer.

The dependence of the radiative (t

rad

) and nonradiative (t

nonrad

) recombination times on the

well layer thickness was deduced. Combined analysis of temperature dependences of PL

decay times and of spectrally integrated PL intensity was carried out. Because the radiative

recombination process is relatively dominant at a low temperature, the values of the

nonradiative process have relatively large error bars. Nevertheless, it can be said that, as

a general trend, the nonradiative recombination time is independent of the well width. The

well width dependence of radiative recombination time can be explained as being due to the

thermal release effect from the localized to delocalized states of excitons. It could be noticed

that the width dependences of the localization depth of excitons and the radiative recombi-

nation times are similar with respect to each other. If the localization effect is absent in QWs,

radiative recombination time is a linearly increasing function of temperature in the entire

temperature range. Because of the localization effect, radiative recombination time becomes

stagnant at low temperatures. It is necessary to systematically estimate the dependence of

radiative recombination time on temprature for QWs having various well width in order to

clarify the radiative recombination mechanism in ZnO MQWs.

[40]

On the other hand, we discuss here the well width dependence of nonradiative

recombination time. Norma lly, the nonradiative recombination becom es shorter in the

MQWs in the case of thinner wells because of the degraded ﬁlm qualities and carrier

leakage outside the well region. However, we have not observed this tendency in this work.

This may suggest that an efﬁcient carrier conﬁnement inside the well region could be

realized in the QWs studied here. Some dispersion in the data is related to certain

inaccuracy in the determination of the well width and magnesium concentration in the

barrier layer, which is not taken into account.

12.7 Stimulated Emission in MQWs

This section is devoted to high excitation effects, stimulated emission and lasing, covering

the range over QWs. As shown in Figure 12.8, the energies of spontaneous PL and

absorption peaks are almost the same at RT.

[28]

The spontaneous emission spectrum was

obtained under the condition of excitation using a 5 mW helium cadmium laser operated in

the continuous-wave mode, while the stimulated emission spectrum was obtained under

the condition of high-power excitation usin g a frequency-tripled mode-locked Nd:YAG

laser (355 nm, 10 Hz, 15 ps) operate d in the pulsed mode. The power of excitation was

varied, as is described later. The agreement between the spontaneous emission and

absorption peaks is an indication of the well-regulated hetero-interfaces as well as the

342 Room-Temperature Stimulated Emission from ZnO Multiple Quantum Wells

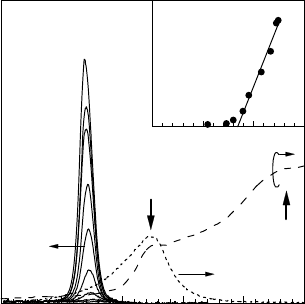
small compositional ﬂuctuations in the barrier layers (well-depth ﬂuctuations). Such RT

PL in the MQWs grown on the sapphire substrates was not found.

We performed high-power excitation experiments to determine the characteristics of

stimulated emission in ZnO MQWs in the optical pumping mode. Figure 12.8 s hows

stimulated em ission spectra of M QWs with x ¼ 0:12 and L

¼ 17:5A



that were

obtained at RT. Strong and s harp emission peaks wer e observed at 3.24 eV above a

very low threshold (I

¼17 kW cm

2

), and their integrated intensities rapidly increased

as the excitation intensity (I

) increased, as can be seen in the inset. Although such

stimulated emission was not observed even at 4.2 K in the case using a sapphire

substrate, RT stimulated emission in MQWs studied here was observed. This is one of

the signiﬁcant improvements achieved b y applying lattice-matching conditions to a

substrate.

The L

dependences of peak energies of the absorption and stimulated emissions are

summarized in Figure 12.9. Similar dependence of the threshold is also shown in

Figure 12.9(c). The stimulated emission energy is 100 meV lower than that of the

absorption peak. The lowest threshold value was 11 kW cm

2

in the case of L

of 47 A



We tested the high-temperature operation of the stimulated em ission f rom the view-

point of possible applications to devices. Figure 12.10 shows the temperature dependence

of the I

stim

I

curves of a MQW with x ¼ 0:26 and L

¼4.2 nm in the temperature ran ge

of 294–377 K. Here, I

stim

is the intensity of the stimulated emission and I

is the excitation

intensity. The threshold of the stimul ated emission (I

) increased gradually with

increasing temperature. The inset shows the temperature dependence of I

on a logarith-

mic scale. Characteristic temperature, which is an index of stability of threshold

PL Intensity

(arb. units)

3.63.53.43.33.23.1

Photon ener

(eV)

Stimulated Emission Intensity (arb. units)

Absorbance

(arb. units)

= 18 Å

294 K

Nd:YAG

exc.

He-Cd

exc.

Well

Barrier

Intensity

20100

(kW/ cm

–2

)

17 kW/ cm

–2

Figure 12.8 Excitation intensity (I

) dependence of the stimulated emission spectra obtained

from a ZnO/Mg

0.12

0.88

OSL(L

¼1.8 nm) under the condition of pulsed excitation at RT.

Spontaneous PL (dotted line) under the condition of continuous-wave excitation and absorp-

tion (broken line) spectra are also shown. Inset shows the integrated intensity of the stimulated

emission peak as a function of I

. Threshold intensity ( I

)is17kWcm

2

[

]

Ohtomo, A., et al.,

Room-temperature stimulated emission of excitons in ZnO/(Mg,Zn)O superlattices, Appl. Phys.

Lett, 77, 2004. Copyright (2000) with permission from American Institute of Physics

Stimulated Emission in MQWs 343