Litton C.W., . Reynolds D.C., Collins T.C. Zinc Oxide Materials for Electronic and Optoelectronic Device Applications

Подождите немного. Документ загружается.

photoluminescence spectra of a homoepitaxial sample with prominent I

(Al-donor) and I

(Ga-donor) neutral donor bound exciton recombinations. The I

(In-donor) recombination is

rather weak. They are accompanied by high energy transitions at 3.3726 and 3.3718 eV

labeled I

and I

, respectively. These two lines have the same intensity ratio as I

and I

Moreover, in samples with prominent I

recombination as found e.g. for the samples grown

on GaN templates (interdiffusion of Ga into the ZnO layer) the I

and I

lines show up almost

exclusively. For samples grown on sapphire substrates (Al

), Al is the dominant impurity

in the ZnO ﬁlms, and I

and I

are observed as correlated pairs. Another correlated pair of

lines exists for I

and I

. In Na-doped ZnO crystals due to compensation the neutral donor

bound exciton recombination I

related to In had the highest luminescence intensity (see

Figure 2 in Meyer et al.)

[21]

followed by I

. The I

and I

recombinations were much lower

in intensity as was the I

line. A similar intensity ratio of I

to I

was found in a Li-doped

sample, here the I

recombination was more pronounced.

[21]

As to the nature of the I

to I

recombinations magneto-optical experiments provided a

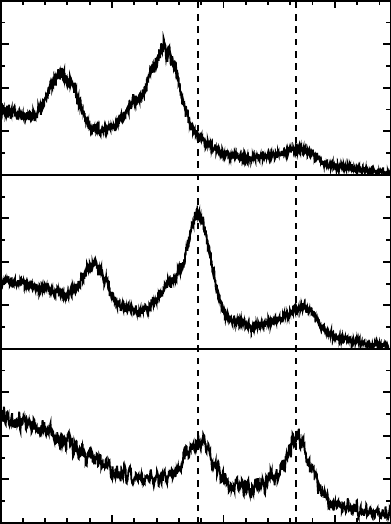
conclusive picture. In Figure 6.8 the magnetic ﬁeld behavior of I

and I

(for I

see the

published data in the literature)

[15,31]

is presented. Both lines show the appearance of an

3.3733.3723.3713.370

PL-intensity (arb. units)

Ener

y (eV)

B = 0 Tesla

B = 2 Tesla

B = 5 Tesla

Figure 6.8 Photoluminescence measurements at T ¼1.6 K on a homoepitaxial ZnO thin ﬁlm

showing the behavior of the I

and I

recombinations in an applied magnetic ﬁeld (Voigt

conﬁguration). Reprinted from B.K. Meyer, J. Sann, S. Lautenschlaeger, M.R. Wagner, and A.

Hoffmann, Ionized and neutral donor-bound excitons in ZnO, Phys. Rev.B 76, 184120.

142 Spectral Identiﬁcation of Impurities and Native Defects in ZnO

additional line at the low energy side of I

and I

in an applied magnetic ﬁeld. In the case of

this component is superimposed by the I

bound exciton transition. Without an applied

magnetic the lines are not visible (see Figure 6.8). The extrapolation of the position of the

low energy Zeeman component to B ¼0 Tesla reveals the presence of a zero ﬁeld splitting.

This is strong evidence for ionized donor bound excitons.

[31]

Additionally, one does not

observe a linear splitting in Voigt conﬁguration as would be expected for a neutral bound

exciton. Therefore, there is convincing evidence that I

to I

are caused by ionized donor

bound exciton recombinations.

In Figure 6.6 the localization energies of the ionized and neutral donor bound excitons

as a function of the donor binding energy are presented. Two aspects are remarkable: The

localization energy E

has a linear dependence on the donor binding energy E

for both

neutral and ionized bound excitons, although with a different slope, and for donor binding

energies of E

G 47 meV, excitons will not be bound to the ionized donors.

The shallow donor impurities in ZnO seem to be of extrinsic origin, hydrogen,

aluminum, gallium and indium in order of increasing binding energy. However, for many

years it was common sense that intrinsic defects dominate the n-type conductivity of

ZnO.

[32,33]

Interstitial zinc and oxygen vacancies were the natural choice. Interstitial zinc

as well as oxygen vacancies should be double donors, and in order to contribute to the

n-type conduction they should have shallow levels, and low formation energies to be

abundant. Theoretical calculations predict indeed that the oxygen vacancies are the main

intrinsic defects in zinc-rich ZnO whereas Zn

have higher formation energies, i.e. are less

abundant.

[34]

However, the oxygen vacancy theory predicts a negative-U behavior with a

transition from the neutral to the twofold positive charge state around E

þ0.5 eV, i.e. the

vacancy does not induce a shallow level.

[34]

From electrical measurements (Hall, DLTS, admittance spectroscopy) on various ZnO

single crystals from different sources as well as on epitaxial ﬁlms there is general consensus

that apart from the shallow donor a deep donor state exists which is located around

130 20 meV below the conduction band. Its concentration falls into the 10

3

range

thus being relevant for the conductivity as well as for the compensation of acceptors.

[35–40]

The Zn interstitial is a double donor in ZnO. It should exist in three charge states, 2þ, þ,

and 0, and thus have two energy levels in the gap. If we assume the level at E

130 meV

is the 2þ/þ level of the zinc interstitial the transition þ/0 would fall in the range where the

binding energies of the extrinsic shallow donors are i.e. from 46 to 53 meV. Look et al.

[41]

reported on Hall effect measurements of electron irradiated ZnO. They concluded with the

presence of a native donor in ZnO whose binding energy would be around 35 meV.

In Figure 6.9 we compare three ﬁlms grown under different Zn/O ratios.

[42]

In the ﬁlm

grown (# 1) under oxygen-deﬁcient conditions the prominent recombination occurs at

3.366 eV (in Reynolds et al.

[15]

and Meyer et al.

[17]

named I

). It has comparable intensity

as the neutral donor bound exciton recombinatio n with Ga as shallow donor (I

3.359 eV). For sample # 1 the additional NO

ﬂux was 100 sccm, for # 2 it was 200 sccm

and for # 3 it was 300 sccm. One notes that with increasing NO

ﬂux the I

recombination

decreases in intensity more and more. Finally, for the sample # 3 the neutral donor bound

exciton recombination I

is the main radiative excitonic recombination. In sample # 3 the

recombination is shifted to lower energy by 0.7 meV, it is connected to a change in the c-

lattice constant as revealed by X-ray diffraction measurements. Also the line width

increased which is consistent with the ﬁndings in Ko et al.

[43]

When a ﬁlm grown under

Zn-rich conditions was annealed in an O

atmosphere at high temperatures e.g. T ¼900



Optical Spectroscopy 143

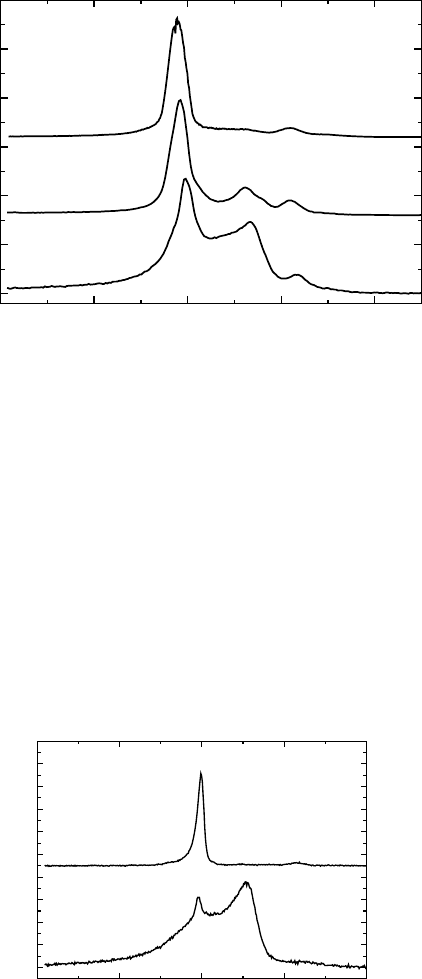
with O

at atmospheric pressures, and for 30 min the I

recombination disappears (see

Figure 6.10). This can be explained by the fact that excess Zn is oxidized to form ZnO.

Both experiments provide evidence that under Zn-rich conditions a shallow center is

introduced that binds excitons and gives rise to the I

recombination. The localization

energy of the I

recombination is 9.9 meV and is hence different from the recombinations

and I

, which are caused by ionized donor bound excitons (see above). If one assumes a

neutral donor bound exciton transition as the origin with E

loc

¼9.9 meV a do nor binding

energy of 37.5 meV will result (using the data shown in Figure 6.6), which is in close

agreement with the data from electrical measurements of 35 meV by Look et al.

[41]

Such a

3.383.373.363.353.34

Ener

y (eV)

(a)

(b)

I3a

PL-intensity (arb. units)

Figure 6.10 Photoluminescence spectra of an as-grown Zn-rich ZnO ﬁlm (a) and after subse-

quent annealing in oxygen atmosphere (b) (for details see text; T ¼4.2 K, HeCd excitation).

Reprinted from B.K. Meyer, S. Lautenschlaeger, S. Graubner, C. Neumann, and J. Sann, Mater.

Res. Soc. Symp. Vol. 891 , 0891-EE08-02.1. Copyright (2006) Materials Research Society

3.383.373.363.353.34

Ener

y (eV)

# 2

# 1

# 3

I3a

Intensity (arb. units)

Figure 6.9 Photoluminescence spectra of ZnO epitaxial ﬁlms grown under different Zn/O

ratios, the additional NO

ﬂux was 100 (# 1), 200 (# 2) and 300 (# 3) sccm, respectively

(T ¼4.2 K, HeCd excitation). Reprinted from B.K. Meyer, S. Lautenschlaeger, S. Graubner, C.

Neumann, and J. Sann, Mater. Res. Soc. Symp. Vol. 891 , 0891-EE08-02.1. Copyright (2006)

Materials Research Society

144 Spectral Identiﬁcation of Impurities and Native Defects in ZnO

shallow donor level will contribute to the electrical transport properties signiﬁcantly. The

electrical properties of the samples grown under Zn- and O-rich conditions were analyzed

by Hall effect measurements in the Van der Pauw conﬁguration at room temperature. The

Zn-rich ﬁlm had almost a factor of ten higher carrier concentration than the O-rich ﬁlm (n-

type carrier density of 6.2 10

3

and a mobility of 110 cm

1

vs 6.7 10

3

and 194 cm

1

In a recent work on MBE grown ZnO

[35]

it was demonstrated that the level ET2

0.12 eV) is present in Zn-rich and stoichiometric ZnO but appears to be less abundant

in O-rich ZnO (concentration 2 10

3

in Zn-rich and 2 10

3

in O-rich). Oh

et al. further concluded that the trap L1 found in bulk ZnO corresponds to ET2 and may be

assigned to the Zn interstitial.

[35]

Its second ionization energy (2þ/þ) was estimated to be

0.2 eV below the conduction band. Oh et al.

[35]

observed another trap ET1 in ZnO with

thermal activation energies of 33 meV under Zn-rich ﬂux conditions and stoichiometric

ﬂux conditions and with 46 meV under O-rich ﬂux conditions. It was a factor 10 lower in

concentration than ET2 but showed a similar trend i.e. being less abundant in O-rich ZnO.

This level is commonly observed

[44–48]

and Hagemark and Chacka

[48]

suggested that the

defect causing this shallow donor level is due to the sing ly ionized Zn interstitial.

The evidence for an intrinsic defect is supported by SIMS experiments. In the ﬁlms

grown either Zn-rich or O-rich the extrinsic impurity content did not change. Since in the

growth experiments beside the Zn/O ratio all other parameters remained unchanged

(growth temperature, ﬁlm thickness, etc.) extrinsic donors cannot be made responsible for

the change in carrier density upon change in stoichiometry. Whether the defect giving rise

to the I

recombination is an isolated point defect or a more complex species remains to be

established.

6.2.2 Recombinations Caused by Nitrogen and Arsenic Doping

In wide-band-gap semiconductors bipolar doping has been a challenge of research for

many years. ZnO obviously has a doping asymmetry, since it can easily be doped n-type

into concentrations where it shows metallic conduction, but reports on successful,

reproducible, stable and homogeneous p-type doping are very rare.

[49–56]

In the late

1950s lithium and sodium doping into ZnO was tested but the group-I elements act as self-

compensating centers – Li (Na) at interstitial sites is a donor and incorporated substitu-

tionally on Zn sites it behaves as an acceptor.

[57–59]

Interstitial Li (Na) donors in ZnO have

so far escaped detection. However, on the basis of mag netic resonance experiments it

could be demonstrated convincingly that Na and Li are on Zn sites. In contrast to other

II–VI semiconductors they are deep (600–800 meV) acceptors

[57–59]

caused by a substan-

tial lattice distortion.

Nitrogen on an oxygen site can be considered to be the best candidate as demonstrated

by its success in p-type doping ZnSe

[60]

and ZnS.

[61]

There are many theoretical reports on

the behavior of N in ZnO revealing a complex behavior by the interaction with intrinsic

defects, passivation by hydrogen and formation of nitrogen molecules.

[62–64]

Experiments

used ion implantation,

[17,28,65]

doping during growth

[49,51,55,66]

and diffusion.

[67]

There are

only a few reports as to the optical properties of the materials in terms of DAP

recombination involving a shallow nitrogen acceptor level.

[51,65–69]

The optical activity

of nitrogen was investigated in samples prepared by three different techniques: Nitrogen

doping of ZnO was carried out by ion implantation (10

ions cm

2

) at room temperature,

Optical Spectroscopy 145

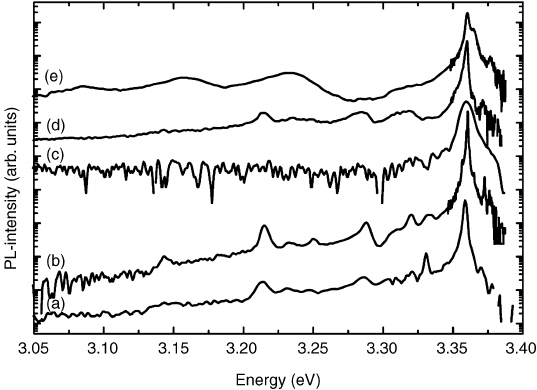
by diffusion from a thermal decomposition of ammonium or lithium nitrate, and during

growth of epitaxial ﬁlms using ammonia as nitrogen sourc e.

[70]

In Figure 6.11 we compare two samples implanted at a total dose of 10

2

in the

as-receivedstate [Figure 6.11(a)] and after rapidthermal annealing at 900



C [Figure6.11(b)].

The spectradiffer only in small details and are representative of the recombinations seen in the

as-grown ZnO substrate. Due to the small mass of nitrogen the implantation damage results in

a reduction of the overall luminescence intensity by a factor 5–10. Similar results were

obtained when the dose was changed to 10

2

. Therefore, Zn and N were subsequently

implanted into ZnO within the same box proﬁle. As seen in Figure 6.11(c) the implantion

damageis now substantial, thebandedgeemissionduetobound excitonsishardly observable.

At temperatures above 800



C [see Figure 6.11(d)] a recovery of the luminescence occurs,and

for a temperature of 900



C nitrogen was activated as seen in the appearance of the DAP

recombination located at 3.23 eV followed by phonon replica [see Figure 6.11(e)]. The

ﬁndings about the recovery of the luminescence are very similar to the results of Schilling

et al.

[25]

in Ar- or Al-implanted ZnO crystals. The luminescence [see Figure 6.11(e)] is still

dominated by the neutral donor bound exciton recombinations (Al is the major trace impurity

in the bulk substrates), and there are no indications as to the appearance of a neutral acceptor

bound exciton line connected with the incorporation of N into ZnO.

Following the approach of Rommelu



ere et al.

[67]

the incorporation of N into ZnO from a

thermal decomposition of ammonium and lithium nitrate salts was studied. The diffusion

of N from the gas phase into ZnO results in the very same DAP recombination as found for

the implanted samples [see Figure 6.12(b); Figure 6.12(a) shows the luminescence of the

Figure 6.11 Photoluminescence spectra of bulk ZnO as-implanted (a) and after annealing of

the as-implanted sample at 900



C (b), dose: 10

2

N; (c) as-implanted, annealing of the

as-implanted sample at 800



C (d) and at 900



C (e), dose: 10

2

N, Zn co-implant.

Reprinted from B.K. Meyer, et al., Shallow donors and acceptors in ZnO, Semicond. Sci.

Technol. 20, S62. Copyright (2005) with permission from IOP Publishing

146 Spectral Identiﬁcation of Impurities and Native Defects in ZnO

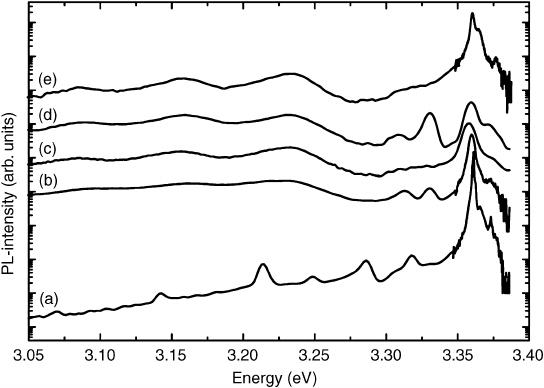
substrate before treatment]. Similar results were obtained for LiNO

[see Figure 6.12(c)}.

Figure 6.12(d) shows the luminescence spectrum of an epitaxial ZnO ﬁlm doped with

N during growth using NH

as a nitrogen source. The observation of the same DAP band in

samples where nitrogen was introduced by three different techniques, implantation, in situ

doping and diffusion from the gas phase, provides strong evidence that nitrogen on oxygen

site is the acceptor.

[70]

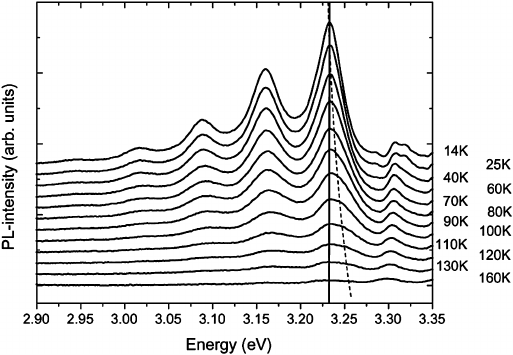
Temperature-dependent measurements could be performed up to 150 K before the

signal disappears in the noise (see Figure 6.13). The transi tion energy is not very

temperature dependent. The band gap energy decreases as a function of temperature

[E(T) ¼E(T ¼0)(5.05 10

4

)/(900T)] which amounts to 16 meV for 150 K. With

increasing temperature a line emerges at higher energies and take s over in intensity which

is typical for a change to a conduction band to acceptor transition when the donors start to

become ionized. Correcting for the shift of band gap on temperature the band-acceptor

transition shifts to higher energies with approximately

The acceptor binding energy can be estimated from the peak position of the zero -

phonon line (ZPL) of the DAP transition at 3.23 eV:

¼ðE

E

Þ½EðD

; A

ÞaN

1=3



where E

is the shallow donor binding energy, N

is its concentration and a ¼3 

5

meV cm. In Zeuner et al.

[66]

time resolved luminescence was used to determine N

and more importantly the acceptor binding energy E

of 165 40 meV. Similar conclu-

sions on E

have been derived by Reuss et al.

[28]

on N-implanted ZnO.

Figure 6.12 Photoluminescence spectra of ZnO samples: (a) bulk crystal as received, and

after annealing in the presence of ammonium nitrate (b) and lithium nitrate (c). Spectrum

(d) shows a ZnO epitaxial ﬁlm with in situ doping by ammonia and (e) is for a comparison the

implanted and annealed sample shown in Figure 6.1. Reprinted from B.K. Meyer, et al.,

Shallow donors and acceptors in ZnO, Semicond. Sci. Technol. 20, S62. Copyright (2005) with

permission from IOP Publishing

Optical Spectroscopy 147

There are a few reports on the optical activity of N in ZnO.

[51,65–69]

Reuss et al.

[28]

studied N-implanted ZnO and agreed in all major points with the ﬁndings presented

elsewhere.

[17,65,66,71]

Yamauchi et al.

[68]

showed in ZnO layers grown by plasma-assisted

epitaxy in mixed oxygen-nitrogen gas plasma a DAP emission at 3.27 eV with practically

identical phonon coupling. They derived an acceptor binding energy of 135 meV assuming

a shallow donor binding energy of 40 meV. Tamura et al.

[69]

reported on a DAP band in

ZnO ﬁlms, the ﬁlms were doped in situ by a radio frequency plasma activated N species .

They observed a more or les s structureless band which for nitrogen concentrations of

2 10

3

was centered around 3.16 eV, and determined a binding energy of 266 meV

for the acceptor. Wang and Giles

[72]

determined the ionization energy of nitrogen

acceptors in bulk ZnO in as-grown, thermally annealed and N

added samples. They

assigned a DAP line at 3.216 eV to the nitroge n acceptor, and estimated a binding energy

of 209 meV. It appears that there is a discrepancy in the data of the acceptor binding energy

of N in ZnO which should be resolved in the future.

SIMS was applied to determine the concentration of nitrogen and unintentional dopants

such as hydrogen in samples containing different nitrogen concentrations.

[71]

Nitrogen

was detected as



and hydrogen as



clusters. The given absolute con-

centrations are accurate to within half an order of magnitude. Nitrogen related vibra-

tions

[70]

were studied by Raman-scattering experiments in backscattering geometry.

Wurtzite ZnO belongs to the C

symmetry group and, theref ore, there are the

Raman-active phonon modes E

(low), E

(high), A

(TO), A

(LO), E

(TO) and E

(LO).

The B

modes are silent. According to the well-known selection rules we expect to observe

the E

modes and the A

(LO) mode in unpolarized Raman spectra taken in backscattering

geometry. Their respective frequencies are 101, 437 and 574 cm

1

[73]

Figure 6.14 shows Raman spectra of nitrogen-doped ZnO. Beside the expected E

(low)

and E

(high) mode of ZnO we ﬁnd a variety of other modes, which need to be explained.

Figure 6.13 Temperature-dependent photoluminescence measurements on the DAP band in

a ZnO epitaxial ﬁlm doped with nitrogen. The straight line gives the line position at low

temperatures; the dashed line indicates the shift to higher energies at high temperatures

148 Spectral Identiﬁcation of Impurities and Native Defects in ZnO

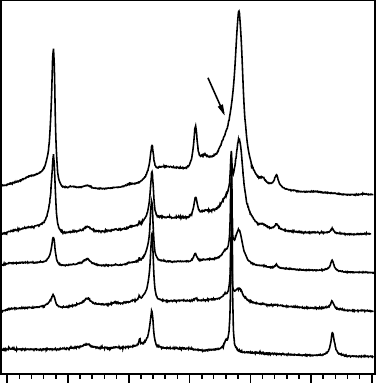
At 569 cm

1

one ﬁnds the E

(high) mode of the GaN template. The feature at 332 cm

1

a second-order structure of ZnO, which was interpreted as 2E

(M) by Calleja and

Cardona.

[74]

There are ﬁve more modes with frequencies of 275, 510, 582, 643 and

856 cm

1

which do not belong to ﬁrst- or second-order structures of ZnO or the GaN

template material and are nitrogen-related.

In total ﬁve samples with different nitrogen concentration as estimated from the growth

parameters were investigated (see Figure 6.14). Sample A is an undoped ZnO reference,

and the estimated nitrogen concentration increases from sample B to sample E. Neither of

the additi onal modes is found in undoped material. Also in ZnO:Ga (not shown) none of

these Raman modes was observed. The intensity of the ﬁve modes increases with nitrogen

concentration. Furthermore, the intensity of the A

(LO) mode from the GaN template

subsequently decreases from A to E. This results from the higher absorption in the visible

spectral range with increasing nitrogen concentration.

[75]

The correlation of the mode

intensities and the nitrogen concentration leads to the conclusion that all the additional

modes are local vibrational modes (LVMs) related to nitrogen in ZnO. For a quantitative

correlation of the nitrogen concentration and the LVMs SIMS measurements (Figure 6 .15)

were performed. The nitrogen concentration was deduced from the signal intensity of the



cluster consisting of

N and

O isotopes. The detection limit of this method is

around 10

3

. As expected from the growth parameters the nitrogen concentration

increases from sample A to E with a maximum concentration of about 10

3

Simultaneously, the hydrogen concentration as detected by the



cluster linearly

increases with the nitrogen content. Since a calibration standard for hydrogen was not

800700600500400300200

EXC

= 514.5 nm

RT, || pol.

(LO) - GaN-

643

582

(high)

- GaN-

510

(high)

(M)

275

ZnO:N

Scattering Intensity (arb. units)

Raman Shift (cm

-1

)

Figure 6.14 Room temperature Raman spectra of ﬁve ZnO samples. Sample A is undoped,

whereas the nitrogen concentration increases from sample B to E. The peak marked by an

asterisk originates from the sapphire substrate. Reprinted from A. Kaschner, et al., Nitrogen-

related local vibrational modes in ZnO:N, Appl. Phys. Lett. 80, 1909. Copyright (1969) with

permission from American Institute of Physics

Optical Spectroscopy 149

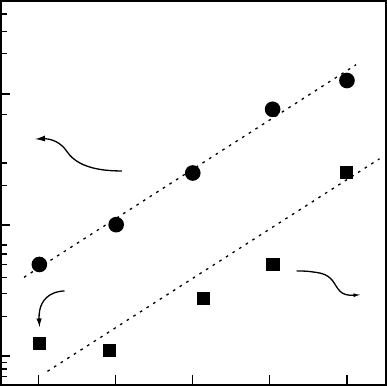
available, a quantitative scaling of the hydrogen concentration is unfortunately not

possible. Nevertheless, a qualitative comparison of the samples can be given. A higher

nitrogen concentration seems to drive the material to build in a higher concentration of

compensating hydrogen (see Figure 6.15). A similar mechanism as for GaN, where the

concentration of magnesium acting as an acceptor and compensating hydrogen are linearly

correlated,

[76]

may be the reason for thi s behavior. This fact may have a strong impact on

the compensation mechanism in ZnO.

The LVMs with frequencies of 275, 510, 582 and 643 cm

1

have a linear dependence on

the nitrogen concentration in the sample, which is the ﬁnal evidence for their origin being

related to vibrations of nitrogen in ZnO.

[71]

The different slopes may indicate different

formation probabilities of the nitrogen-containing complexes.

[71]

The exact conﬁguration

of the vibrating nitrogen-related complex cannot be deduced from these experiments.

First-principles calculations show that the formation of N

and related complexes is very

likely in ZnO.

[77]

The vibrational properties and the correlation between the acceptor and

hydrogen seems very similar in ZnO:N and GaN:Mg.

[78]

The research on p-type doping was stimulated when in 1997 Minegishi et al.

[49]

reported on p-type behavior of chemical vapor deposition (CVD) grown ZnO ﬁlms doped

with nitrogen. Indeed the ﬁrst light-emitting device based on a ZnO p-n homojunction used

nitrogen as acceptor dopant.

[55]

The authors demonstrated that the solubility of nitrogen is

high only at low growth temperatures (G400



C). Substrate temperatures in CVD and

metal organic chemical vapor decposition are typically 200 and 400



C higher limiting the

incorporation of nitrogen. Alternatives have been searched and found by using P and As

AB EDC

Sample

Detection

Limit

(

–

Hydro

en Concentration (arb. units)

Nitrogen Concentration (cm

–3

)

ZnO:N

(

–

Figure 6.15 Nitrogen and hydrogen concentration in samples A–E as determined from SIMS.

The nitrogen concentration increases from sample A to E. The hydrogen concentration linearly

correlates with the nitrogen concentration. Dashed lines are a guide to the eye only. Reprinted

from A. Kaschner, et al., Nitrogen-related local vibrational modes in ZnO:N, Appl. Phys. Lett.

80, 1909. Copyright (1969) with permission from American Institute of Physics

150 Spectral Identiﬁcation of Impurities and Native Defects in ZnO

despite the fact that there is a considerable size mismatch (in terms of ionic radii) of P and

As compared with O [assuming P (As) replaces O].

In comparison with ZnO:N As doping during growth by CVD

[79]

leads to a fundamen-

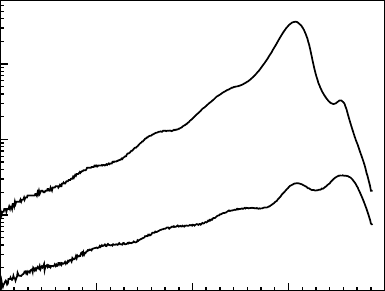
tally different PL spectrum (see Figure 6.16). D

X recombinations are completely absent.

The ﬁrst transition is located at 3.354 eV followed by the most intense line at 3.307 eV. A

sequence of transitions separated by 72 meV (LO phonon frequency) follows which can be

seen more clearly at T ¼110 K (see Figure 6.16). The line at 3.307 eV is assigned to a free

to bound transition, i.e. from the conduction band to an acceptor level (eA

). This

assignment is supported by the temperature-dependent studies, which allowed the acceptor

binding energy of 135 5 meV to be determined. Meanwhile this characteristic transition

centered around 3.31 eV has been observed in a variety of ZnO materials (bulk crystals,

thin ﬁlms and nanostructures) often most prominent in ZnO:P, ZnO:As, ZnO:Sb and ZnO:

N (for a compilation see Schirra et al.).

[80]

It is also present in undoped material and

Schirra et al.

[80]

have made an extensive study on the behavior of this line by temperature-

dependent cathodoluminescence with high spectral resolution, by scanning electron

microscopy and by transmission electron microscopy. A detailed temperature dependence

of the band-acceptor transition and replicas is shown in Figure 6.17. From a line shape

analysis at different temperatures the acceptor binding energy of 130 3 meV was

determined in very close agreement with the value obtained in ZnO:As mentioned above.

The ﬁrst attempt of doping ZnO with As goes back to Ryu et al.

[53]

growing ZnO on

GaAs substrates. They showed by SIMS the diffusion from As into ZnO which was high

close to the interface GaAs/ZnO but maintained a level of 10

3

throughout the rest of

the ﬁlm. They claimed the recombination at 3.32 eV seen in their ﬁlms is caused by an

acceptor bound exciton. In 2003 Ryu et al.

[54]

reported on As-doped p-type ZnO grown by

hybrid beam deposition. The lightly doped ﬁlms with N

in the low 10

3

range [see

Figure 2(b) in Ryu et al.)

[54]

showed recombinations at 3.359 eV attributed to neutral

acceptor bound excitons (A

X), at 3.32 and 3.273 eV attributed to free to bound transitions

(eA

), and at 3.204 eV attributed to DAP recombination. Thus two acceptors with binding

3.43.33.23.13.0

PL-intensity (arb. units)

Ener

y (eV)

(a)

(b)

Figure 6.16 Temperature-dependent photoluminescence measurements in a ZnO epitaxial

ﬁlm doped with arsenic at 4 K (a) and 110 K (b)

Optical Spectroscopy 151