Kurfess T.R. Robotics and Automation Handbook
Подождите немного. Документ загружается.

17
-18 Robotics and Automation Handbook
the latter term following since θ is constant, i.e.,
˜
θ =
ˆ
θ. Using the parameter update law (17.102) gives
˙
V =−e
T
Qe (17.105)
From this it follows that the position tracking errors converge to zero asymptotically and the parameter
estimation errors remain bounded. Furthermore, it can be shown that the estimated parameters converge
to the true parameters provided the reference trajectory satisfies the condition of persistency of excitation,
αI ≤
t
0
+T
t
0
Y
T
(q
d
,
˙
q
d
,
¨
q
d
)Y(q
d
,
˙
q
d
,
¨
q
d
)dt ≤ β I
(17.106)
for all t
0
,whereα, β, and T are positive constants.
In order to implement this adaptive feedback linearization scheme, however, one notes that the accel-
eration
¨
q is needed in the parameter update law and that
ˆ
M must be guaranteed to be invertible, possibly
by the use of projection in the parameter space. Later work was devoted to overcome these two drawbacks
to this scheme by using so-called indirect approaches based on a (filtered) prediction error.
17.3.7 Passivity-Based Approaches
One may also exploit the passivity of the rigid robot dynamics to derive robust and adaptive control
algorithms for manipulators. These methods are qualitatively different from the previous methods which
were based on feedback linearization. In the passivity-based approach, we modify the inner loop control
as
τ =
ˆ
M(q)a +
ˆ
C(q,
˙
q)v +
ˆ
g(q) − Kr
(17.107)
where v, a, and r are given as
v =
˙
q
d
−
q
a =
˙
v =
¨
q
d
−
˙
q
r =
˙
q
d
− v =
˙
q +
q
with K , diagonal matrices of positive gains. In terms of the linear parametrization of the robot dynamics,
the control (17.107) becomes
τ = Y(q,
˙
q, a, v)
ˆ
θ − Kr
(17.108)
and the combination of Equation (17.107) with Equation (17.41) yields
M(q)
˙
r +C(q,
˙
q)r + Kr = Y
˜
θ
(17.109)
Note that, unlike the inverse dynamics control, the modified inner loop control (17.41) does not achieve
a linear, decoupled system, even in the known parameter case
ˆ
θ = θ . However, in this formulation the
regressor Y in Equation (17.109) does not contain the acceleration
¨
q nor is the inverse of the estimated
inertia matrix required. These represent distinct advantages over the feedback linearization based schemes.
17.3.8 Passivity-Based Robust Control
In the robust passivity-based approach of [36], the term
ˆ
θ in Equation (17.108) is chosen as
ˆ
θ = θ
0
+ u (17.110)
where θ
0
is a fixed nominal parameter vector and u is an additional control term. The system (17.109)
then becomes
M(q)
˙
r +C(q,
˙
q)r + Kr = Y(a, v, q,
˙
q)(
˜
θ +u)
(17.111)
Copyright © 2005 by CRC Press LLC

Robust and Adaptive Motion Control of Manipulators 17
-19
where
˜
θ = θ
0
− θ is a constant vector and represents the parametric uncertainty in the system. If the
uncertainty can be bounded by finding a nonnegative constant, ρ ≥ 0, such that
˜
θ=θ
0
− θ ≤ρ (17.112)
then the additional term u can be designed according to the expression,
u =
−ρ
Y
T
r
||Y
T
r ||
,if||Y
T
r || >
−
ρ
Y
T
r,if||Y
T
r || ≤
(17.113)
The Lyapunov function
V =
1
2
r
T
M(q)r +
q
T
K
q (17.114)
is used to show uniform ultimate boundedness of the tracking error. Note that
˜
θ is constant and so is not
a state vector as in adaptive control. Calculating
˙
V yields
˙
V =r
T
M
˙
r +
1
2
r
T
˙
Mr + 2
q
T
K
˙
q (17.115)
=−r
T
Kr + 2
q
T
K
˙
q +
1
2
r
T
(
˙
M − 2C)r + r
T
Y(
˜
θ +u) (17.116)
Using the passivity property and the definition of r, this reduces to
˙
V =−
q
T
T
K
q −
˙
q
T
K
˙
q +r
T
Y(
˜
θ +u)
(17.117)
Uniform ultimate boundedness of the tracking error follows with the control u from (17.113). See [36]
for details.
Comparing this approach with the approach in the section (17.3.5), we see that finding a constant bound
ρ for the constant vector
˜
θ is much simpler than finding a time-varying bound for η in Equation (17.44).
The bound ρ in this case depends only on the inertia parameters of the manipulator, while ρ(x, t)in
Equation (17.69) depends on the manipulator state vector and the reference trajectory and, in addition,
requires some assumptions on the estimated inertia matrix
ˆ
M(q).
17.3.9 Passivity-Based Adaptive Control
In the adaptive approach the vector
ˆ
θ in Equation (17.109) is now taken to be a time-varying estimate of
the true parameter vector θ. Combining the control law (17.107) with (17.41) yields
M(q)
˙
r +C(q,
˙
q)r + Kr = Y
˜
θ
(17.118)
The parameter estimate
ˆ
θ may be computed using standard methods such as gradient or least squares. For
example, using the gradient update law
˙
θ =−
−1
Y
T
(q,
˙
q, a, v)r (17.119)
together with the Lyapunov function
V =
1
2
r
T
M(q)r +
q
T
K
q +
1
2
˜
θ
T
˜
θ
(17.120)
results in global convergence of the tracking errors to zero and boundedness of the parameter estimates
since
˙
V =−
q
T
T
K
q −
˙
q
T
K
˙
q +
˜
θ
T
{
˙
ˆ
θ + Y
T
r } (17.121)
See [38] for details.
Copyright © 2005 by CRC Press LLC
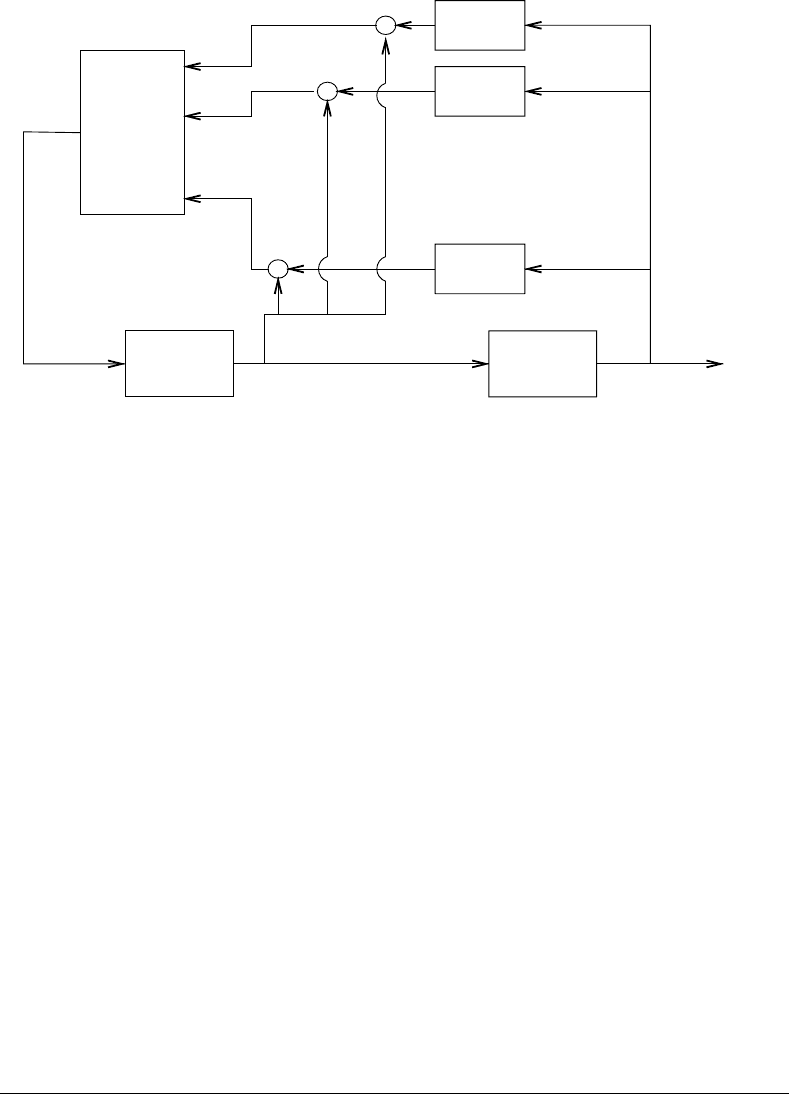
17
-20 Robotics and Automation Handbook
PERFORMANCE
MEASURE
+
+
+
CONTROL
ROBOT
MODEL
N
MODEL
2
MODEL
1
–
–
–
min J
i
J
1
J
2
J
N
e
t2
e
t
N
e
t1
t
^
N
t
^
2
t
^
1
(
q
,
q
.
)
q
^
t
•
•
•
FIGURE 17.9 Multiple-model-based hybrid control architecture.
17.3.10 Hybrid Control
A Hybrid System is one that has both continuous-time and discrete-event or logic-based dynamics.
Supervisory Control, Logic-Based Switching Control, and Multiple-Model Control are typical control
architectures in this context. In the robotics context, hybrid schemes can be combined with robust and
adaptive control methods to further improve robustness. In particular, because the preceeding robust and
adaptivecontrolmethods provide only asymptotic (i.e., as t →∞)error bounds, the transientperformance
may not be acceptable. Hybrid control methods have been shown to improve transient performance over
fixed robust and adaptive controllers.
The use of the term Hybrid Control in this context should not be confused with the notion of Hybrid
Position/Force Control [41]. The latter is a familiar approach to force control of manipulators in which
the term hybrid refers to the combination of pure force control and pure motion control.
Figure 17.9 shows the Multiple-Model approach of [12], which has been applied to the adaptive control
of manipulators. In this architecture, the multiple models have the same structure but may have different
nominal parameters in case a robust control scheme is used, or different initial parameter estimates if
an adaptive control scheme is used. Because all models have the same inputs and desired outputs, the
identification errors e
I
j
are available at each instant for the jth model. The idea is then to define a
performance measure, for example,
J (e
I
j
(t)) = γ e
2
I
j
(t) + β
t
0
e
2
I
j
(σ )dσ with γ, β>0 (17.122)
and switch into the closed loop the control input that results in the smallest value of J at each instant.
17.4 Conclusions
We have given a brief overview of the basic results in robust and adaptive control of robot manipulators.
In most cases, we have given only the simplest forms of the algorithms, both for ease of exposition and
for reasons of space. An extensive literature is available that contains numerous extensions of these basic
results. The attached list of references is by no means an exhaustive one. The book [10] is an excellent and
Copyright © 2005 by CRC Press LLC
Robust and Adaptive Motion Control of Manipulators 17
-21
highly detailed treatment of the subject and a good starting point for further reading. Also, the reprint
book [37] contains several of the original sources of material surveyed here. In addition, the two survey
papers [1] and [28] provide additional details on the robust and adaptive control outlined here.
Several important areas of interest have been omitted for space reasons including output feedback
control, learning control, fuzzy control, neural networks, and visual servoing, and control of flexible
robots. The reader should consult the references at the end for background on these and other subjects.
References
[1] Abdallah, C. et al., Survey of robust control for rigid robots, IEEE Control Systems Magazine, Vol. 11,
No.2,pp.24–30, Feb. 1991.
[2] Amestegui, M., Ortega, R., and Ibarra, J.M., Adaptive linearizing-decoupling robot control: A com-
parative study, Proc. 5th Yale Workshop on Applications of Adaptive Systems Theory, NewHaven,CT,
1987.
[3] Balestrino, A., De Maria, G., and Sciavicco, L., An adaptive model following control for robotic
manipulators, ASME J. Dynamic Syst., Meas., Contr., Vol. 105, pp. 143–151, 1983.
[4] Bayard, D.S. and Wen, J.T., A new class of control laws for robotic manipulators-Part 2. Adaptive
case, Int. J. Contr., Vol. 47, No. 5, pp. 1387–1406, 1988.
[5] Becker, N. and Grimm, W.M., On L
2
and L
∞
-stability approaches for the robust control of robot
manipulators, IEEE Trans. Automat. Contr., Vol. 33, No. 1, pp. 118–122, Jan. 1988.
[6] Berghuis, H. and Nijmeijer, H., Global regulation of robots using only position measurements, Syst.
Contr. Lett., Vol. 1, pp. 289–293, 1993.
[7] Berghuis, H., Ortega, R., and Nijmeier, H., A robust adaptive robot controller, IEEE Trans. Robotics
Automat., Vol. 9, No. 6, pp.825–830, Dec. 1993.
[8] Brogliato, B., Landau, I.D., and Lozano, R., Adaptive motion control of robot manipulators: A
unified approach based on passivity, Int. J. Robust Nonlinear Contr., Vol. 1, pp. 187–202, 1991.
[9] Campion, G. and Bastin, G., Analysis of an adaptive controller for manipulators: Robustness versus
flexibility, Syst. Contr. Lett., Vol. 12, pp. 251–258, 1989.
[10] Canudas de Wit, C. et al., Theory of Robot Control, Springer-Verlag, London, 1996.
[11] Canudas de Wit, C. and Fixot, N., Adaptive control of robot manipulators via velocity estimated
state feedback, IEEE Trans. Automatic Contr., Vol. 37, pp. 1234–1237, 1992.
[12] Ciliz, M.K. and Narendra, K.S., Intelligent control of robotic manipulators: A multiple model based
approach, IEEE Conf. on Decision and Control, New Orleans, LA, pp. 422–427, December 1995.
[13] Corless, M. and Leitmann, G., Continuous state feedback guaranteeing uniform ultimate bound-
edness for uncertain dynamic systems, IEEE Trans. Automatic Contr., Vol. 26, pp. 1139–1144, 1981.
[14] Craig, J.J., Adaptive Control of Mechanical Manipulators, Addison-Wesley, Reading, MA, 1988.
[15] Craig, J.J., Hsu, P., and Sastry, S., Adaptive control of mechanical manipulators, Proc. IEEE Int. Conf.
Robotics Automation, San Francisco, CA, March 1986.
[16] De Luca, A., Dynamic control of robots with joint elasticity, Proc. IEEE Conf. on Robotics and
Automation, Philadelphia, PA, pp. 152–158, 1988.
[17] Desoer, C.A. and Vidyasagar, M., Feedback Systems: Input-Output Properties, Academic Press, New
York, 1975.
[18] Filippov, A.F., Differential equations with discontinuous right-hand side, Amer. Math. Soc. Transl.,
Vol. 42, pp. 199–231, 1964.
[19] Hager, G.D., A modular system for robust positioning using feedback from stereo vision, IEEE Trans.
Robotics Automat., Vol. 13, No. 4, pp. 582–595, August 1997.
[20] Ioannou, P.A. and Kokotovi
´
c, P.V., Instability analysis and improvement of robustness of adaptive
control, Automatica, Vol. 20, No. 5, pp. 583–594, 1984.
[21] Khatib, O., A unified approach for motion and force control of robot manipulators: the operational
space formulation, IEEE J. Robotics Automat., Vol. RA–3,No.1,pp.43–53, Feb. 1987.
Copyright © 2005 by CRC Press LLC
17
-22 Robotics and Automation Handbook
[22] Kim, Y.H. and Lewis, F.L., Optimal design of CMAC neural-network controller for robot manipu-
lators, IEEE Trans. on Sys. Man and Cybernetics, Vol. 30, No. 1, pp. 22–31, Feb. 2000.
[23] Koditschek, D., Natural motion of robot arms, Proc. IEEE Conf. on Decision and Control, Las Vegas,
NV, pp. 733–735, 1984.
[24] Kreutz, K., On manipulator control by exact linearization, IEEE Trans. Automat. Contr., Vol. 34,
No.7,pp.763–767, July 1989.
[25] Latombe, J.C., Robot Motion Planning, Kluwer, Boston, MA, 1990.
[26] Luh, J., Walker, M., and Paul, R., Resolved-acceleration control of mechanical manipulators, IEEE
Trans. Automat. Contr., Vol. AC–25, pp. 468–474, 1980.
[27] Middleton, R.H. and Goodwin, G.C., Adaptive computedtorque controlfor rigid link manipulators,
Syst. Contr. Lett., Vol. 10, pp. 9–16, 1988.
[28] Ortega, R. and Spong, M.W., Adaptive control of rigid robots: a tutorial, Proc. IEEE Conf. on Decision
and Control, Austin, TX, pp. 1575–1584, 1988.
[29] Paul, R.C., Modeling, trajectory calculation, and servoing of a computer controlled arm, Stanford
A.I. Lab, A.I. Memo 177, Stanford, CA, Nov. 1972.
[30] Dahleh, M.A. and Pearson, J.B., L
1
-optimal compensators for continuous-time systems, IEEE Trans.
Automat. Contr., Vol. AC-32, No. 10, pp. 889–895, Oct. 1987.
[31] Porter, D.W. and Michel, A.N., Input-output stability of time varying nonlinear multiloop feedback
systems, IEEE Trans. Automat. Contr., Vol. AC-19, No. 4, pp. 422–427, Aug. 1974.
[32] Sadegh, N. and Horowitz, R., An exponentially stable adaptive control law for robotic manipulators,
Proc. American Control Conf., San Diego, pp. 2771–2777, May 1990.
[33] Schwartz, H.M. and Warshaw, G., On the richness condition for robot adaptive control, ASME
Winter Annual Meeting, DSC-Vol. 14, pp. 43–49, Dec. 1989.
[34] Slotine, J.-J.E. and Li, W., On the adaptive control of robot manipulators, Int. J. Robotics Res., Vol. 6,
No.3,pp.49–59, 1987.
[35] Spong, M.W., Modeling and control of elastic joint manipulators, J. Dyn. Sys., Meas. Contr., Vol. 109,
pp. 310–319, 1987.
[36] Spong, M.W., On the robust control of robot manipulators, IEEE Trans. Automat. Contr., Vol. 37,
pp. 1782–1786, Nov. 1992.
[37] Spong, M.W., Lewis, F., and Abdallah, C., Robot Control: Dynamics, Motion Planning, and Analysis,
IEEE Press, 1992.
[38] Spong, M.W., Ortega, R., and Kelly, R., Comments on ‘Adaptive manipulator control’, IEEE Trans.
Automat. Contr., Vol. AC–35, No. 6, pp. 761–762, 1990.
[39] Spong, M.W. and Vidyasagar, M., Robust nonlinear control of robot manipulators, Proc. 24th IEEE
Conf. Decision and Contr., Fort Lauderdale, FL, pp. 1767–1772, Dec. 1985.
[40] Spong, M.W. and Vidyasagar, M., Robust linear compensator design for nonlinear robotic control,
IEEE J. Robotics Automation, Vol. RA-3, No. 4, pp. 345–350, Aug. 1987.
[41] Spong, M.W. and Vidyasagar, M., Robot Dynamics and Control, John Wiley & Sons, New York,
1989.
[42] Su, C.-Y., Leung, T.P., and Zhou, Q.-J., A novel variable structure control scheme for robot trajectory
control, IFAC World Congress, Vol. 9, pp. 121–124, Tallin, Estonia, August 1990.
[43] Vidyasagar, M., Control Systems Synthesis: A Factorization Approach, MIT Press, Cambridge, MA,
1985.
[44] Vidyasagar, M., Optimal rejection of persistent bounded disturbances, IEEE Trans. Automat. Contr.,
Vol. AC–31, No. 6, pp. 527–534, June 1986.
[45] Yaz, E., Comments on On the robust control of robot manipulators by M.W. Spong, IEEE Trans.
Automatic Control, Vol. 38, No. 3, pp. 511–512, Mar. 1993.
[46] Yoo, B.K. and Ham, W.C., Adaptive control of robot manipulator using fuzzy compensator, IEEE
Trans. on Fuzzy Systems, Vol. 8, No. 2, pp. 186–199, Apr. 2000.
[47] Yoshikawa, T., Foundations of Robotics: Analysis and Control, MIT Press, Cambridge, MA, 1990.
Copyright © 2005 by CRC Press LLC
Robust and Adaptive Motion Control of Manipulators 17
-23
[48] Youla, D.C.,Jabr, H.A., and Bongiorno, J.J., Modern Wiener-Hopf design of optimal controllers–Part
2: the multivariable case, IEEE Trans. Automatic Control, Vol. AC-21, pp. 319–338, June 1976.
[49] Zhang, F., Dawson, D.M., deQueiroz, M.S., and Dixon, W.E., Global adaptive output feedback
tracking control of robot manipulators, IEEE Trans. Automat. Contr., Vol. AC–45, No. 6, pp. 1203–
1208, June 2000.
Copyright © 2005 by CRC Press LLC

18
Sliding Mode
Control of Robotic
Manipulators
Hector M. Gutierrez
Florida Institute of Technology
18.1 Sliding Mode Controller Design–An Overview
18.2 The Sliding Mode Formulation of the Robot
Manipulator Motion Control Problem
18.3 Equivalent Control and Chatter Free Sliding
Control
18.4 Control of Robotic Manipulators by Continuous
Sliding Mode Laws
Sliding Mode Formulation of the Robotic Manipulator Motion
Control Problem
•
Sliding Mode Controller Design
18.5 Conclusions
18.1 Sliding Mode Controller Design -- An Overview
Sliding mode design [1–6] has several features that make it an attractive technique to solve tracking
problemsin motion control of robotic manipulators, the most importantbeing its robustnessto parametric
uncertainties and unmodelled dynamics, and the computational simplicity of the algorithm. One way of
looking at sliding mode controller design is to think of the design process as a two-step procedure. First, a
region of the state space where the system behaves as desired (sliding surface) is defined. Then, a control
action that takes the system into such surface and keeps it there is to be determined. Robustness is usually
achieved based on a switching control law. The design of the control action can be based on different
strategies, a straightforward one being to define a condition that makes the sliding surface an attractive
region for the state vector trajectories. Consider a nonlinear affine system of the form:
x
(n
i
)
i
= f
i
(x) +
m
j=1
b
ij
(x)u
j
, i = 1, ..., m, j = 1, ..., m (18.1)
where u = [u
1
, ..., u
m
]
T
is the vector of m control inputs, and the state x is composed of the x
i
coordinates
to be tracked and their first (n
i
− 1) derivatives. Such systems are called square systems because they
have as many control inputs u
j
as outputs to be controlled x
i
[3]. The motion control problem to be
addressed is the one of making the state vector x track a desired trajectory r. Consider first the time-varying
manifoldσ givenbytheintersectionofthesurfacess
i
(x, t) =0, i =1, ..., m, specified bythe componentsof
Copyright © 2005 by CRC Press LLC

18
-2 Robotics and Automation Handbook
S(x, t) = [s
1
(x, t), ..., s
m
(x, t)]
T
,where
s(x, t) =
d
dt
+ λ
i
n
i
−1
(x
i
−r
i
) = 0 (18.2)
whichcan becomputedfrom x and r , λ
ι
beingsomepositiveconstant.Suchmanifoldis usually called sliding
surface; any state trajectory lying on it tracks the reference r since Equation (18.2) defines a differential
equationon theerror vectorthat stably convergesto zero. Anintegral term can be incorporated to the sliding
surface to further penalize tracking error. For instance, Equation (18.2) can be rewritten for n
i
= 3as
s(x, t) =
d
dt
+ λ
i
2
t
0
(x
i
−r
i
)dt
= (
˙
x
i
−
˙
r
i
) +2λ
i
(x
i
−r
i
) +λ
2
i
t
0
(x
i
−r
i
)dt = 0 (18.3)
There are also several possible strategies to design the control action u
j
that takes the system to the sliding
surface. One such strategy is to find u
j
in a way that each component of the sliding surface s
i
is an attractive
region of the state space by forcing the control action to satisfy a geometric condition such as
1
2
d
dt
s
2
i
≤−η
i
|s
i
|⇔s
i
ds
i
dt
≤−η
i
|s
i
|≤0 (18.4)
whereη
i
is a strictly positiveconstant.This conditionforcesthe squared distance tothe surface (as measured
by s
2
i
) to decrease along all state trajectories [3]. In other words, all the state trajectories are constrained
to point toward σ . A simple solution that satisfies the sliding condition (18.4) in spite of the uncertainty
on the dynamics (18.1) is the switching control law:
u
sw
= k(x, t)sgn(s ), sgn(s ) =
1, s > 0
−1, s < 0
(18.5)
The gain k(x, t) of this infinitely fast switching control law increases with the extent of parametric un-
certainty, introducing a discontinuity in the control signal called chatter. This is an obviously undesirable
practical problem that will be discussed in Section 18.3.
18.2 The Sliding Mode Formulation of the Robot
Manipulator Motion Control Problem
The sliding mode formulation of the robot manipulator motion control problem starts from the basic
dynamic equations that link the input torques to the vector of robot joint coordinates:
H(q)
¨
q + g (q, t) = τ
(18.6)
where τ is the n × 1 vector of input torques, q is the n × 1 vector of joint coordinates, H(q) is the
n ×n diagonal positive definite inertia matrix, and g (q, t)isthen ×1 load vector that describes all other
mechanical effects acting on the robot. This basic formulation can be expanded to describe more specific
motion control problems, e.g., for an ideal rigid-link rigid-joint robot with n links interacting with the
environment [7]:
H(q)
¨
q + c(q,
˙
q) + g (q) − J
T
(q)F = τ (18.7)
where c(q,
˙
q)isann × 1 vector of centrifugal/Coriolis terms, g (q) is the n × 1 vector of gravitational
forces, J
T
(q)isthen × m transpose of the robot’s Jacobian matrix, and F is the m × 1vectorofforces
that the (m-dimensional) environment exerts in the robot end-effector. The motion control problem is
Copyright © 2005 by CRC Press LLC
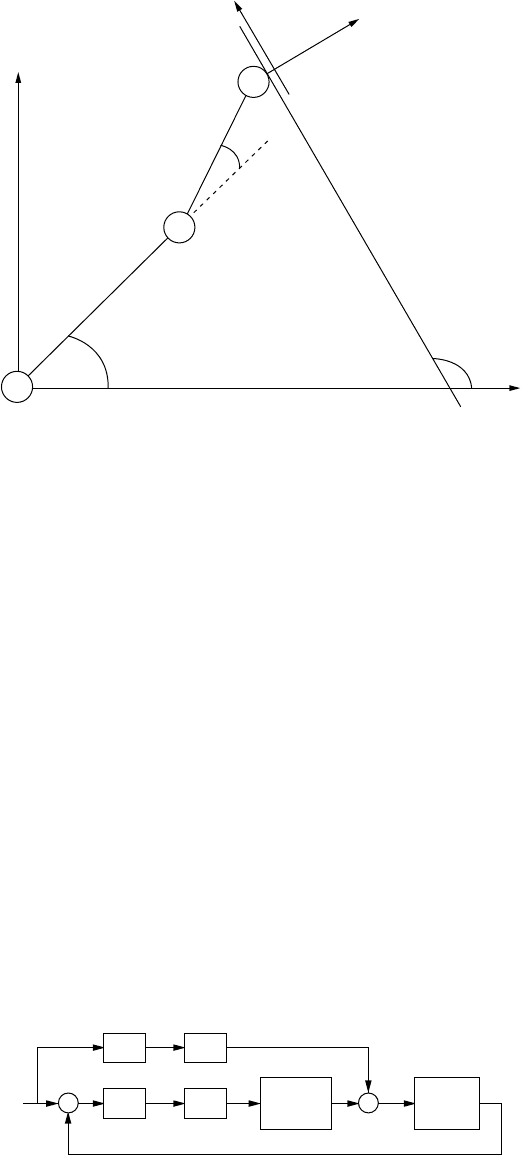
Sliding Mode Control of Robotic Manipulators 18
-3
J
1
q
1
r
1
J
2
m
1
m
2
q
2
r
2
y
c
x
c
constrain surface
q
FIGURE 18.1 Rigid-link rigid-joint robot interacting with a constrain surface.
therefore either a position tracking problem in the q-state space or a force control problem or a hybrid of
both.
Example 18.1
Consider the force control problem of the two-link robot depicted in Figure 18.1 [7]. A control scheme to
track a desired contact force F
d
is shown in Figure 18.2, where J
c
is the robot’s Jacobian matrix relative to
the coordinate system fixed at the point of contact x
c
, y
c
, T is the n × n diagonal selection matrix with
elements equal to zero in the force controlled directions, F is the measured contact force, τ
ff
is the output
of the feed-forward controller, and τ
sm
the output of the sliding mode controller (Figure 18.2).
The matrices used to estimate the torque vector are
H(q) =
(m
1
+ m
2
)r
2
1
+ m
2
r
2
2
+ 2m
2
r
1
r
2
C
2
+ J
1
m
2
r
2
2
+ m
2
r
1
r
2
C
2
m
2
r
2
2
+ m
2
r
1
r
2
C
2
m
2
r
2
2
+ J
2
(18.8)
c(q,
˙
q) =
−m
2
r
1
r
2
S
2
˙
q
2
2
− 2m
2
r
1
r
2
S
2
˙
q
2
1
˙
q
2
2
m
2
r
1
r
2
S
2
˙
q
2
1
(18.9)
F
d
F
I-T J
c
T
force
controller
robot and
constrain
surface
I-T J
c
T
++
+
–
τ
ff
τ
sm
τ
es
FIGURE 18.2 Force controller with feed-forward compensation.
Copyright © 2005 by CRC Press LLC
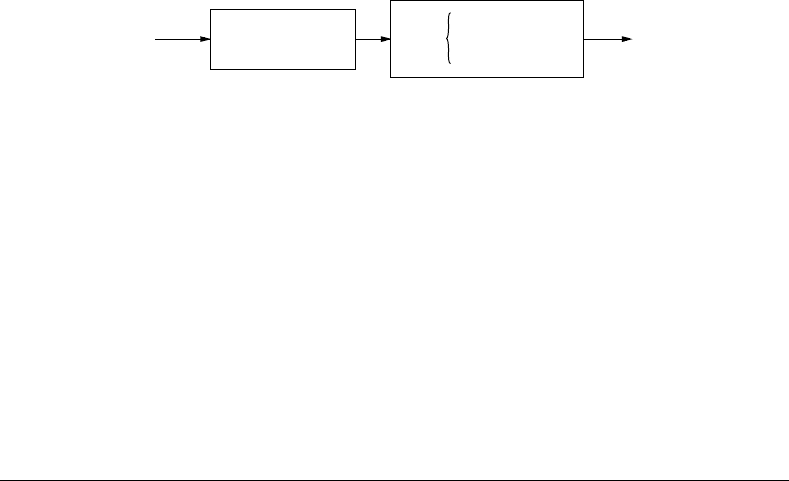
18
-4 Robotics and Automation Handbook
s
es
s
i
s
i
= s
es
+ k
i
∫
s
es
dt
s
sm
i
=
s
max
i
,
s
i
≥ 0
-s
max
i
,
s
i
< 0
sliding surface switching control law
s
sm
FIGURE 18.3 Sliding mode force controller.
g(q) =
g(m
1
r
1
C
1
+ m
2
r
1
C
1
+ m
2
r
2
C
12
gm
2
r
2
C
12
(18.10)
J
T
(q) =
−r
1
S
1
−r
2
S
12
r
1
C
1
+r
2
C
12
−r
2
S
12
r
2
C
12
(18.11)
where C
i
= cos(q
i
), C
ij
= cos(q
i
+q
j
), S
i
= sin(q
i
), S
ij
= sin(q
i
+q
j
).
A similar compensation scheme can be used to solve the position tracking problem.
18.3 Equivalent Control and Chatter Free Sliding Control
Sliding mode control comprises two synergistic actions: reaching the sliding surface and letting the state
vector ride on it. These are represented as two different components of the control action: a switching term
(u
sw
) and a so-called equivalent control (u
eq
). The robustness of sliding control is given by the switching
term, u
sw
, derived from the sliding condition (18.4). This term takes the system from its arbitrary set
of initial conditions to the sliding surface and accommodates parametric uncertainties and unmodelled
dynamics by virtue of the switching action [3, 4, 5]. A purely switching controller can only provide high
performance at the expense of very fast switching. While in theory infinitely fast switching can provide
asymptotically perfect tracking, in practice it implies a trade-off between tracking performance and control
signal chatter, which is either undesirable due to noise considerations or impossible to achieve with real
actuators.
After sliding has been achieved, the system dynamics match that of the sliding surface. Equivalent
control (u
eq
) is the control action that drives the system once sliding has been achieved and is calculated
from solving dS/dt = 0. It is a nonrobust control law because it assumes the plant model to be exact, but
does not generate control chatter because it is a continuous function [5]. In systems that are affine in the
input, such as the one described by Equation (18.1), both actions can be combined into a single control
law (u = u
eq
+u
sw
), and several possible switching terms can be derived by forcing the compound control,
u, to satisfy the sliding condition [5]. An equivalent control term improves tracking performance while
reducing chatter, alleviating the performance trade-off implied by the switching gain k(x, t) in (18.5).
Several different methods have been proposed to deal with control chatter. Two basic approaches can
be distinguished.
1. The boundary layer method, which essentially consists of switching the control action in finite time
as the system’s trajectory reaches an ε-vicinity of the sliding surface.
2. The equivalent control method, which consists of finding a continuous function that satisfies the
sliding condition (18.4). This is particularly important in systems where the control action is
continuous in nature, such as motion control systems where torque, force, or current are treated
as the control input.
A boundary layer [3, 5] is defined as
b
L
(t) ={x, s (x, t)|≤} (18.12)
Copyright © 2005 by CRC Press LLC
