Kounadis A.N., Gdoutos E.E. (Eds.) Recent Advances in Mechanics

Подождите немного. Документ загружается.

Some Actual Problems of Fracture Mechanics of Materials and Structures 419

It is stated that criteria (3) agrees well with experimental data for deformed-

strengthened materials (

σσ 1, 4

> ), when

n = 4, and for deformed-softened

materials (

σσ 1, 4

< ) when

= 2. All experimental data are situated in the

region bounded by curves 1 and 2 (Fig. 4).

Thus it is possible to plot diagrams of limiting-equilibrium state of structural

elements with available cracks or sharp stress concentrators under complex load-

ing of the structure in the frames of LFM. But we still have no appropriate appro-

bation of criteria (3) for cases when one of SIFs is negative.

5 Non-linear Fracture Mechanics

It is known that prior to failure of real structural materials, first of all metals, the

plastic zones at the stress concentrators, in particular crack-like defects, are

formed. The typical linear dimensions of such zones (П-states) can be commensu-

rable with the defect size or the typical linear size of the deformed body. In such

cases the application of the Griffith-Irwin concept (LFM) without additional re-

finements is not correct.

To solve these problems different deformation criteria, in particular the crite-

rion of critical crack tip opening displacement (CTOD criterion) and also the con-

cept of the

δ -model for evaluation of crack opening displacement near its tip,

proposed in [22-24] (1959,1960), are used. The given concept was the beginning

of the investigations in non-linear fracture mechanics of materials, i.e. when the

typical linear dimension of the region of plastically deformed material at the sharp

stress concentrator-crack (П, Fig. 1) is commensurable with the typical size of a

defect or a body (

llΔ≈ , Fig. 5).

Fig. 5. A plate with a central crack (2l

) and model plastic zones (Δl); δ

– displacement of

the initial crack edges in the deformed body under loading p

420 V. Panasyuk and I. Dmytrakh

According to the

δ -model the regions of the body (Fig. 5) where plastic zones

have appeared, are modelled by a cut (cuts) opposite sides of which are attracted

with stresses

σ , which are the averaged local stresses arising in the plastic zone

of the material (for materials without hardening we can assume that

0 T

σ≈σ,

where

σ is yield stress of the material). At all points of the deformed body (out-

side the cuts) the deformations are elastic. Crack opening

δ near the initial crack

tip (mutual displacement of edges) at the moment of the crack start is equal to

constant

()

δ of the material (Fig. 5). Within the framework of the accepted

model for elasoplastic material there exists the equality

σδ 2

between val-

ues

δ ,

σ and density of material fracture

, where

is the average value of

the energy necessary for formation of the surface unity in the given material.

Similar approaches, however partial and developed somewhat later, were pro-

posed by D.S. Dugdale [26] (1960) and A.A. Wells [27].

The concept of the

δ -model was realized for the first time by M.Ya. Leonov

and V.V. Panasyuk, M.Ya. Leonov and P.V. Vytvytskyi on the example of the

generalized problem of A.A. Griffith and M.Ya. Leonov and V.V. Panasyuk using

the Sack's generalized problem (see ref. in [25]).

For the generalized Griffith problem i.e. for the cracked plate under tension

(Fig. 5) when the value of plastic zone

()

lΔ is commensurable with

l (

llΔ≈ )

in the frames of the

δ -model the value of the limiting loading pp

∗

= and

δ value was established for the first time (1960) as

σ arccosexp , ln cos ,

π 2

∗

⎛⎞ ⎛ ⎞

⎛⎞

=−δ=−

⎜⎟ ⎜ ⎟

⎜⎟

πσ

⎝⎠

⎝⎠ ⎝ ⎠

(4)

where

()()

∗

=πδ σ ; E is Young’s modular,

δ is material constant.

The size of the area of inelastic (plastic) deformations in the crack plane (the

value of the zone П) in this case is determined by the equality

sec 1

dlll

⎡

⎤

=− = −

⎢

⎥

⎣

⎦

. (5)

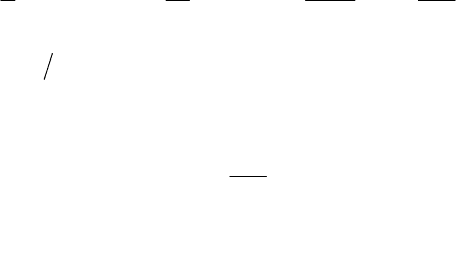
Formula (4) can be used for the crack of any initial length



0lldf



. Accord-

ing to this formula the critical loading

∗

is always finite and (when

0l → )

tends to

σ . This physically sound result is not obtained in the Griffith–Irwin the-

ory (Fig. 6).

Some Actual Problems of Fracture Mechanics of Materials and Structures 421

Fig. 6. Specimen for crack growth resistance of material (K

IIc

, K

IIIc

) determination: 1, 2 –

circular concentrator; 3, 4 – symmetrical cracks; 5, 6 – places of specimen gripping during

torsion (K

IIIc

) or tension (K

IIc

)

In the 80s of the last century (see references in [5]) the

δ -model concept was

generalized for mode II and mode III cracks, and the methods of experimental de-

termination of critical opening displacement (

Іc

δ ) and critical displacement (

ІIc

δ ,

ІIIc

δ ) of the crack edges were proposed.

At the beginning of the 90ies M.P. Savruk et al. [28] used the

δ -model for

plastic bands, initiating from the crack tip at arbitrary angle.

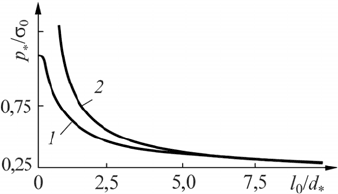
It should be noted that the effective and reliable experimental methods for the

estimation of deformation characteristics of crack growth resistance (

Іc

δ ) have

not been developed yet. Here we meet difficulties presented in [29]. The precise

analysis of elastic-plastic deformation of the material near the crack tip in the ten-

sioned plate basing on the approach that is grounded on the correlation of speckle-

images of the surface in the prefracture zone (Fig. 7) has been done in the above

paper. Here the value of deformation in this zone was assessed at the different

measurement bases

123

(, , )bb b b . Specimens of Д16AT alloy were tested. As a re-

sult the distribution of deformations in the process zone in the loaded and

unloaded plates was established. This distribution is presented in Fig. 7 (curves 1–

3 for different measurement bases

123

(, , )bb b b and

constp =

, and curves 1'–3'

after unloading of the plate accordingly). The received results show that the ex-

perimental determination of critical crack opening displacement (

) between the

crack edges near its tip is not constant, i.e. these displacements are different de-

pending on measurement base (

b ). At the same time experimental results in Fig. 9

illustrate that the size of the process zone

d dose not depend on the measure-

ment base. It remains constant for the given material. This can be used for the

modification of the

-model [25], and of the experimental method for

char-

acteristics determination.

Taking into account the above-mentioned let us consider that the critical length

of the process zone (

∗

) to be the material constant. Than using formulas (4)–(5)

422 V. Panasyuk and I. Dmytrakh

Fig. 7. Distribution of deformations e

(x) near the crack tip under different measurement

bases b (1 – b

= 1.28 mm; 2 – b

= 2.56 mm; 3 – b

= 3.08 mm) in loaded (1–3) and

unloaded (1′–3′) Д16AT alloy specimen; p = const

for the macrocrack in the plate under tension (Fig. 5) one receives such depend-

ence between the averaged value of the deformational crack growth resistance

(





)

and ( d

∗

) – value of the structural material:







10* *

į 8ıʌ,

dE d l





. (6)

Using formula (6) for experimental determination of





one can avoid difficulties

arising in its direct evaluation, and also those concerning loading amplitudes of

the materials specimen when the incoming macrocrack initiates in it.

The following important task is the experimental approbation of the





charac-

teristics evaluation according to formula (6) and application of this approach to es-

tablishing

ȱI





ȱII





6 Fatigue Crack Nucleation and Growth (Fatigue of Materials)

The problem of materials fatigue is one of the central problems of fracture mechan-

ics and prediction of structural elements lifetime (durability). Great efforts have been

spent by scientists and engineers for this problem solution since the 19

century,

when this phenomenon was considered for the first time. The concepts of fracture

mechanics, that is the concepts of cracks initiation and growth in the cyclically

deformed body, are very important for the solution of this problem. If the initiation

period (

N ) of the minimum macrocrack in the given material and its propagation

period (

N ) are known, the total life time ( N

∗

) is determined by formula:

∗

= N

+ N

(7)

where

N and

N are determined using corresponding theoretical and experi-

mental approaches of fracture mechanics [30], in particular using corresponding

characteristics on materials crack growth resistance.

Some Actual Problems of Fracture Mechanics of Materials and Structures 423

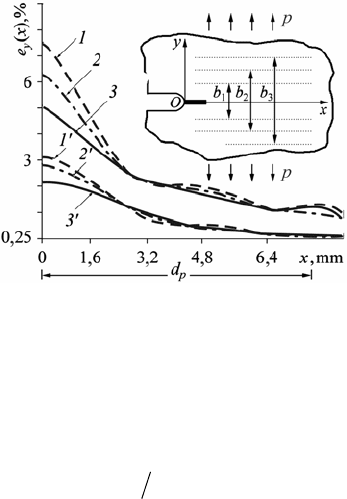
It is known [30, 31] that the material ability to resist crack initiation (resistance

to macrocrack propagation in it) represents the dependence of crack growth rate

(v) on the stress intensity factor (K

) or deformation amplitude of the material at

the crack tip (Fig. 8).

As a result of experimental investigations it was shown that such diagrams ((v–

K)-diagrams) are of S-shape and on a certain region 2 (Fig. 8) they can be consi-

dered rectilinear and can be described ([5] volumes 3 and 4) by Paris equation

()

or 10 ,

vCK v KK

−∗

== (8)

where K

∗

is the value of K

at which the crack growth rate in the given material is

–7

m/cycle (Fig. 8); C, K*, m, and n are material constants. To construct the (v–

K)-diagrams the range of the stress intensity factor ΔK

(ΔK

= K

Imax

– K

Imin

and

ΔK

= K

Imax

when K

Imin

= 0) is used instead of the value of K

= K

Imax

. Every (v–K)-

diagram is bounded on the left by the threshold value of K

Ith

i.e. by such a value of

or ΔK

that for K

Imax

< K

Ith

(or ΔK

Imax

< ΔK

Ith

) the crack does not propagate. On

the right this diagram is bounded by the value of K

Ifc

i.e. by such a SIF value at

which spontaneous failure occurs (v → ∝).

Fig. 8. Diagram of fatigue crack growth resistance of the material (or (v–K)-diagram): 1 is

the region close to threshold K

; 2 is practically rectilinear region; 3 is the region of rapid

crack growth and entire failure under condition K

Imax

= K

Ifc.

So for fatigue fracture of the material we have the following basic fatigue crack

growth resistance characteristics: C, K

Ith

, K

∗

, K

Ifc

, m, n .

For description of complete (v–K)-diagram such formula was proposed in [31]:

0II I I

()/()

th fc

vvKK K K

⎡⎤

=− −

⎣⎦

, (9)

where v

, K

Ith

, K

, q are material constants, obtained experimentally.

424 V. Panasyuk and I. Dmytrakh

If one takes into consideration that the plastic deformations zone at the fatigue

crack tip is small to compare with the crack length, i.e. in case of macrocrack (Δl

<< l

) it is possible to establish [1] the relationship between K

Imax

and

(max)

where δ

is the distance between the opposite crack edged at its tip under the load-

ing of the body by the force p (Fig. 5).

Using the diagram, of fatigue crack growth resistance (Fig. 8), in particular

formula (9),

N value is calculated from the formula:

[()]

() ,

cfc

vK l

Kl K

∗

∫

, (10)

where

∗

is the minimum length of the macrocrack for this material.

The construction of the minimum macrocrack (

∗

) initiation period depending

on the cyclic loading amplitude is a more complicated problem and still has been

no effectively solved. At the same time a certain progress in this direction of in-

vestigations was noticed [30].

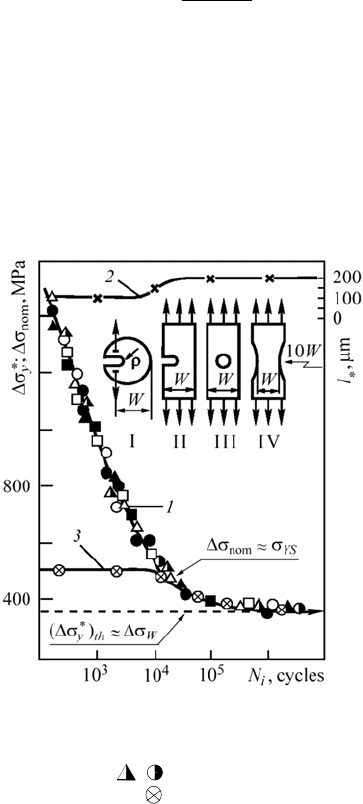
Fig. 9. Dependences of Δσ

nom

∼ N

, (curve 1) and l

∼ N

(curve 2) for notched specimens and

Δσnom ∼ N

(curve 3) for smooth specimens of aluminium Д16чАТ alloy; specimen types: І –

W = 64 mm; ρ = 0,75 and 6,5 mm (U,{); ІІ – W = 64 mm; ρ = 0,75 and 6,5 mm (S, z);

W = 30 mm; ρ = 0,75 and 2,0 mm (

, ); III – W = 30 mm; ρ = 0,75 mm (); W = 20

mm; ρ = 0,75 mm (); IV – W = 10 mm (

)

Some Actual Problems of Fracture Mechanics of Materials and Structures 425

Special investigations on the dependence of the period (

N ) of the minimum

macrocrack initiation in the plates with concentrators on cyclic loading amplitude

(

Δσ ) for the Д16чАТ alloy specimens (Fig. 9) were done [30, 32–34]. The

value of the macrocrack was measured by microscope.

On the basis of dependences

()

1yi

∗

Δσ = it is also possible to establish the

threshold value of

∗

Δσ for the given material, i.e. the value of (

∗

Δσ )

below

which

the crack does not initiate. Let us denote this value

∗

Δσ (threshold) by

(

∗

Δσ )

(see Fig. 9). It equals the ordinate asymptote to the curve

()

1yi

∗

Δσ =

when

N →∞. The value of (

∗

Δσ )

is an analogue to fatigue limit (Δσ

) of the

material for smooth macrospecimens (without concentrator). That is why (by ana-

logue to Δσ

) it can be stated that for amplitude of cyclic loading

∗

Δσ <(

∗

Δσ )

the initiation of the macrocrack near the stress concentrator will not occur and the

fatigue of the material will be not realized.

Ostash O.P. et al [35, 36] established the dependence between the characteris-

tics of macrospecimens fatigue fracture mechanics and characteristics of the mac-

rocrack propagation in the deformed body, and also between the amplitude cyclic

loading (

∗

Δσ ) and minimum value of the crack ( l

∗

), that is

І y

Δ 0,886Δ

eff

∗

=σ,

()

theff R

∗

=η Δ Δσ (η ~ 1,25), (11)

where

І IIeff op

KKKΔ=Δ−Δ,

Iop

KΔ is SIF at which the crack opens; η is the

numerical factor close to unit;

Δσ is the fatigue limit of the material on smooth

standard specimens.

Using these correlations, the diagram (

eff

vK≈ ) can be changed by the dia-

gram (

∗

Δσ ∼ N

) or vice versa. This procedure is shown in Fig. 10. It consists of

such operations.

Let us consider a certain point on the (v ∼ K

Іeff

) diagram. For example, this is a

point

A on the diagram 3 (Fig. 10b). For this point abscissa

Ieff

KΔ is connected

with values

∗

Δ (amplitude of cyclic loading near the stress concentrator or crack

tip) by formula (11). Using this formula one can calculate the value

∗

Δ and fix it

as an ordinate of the point

A on the diagram 1 (Fig. 10а). Further consider the

macrocrack growth to be step-wise (as it is shown in [35]). In means that each time

the macrocrack in the cyclically deformed body grows by a step

∗

. So, the average

macrocrack growth rate on diagram 3 (Fig. 10b) is evaluated by formula

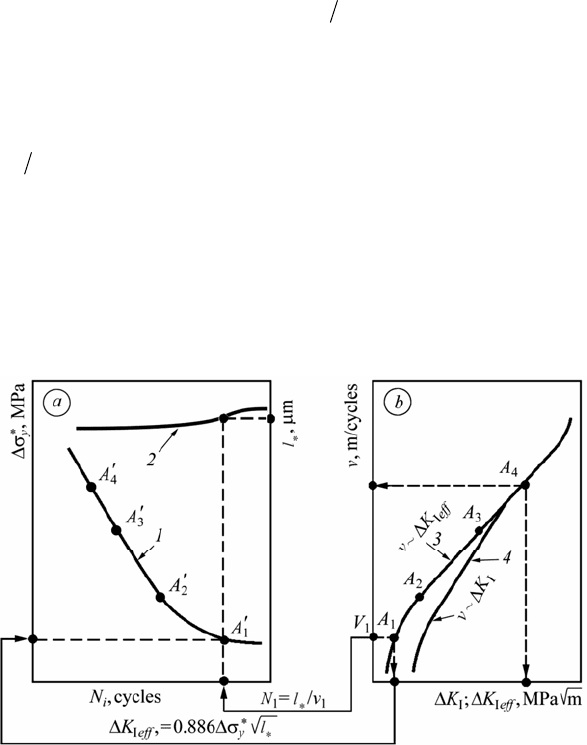
426 V. Panasyuk and I. Dmytrakh

vlN

∗

= , (12)

where

N is the period of the macrocrack l

∗

formation, this period depends on

the loading amplitude (

∗

Δ or

Ieff

KΔ ).

Thus, for the point

A on the diagram 3 (Fig. 10b) we have the value

Nlv

∗

= . Let us fix this value as abscissa of the point

A on the diagram 1

(Fig. 10а). Continue this procedure for points

,...AA of the diagram 1 (Fig.

10b) and receive corresponding points

,...AA of the diagram 1 (Fig. 10а). In

such a way we receive the diagram for the determination of the period (

N ) of the

minimum macrocrack initiation for the given material near the stress concentrator.

Diagram 2 in Fig. 10а is constructed by formula (11).

Fig. 10. A scheme of construction of the initiation (N

) of the minimum length (l

) macro-

crack when the amplitude (

∗

Δ ) of cyclic loading of the body near the stress concentrator

on the bases of v ∼ K

Іeff

diagram is known

Construction of diagrams 1 and 2 for the evaluation of period (

N ) of the l

∗

length crack initiation (Fig. 10a) on the basis of the diagram (v ∼ ∆K

Іeff

) for the

evaluation of the structural elements durability becomes very important for engi-

neering practice. Tables 1 and 2 illustrate the application of this approach.

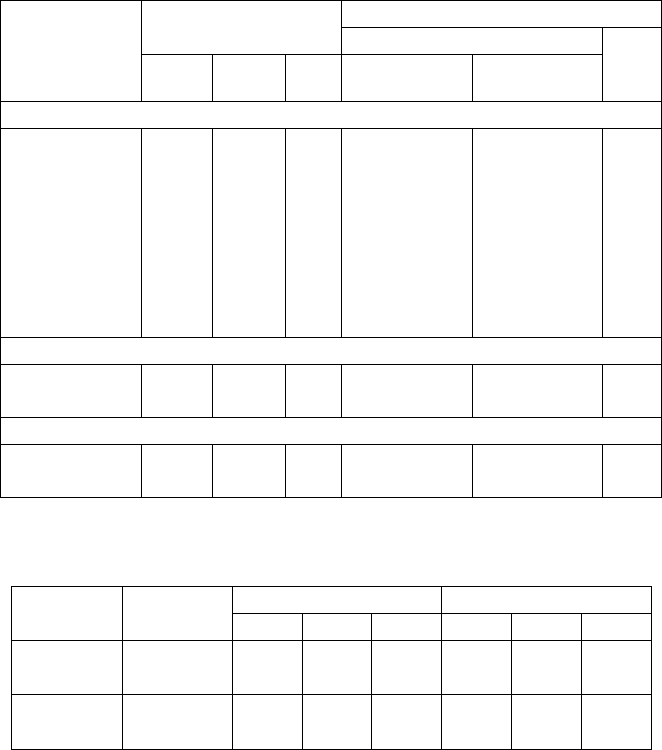
Some Actual Problems of Fracture Mechanics of Materials and Structures 427

Table 1. Experimental and calculated values of the process zone [30, 36, 37]

Value l

, μm

Characteristics

Experiment

Material

, MPa σ

, MPa δ, %

Low-cycle fatigue

= 10

÷ 10

)

High-cycle fatigue

= 10

÷ 10

)

Formula

(11)

Aluminium alloy

Д16T 350 460 15 100 100 135

Д16чAT1 442 475 10 40 180 159

Д16чATН 417 533 17 60 80 100

Д16очT 330 454 22 160 200 237

В95pchT2 456 510 12 100 100 180

В95рчТ3 432 498 14 80 80 78

1420T1 282 431 19 110 110 97

1201T1 340 442 10 130 130 154

Steels

08кп 190 270 48 200 250 208

35ХС2Н3МФ 1700 1950 10 5 10 7

Cast irons

ВЧ50 310 510 14 200 200 158

ВЧ90 850 980 3 50 150 208

Table 2. Experimental and calculation characteristics N

, N

for specimens-bands with a

central hole [36]

Experiment, cycles ⋅10

–3

Calculation, cycles ⋅10

–3

Hole diameter,

Loading range

ΔP, kN

Ni Np N* Ni Np N*

9.0 135 15 150 132.3 10.3 142.6

3.2

12.6 25.2 6 32.2 17.2 4.5 21.7

9.0 118 5 123 100.9 6.6 107.5

5.0

13.5 15.3 3.3 18.6 10.1 2.2 12.3

Thus the proposed concept of the evaluation for structural elements durability

under long-term cyclic loading has a certain experimental confirmation and can be

recommended for engineering practice to determine their life time. Theoretical

and experimental investigations should be continued in order to develop the most

effective and simple procedures for the evaluation of the structural elements dura-

bility in the given service conditions.

7 Interaction between Environments and Deformed Metal

In the mid 50’s of the 20

century H.V. Karpenko was the first [37] who discovered

the phenomenon of the steels fatigue limit decrease (approximately by 10–15%) in

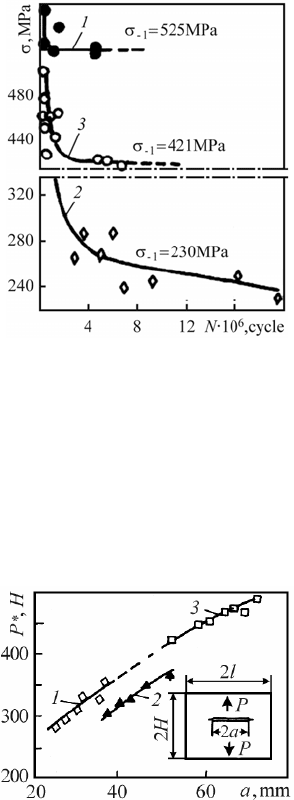
428 V. Panasyuk and I. Dmytrakh

surface-active environments (Fig. 11). However, tests on the static tension of steel

cylindrical specimens 10 mm in diameter in the same surface-active environments

showed no influence on the changes of static strength of the steel. This contradiction

was explained by the short-time action of the environment and, as a result, it was

considered that the adsorption effect was not apparent. Such interpretation of the ad-

sorption effect cannot be convincing.

Fig. 11. Fatigue curves under the circular tension of d = 7,62 mm in diameter cylindrical

specimen (40 Х steel, structure – sorbite) under different test conditions: 1 – air; 2 – water;

3 – machine (liquid paraffin) oil, activated by 0.3% of oleic acid [37]

Within the frames of the deformed bodies fracture mechanics concept the fol-

lowing experiment was made [38] taking into account that the fatigue fracture oc-

curs by the crack formation and propagation. A plate with the concentrated forces

P was tensioned as it is shown in Fig. 12.

Fig. 12. Critical loading P = P

dependence on the crack length under the tension of glass

plates in dry air (1, 3) and water (3)