Kounadis A.N., Gdoutos E.E. (Eds.) Recent Advances in Mechanics

Подождите немного. Документ загружается.

Reinforcement of a Cracked Infinite Elastic Plate with Defects 399

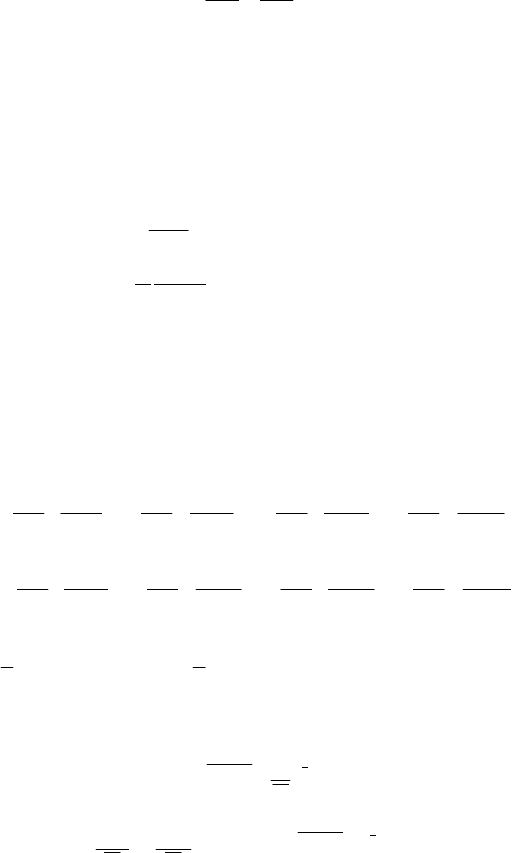
c) On boundary

L between the plate and the straight stringer we have the

following combination of boundary conditions:

ntnt

str

uiu uiutL

du du

dx dx

σσ

+−

++−−

+−

⎧

⎪

+=+ ∈

⎨

⎪

⎩

(3)

where

tde xe

=+,

d is the distance from the center of the system until the

middle of

L ,

is the corner between the two centers and

is the corner

between the x-axis and the direction of

L .

d) On the boundary

L between the plate and the curvilinear stringer we will

have the following relations:

220

()

()0

1()

()0

()

ntnt

str

uiu uiu

σσ

θε

+−

++−−

⎧

−+−=

⎪

+−=

∈

⎨

⎪

+=+

⎪

=Ε

⎩

(4)

where

tde t

=+ and

= .

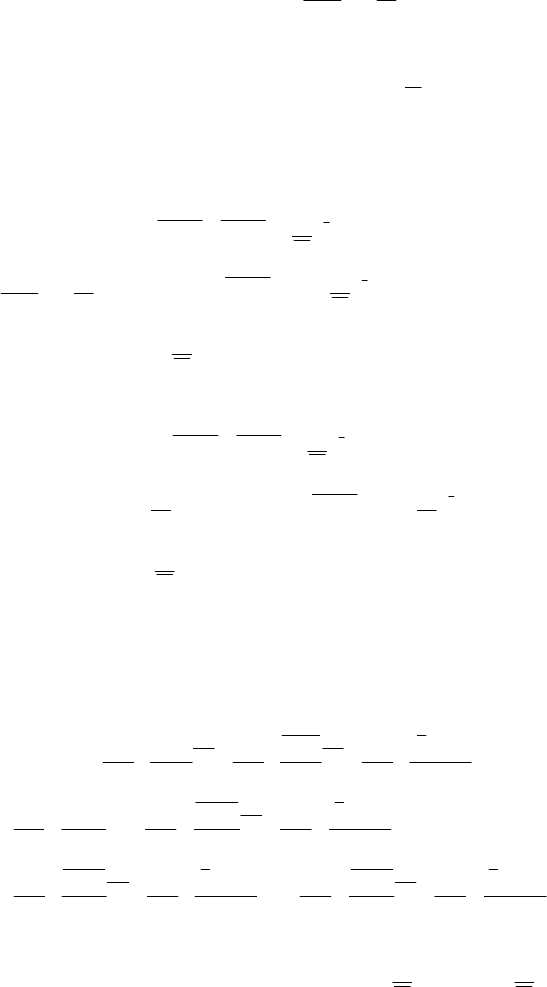
In order to deduce the state equations we will use the following complex

potentials:

12 1 2

() () () ()

11 1 1

()

22 2 2

zdddd

iz iz iz i z

φτ φτ μτ μτ

ττττ

πτ πτ πτ π τ

Φ=Γ+ + + +

−− − −

∫∫ ∫ ∫



(5)

12 1 2

121 2

() () () ()

11 1 1

() '

22 2 2

yyGG

zdddd

iz iz iz i z

ττττ

τττ τ

πτ πτ πτ π τ

Ψ=Γ+ + + +

−−−−

∫∫∫∫



(6)

where

()

Γ= Ν +Ν and

'( )

Γ=− Ν−Ν .

Based on the boundary values of the complex potential the boundary conditions

(1) and (2) we take the following form:

101000

: ( ) () '() ()

tittttt

σσ

±±± ± ± ±

⎡

⎤

∈−=Φ+Φ+Φ+Ψ

⎣

⎦

 (7)

20000

: 2 () () '() ()

du d

tittttt

dt dt

μκ

±±

±± ±±

⎡⎤

⎡

⎤

∈ − + =Φ−Φ+Φ +Ψ

⎢⎥

⎣

⎦

⎣⎦

 (8)

400 S.M. Mkhitaryan and D.I. Bardzokas

The combination of relations (3) and (4) will yield the next relations:

()() ()

:(1)0

nt nt nt n

tL ih i i ie v

Edt

σσ σσ σσ σ

++ +− ++ +

⎡⎤⎡⎤

∈−−−+ +−+=

⎣⎦⎣⎦

(9)

()()

()

2222

:1(1)0

nt nt ns n

t L ERh i i E S t d e v

σσ σσ σσ σ

+− +− ++ +

⎡⎤

⎡⎤ ⎡⎤

∈−−−−−−×+−+=

⎢⎥

⎣⎦ ⎣⎦

⎣⎦

(10)

Boundary conditions (9) and (10) based on the boundary values of the complex

potential will take the following form:

()

{

() () () () ' () '() () ()

(1 ) () () Re '() ()

2(1 ) (1 ) Re ' ,

ih t t t t t t t t t

ddt

ie v t t t t t

Edt

vv tL

+− +− + − +−

++ ++

⎡

⎤

⎡⎤

Φ−Φ +Φ−Φ + Φ −Φ +Ψ−Ψ +

⎣⎦

⎣

⎦

⎡⎤

+−Φ+Φ−Φ+Ψ+

⎢⎥

⎣⎦

⎫

⎛⎞

+−Γ−− Γ ∈

⎬

⎜⎟

⎝⎠

⎭

(11)

()

{

22 2

() () () () () () () ()

1 (1 ) () () Re '() ()

2(1 ) (1 ) Re ' ,

ERhtt tt ttt tt

ES t de v t t t t t

dt dt

vv tL

+− +− +− +−

++ ++

⎡

⎤

Φ−Φ+Φ−Φ+ Φ−Φ++Ψ−Ψ−

⎢⎦

⎣

⎛⎞ ⎡ ⎤

⎡⎤

−−− −Φ+Φ− Φ+Ψ+

⎜⎟

⎢⎥

⎣⎦

⎝⎠ ⎣ ⎦

⎫

⎛⎞

+−Γ−− Γ ∈

⎬

⎜⎟

⎝⎠

⎭

(12)

By subtraction of the reciprocal relations in (7) and (8), and taking into account

relations (3) and (4) as well as the Sohotsky – Plemely formulas for the boundary

values of the complex potentials relation (6) will take the following form:

111

22 2

11 2

11 1

12 2

1112

() () ()

111

() '

222

()

() () ()

22 2()

() () () ()

22 22() ()

LL L

zddd

iz iz i

dd d

ziziz

ddd

iz i iz izz

τφτ τφτ

ττ τ

πτ πτ π

τϕτ τϕτ

ττ τ

πι τ π τ π τ

μτ τμτ μτ τμτ

κκ

τττ

πτ π πτ πττ

Ψ=Γ+ − − +

−−

−

++ − +

−− −

+− +−

−−−−

∫∫∫

∫∫ ∫



 

∫

(13)

where

()()

()

nn t t

qt i

σσ σσ

+− +−

=−− −,

()()

() 2

gt u u i

dt dt

μυυ

+− +−

⎡

⎤

=− −+ −

⎢

⎥

⎣

⎦

Reinforcement of a Cracked Infinite Elastic Plate with Defects 401

Taking into consideration the reciprocal Sohotsky – Plemely formulas for the

complex potentials (5) and (13) in relations (7

)+(7

), (8

)+(8

), (11), (12) we will

get a system of four singular integral equations with respect to densities

12 1 2

(), (), (), ()ttt t

φφ μμ

. The system must be augmented with the condition of

uniqueness of displacements for the crack

 . The above system fully describes

the stress – strain field of the cracked plate that is reinforced by stringers.

3 Reinforcement of a Plate with a Patch

Let us consider the case when we put a circular patch

S to reinforce a crack that

exists in an infinite and isotropic plate S . The infinite plate is loaded at infinity

with prime stresses

,ΝΝ, the stresses ()

σσ

±±

− or displacements ( )ui

±±

on the crack lips are considered known. On the boundary

between the plate S

and the patch

S the following boundary relations for the unknown deformations

and stresses hold true:

()( ),

() () ()

nt nt

tS tS

ui u i t

dt dt

iift

γγ

υυγ

σσ σσ

∈∈ ∈∈

⎧

+= + ∈

⎪

⎨

⎪

+=−+=

⎩

(14)

The elastic constants of the two bodies S and

S will be ,

and

reciprocally. For the description of the stress – strain field of the composite body

we will need two pair of complex potentials.

For the plate:

1() 1 ()

()

22(1)

1() () 1 ()

() '

2 2 (1 ) 2 (1 ) ( )

zd d

iz z

yf f

zd d d

iz z z

γγ

φτ

ττ

πτ π κ τ

τκτ ττ

ττ τ

πτ π κ τ π κ τ

⎧

Φ=Γ+ +

⎪

−+−

⎪

⎨

⎪

Ψ=Γ+ − +

⎪

−+−+−

⎩

∫∫

∫∫∫







(15)

for the circular patch

()

dzS

πτ

⎧

∈

⎪

−

Φ=

⎨

⎪

∉

⎩

⎧

∈

⎪

−

Ψ=

⎨

⎪

∉

⎩

∫



(16)

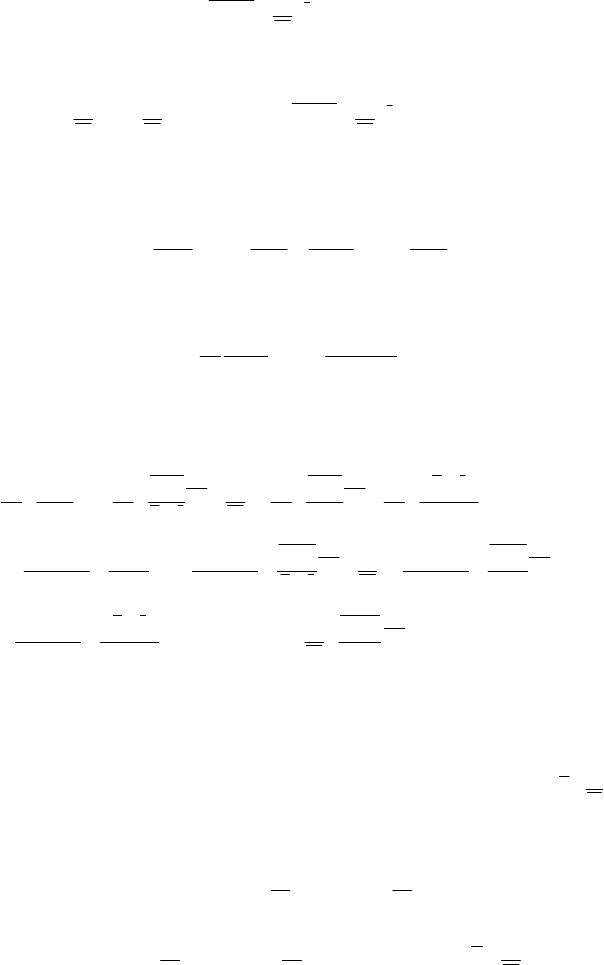
402 S.M. Mkhitaryan and D.I. Bardzokas

The boundary conditions on the crack lips will be written as follows, if we

consider the complex potential (15):

() () '() () ,

itttttt

σσ

±±± ± ± ±

⎡⎤

− =Φ+Φ+ Φ +Ψ ∈

⎣⎦

 (17)

2 () () '() () ,

dd dt

ui t t t t t t

dt dt dt

μυκ

±±± ± ±±

⎡⎤

−+ =Φ−Φ+Φ+Ψ ∈

⎢⎥

⎣⎦



Based on conditions (14) and the expressions for the stresses and the

displacements through the complex potentials (15) and (16):

() ()

ft ft

tt Gt

μμμμ

∈Φ+= − (18)

() () ()

1(1)

Gt t f t

μμμ

μκ μκ

=Φ+

(19)

Following the know process we analyzed in the previous section we will get two

integral equations on

 and the boundary

of the patch

S .

1() () () 1

()

1() () ()

(1 ) (1 ) (1 )

()

() ()

(1 ) ( )

dt t

dd d d

it i it i t

tdt

ffdtf

dd d

tdt

fd qt d

γγ γ

φτ λ φτ λ φτ τ

ττ τ φττ

πτ π πτ π τ

τλτ κτ

ττ τ

πκτ πκ πκτ

ττ

πκ ττ

⎡

⎤

−

+−−+ +

⎢

⎥

−−−

−

⎣

⎦

⎡

++−−+

⎢

+− + +−

−

⎣

⎢

⎣

⎤

−

+=−

⎥

+−−

⎥

⎦

∫∫ ∫∫

∫∫ ∫

∫

  

 



, t

∈

∫



(20)

where

a) when the stresses on the crack lips are given

()() ()()

1, ( ) , ( ) 2 '

nn t t nn t t

qt i qt i

λ σσσσ σσσσ

+− +− +− +−

⎛⎞

=− =−− − =+− +−Γ+Γ+Γ

⎜⎟

⎝⎠

b) when the displacements on the crack lips are given

()()

,()2 ,

qt u u i

dt dt

λκ μ υ υ

+− +−

⎡

⎤

==−−+−

⎢

⎥

⎣

⎦

()()

() 2 2 '

dd dt

qt u u i

dt dt

μυυκ

+− +−

⎡⎤⎛⎞

=− ++ + −Γ−Γ+Γ

⎜⎟

⎢⎥

⎣⎦⎝⎠

Reinforcement of a Cracked Infinite Elastic Plate with Defects 403

The integral equation on the boundary

has the following form,

∈

()

1() 1() 1() 1 1()

() ()

GGzdtG t dtf

dd d Gdft d

it i it i it

tdt dt

γγ γγ γ

τττ τ

ττ τ ττ τ

πτ π πτ π πτ

⎤

−

⎡

−− + =−+

⎥

⎢

−− −

−

⎣

−

⎥

⎦

∫∫ ∫∫ ∫  

(21)

also relation (19) can be written as an integral equation

1 1

11()1()

() (),

12 2 (1)

ddftGtt

it i t

μμμ

κφττ

ττ

μκ π τ π τ μκ

⎡⎤

Γ+ + + = ∈

⎢⎥

+−−+

⎢⎥

⎣⎦

∫∫





(22)

The system of equations (20) – (22) augmented with the condition of uniqueness

of displacements on the crack, when the stresses are given, can fully describe the

stress – strain field of the composite cracked plate.

4 Linear Crack of Finite Size, the Lips of Which Are

Connected with Elastic Springs

Let us consider, in the framework of generalized plain strain an infinite isotropic

and elastic plate of height h, and elastic constants

,vΕ . We will use the Cartesian

system of coordinates Oxy to describe the plate. On Ox-axis a mathematical crack

exists in the space [

a,a− ]. In this section, we will examine two problems. In the

first problem the lips of the crack in the extreme points

),(),( abba ∪−−

, where

ab <

are connected between them with equally dispersed linear elastic springs

with stiffness kΕ , while on the rest part of the crack

()

,bb− act perpendicular

tensions, the intensity of which is

)(xp

(the signs refer to each of the crack lips).

The plate is subjected to an equally dispersed and perpendicular to the crack

tension

at infinity. In the second problem, the springs and the loading are

switched between each other.

Using the known processes, we transfer the tension

from infinity on the

crack lips. This will be achieved by drawing an imaginary incision of the plate in

the direction of Ox-axis thus separating it in to two half-planes symbolized as

± .

For the first problem, under the assumption that the springs behave linearly we

will have:

(), | |

() (),

(), | |

px x b

x k xbxa

xxa

σσυ

±±

=±

−− <



=−Σ = − Ε < <

−>

(23)

0, | |

()

(), | |

xxa

=±

⎧

=− =

⎨

−>

⎩

(24)

404 S.M. Mkhitaryan and D.I. Bardzokas

For the second problem we will have

(), | |

() (), | |

(), | |

k xxb

xpxbxa

xxa

συ

σσ

±±

=±

−Ε <



=−Σ = − − < <

−>

(25)

while the boundary condition

=±

will be the same as (24)

In the above

(, )

and (, )

are the perpendicular and shear stresses with

respect to the height h of the plate, ( )x

and ( )x

with the opposite sign the

perpendicular and shear stresses outside of the crack and along the Ox-axis. Also

()x

are the components of the perpendicular displacements of the boundary

points of the upper and lower half-plate reciprocally. We notice that the shear

stresses

()

στ

=− outside of the crack and on its direction appear due to the

existence of perpendicular loading ( )px

that acts on the crack.

In order to deduce the state equations of the above boundary problems we will

express the two components of the displacements

(), ()ux x

±±

of the boundary

points of the upper and lower half-planes as a functions if the perpendicular and

shear loadings (23) – (25), which will take the form

x−∞ < < +∞ :

21 1

() ln () ( ) ()

|| 2

u x T s ds sign x s s ds C

Exs E

∞∞

±± ±

−∞ −∞

−

=± − − Σ +

−

∫∫

(26)

21 1

() ln () ( ) ()

|| 2

x s ds sign x s s ds C

xs E

∞∞

±± ±

−∞ −∞

−

=± Σ + − Τ +

Ε−

∫∫

(27)

Consequently, we introduce the following two functions of the displacement

jumps on the crack lips:

(), | |

() () ()

0, | |

xxa

xxx

υυ

+−

⎧

−=Φ=

⎨

⎩

(28)

(), | |

() () ()

0, | |

xxa

ux ux x

+−

⎧

−=Ψ=

⎨

⎩

(29)

Based on the methods described in works [2,9,10] and relations (26) – (29) for the

first problem we will have the next singular integral state equations:

(), | |

1'()

(), | |1

ξξρ

χη

σλχξ ρξ

πηξ

−

−− <

⎧

⎨

−+ <<

−

⎩

∫

(30)

(1) (1) 0

χχ

−= = (31)

Reinforcement of a Cracked Infinite Elastic Plate with Defects 405

000

'( ) ( ) ( )

2,||1

dv dv d

ρρ

ψη υη η

ηλ η ηξ

ηξ ηξ ηξ

−

−− −

⎛⎞

=+ − <

⎜⎟

−−−

⎝⎠

∫∫∫∫

(32)

(1) (1) 0

ψψ

−= = (33)

000

111

() ln () ln ()

|| 2 ||

||1

dqd

ρρ

υη η η η

πξηπξη

ρξ

−

−−

⎛⎞

++ =

⎜⎟

−−

⎝⎠

∫∫ ∫

(34)

In the above equations are used some dimensionless quantities and coordinates

,,,2,4/

xsb

ξηρλασσ

ααα

==== =Ε

()

00 0

1/4,()4()/,()4(,)/vv

σξ σαξ τξ ταξ

=− = Ε = Ε

0000

() ( )/, () 4 ( )/ , () () ()

aap pa p p p

χξ ϕ ξ ξ ξ ξ ξ ξ

±+−

⎡

⎤

==Ε=+

⎣

⎦

[]

0000 0

() () (), () ( )/, () ( )/ ( ) ( )/qpp aa aaa aa

ξξξψξψξυξυξυξυξ

+−

=− = = = +

Equations (30) and (32) that enable us to calculate the unknown normalized

densities of the dislocations of the displacement components on the crack lips should

be examined in combination with conditions (31) and (33) reciprocally. Those

relations express the continuity of the displacement components in the crack lips.

It is obvious that the combination of (30)-(31) can be separated from relations

(32)-(33), which means that those systems can be examined undependably.

System (30)-(31) contains the basic information of the first problem, while for the

second problem equation (30) will become:

(), | |

1 '()

(), | |1

σλ

χ ξξρ

χη

σξρξ

π η ξ

−

−+ <



−

(35)

when (31) as well as the rest of the equations (32)-(34) while remain almost the

same, with a just some simple modifications.

We have to note here that for

the system (30)-(31), as well as (35) are

transformed in the known integro-differential equation Prandtl [1]. In the case that

and

, the above equations will result to the known equation for the

crack [2,9,10].

After the solving the system (30)-(34) the perpendicular and shear dimensionless

stresses outside the crack, on the direction of Ox-axis are given by:

1'()

() , | |1d

χη

σξ η ξ

πηξ

−

=− >

−

∫

(36)

000 0 0

'( ) ( ) 2 ( )

() ,| |1

vq v v

dd d

ρρ

ψη η λ η

τξ η η η ξ

πηξ πηξ π ηξ

−− −

⎛⎞

=− − + + >

⎜⎟

−− −

⎝⎠

∫∫ ∫∫

(37)

406 S.M. Mkhitaryan and D.I. Bardzokas

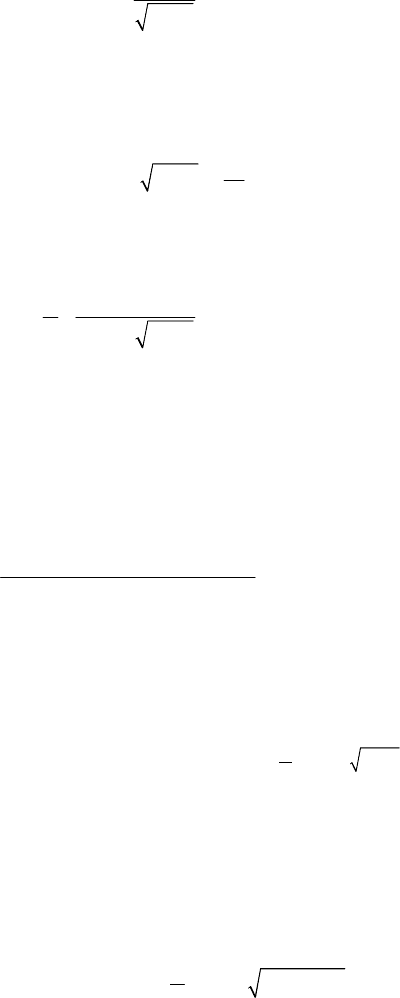
To solve the system (30)-(31) we will use an infinite series:

'( ) ( ), | | 1

χξ ξ ξ

∞

=Τ<

−

∑

(38)

with unknown factors ( 0,1,2,...)

xn= where ( )

Τ is the Chebysev polynomial

of the first type. By integration of (38) on

()

− and taking into consideration

relation (31), we note that

0x = and ( )

χξ

become:

() 1 (), | |1

χξ ξ ξ ξ

∞

−

=− − ≤

∑

(39)

where

()

−

is the Chebyshev polynomial of the second type. Consequently, we

note that equation (30) based on (38), (39) and the known relation:

()

(), | |1, 1,2,...

dU n

ηξξ

ηξ η

−

=<=

−−

∫

can be reduced to an infinite system of algebraic equations, with respect to the

unknown constants ( 0,1,2,...)

xn= :

, ( 1,2,...)

nknnk

xxak

∞

+Κ= =

∑

(40)

where:

()

() ()

()

2222

22 22

21 (1)

1sin sin cos

(1) (1)

1 sin cos sin 1 cos sin sin

2 1 cos cos cos , 1, 1

0, 1, 1, , 1, 2, ...

nn k k n

nn k n k

kk n k k n k n

kn k n nk nk

nk nk kn

ααα

ααα ααα

ααα

⎧

⎡⎤

+−

⎣⎦

⎡

⎪

−−−⋅⋅+

⎣

⎡⎤⎡⎤

−− −+

⎪

⎣⎦⎣⎦

⎪

Κ=

⎨+−− ⋅ ⋅−+− ⋅ ⋅ +

⎪

+ − ⋅ ⋅ ≠− ≠+

⎤⎪

⎦

⎪

=− =+ =

⎪

⎩

(1) (2) (1)

1, 1

0, 2,3,...

kkkk

aaaa

⎧

=− − =

⎨

⎩

(2) 2

()1 () , arccos

ap Ud

ξξ ξξ

−

=− =

∫

In the same way, we can deduce a similar infinite and linear algebraic system of

equations for the second problem. The non-singularity and the uniqueness of the

solution of the system (40) is proven in [1], so the issue of solving this problem is

fully covered.

For the stress intensity factors (SIF) on the edges of the crack we will have,

based on (38) and known expressions from [5,6]:

()

lim 2 '( )

πα ϕ

→±

⎡

⎤

Κ=

⎣

⎦

∓

∓∓

Reinforcement of a Cracked Infinite Elastic Plate with Defects 407

If we introduce the dimensionless quantities of the SIFs and putting

, we

will have:

()

lim 2 1 '( )

ξχξ

→±

⎡

⎤

Κ=

⎣

⎦

∓

∓∓

±±

Κ=Κ Ε

and taking into consideration expression (38) we have:

,(1)

aan

ππ

∞∞

−

Κ=− Κ = −

∑∑

(41)

Based on (37) with a similar process we will get expressions for the SIFs that are

analogous to (41).

With the help of mathematical tools of the Chebyshev polynomials, the system

of equations (32)-(33) can be also reduced to an infinite system of linear

equations, while equation (34) can be transformed to a system of second type

Fredholm integral equations with logarithmic kernels, and on that system method

[12] can be applied. On equations (30)-(31) and (32)-(33) we can use the known

arithmetic-analytic method of solving singular integral equations that is presented

in [7,8].

5 Linear Crack of Finite Size, with Transverse Loading, the

Lips of Which Are Connected with Thin Inclusions

As before, let us consider an infinite elastic plate with the same geometrical and

elastic characteristics. Using a Cartesian system of coordinates Oxy, we will have

a crack

()

,aa− on Ox-axis. On the edges of the crack

()()

,,ab ba−− ∪ where

ba< , the crack is reinforced with thin inclusions that are described by Winker’s

model when in shear loading. On the rest part

()

,bb− , and on the upper and lower

part of the crack act equally distributed shear loadings with intensity ( )

reciprocally. We also assume that there is a equally distributed load at infinity, the

intensity of which is

q .

We divide the plate in two half-planes by making an imaginary cut along the

Ox-axis and, as we did on part 3, we will transfer the loading from infinity on the

crack lips, taking into consideration the linear relation between shear stresses and

horizontal displacements that the Winkers model suggests. We will have the next

boundary conditions:

(), | |

() (), | |

(), | |

qxxb

Tx qGkux b x a

xxa

±±

=±

−− <

⎧

⎪

=− = − ± < <

⎨

⎪

−>

⎩

(42)

408 S.M. Mkhitaryan and D.I. Bardzokas

0, | |

()

(), | |

xxa

=±

⎧

=−Σ =

⎨

−>

⎩

(43)

here Gk is the coating coefficient of the Winkers’ model, expressed through the

shear modulus

2(1 )GE v=+ of the plate. Begging form (42)-(43) and following

the process of part 4 we can deduce the necessary state equations. If we apply the

arithmetical method we described before on them, we will get in an infinite linear

algebraic system of equations, the solution of which gives us the stress-strain field

of the composite cracked plate.

6 Reinforcement of the Crack Lips with Stringers and Elastic

Springs

Let us again consider the case when a linear crack

()

,aa− exists on Ox-axis. On

the center part of the crack

()

,bb− the crack lips are connected with a thin elastic

stringer, parts

()()

,,cb bc−− ∪ are free of stresses, while on the edges of the crack

()()

,,ac ca−−∪ the crack lips are connected to each other via elastic springs of

kΕ -stifness. It is

0 bca<<<. We also consider that at infinity and along Oy-axis

there is an equally distributed tension

, when on Ox-axis pressure p is applied.

The stringer will be described by the one-dimensional elastic continuous

medium [1,6,13]. On the edges of the stringer

xb=± tensile concentrated and

horizontal forces

are applied. The elasticity modulus of the stringer is

Ε and

the height of its orthogonal cross section is

()

δδ

<< , the width is d and as a

special case we have dh= . From the assumptions above, the axial deformation of

the stringer will be expressed as in [1]:

()

2(), , 2

xx s

Qdsds bxb d

ετ δ

−

⎡⎤

=+ −≤≤Α=

⎢⎥

⎣⎦

∫

(44)

where ( )x

is the distribution function of the unknown shear contact stresses on

the crack lips at the part that the stringer exists. Those stresses are the same due to

symmetry with respect to Ox-axis.

The equilibrium equation of the stringer has the form:

2()

dsdsQQ

+−

−

=−

∫

(45)

If we take into consideration the contact condition of the stringer and the plate we

have [1,6]: