Klyatskin V.I. Lectures on Dynamics of Stochastic Systems

Подождите немного. Документ загружается.

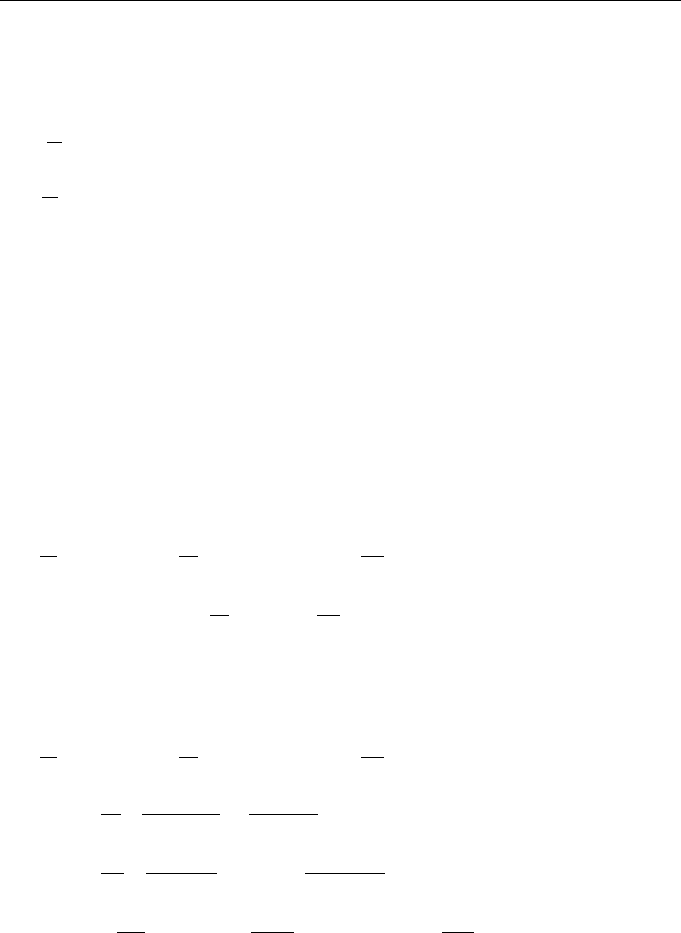
246 Lectures on Dynamics of Stochastic Systems

Here, we consider in greater detail the method that is most often used in the statistical

analysis.

Assume the stochastic system is described by the dynamic equations

x(t) = A(x,

φ) + z(t)B(x,

φ),

φ(t) = C(x,

φ) + z(t)D(x,

φ),

(9.55)

where

φ(t) = ω

t + φ(t),

functions A(x,

φ), B(x,

φ), C(x,

φ), and D(x,

φ) are the periodic functions of variable

φ, and z(t) is the Gaussian delta-correlated process with the parameters

z(t)

= 0,



z(t)z(t

)



= 2Dδ(t − t

), D = σ

Variables x(t) and φ(t) can mean the vector module and phase, respectively. The

Fokker–Planck equation corresponding to system of equations (9.55) has the form

∂

∂t

P(x, φ, t) = −

∂

∂x

A(x,

φ)P(x, φ, t) −

∂

∂φ

C(x,

φ)P(x, φ, t)

+ D



∂

∂x

B(x,

φ) +

∂

∂φ

D(x,

φ)



P(x, φ, t). (9.56)

Commonly, Eq. (9.56) is very complicated for immediately analyzing the joint

probability density. We rewrite this equation in the form

∂

∂t

P(x, φ, t) = −

∂

∂x

A(x,

φ)P(x, φ, t) −

∂

∂φ

C(x,

φ)P(x, φ, t)

− D

∂

∂x



∂B

(x,

φ)

2∂x

∂B(x,

φ)

∂

D(x,

φ)



P(x, φ, t)

− D

∂

∂φ



∂D(x,

φ)

∂x

B(x,

φ) +

∂D

(x,

φ)

2∂

)



P(x, φ, t)

+ D



∂

∂x

(x,

φ) + 2

∂

∂x∂φ

B(x,

φ)D(x,

φ) +

∂

∂φ

(x,

φ)



P(x, φ, t).

(9.57)

Now, we assume that functions A(x,

φ) and C(x,

φ) are sufﬁciently small and ﬂuc-

tuation intensity of process z(t) is also small. In this case, statistical characteristics of

system of equations (9.55) only slightly vary during times ∼ 1/ω

. To study these

small variations (accumulated effects), we can average Eq. (9.57) over the period of

Methods for Solving and Analyzing the Fokker–Planck Equation 247

all oscillating functions. Assuming that function P(x, φ, t) remains intact under ave-

raging, we obtain the equation

∂

∂t

P(x, φ, t) = −

∂

∂x

A(x,

φ) P(x, φ, t) −

∂

∂φ

C(x,

φ) P(x, φ, t)

− D

(

∂

∂x

∂B

(x,

φ)

2∂x

∂B(x,

φ)

∂

D(x,

φ)

∂

∂φ

∂D(x,

φ)

∂x

B(x,

φ)

)

P(x, φ, t)

+ D



∂

∂x

(x,

φ) + 2

∂

∂x∂φ

B(x,

φ)D(x,

φ) +

∂

∂φ

(x,

φ)



P(x, φ, t), (9.58)

where the overbar denotes quantities averaged over the oscillation period.

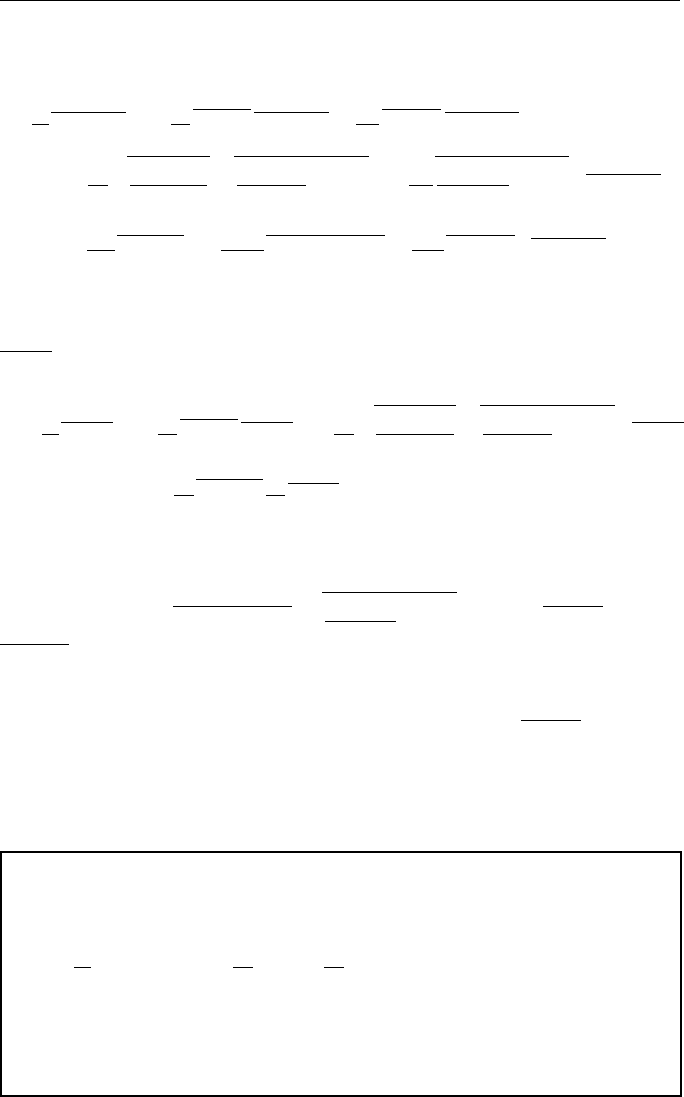
Integrating Eq. (9.58) over φ, we obtain the Fokker–Planck equation for function

P(x, t)

∂

∂t

P(x, t) = −

∂

∂x

A(x,

φ) P(x, t) + D

∂

∂x

∂B

(x,

φ)

2∂x

∂B(x,

φ)

∂

D(x,

φ)

P(x, t)

+ D

∂

∂x

(x,

φ)

∂

∂x

P(x, t). (9.59)

Note that quantity x(t) appears to be the one-dimensional Markovian random pro-

cess in this approximation.

If we assume that B(x,

φ)D(x,

φ) =

∂D(x,

φ)

∂x

B(x,

φ) = 0, and C(x,

φ) = const,

(x,

φ) = const in Eq. (9.58), then processes x(t) and φ(t) become statistically inde-

pendent, and process φ(t) becomes the Markovian Gaussian process whose variance

is the linear increasing function of time t. This means that probability distribution of

quantity φ(t) on segment [0, 2π] becomes uniform for large t (at C(x,

φ) = 0).

Problems

Problem 9.1

Solve the Fokker–Planck equation

∂

∂t

p(x, t|x

, t

) = D

∂

∂x

− 1)

∂

∂x

p(x, t|x

, t

) (x ≥ 1),

p(x, t

, t

) = δ(x − x

(9.60)

Instruction Use the integral Meler–Fock transform.

248 Lectures on Dynamics of Stochastic Systems

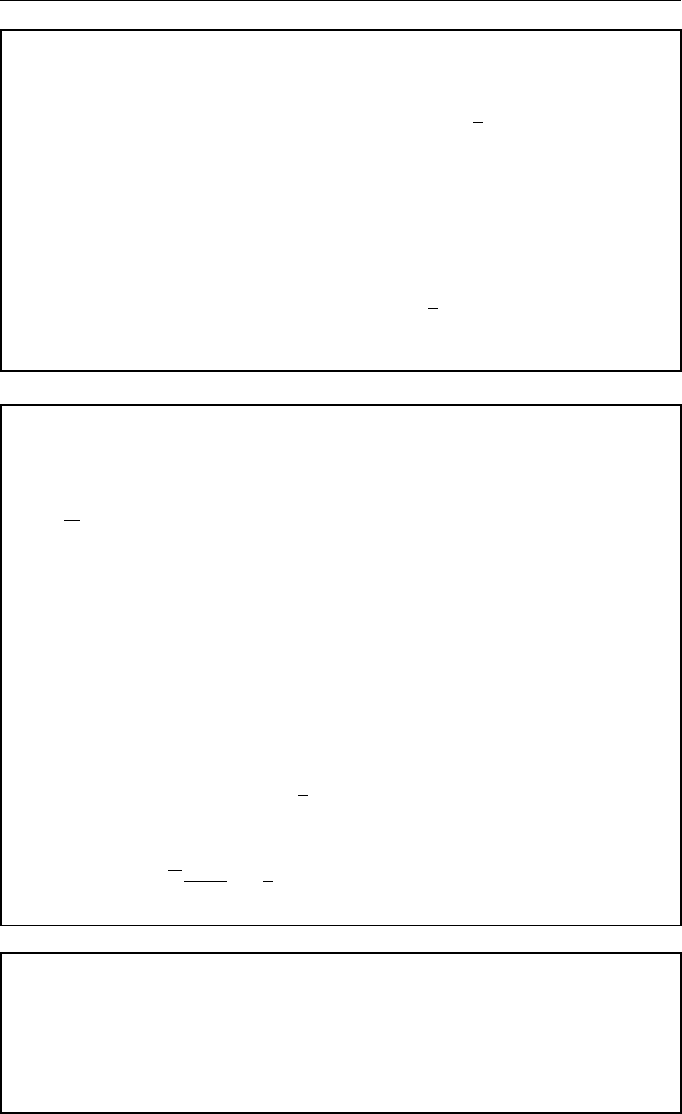
Solution

p(x, t|x

, t

) =

∞

dµ µ tanh(πµ) exp



−D





(t − t

)



−1/2+iµ

(x)P

−1/2+iµ

If x

= 1 at the initial instant t

= 0, then

P(x, t) =

∞

dµ µ tanh(πµ) exp



−D





(t − t

)



−1/2+iµ

(x).

Problem 9.2

In the context of singular stochastic problem (1.29), page 18, for λ = 1

x(t) = −x

(t) + f (t), x(0) = x

, (9.61)

where f (t) is the Gaussian delta-correlated process with the parameters

f (t)

= 0,



f (t)f (t

)



= 2Dδ(t − t

) (D = σ

estimate average time required for the system to switch from state x

to state

(−∞) and average time between two singularities.

Solution

T(x

)

−∞

dξ

∞

dη exp





− η





T(∞)

√

1/6





≈ 4.976.

Problem 9.3

Find the steady-state probability distribution for stochastic problem (9.61).

Instruction Solve the Fokker–Planck equation under the condition that function

x(t) is discontinuous and deﬁned for all times t in such a way that its value of

Methods for Solving and Analyzing the Fokker–Planck Equation 249

−∞ at instant t → t

− 0 is immediately followed by its value of ∞ at instant

t → t

+0. This behavior corresponds to the boundary condition of the Fokker–

Planck equation formulated as continuity of probability ﬂux density.

Solution

P(x) = J

−∞

dξ exp





− x





, (9.62)

where

J =

T(∞)

is the steady-state probability ﬂux density.

Remark From Eq. (9.62) follows the asymptotic formula

P(x) ≈

T(∞)

(9.63)

for great x.

Problem 9.4

Show that asymptotic formula (9.63) is formed by discontinuities of function x(t).

Instruction Near discontinuous points t

, represent the solution x(t) in the form

x(t) =

t − t

Problem 9.5

Derive the Fokker–Planck equation for slowly varying amplitude and phase of

the solution to the problem

x(t) = y(t),

y(t) = −2γ y(t) − ω

[1 + z(t)]x(t), (9.64)

where z(t) is the Gaussian process with the parameters

z(t)

= 0,



z(t)z(t

)



= 2σ

δ(t − t

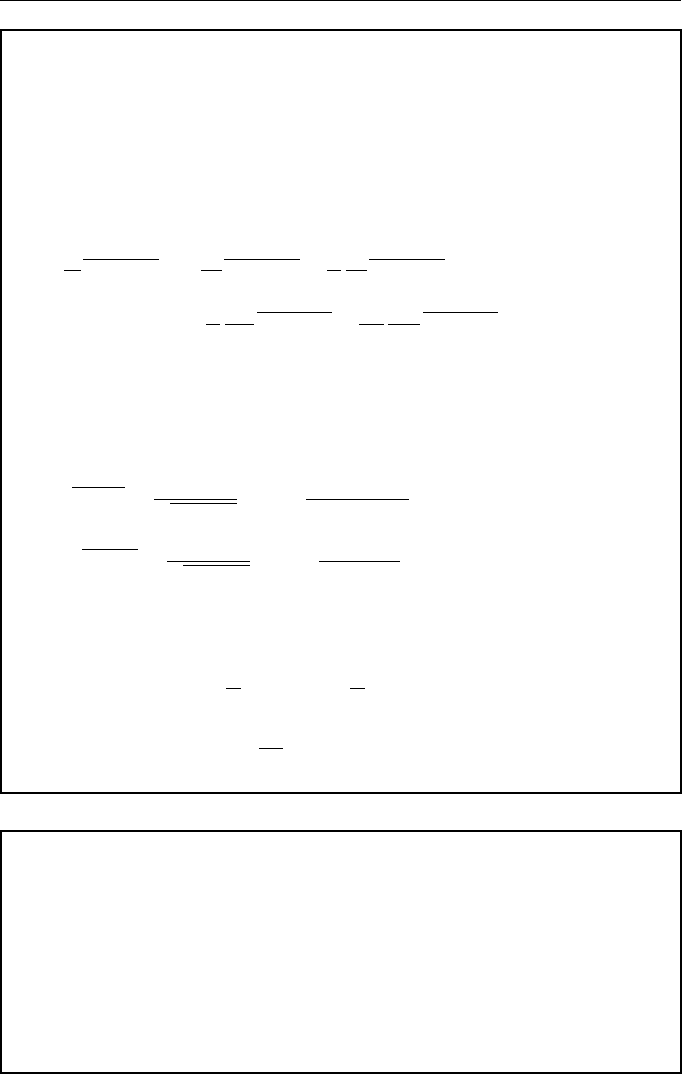
250 Lectures on Dynamics of Stochastic Systems

Instruction Replace functions x(t) and y(t) with new variables (oscillation ampli-

tude and phase) according to the equalities

x(t) = A(t) sin

(

t + φ(t)

)

, y(t) = ω

A(t) cos

(

t + φ(t)

)

and represent amplitude A(t) in the form A(t) = e

u(t)

Solution

∂

∂t

P(u, φ, t) = γ

∂

∂u

P(u, φ, t) −

∂

∂u

P(u, φ, t)

∂

∂u

P(t;u, φ) +

∂

∂φ

P(u, φ, t), (9.65)

where D = σ

. From Eq. (9.65) follows that statistical characteristics of

oscillation amplitude and phase (averaged over the oscillation period) are statis-

tically independent and have the Gaussian probability densities

P(u, t) =

2πσ

(t)

exp

(

−

(

u −

u(t)

)

2σ

(t)

)

P(φ, t) =

2πσ

(t)

exp

(

−

(

φ − φ

)

2σ

(t)

)

(9.66)

where

u(t)

= u

− γ t +

t, σ

(t) =

φ(t)

= φ

, σ

(t) =

Problem 9.6

Using probability distributions (9.66), calculate quantities

x(t)

and



(t)



Derive the condition of stochastic excitation of the second moment.

Solution Average value

x(t)

= e

−γ t

sin(ω

coincides with the problem solution in the absence of ﬂuctuations.

Methods for Solving and Analyzing the Fokker–Planck Equation 251

Quantity

(t)

(D−2γ )t

1 − e

−3Dt/2

cos(2ω

, (9.67)

coincides in the absence of absorption with Eq. (6.91) to terms about D/ω

 1.

Problem 9.7

Find the condition of stochastic parametric excitation of function

(t)

Solution We have



(t)



nu(t)

= A

exp



−nγ t +

n(n + 2)Dt



and, consequently, the stochastic dynamic system is parametrically excited

beginning from moment function of order n if the condition

8γ < (n + 2)D

is satisﬁed.

Remark The typical realization curve of random amplitude has the form

∗

(t) = A

exp



−



γ −





and exponentially decays in time if absorption is sufﬁciently small, namely, if

1 < 4

< 1 +

which always holds for sufﬁciently great n, whereas all moment functions of

random amplitude A(t) of order n and higher exponentially grow in time. This

means that statistics of random amplitude A(t) are formed mainly by large spikes

over the exponentially decaying typical realization curve. This fact follows from

the lognormal property of random amplitude A(t).

This page intentionally left blank

Lecture 10

Some Other Approximate Approaches to

the Problems of Statistical

Hydrodynamics

In previous Lectures, we analyzed statistics of solutions to the nonlinear equations of

hydrodynamics using the rigorous approach based on deriving and investigating the

exact variational differential equations for characteristic functionals of nonlinear ran-

dom ﬁelds. However, this approach encounters severe difﬁculties caused by the lack

of development of the theory of variational differential equations. For this reason,

many researchers prefer to proceed from more habitual partial differential equations

for different moment functions of ﬁelds of interest. The nonlinearity of the input dyna-

mic equations governing random ﬁelds results in the appearance of higher moment

functions of ﬁelds of interest in the equations governing any moment function. As

a result, even determination of average ﬁeld or correlation functions requires, in the

strict sense, solving an inﬁnite system of linked equations.

Thus, the main problem of this approach consists in cutting the mentioned system of

equations on the basis of one or another physical hypothesis. The most known example

of such a hypothesis is the Millionshchikov hypothesis according to which the higher

moment functions of even orders are expressed in terms of the lower ones by the laws

of the Gaussian statistics. The disadvantage of such approaches consists in the fact

that the validity of the hypothesis usually cannot be proved; moreover, cutting the

system of equations may often yield physically contradictory results, namely, energy

spectra of turbulence can appear negative for certain wave numbers. Nevertheless,

these approximate approaches provide a deeper insight into physical mechanisms of

forming the statistics of strongly nonlinear random ﬁelds and make it possible to derive

quantitative expressions for ﬁelds’ correlation functions and spectra. It seems that the

Millionshchikov hypothesis provides correct spectra of the developed turbulence in

viscous interval [35].

We emphasize additionally that the mentioned approximate equations reveal many

nontrivial effects inherent in nonlinear random ﬁelds and have no analogs in the beha-

vior of deterministic ﬁelds and waves. Here, we illustrate these methods of analy-

sis by the example of an interesting physical effect, namely, that average ﬂows of

Lectures on Dynamics of Stochastic Systems. DOI: 10.1016/B978-0-12-384966-3.00010-6

254 Lectures on Dynamics of Stochastic Systems

noncompressible liquids superimposed on the background of developed turbulent

pulsations acquire quasi-elastic wave properties. This effect was ﬁrst mentioned by

Moffatt [57] who studied the reaction of turbulence on variations of the transverse

gradient of average velocity. Moffatt noted that turbulent medium behaves in some

sense like an elastic medium; namely, variations of average velocity proﬁle of a plane-

parallel ﬂow satisfy the wave equation.

10.1 Quasi-Elastic Properties of Isotropic and Stationary

Noncompressible Turbulent Media

Let u

(r, t) be the random velocity ﬁeld of developed turbulence stationary in time

and homogeneous and isotropic in space (we assume that



(r, t)



= 0). In actuality,

regular average ﬂows are imposed on turbulent pulsations of liquid. We denote U(r, t)

the velocity ﬁeld of these regular ﬂows. Because the turbulent pulsations and average

ﬂows interact nonlinearly, we can represent the total velocity ﬁeld in the form

u(r, t) = U(r, t) + u

(r, t) + u

(r, t), (10.1)

Here, u

(r, t) is the unperturbed turbulent ﬁeld in the absence of average ﬂows and

(r, t) is the turbulent ﬁeld disturbance caused by the interaction with the regular ﬂow

(we assume that



(r, t)



= 0).

Investigation of nonlinear interactions between regular ﬂows and turbulent pulsa-

tions (and the analysis of turbulent pulsations u

(r, t) by themselves, though) is very

difﬁcult in the general case. However, in the case of weak average ﬂows, i.e., under

the condition that

(r, t)  2T(t),

where T(t) =



(r, t)



is the average density of turbulent energy pulsation, we

can discuss the effect of turbulence on the average ﬂow evolution in sufﬁcient detail by

considering the linear approximation in small disturbances of ﬁelds U(r, t) and u

(r, t)

under the assumption that the spectrum of turbulent pulsations u

(r, t) is known.

The total velocity ﬁeld (10.1) and its turbulent component u

(r, t) satisfy the

Navier–Stokes equation (1.95), page 43. Hence, substituting Eq. (10.1) in Eq. (1.95)

and linearizing the equations in regular (average) ﬁeld U(r, t) and ﬂuctuation compo-

nent u

(r, t), we arrive at the approximate system of equations

∂

∂t

(r, t) +

∂

∂r

(r, t) = −

∂

∂r

P(r, t), (10.2)

∂

∂t

(r, t) +

∂

∂r

[

(r, t)u

(r, t) −

(r, t)u

(r, t)

]

+ u

(r, t)

∂U

(r, t)

∂r

+ U

(r, t)

∂u

(r, t)

∂r

= −

∂

∂r

(r, t), (10.3)

Some Other Approximate Approaches to the Problems of Statistical Hydrodynamics 255

where T

(r, t) =

(r, t)u

(r, t)

is the Reynolds stress tensor and P(r, t) and p

(r, t)

are the average and perturbed turbulent pressure components, respectively. Here and

below, we neglect the effect of viscosity on dynamics and statistics of regular compo-

nent U(r, t) and perturbed ﬁeld u

(r, t). In addition, we will bear in mind that ﬁelds

U(r, t) and u

(r, t) satisfy the incompressibility condition (1.95), page 43,

div U(r, t ) = 0, div u

(r, t) = 0. (10.4)

Being combined with Eq. (1.95), page 43, for turbulent pulsations u

(r, t),

Eq. (10.3) yields the following equation for the Reynolds stress tensor T

(r, t)

∂

∂t

(r, t) +

∂

∂r

(r, t)u

(r, t)

(r, t)u

(r, t)

∂U

(r, t)

∂r

(r, t)u

(r, t)

∂U

(r, t)

∂r

+ U

(r, t)

∂



(r, t)u

(r, t)



∂r

−



(r, t)

∂

∂r

(r, t) + u

(r, t)

∂

∂r

(r, t)



. (10.5)

Here,

(r, t)u

(r, t) = u

(r, t)u

(r, t) + ··· .

Pressure pulsations p

in this equations can be expressed in terms of perturbed

velocities U(r, t) and u

(r, t),

(r, t)

= −1

−1

(r, r

)



∂

∂r



, t)u

, t) −



, t)u

, t)



+ 2

∂u

, t)

∂r

∂U

, t)

∂r

)

where 1

−1

(r, r

) is the integral operator inverse to the Laplace operator.

Equations (10.2), (10.5), and the expression for pressure p

(r, t) form the system of

equations in average ﬁeld U(r, t ) and stress tensor T

(r, t). These equations are not

closed because they depend on higher-order velocity correlators like the triple corre-

lator



(r, t)u

(r, t)



. Assuming that such correlators only slightly affect the

dynamics of perturbations and taking into account conditions (10.4) and statistical

homogeneity of ﬁeld u

(r, t), we reduce the system of equations (10.2), (10.5) to the

form (τ

(r, t) =

∂

∂r

(r, t))

∂

∂t

(r, t) + τ

(r, t) = −

∂

∂r

P(r, t), (10.6)