Keyfitz N., Caswell H. Applied Mathematical Demography

Подождите немного. Документ загружается.

9.2. The Stable Equivalent Population 215

9.2.1 Other Scalings of the Eigenvectors

Since the time of Fisher (1930) it has been customary to scale reproductive

value so that v

= 1, as was done in Section 8.8. But if w is also scaled to

sum to 1, this means that Q must be modiﬁed to

Q =

∗

. (9.2.5)

For age-classiﬁed matrix models, it can be shown that, with this scaling of

v and w, the denominator

∗

w = λ

−1

BA, (9.2.6)

where B is the ﬁnite birth rate and

A is the mean age of childbearing in

the stable population [the equivalent of κ in (8.1.2)]. Thus (9.2.5) is the

analogue of the continuous-time result (8.8.5); see also Goodman (1968).

It does not appear that the interpretation of v

∗

w in terms of birth rate

and generation time holds for general stage classiﬁcations, so in the general

case it is easier to compute Q by scaling w = 1 and v

∗

w = 1 and

sacriﬁcing v

=1.

Understanding Population

Characteristics

To understand a phenomenon we must break it down into simple elements

and then put these elements together again in such a way as to reconstruct

the phenomenon. This was the method Descartes proposed for study of

the physical world, and it can be used to make intelligible the population

characteristics presented as census and other data. Such characteristics as

age, sex, marital status, birthplace, occupation, and industry can be treated

by the Cartesian method, though not all with equal eﬀectiveness.

The transition from one year of age to the next against the hazard of

death, and the population rate of increase, are elements that help to ex-

plain age distribution. The transition from one school grade to the next in

the face of the hazard of dropping out has a bearing both on the grade dis-

tribution of pupils presently in the schools and on the distribution by years

of schooling completed in the population at large. The transition into the

labor force and that into retirement go some distance toward accounting

for the distribution of the population by labor force status. These transi-

tions can be incorporated into models capable of approximating the present

distribution of ages, schooling, and labor force participation.

Explanation involves many levels arranged in an inﬁnite regression. Why

the age distribution or the sex ratio is as it is, is traceable through deeper

and deeper stages, involving fetal mortality and its causes and the causes

of death in young and older people. The present argument can go only one

short step in this regression.

10.1. Accounting for Age Distribution 217

10.1 Accounting for Age Distribution

10.1.1 Young and Old Populations

In 1976, the United States was celebrating its 200th birthday; France was

older, having gained its independence from Roman colonialism about the

ﬁfth century and having become a uniﬁed nation in the seventeenth century;

Taiwan was much younger than either, having been established after World

War II. In the United States the percentage of children under 15 years of

age was 30.9, while in France it was 24.6; Taiwan had a larger proportion

of children, 45.2 percent (all 1965 ﬁgures). For these three countries and

for many others, the older the country as a political entity the smaller is

the fraction of its population under 15 years of age. Yet no one could take

seriously an assertion relating political to demographic age. The correlation

can only be called spurious since we have no reason in logic to think that

the fraction of children is related to political youth or age. Chapter 17

shows other weaknesses of a purely empirical approach to demography.

Let us here drop political age and call a country (demographically) young

if it has a large fraction of children and a small fraction of old people.

“Young” and “old” in terms of this deﬁnition will be explainable by the

life table and rate of increase. Of 800 age distributions for various countries

and times that are available for examination, that of Honduras in 1965 is

the youngest, with 50.8 percent of its population reported as under 15

years of age. The average age of Honduran males was 19.8 years; of United

States males, 30.8 years; of Swedish males, 36.1 years (again all for 1965).

Demographic youth or age can have direct and traceable consequences.

Other things being equal, if a country has many children to support, it will

be occupied in building houses and schools for them and will have fewer

resources for building factories to increase its future income. This issue will

reappear in Section 17.6.

Our ﬁrst attempt at explanation will again be Euler’s stable age distri-

bution (5.1.1), by which, under a ﬁxed regime of mortality and fertility

including the probability l(a) of surviving from birth to age a and a rate

of increase r, the population between ages a and a + da is e

−ra

l(a) da per

current birth, and as a proportion this is divided by its integral over the

range zero to ω.

A comparison of (5.1.1) with observed proportions for two age groups

and three countries appears in Table 10.1, all for females. Of the diﬀerence

in the under 15 group between France and Taiwan (45.2 − 23.6=21.6

percent) about two-thirds (40.2 − 26.5=13.7 percent) is accounted for on

the stable model. The stable model accounts for a similar fraction of the

diﬀerences in the numbers over 65.

The stable model we have constructed involves nothing but the l(a),

which depend on the present life table, and r, the rate of increase. Since it

is based only on current information on birth and death, and does not take

218 10. Understanding Population Characteristics

Table 10.1. Percentage of females under 15 and over 65 for three countries in 1965

Percentage under 15 Percentage over 65

Country Observed Stable Observed Stable

Taiwan 45.2 40.2 3.1 4.5

United States 29.8 28.4 10.4 10.6

France 23.6 26.5 15.3 12.2

account of migration or of past wars and epidemics, we should be impressed

by its capacity to explain so large a part of the diﬀerences among real age

distributions.

10.1.2 Age Distribution as a Function of Rate of Increase

We saw earlier how the fraction of any age in a stable population is ex-

pressible in terms of the rate of increase of the population and the mean

ages of the relevant subgroup. A special case, the fraction of population

65 years of age and over was treated in Section 5.7. In general terms the

fraction between any pair of ages α and β is equal to

β−α



−ra

l(a) da



−ra

l(a) da

. (10.1.1)

By a proposition cited in Section 6.2, log[



−ra

f(a) da] generates the

cumulants of the density distribution f(a), the ﬁrst cumulant being the

mean, and the second and third cumulants being moments about the mean.

We may deﬁne f(a)asl(a)/



∞

l(x) dx for the denominator, and the same

for the numerator between α and β, outside of which f(a) = 0. Normalizing

(10.1.1) by dividing by



l(a) da/



l(a) da, taking logarithms of both

sides, and expanding the two cumulant-generation functions of −r gives

log

β−α

=log



β−α



−



r −

−···

−



r −

−···



(10.1.2)

where the k’s are the cumulants of the life table distribution between α

and β, and the κ’s are the cumulants over the whole range of the age

distribution.

Hence to a ﬁrst approximation, and on taking derivatives and then

entering ﬁnite increments,

∆

β−α

≈ (κ

− k

)

β−α

∆r, (10.1.3)

10.1. Accounting for Age Distribution 219

as was established for special cases in Sections 5.3 and 5.7.

Putting α = 0 and β = 15 shows that the proportion under age 15 goes

up as r goes up, since k

, the mean age of those under 15, is bound to be

less than κ

, the mean age of the whole population. Similar considerations

apply to other age intervals. The relation can be studied as the exponential

of a quadratic or cubic wherever r is large enough to make further terms

important.

The stable model does not always ﬁt. Among 800 populations tested,

that of England and Wales, 1881, comes closest; the Netherlands, 1901, is

second. But even there agreement is not perfect. Females aged 0 to 14 in

the actual population of England and Wales, 1881, comprised 35.5 percent;

in the ﬁtted stable model, 35.0 percent. Discrepancies between the model

and the observed populations invariably demand our attention.

Since in Table 10.1 the 1965 rates of death were the source of the life

table l(a), and the 1965 intrinsic rate of increase was the source of r,one

reason why the model can be at variance with reality is that diﬀerent rates

of death and increase applied in earlier years. This indeed appears to be

the main source of discrepancy. In Taiwan birth rates have been falling;

the 1965 birth rates are too low to represent the preceding 15 years. A

calculation based on the stable model with 1959–61 birth and death rates

shows 43.6 percent under 15, a value closer to the observed one.

For intervals α to β straddling the mean age, k and κ will not be very

diﬀerent; (10.1.3) shows that diﬀerences in r do not greatly aﬀect the middle

ages. By a similar argument drastic declines in the later ages are associated

with increases in r.

10.1.3 Neutral and Nonneutral Change in Mortality

How do populations that have been subject to diﬀerent death rates diﬀer

in age? If the diﬀerence is the same amount at all ages, it has no eﬀect on

the age distribution. If birth rates are the same and mortality at all ages

has been higher by exactly 0.01 in one population than in the other, the

age distributions will be identical (Coale, 1968).

This seems to be a paradox. A rise in mortality that prevents people from

living to as old an age as they once did ought to make the population consist

to a greater degree of young people, one would think. It certainly makes

the prospect of attaining old age less than it was before for an individual;

why does it not do the same for the community? Here, as in many other

situations, the same rule does not apply to populations as to individuals.

When mortality is higher with fertility unchanged, the rate of increase is

just enough lower to compensate as far as age distribution is concerned for

the change in the life table. The canceling out is a property of exponential

growth dovetailing with the exponential eﬀect on l(x) of an addition to

µ(x).

220 10. Understanding Population Characteristics

If k (e.g., 0.01) is added to the mortality rate µ(x) at each age, so that

it becomes µ

∗

(x)=µ(x)+k, the probability of surviving to age x is

∗

(x)=e

−kx

l(x) instead of l(x).

But if the age-speciﬁc birth rates are unchanged, the rate of increase

of the population diminishes, and by exactly the same amount, k.The

proof of this statement is that the new rate of increase r

∗

must satisfy the

characteristic equation



−r

∗

(x)m(x) dx =1



−r

∗

−kx

l(x)m(x) dx =1



−(r

∗

+k)x

l(x)m(x) dx =1.

Since the original r satisﬁed the same equation that r

∗

+ k now satisﬁes,

by virtue of the uniqueness of the real root we have r = r

∗

+ k,sothat

∗

= r − k; the new rate is exactly k less than the old rate.

Combining the preceding two paragraphs gives, for the new stable

population on a radix of one current birth per year,

−r

∗

(x)=e

−(r−k)x

−kx

l(x)=e

−rx

l(x);

hence the number of persons at age x per current birth is the same under

the new regime as under the old.

Changes in mortality that have occurred historically can be distinguished

according to whether they tended to be at younger or older ages, that is,

whether or not they tended to lower the mean age of the stable population

if the birth rate remained the same. We ﬁnd that the improvements in

England and Wales from 1861 to 1891 were on balance at younger ages,

those between 1891 and 1911 were at older ages; and those from 1911 to

1931 again aﬀected younger ages to a greater degree (Keyﬁtz 1968, p. 191).

10.1.4 Accounting for Observed Ages

The ﬁrst part of the analysis of age distributions here outlined is con-

cerned with the regime of mortality and natural increase actually existing;

the second, with trends in the regime during the lifetimes of the current

population; the third, with extraordinary historical events, especially those

that cause short-term ﬂuctuations in birth and death. These events include

wars, which reduce the numbers of males in their twenties; immigration,

which usually consists of young adults; and famines, which especially af-

fect young children. In this third part of the analysis theory does not help

much; we must look at the record to see what happened.

10.1. Accounting for Age Distribution 221

These results are a special case of perturbation analysis of the right

eigenvector of the population projection operator. A general formula is

available for matrix population models (Section 13.3), but its results rarely

lend themselves to interpretation in the way the approach here does.

10.1.5 Are Birth or Death Rates the Major Inﬂuence on Age

Distribution?

The stable model, which can reconstruct an age distribution from a life

table and rate of increase, enables us to compare two populations, say

those of the United States and Madagascar, of which one is old and the

other young, and to explain the diﬀerence between them. We know that

the United States has lower birth rates as well as lower death rates; the

question is, how much of the diﬀerence in ages is due to the diﬀerence in

births, and how much to the diﬀerence in deaths?

Without the Cartesian decomposition, through the stable or some other

model, no answer can be given to such a question. The rates of birth and

death of both countries are what they are; their age distributions are what

they are. We cannot even think of an experimental treatment of real pop-

ulations that would answer the question, let alone perform one. But in a

model we can vary one factor and see how the age distribution changes, and

then vary the other and again see how the distribution changes. If the two

kinds of change add up to the total change, we have decomposed the total;

our model has paid oﬀ in providing without cost an experiment that tells

the relative eﬀects of birth and death on age when everything else remains

unchanged.

Table 10.2 provides examples of the experimental treatment. For the

stable model, with the female age-speciﬁc rates of birth and death observed

in the United States in 1967, it shows 24.5 percent under 15 years of age

(second item in second row). If we now alter the death rates to those of

Madagascar, 1966, leaving the birth rates as they were, we ﬁnd 22.0 percent

under age 15. The higher death rates of Madagascar lower the proportion

under 15 by 2.5 percentage points.

Now, retaining the death rates of the United States but entering in the

stable model the birth rates of Madagascar, we ﬁnd the proportion under

age 15 rising from the original 24.5 percent to 48.6 percent. The higher birth

rates of Madagascar raise the proportion under 15 by 48.6 − 24.5=24.1

percentage points.

The two preceding paragraphs show that birth rates have about 10 times

as much eﬀect as do death rates on the proportion of a population under

15 years of age. As a check we take the diﬀerences in the other direction,

starting with the same 45.2 percent for Madagascar births and deaths,

subtracting 48.6 to ﬁnd the eﬀect of death rates at −3.4, and subtracting

22.0 to ﬁnd the eﬀect of birth rates at 23.2, so now births are 7 times as

inﬂuential as deaths. The discrepancy is (45.2−22.0)−(48.6−24.5) = −0.9.

222 10. Understanding Population Characteristics

Table 10.2. Features of age distribution and rate of increase obtained by

combinations of female birth and death rates from ﬁve countries, stable model

Age-speciﬁc birth rates of:

United England

Age-speciﬁc Venezuela, States, Madagascar, and Wales, Sweden,

death rates of: 1965 1967 1966 1968 1803–07

Percent under 15: 100

Venezuela 47.7 23.9 47.8 23.6 34.2

United States 48.5 24.5 48.6 24.2 34.8

Madagascar 45.0 22.0 45.2 21.8 32.1

England and Wales 48.5 24.5 48.6 24.2 34.8

Sweden 43.6 21.0 43.8 20.8 31.3

Dependency ratio percent: 100(

∞

Venezuela 102.1 58.8 102.4 58.7 70.3

United States 105.4 61.1 105.6 60.9 72.5

Madagascar 91.3 51.5 91.8 51.3 62.8

England and Wales 105.2 60.3 105.5 60.1 72.1

Sweden 85.6 46.7 86.2 46.6 58.9

Percent 65 and over: 100

∞

Venezuela 2.8 13.1 2.8 13.3 7.1

United States 2.8 13.5 2.8 13.7 7.3

Madagascar 2.7 12.0 2.7 12.2 6.5

England and Wales 2.8 13.1 2.7 13.3 7.1

Sweden 2.5 10.9 2.5 11.0 5.8

Intrinsic rate of natural increase per 1000: 1000r

Venezuela 38.5 4.9 38.6 4.5 19.5

United States 40.7 7.6 40.8 6.7 21.5

Madagascar 22.3 −11.3 22.5 −11.63.8

England and Wales 41.0 7.4 41.1 7.0 21.8

Sweden 24.3 −9.4 24.6 −9.66.4

This quantity is the interaction between birth and death: it is the diﬀerence

between the eﬀect of births in the presence of Madagascar deaths and the

eﬀect of births in the presence of United States deaths. It measures the

uncertainty in the decomposition, and is small enough in this case not

to aﬀect our assertion that the age diﬀerence is due chieﬂy to the birth

diﬀerence.

This may seem obvious for the proportion under age 15, which represents

the births of the last 15 years and therefore ought to be closely related to

the birth rate. More surprising is the outcome of the same analysis for

the fraction of the population 65 years of age and older. Now we have for

United States births and deaths 13.5 percent; a drop of only 1.5 to 12.0 for

Madagascar with the change in deaths alone, and a drop of 10.7 to 2.8 with

10.2. More Women Than Men 223

the change in births alone. Again births are the main factor, accounting

for 8 times as much of the change as do deaths.

Similar calculations may be performed on Table 10.2 for the dependency

ratio (

∞

,where

is the population between exact

ages x and x+n, as well as for the intrinsic rate r. Variation along rows (due

to births with deaths held constant) is everywhere greater than variation

down columns.

One cannot but be puzzled on noting that historically the birth rate

has changed little in the countries of Asia and Africa since the time when

they had a much lower proportion of young people than they now have.

With little change over time in births, and much change in deaths, rates of

increase and age distribution have changed drastically; how is it that Table

10.2 shows births as the cause? The answer is that cross-sectional analysis

need have no relation to longitudinal (see Section 12.4). It is the fact that

people are always born at age zero, whereas they die at all ages, that gives

the birth rate more leverage on age distribution in a cross-sectional analysis

such as that of Table 10.2; the historical trend is mostly determined by the

acceleration of population increase due to falling mortality at young ages.

10.2 Why There Are More Women Than Men

at Older Ages in Modern Populations

On the whole the population of the United States contains more females

than males, but the diﬀerence is by no means uniform through the several

ages. Males are in excess to age 20, but from then on there are more females,

with large proportional diﬀerences after age 65 (Figure 10.1). Can theory,

combined with known facts of mortality, account for this diﬀerence between

male and female age distributions? Speciﬁcally, can theory tell why in 1967

there are 2,236,000 men aged 70 to 74 against 2,941,000 women, a sex ratio

of 76 males per 100 females? Why has the ratio of males to females in the

United States declined steadily during the course of the present century?

Essentially the same method of study that worked for age distribution will

help to account for the varieties of sex ratios in observed populations.

Our search, as before, will start with the current age-speciﬁc rates of

birth and death, now pertaining to the two sexes separately, from which a

stable model may be constructed. What is left unexplained by the stable

model based on current rates may be referred to trends in the rates in

recent years; what is then still unexplained can be pursued by the study of

migration and sudden changes in death rates due to wars, epidemics, and

other historical events.

To convert the one-sex model used earlier for the analysis of ages into the

(very primitive) two-sex model needed now, one item of data is required:

the sex ratio at birth, say s.Ifs boys are born for each girl, and B girls are

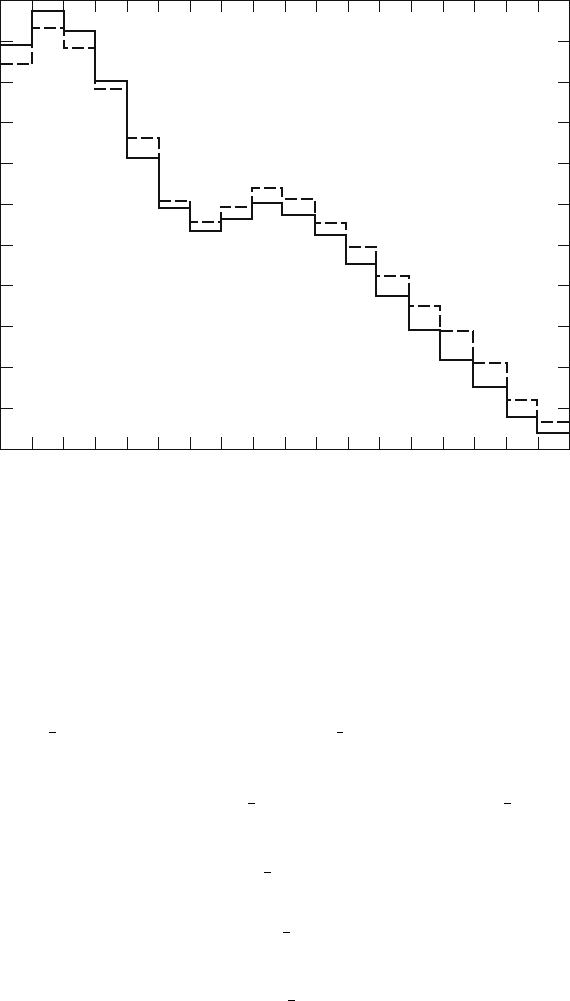
224 10. Understanding Population Characteristics

Male

Female

5 1015202530354045505560657075808590

Age

Millions of persons

Figure 10.1. Male and female populations by age, United States, 1967.

born in the current year, then the number of boys born is sB.Bythesame

argument as was used for (5.1.1) we can write down the sex–age distribution

if the regime of mortality and fertility is ﬁxed and population is increasing

in a geometrical sequence with a ratio e

of increase over any 5-year period.

Births, as well as each age–sex group, are all supposed to be increasing in

this ratio; hence, if currently there are B female births per year, on an

approximation to the stable population argument of Section 5.1 there will

be B

−2

females aged 0 to 4, B

−7

aged 5 to 9, and similarly

for later ages. We are here satisﬁed with the common approximation



x+5

−ra

l(a) da ≈ e

−r(x+2

)



x+5

l(a) da =

−r(x+2

)

The corresponding male births are sB, and male survivors at the end of

the ﬁrst 5-year period are Bs

∗

−2

, the same as for females but using

s and the male life table function

∗

. Thus the number of females is

−(2

+x)r

andofmalesis

∗

−(2

+x)r

both in the age group x to x + 4 at last birthday, where we suppose the

two sexes to be increasing at the same rate r.