Jenkins N., Strbac G., Ekanayake J. Distributed Generation

Подождите немного. Документ загружается.

Chapter 5

Integration of distributed generation in

electricity system planning

Prototype SeaGen marine current turbine, Strangford Lough, Northern Ireland

(1.2MW)

The world’s first grid-connected tidal turbine, installed and operati onal at

Strangford Lough since 2008 – capturing energy from tidal flows [RWE npower

renewables]

5.1 Introduction

For full integration of distributed generation, it is critically important to quantify its

benefits and costs associated with electricity system development. As discussed

earlier, distributed generation will displace not only the energy produced by central

generation, but also the capacity of central generation and contribute to providing

network security. In this context, this chapter describes the concepts and techniques

needed for the impacts of distributed generation on the electricity system devel-

opment to be determined, covering two key areas the:

● ability of distributed generation to displace the capacity of incumbent con-

ventional generation and contribute to generation capacity adequacy

● ability of distributed generation to substitute for distribution network capacity

and hence contribute to delivery of network security

5.2 Distributed generation and adequacy of supply

Sustainable energy systems with a significant penetration of distributed generation

may require a diverse mix of generation technologies to maintain reliability of

supply comparable to that of a conventional thermal system. Increasing the pene-

tration of distributed generation will displace energy produced from existing plant,

but it may not necessarily displace a corresponding amount of the generation

capacity that is required to maintain an appropriate level of system reliability.

The impact of a generation technology on system reliability may be described

by its capacity value or capacity credit. To calculate the capacity value of dis-

tributed generat ion technologies, the starting point is to establish the amount of

conventional capacity required to maintain a given level of reliability of supply.

Distributed generation is then introduced into the system, and an assessment is

made to determine the amount of conventional generation capacity that can be

retired to maintain the system security to its original level. This section discu sses

how the capacity value of distributed generation can be determined and how the

corresponding cost or benefits can then be attributed to distributed generation.

5.2.1 Generation capacity adequacy in conventional thermal

generation systems

In order to supply demand that varies daily and seasonally, and given that demand

is at present largely uncontrollable while interruptions are very costly, the installed

generation capacity must be able to meet the maximum (peak) demand. In addition,

there needs to be sufficient capacity available to deal with the uncertainty in gen-

erator availability and unpredicted demand increases.

The planning of generation systems involves evaluating the generation capa-

city that is required to meet future demand with a certain level of reliability. The

installed generation capacity should always be above the system maximum demand

in order to deal with the uncertainties associated with both changes in demand and

the availability of generation. Generation availability can be affected by forced

outages of thermal generation plant and/or reduced water availability of hydro

generation . The ‘excess’ of installed generation capacity above expected peak

demand is called the capacity margin. Different capacity margins will deliver dif-

ferent reliability performance of the generation system.

The amount of generation capacity in a power system is considered to be ade-

quate if it meets electricity demand with an ‘economically efficient’ level of relia-

bility. Conceptually this ‘optimal’ level of capacity to be installed would be

128 Distributed generation

determined by balancing generation invest ment costs against benefits ass ociated with

the improvements in reliability of supply, i.e. the reduction in loss of supply to con-

sumers. Instead of conducting such a cost–benefit analysis, generation system plan-

ners traditionally aim to maintain a certain level of capacity margin that will deliver a

minimum reliability performance as measured by various reliability indices.

In a centrally planned system dominated by thermal generation, one of the

frequently used indices is ‘loss of load probability’ (LOLP). This specifies the

probability (or risk) that the system peak demand will be greater than the available

generation (i.e. the probability that peak demand will not be met). The acceptable

level of LOLP would always be below a certain threshold. In the last security

standard employed in the United Kingdom, by the Central Electricity Generation

Board ahead of privatisation in 1990, the risk of peak demand exceeding the

available supply was required not to exceed 0.09, or 9% (interruptions in supply

should not occur, on average, in more than nine winters in a century). Based on the

probabilities of plant failure including uncertainty in the timely development of

generation that corresponded to an equivalent generation availability of 85%, this

standard would require a capacity margin of approximately 20%.

In determining the probability of various possible available outputs of a generator

system, a standard tw o-state generator model was generally applied to simulate the

behaviour of the generating unit. This ass umes a generator unit is fully available, with

the probability of 0.85, while also assuming that the generator unit wi ll be completely

unavailable, with the probability of 0.15. It was fur ther assumed that there is no

correlation between the availabilities of individual conventional units, i.e. the failure

of one unit does not incre ase the risk of failure of others. Based on thes e assumptions

it is poss ible to construct a generation system probability dist ribution that links the

available level of the entire generation system output with the corresp onding prob-

ability. We can also consider demand uncertainty through a probability distribution

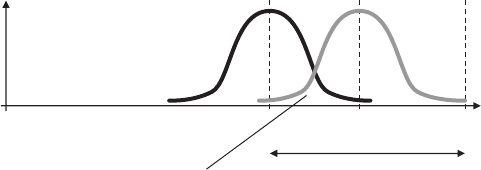
with a mean equal to the expected peak demand. This is shown in Figure 5.1.

Peak

demand

forecast

Probability

Generation

available at peak

100%

Generation

Capacity margin

Risk of insufficient

eneration

(

LOLP

)

Figure 5.1 Probability distributions of demand and available generation

Figure 5.1 illustrates the relationship between the total capacity of installed

generation and the system LOLP performance. It is clear that the higher the

Integration of distributed generation in electricity system planning 129

installed capacity of generation (higher capacity margins) the lower the LOLP (this

moves the generation availability curve to the right and LOLP reduces). The gen-

eration capacity is considered adequate if the evaluated LOLP of a system (or

future system in the planning domain) meets the threshold level.

This approach to planning and design of generation systems worked satisfac-

torily for several decades. Various reliability indices have been applied in different

systems to evaluate adequate levels of generation capacity. More common indices

include reserve margin, loss of load probability (LOLP), loss of load expectation

(LOLE), expected energy not served (EENS) while less common indices include

loss of energy probability, frequency and duration of failures, effective load car-

rying capability and firm equivalent capacity. It should be noted that the popularity

of an index is not necessarily based on it giving a more accurate assessment of

system reliability rather it was mostly due to the ease of its use and required input

or data for its assessment.

Examples of applied reliability indices:

● The North American Electricity Reliability Council (NERC) reports the use of

LOLP, LOLE and reserve margin to evaluate the adequacy of their regional

generation portfolios. Many of the regional reliability councils (regions) in

NERC’s jurisdiction apply either LOLP (1 in ten years) or LOLE (0.1 day/year

or 2.4 hour/year) [1].

● In Australia the reliability standard for generation and bulk supply are

expressed in terms of the maximum permissible unserved energy or the max-

imum allowable level of electricity at risk of not being supplied to consumers.

This is 0.002% of the annual energy consumption for the associated region or

regions per financial year [2].

● France [3] and Republic of Ireland [4] apply LOLE criteria of 3 and 8 hours/

year, respectively, to plan their generation systems.

These traditional planning approaches have been common practice for several

decades in particular for a centrally planned thermal dominated system.

5.2.2 Impact of distributed generation

The methodology described in Section 5.2.1 can be extended to determine the

adequate level of generation capacity in a system with wind, and other intermittent,

generation . We primarily focus on the impact of wind generation, but the concepts

are applicable to other forms of distributed generation. Unlike a two-state repre-

sentation of conventional thermal generators, that are either in service and available

to produce full output or not available at all (zero output), wind is modelled as a

generator with multiple levels of outputs characterised by different probabilities.

The frequency distribution of the aggregate wind generation can be obtained from

the annual half-hourly profiles of wind output from historic wind output data.

This assumes that there is no correlation between peak demand and the level of wind output (which is

generally conservative as wind farms in Europe tend to produce higher levels of output during winter

peak periods).

130 Distributed generation

The behaviour of conventional generation units and wind generation is then

combined statistically, enabling the risk of loss of load (LOLP) to be calculated for

systems that include both conventional and wind generation. This enables the

capacity credit (value) of wind generation to be evaluated. The calculation starts

with a conventional generation system that provides a reference level of LOLP.

Wind generation is then added to the system, which will improve the reliability

performance of the system (LOLP reduces below the reference value). We then

determine the amount of conventional plant that can be removed from the system

so that LOLP is equal to the reference level of the thermal only generation system.

The ratio of the capacity of the conventional plant displaced by wind power to the

total installed capacity of wind gives the capacity credit of wind.

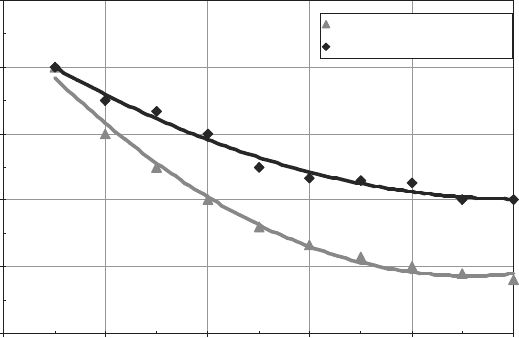
The capacity credit changes with the levels of penetration of wind. Assuming a

capacity factor of wind of 40%, Figure 5.2 illustrates the typical changes in the

capacity credit of wind for different levels of its penetration. This is expressed as

the installed capacity as a percentage of peak demand. The following observations

can be made:

● The capacity credit reduces with wind penetration level.

● The capacity credit for low penetration levels approaches the load factor of

wind.

● A diverse wind profile with wind farms occupying relatively large geo-

graphical area will exhibit higher capacity credit compared to a non-diverse

profile with wind farms being concentrated in a small geographical area.

● The capacity credit of wind is greater than zero even though there will be finite

probability of the wind output being zero during peak demand conditions.

Wind penetration level

(

% of peak demand

)

Capacity credit (% of wind installed)

Non-diverse wind source

Diverse wind source

5040302010

Figure 5.2 The capacity credit of wind generation versus the level of wind

penetration in the UK electricity system

Integration of distributed generation in electricity system planning 131

As wind generation has a relatively low capacity credit, it will displace pro-

portionately more energy produced by conventional generation than the capacity of

conventional generation. This will result in reduced utilisation of conventional

plant. More generally, the relationship between energy and capacity displaced, and

the resulting impact on average utilisation of the incumbent conventional genera-

tion, can be shown to be the primary driver for system costs associated with gen-

eration capacity:

¼ 1 

 C

ð5:1Þ

where DC

= additional system costs of distributed generation (£/MWh) that

should be added to every megawatt hour produced by distributed generation (note

that these costs could be positive and negative, depending on the actual technolo-

gies of distributed generation and the system), D

= percentage displaced capacity

of incumbent conventional technology (due to penetration of distributed generation

technology), D

= percentage displaced energy of incum bent (central) conventional

technology (due to penetration of distributed generation technology) and C

= cost

of capacity of the incumbent conventional generation expressed in pounds per

megawatt hour. This cost is simply equal to the annuitised investment capacity cost

of the incumbent conventional generation system (£/annum) divided by the total

annual energy produced (MWh/annum). For a system based on CCGT, with a

capacity of 84 GW (required to meet peak demand of 70 GW) generating 400 TWh/

annum, the cost of generation capacity wil l be 14.07 £/MWh (with the annuitised

capacity cost of CCGT of 67 £/k W/annum).

The values of these additiona l costs for wind generation of different char-

acteristics, based on the UK wind generation profiles, are shown in Table 5.1.

Table 5.1 Additional system cost of wind power technology (£/MWh)

Capacity credit (%)

Load factor (%) 0 10 20 30

20 14.07 10.24 6.42 2.60

30 14.07 11.52 8.97 6.42

40 14.07 12.16 10.25 8.33

It can be observed that increase in capacity credit significantly reduces the

additional system costs. We also see that an increase in load factor for a fixed

capacity credit increases additional cost, which is caused by the reduction in the

utilisation of incumbent central conventional generation technology. Taking an

extreme position by assuming that wind has no capacity value the additional system

cost of wind would amount to 14.07 £/MWh (this means that 14.07 £/MWh should

132 Distributed generation

be added to every megawatt hour produced by wind generation). It is important to

mention that any base load plant that is added to the system (e.g. base load CCGT

or nuclear) would also impose additional system costs. Such plant running at a load

factor higher (say 85%) than that of average primary plant (say 55%) will displace

more energy than capacity of the primary technology (similar to wind), causing a

reduction in the (average) utilisation of the primary technology. This gives rise to

an additional system cost of base load plant of about 5 £/MWh.

In contrast, micro-CHP output will typically be coincident with the winter peak

demand, as this is coincident with the time of peak heating demands. Micro CHP

will then effectively reduce the demand at the winter peak, thus reducing the

capacity of conventional generation that is required to maintain system reliability.

On the other hand the annual load factor of micro CHP will be lower than that of

the average conventional generation. Hen ce, the conventional generation capacity

displaced by micro CHP is greater than the displaced energy produced by con-

ventional plant and the additional system cost in expression (5.1) is less than zero,

indicating that micro CHP brings capacity benefits to the system.

In contrast to domestic CHP, PV generation installed in Northern Europe

would have no capacity value as it cannot displace peak generation (e.g. it is dark at

the time of GB peak demand, in the evenings of January and February). However,

PV generation in Southern Europe, for example, where peak demand is in summer

daytime, can contribute to reducing peak demand seen by conventional generation

and hence bring benefits.

5.3 Impact of distributed generation on network design

At present distributed generation is not considered in the design of distribution

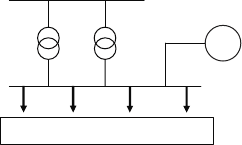
networks. A simple example is set out in Figure 5.3 that illustrates how distribution

network planners traditionally ensure security of supply of demand in a typical 33/

11 kV substation design. As can be seen, the demand of 50 MW can be supplied

through either of the distribution transformers such that if one circuit were to fail,

the demand could be accommodated through the remaining transformer. In this

example, the network planners would ignore the presence of the distributed gen-

erator and no security contribution is allocated to this resource.

Group demand 50 MW

Figure 5.3 Example of secure network design without generation contribution

Integration of distributed generation in electricity system planning 133

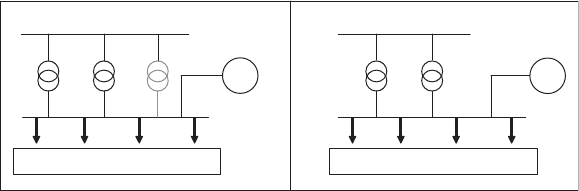
If the demand in this example were to grow to 55 MW, the supplying network

would no longer be adequate, thus requiring the distribution network planners to

seek some form of network reinforcements. Figure 5.4 illustrates two different

approaches available to the planning engineers, which would secure sufficient

additional capacity to make the network adequate. If the contribution of distributed

generation is ignored, a third transformer would need to be installed to meet the

security shortfall (Figure 5.4(A)). Alternatively, the distributed generator could

potentially substitute for network reinforcement (Figure 5.4(B)).

Group demand 55 MW

30 MW

(B)(A)

Figure 5.4 Network (A) and generation (B) solutions to a distribution network

security of supply shortfall

The lack of an established framework by which distribution network planners can

consider distributed generators for the provision of network security had been iden-

tified as a barrier to distributed generation. In the United Kingdom, the distribution

network standards have changed recently to enable network planners to incorporate

the contribution of distributed generation in network design. The concepts and tech-

niques that can be applied to quantify the ability of distributed generation to displace

network capacity and hence used in network planning are discussed in this section.

5.3.1 Philosophy of traditional distribution network planning

The UK network security standard defines the network design philosophy and the

need to comply with it is a key network cost driver [5,6]. The level of security in

distribution networks is defined in terms of the time taken to restore power supplies

following a predefined set of outages. Consistent with this concept, security levels on

distribution systems are graded according to the total amount of peak power that can

be lost. A simplified illustration of this network design philosophy is presented in

Figure 5.5. For instance small demand groups, less than 1 MW peak, are provided

with the lowest level of security, and have no redundancy (n  0 security). This

means that any fault will cause an interruption and the supply will be restored only

after the fault is repaired. It is expected that this could take up to 24 hours.

For demand groups between 1 MW and 100 MW, although a single fault may

lead to an interruption, the bulk of the lost load should be restored within 3 hours.

This requires the presence of network redundancy, as 3 hours is usually insufficient

to implement repairs, but it does allow network reconfiguration. Such network

designs are often described as providing n  1 security. For demand groups larger

134 Distributed generation

than 100 MW, the networks should be able to not only provide supply continuity to

customers following a single circuit outage (with no loss of supply), but also pro-

vide significant redundancy to enable supply restoration following a fault on

another circuit superimposed on the existing outage, i.e. n2 security.

5.3.2 Methodology for the evaluation of contribution of distributed

generation to network security

Distributed generation has challenged this conventional distribution network plan-

ning philosophy. In this section, we present concepts that have been applied in the

United Kingdom to update network design standards to include effects of distributed

generation. Given that the level of supply security provided by a network is mea-

sured through traditional reliability index such as expected energy not supplied

(EENS), we examine the ability of distributed generation to substitute for network

capacity through comparing EENS when supplied through a perfectly reliable net-

work and a generator or a group of generators. This concept is shown in Figure 5.6.

Same EENS

Hypothetical

perfect circuit

Figure 5.6 Comparing generation with a hypothetical perfect circuit.

Note: GD – group demand.

Time to restore supplies (hours)

243

n − 1

n − 2

n − 0

Redundanc

11kV

0.4 k

100

33/66 kV

132 kV

Figure 5.5 Restoration time philosophy relative to peak network demand

Integration of distributed generation in electricity system planning 135

5.3.2.1 Treatment of non-intermittent distributed generation

The basic model for assessing the reliability of generation systems, the units of

which are not constrained by intermittent energy sources and behave independently

of each other, is best represented by a capacity outage probability table (COPT).

The detailed theory relating to these is given in various reliability texts [7,8], but

can be summarised as follows.

If all units in a given case are identical and behave independently, the capacity

outage probability table can be evaluated using the binomial distribution in which

the probability P{r} of a specific state {r} is given by:

Pfr g¼

r!ðn  rÞ!

nr

ð5:2Þ

where n = number of units, r = number of available units, (n  r) = number of

unavailable units, p = availability and q = unavailability of each unit.

If all units are not identical but still behave independently, the capacity outage

probability table can be evaluated using the principle of state enumeration, i.e. if

and P

are the probabilities of state {i} and state {j} respectively, then the

probability of the combined state {ij} is given by:

¼ P

 P

ð5:3Þ

Consider the case of three identical units each having a capacity C and availability

p. Using (5.1), the resulting capacity outage probability table is shown in Table 5.2.

Table 5.2 Capacity outage probability table for three identical units

Capacity available Capacity unavailable State probability

3C 0 p

2C C 3p

(1  p)

C 2C 3p(1  p)

03C (1 p)

Total 1.0

In the case of three non-identical units having capacities C

and C

with

availabilities of p

and p

respectively, the resulting capacity outage probability

table is shown in Table 5.3.

Load is usually presented using the standard load duration curve (LDC),

schematically presented in Figure 5.7. The specified period of time T, i.e. total

horizontal axis, can be any time period of concern, e.g. one whole year, one season,

one month, etc. The time units along the horizontal axis are usually hourly values.

LDC represents the variation in load over a specified period of time in terms of the

time durat ion the demand exceeds a particular load level (the demand exceeds load

level L for t time units).

136 Distributed generation