Jacques I. Mathematics for Economics and Business
Подождите немного. Документ загружается.


Partial Differentiation
420
Practice Problems
4 Use Lagrange multipliers to maximize
z = x + 2xy
subject to the constraint
x + 2y = 5
5 A firm that manufactures speciality bicycles has a profit function
π=5x
2
− 10xy + 3y
2
+ 240x
where x denotes the number of frames and y denotes the number of wheels. Find the maximum profit
assuming that the firm does not want any spare frames or wheels left over at the end of the produc-
tion run.
6 A monopolistic producer of two goods, G1 and G2, has a total cost function
TC = 5Q
1
+ 10Q
2
where Q
1
and Q
2
denote the quantities of G1 and G2 respectively. If P
1
and P
2
denote the corres-
ponding prices then the demand equations are
P
1
= 50 − Q
1
− Q
2
P
2
= 100 − Q
1
− 4Q
2
Find the maximum profit if the firm’s total costs are fixed at $100. Estimate the new optimal profit if
total costs rise to $101.
7 Find the maximum value of
Q = 10√(KL)
subject to the cost constraint
K + 4L = 16
Estimate the change in the optimal value of Q if the cost constraint is changed to
K + 4L = 17
8 A consumer’s utility function is given by
U =αln x
1
+βln x
2
Find the values of x
1
and x
2
which maximize U subject to the budgetary constraint
P
1
x
1
+ P
2
x
2
= M
Advice
There now follow five new Practice Problems for you to attempt. If you feel that you need
even more practice then you are advised to rework the nine Practice Problems given in
Section 5.5 using Lagrange multipliers.
MFE_C05f.qxd 16/12/2005 10:43 Page 420

chapter 6
Integration
This chapter concludes the topic of calculus by considering the integration of func-
tions of one variable. It is in two sections, which should be read in the order that
they appear.
Section 6.1 introduces the idea of integration as the opposite process to that of dif-
ferentiation. It enables you to recover an expression for the total revenue function
from any given marginal revenue function, to recover the total cost function from
any marginal cost function and so on. You will no doubt be pleased to discover that
no new mathematical techniques are needed for this. All that is required is for you
to put your brain into reverse gear. Of course, driving backwards is a little harder to
master than going forwards. However, with practice you should find integration
almost as easy as differentiation.
Section 6.2 shows how integration can be used to find the area under the graph of
a function. This process is called definite integration. We can apply the technique to
supply and demand curves and so calculate producer’s and consumer’s surpluses.
Definite integration can also be used to determine capital stock and to discount a
continuous revenue stream.
MFE_C06a.qxd 16/12/2005 11:22 Page 421

MFE_C06a.qxd 16/12/2005 11:22 Page 422
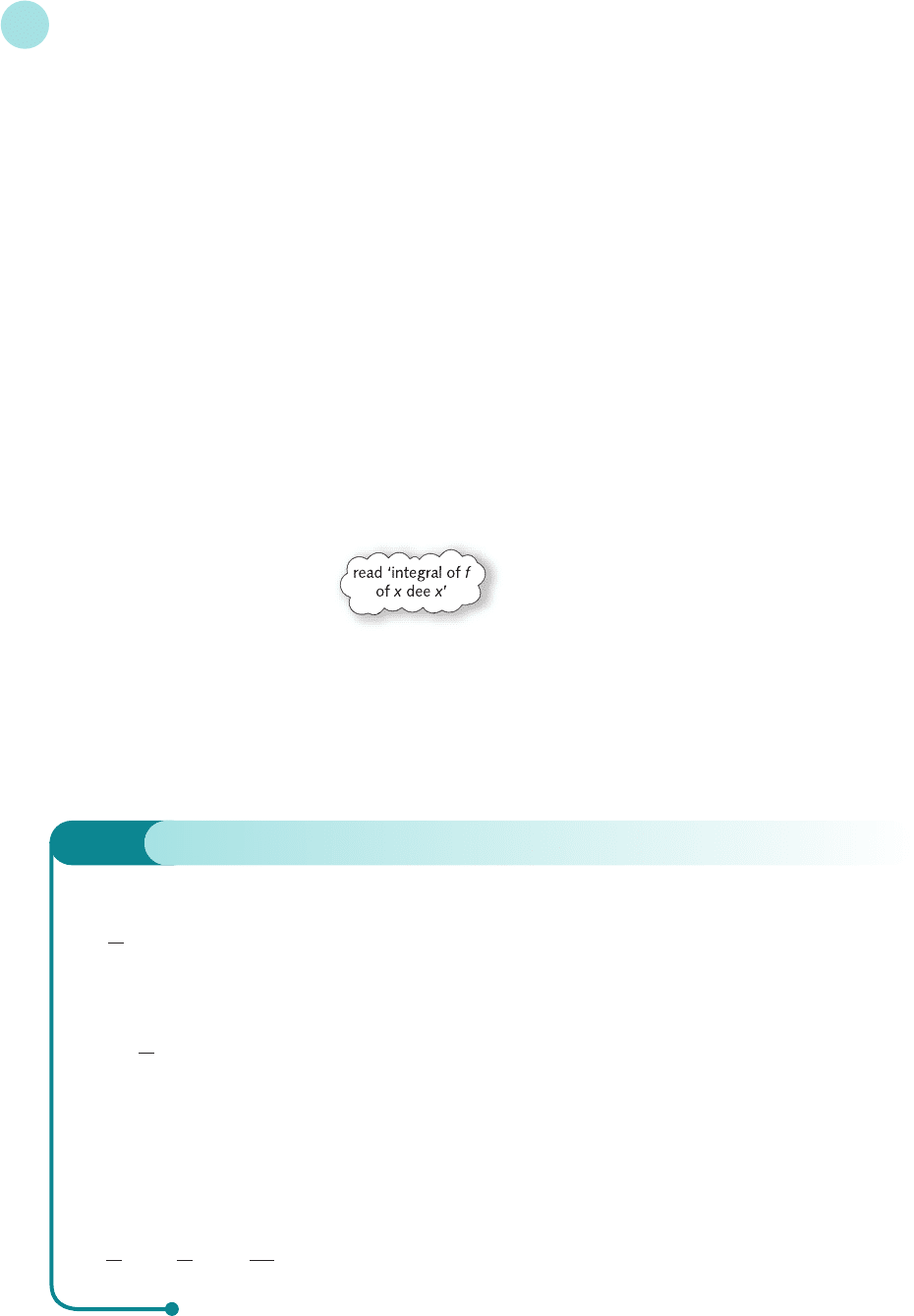
section 6.1
Indefinite integration
Throughout mathematics there are many pairs of operations which cancel each other out and
take you back to where you started. Perhaps the most obvious pair is multiplication and divi-
sion. If you multiply a number by a non-zero constant, k, and then divide by k you end up with
the number you first thought of. This situation is described by saying that the two operations
are inverses of each other. In calculus, the inverse of differentiation is called integration.
Suppose that you are required to find a function, F(x), which differentiates to
f(x) = 3x
2
Can you guess what F(x) is in this case? Given such a simple function it is straightforward to
write down the answer by inspection. It is
F(x) = x
3
because
F′(x) = 3x
2
= f (x) ✓
as required.
Objectives
At the end of this section you should be able to:
Recognize the notation for indefinite integration.
Write down the integrals of simple power and exponential functions.
Integrate functions of the form af(x) + bg(x).
Find the total cost function given any marginal cost function.
Find the total revenue function given any marginal revenue function.
Find the consumption and savings functions given either the marginal propen-
sity to consume or the marginal propensity to save.
MFE_C06a.qxd 16/12/2005 11:22 Page 423
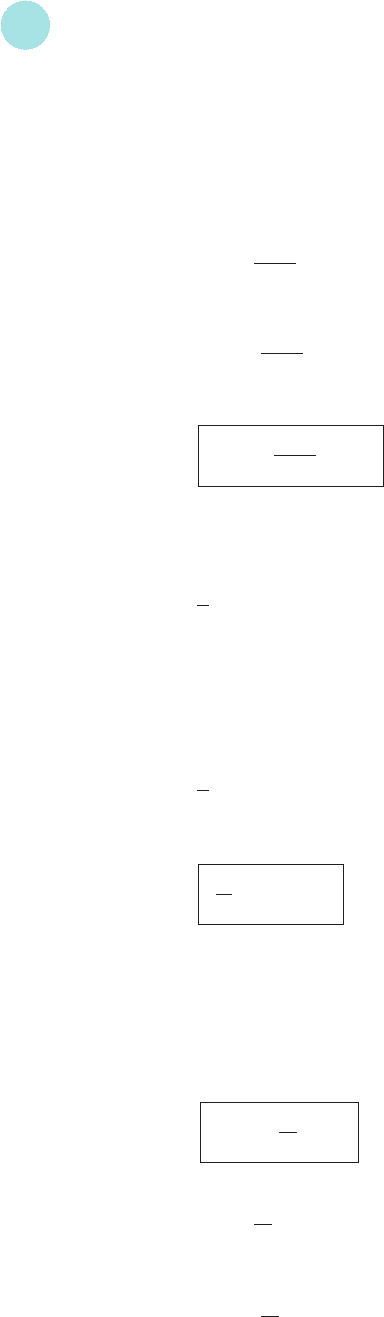
As a second example, consider
f(x) = x
7
Can you think of a function, F(x), which differentiates to this? Recall that when power
functions are differentiated the power decreases by 1, so it makes sense to do the opposite here
and to try
F(x) = x
8
Unfortunately, this does not quite work out, because it differentiates to
8x
7
which is eight times too big. This suggests that we try
F(x) =
1
/8 x
8
which does work because
F′(x) =
8
/8 x
7
= x
7
= f (x) ✓
In general, if F′(x) = f(x) then F(x) is said to be the integral (sometimes called the anti-
derivative or primitive) of f(x) and is written
F(x) =
f(x)dx
In this notation
3x
2
dx = x
3
and
x
7
dx =
1
/8 x
8
Integration
424
Example
Find
dx
Solution
Writing in the form x
− 4
suggests that we try
F(x) = x
−3
which gives
F ′(x) =−3x
− 4
.
This is (−3) times too big, so
dx =− x
− 3
=−
1
3x
3
1
3
1
x
4
1
x
4
1
x
4
MFE_C06a.qxd 16/12/2005 11:22 Page 424

Here is a problem for you to try. Do not let the notation
dx
put you off. It is merely an instruction for you to think of a function that differentiates to what-
ever is squashed between the integral sign ‘∫’ and dx. If you get stuck, try adding 1 on to the
power. Differentiate your guess and if it does not quite work out then go back and try again,
adjusting the coefficient accordingly.
6.1 • Indefinite integration
425
In Problem 1(a) you probably wrote
2xdx = x
2
However, there are other possibilities. For example, both of the functions
x
2
+ 6 and x
2
− 59
differentiate to 2x, because constants differentiate to zero. In fact, we can add any constant, c,
to x
2
to obtain a function that differentiates to 2x. Hence
2xdx = x
2
+ c
The arbitrary constant, c, is called the constant of integration. In general, if F(x) is any function
that differentiates to f(x) then so does
F(x) + c
Hence
f(x)dx = F(x) + c
In Problem 1 you used guesswork to find various integrals. In theory most integrals can be
worked out in this way. However, considerable ingenuity (and luck!) may be required when
integrating complicated functions. It is possible to develop various rules similar to those of dif-
ferentiation, which we discussed in Chapter 4, although even then we sometimes have to resort
to sheer trickery. It is not our intention to plod through each rule as we did in Chapter 4, for
the simple reason that they are rarely needed in economics. However, it is worthwhile showing
you a direct way of integrating simple functions such as
2x − 3x
2
+ 10x
3
and x − e
2x
+ 5
We begin by finding general formulae for
x
n
dx and
e
mx
dx
Practice Problem
1 Find
(a)
2xdx (b)
4x
3
dx (c)
100x
99
dx (d)
x
3
dx (e)
x
18
dx
MFE_C06a.qxd 16/12/2005 11:22 Page 425
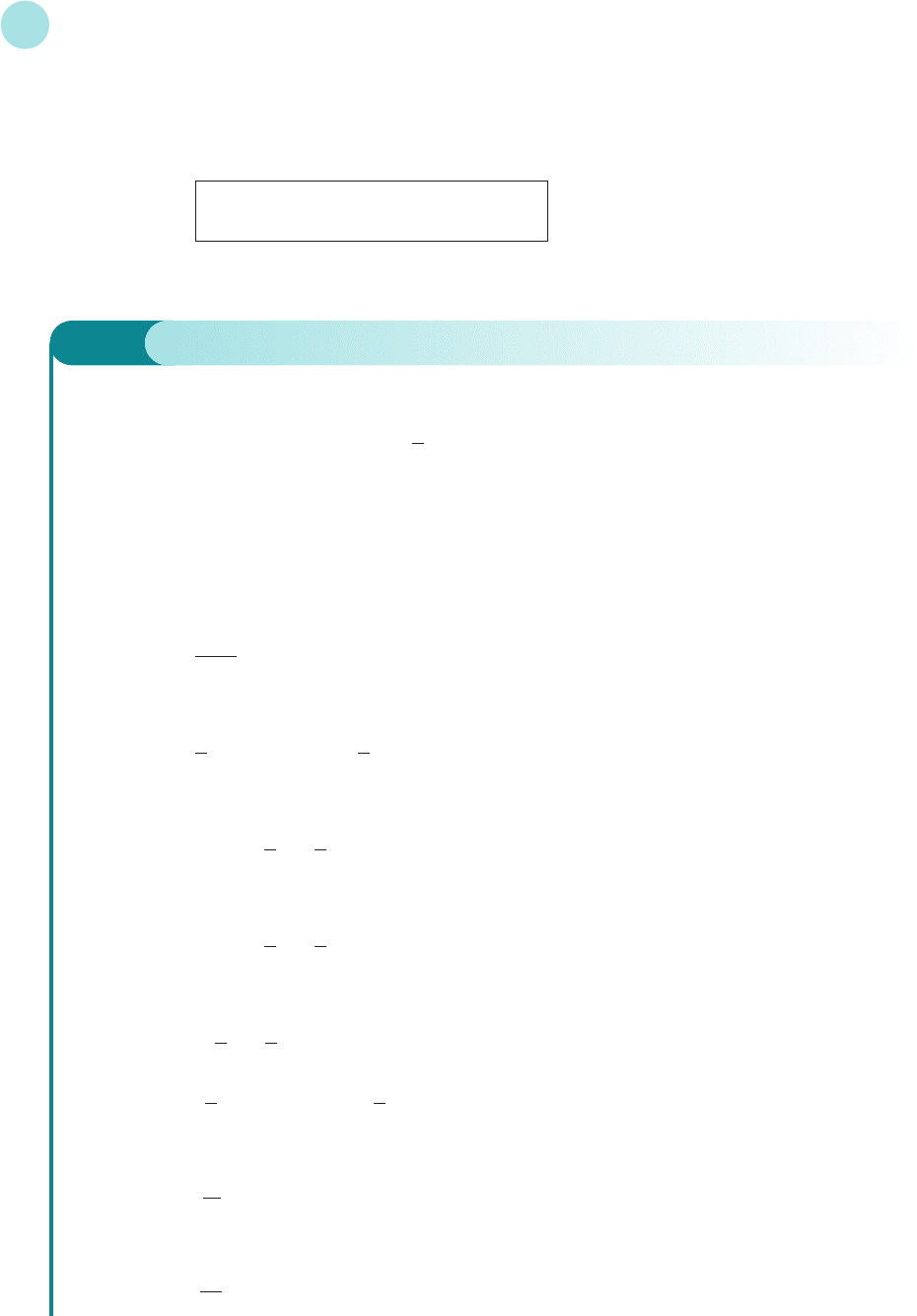
To integrate f(x) = x
n
an obvious first guess is
F(x) = x
n +1
This gives
F′(x) = (n + 1)x
n
which is n + 1 times too big. This suggests that we try again with
F(x) = x
n+1
which checks out because
F′(x) = x
n
= x
n
= f (x) ✓
Hence
x
n
dx = x
n+1
+ c
To integrate a power function you simply add 1 to the power and divide by the number you
get. This formula holds whenever n is positive, negative, a whole number or a fraction. There
is just one exception to the rule, when n =−1. The formula cannot be used to integrate
because it is impossible to divide by zero. An alternative result is therefore required in this case.
We know from Chapter 4 that the natural logarithm function
ln x
differentiates to give
and so
dx = ln x + c
The last basic integral that we wish to determine is
e
mx
dx
In Section 4.8 we showed that to differentiate an exponential function all we need to do is to
multiply by the coefficient of x. To integrate we do exactly the opposite and divide by the
coefficient of x, so
e
mx
dx = e
mx
+ c
It is easy to check that this is correct, because if
F(x) = e
mx
then
F′(x) = e
mx
= e
mx
✓
m
m
1
m
1
m
1
x
1
x
1
x
1
n + 1
n + 1
n + 1
1
n + 1
Integration
426
MFE_C06a.qxd 16/12/2005 11:22 Page 426

6.1 • Indefinite integration
427
Example
Find
(a)
x
6
dx (b)
dx (c)
x dx (d)
e
2x
dx
Solution
The formula
x
n
dx = x
n+1
+ c
can be used to find the first three integrals by substituting particular values for n.
(a) Putting n = 6 gives
x
6
dx = x
7
+ c
(b) Putting n =−2 gives
dx =
x
−2
dx = x
−1
+ c =− +c
(c) Putting n =
1
/2 gives
x dx =
x
1/ 2
dx = x
3/2
+ c = x
3/2
+ c
(d) To find
e
2x
dx
we put m = 2 into the formula
e
mx
dx = e
mx
+ c
to get
e
2x
dx = e
2x
+ c
1
2
1
m
2
3
1
3/2
1
x
1
−1
1
x
2
1
7
1
n + 1
1
x
2
Practice Problem
2 Find
(a)
x
4
dx (b)
dx (c)
x
1/3
dx (d)
e
3x
dx (e)
1dx
(f)
xdx (g)
dx
[Hint: in parts (b), (e) and (f) note that 1/x
3
= x
−3
, 1 = x
0
and x = x
1
respectively.]
1
x
1
x
3
In Section 4.2 we described three rules of differentiation known as the constant, sum and
difference rules. Given that integration is the inverse operation, these three rules also apply
MFE_C06a.qxd 16/12/2005 11:22 Page 427
whenever we integrate a function. The integral of a constant multiple of a function is obtained
by integrating the function and multiplying by the constant. The integral of the sum (or dif-
ference) of two functions is obtained by integrating the functions separately and adding (or
subtracting). These three rules can be combined into the single rule:
[af(x) + bg(x)]dx = a
f(x)dx + b
g(x)dx
This enables us to integrate an expression ‘term by term’, as the following example
demonstrates.
Integration
428
Example
Find
(a)
(2x
2
− 4x
6
)dx (b)
7e
−x
+ dx (c)
(5x
2
+ 3x + 2)dx
Solution
(a)
(2x
2
− 4x
6
)dx = 2
x
2
dx − 4
x
6
dx
Putting n = 2 and n = 6 into
x
n
dx = x
n+1
gives
x
2
dx = x
3
and
x
6
dx = x
7
Hence
(2x
2
− 4x
6
)dx = x
3
− x
7
Finally, we add an arbitrary constant to get
(2x
2
− 4x
6
)dx = x
3
− x
7
+ c
As a check:
if F(x) = x
3
− x
7
+ c then F′(x) = 2x
2
− 4x
6
✓
(b)
7e
−x
+ dx = 7
e
−x
dx + 2
dx
Now
e
mx
dx = e
mx
so putting m =−1 gives
e
−x
dx = e
−x
=−e
−x
1
−1
1
m
1
x
D
F
2
x
A
C
4
7
2
3
4
7
2
3
4
7
2
3
1
7
1
3
1
n + 1
D
F
2
x
A
C
MFE_C06a.qxd 16/12/2005 11:22 Page 428

Also, we know that the reciprocal function integrates to the natural logarithm function, so
dx = ln x
Hence
7e
−x
+ dx =−7e
−x
+ 2 ln x
Finally, we add an arbitrary constant to get
7e
−x
+ dx =−7e
−x
+ 2 ln x + c
As a check:
if F(x) =−7e
−x
+ 2 ln x + c then F ′(x) = 7e
−x
+ ✓
(c)
(5x
2
+ 3x + 2)dx = 5
x
2
dx + 3
xdx + 2
1dx
Putting n = 2, 1 and 0 into
x
n
dx = x
n+1
gives
x
2
dx = x
3
,
xdx = x
2
,
1dx = x
Hence
(5x
2
+ 3x + 2)dx = x
3
+ x
2
+ 2x
Finally, we add an arbitrary constant to get
(5x
2
+ 3x + 2)dx = x
3
+ x
2
+ 2x + c
As a check:
if F(x) = x
3
+ x
2
+ 2x + c then F′(x) = 5x
2
+ 3x + 2 ✓
3
2
5
3
3
2
5
3
3
2
5
3
1
2
1
3
1
n + 1
2
x
D
F
2
x
A
C
D
F
2
x
A
C
1
x
6.1 • Indefinite integration
429
Advice
We have written out the solution to this example in detail to show you exactly how
integration is performed. With practice you will probably find that you can just write
the answer down in a single line of working, although it is always a good idea to check (at
least in your head, if not on paper), by differentiating your answer, that you have not
made any mistakes.
The technique of integration that we have investigated produces a function of x. In the next
section a different type of integration is discussed which produces a single number as the end
result. For this reason we use the word indefinite to describe the type of integration considered
here to distinguish it from the definite integration in Section 6.2.
MFE_C06a.qxd 16/12/2005 11:22 Page 429
