Jacques I. Mathematics for Economics and Business

Подождите немного. Документ загружается.

section 3.4

Investment appraisal

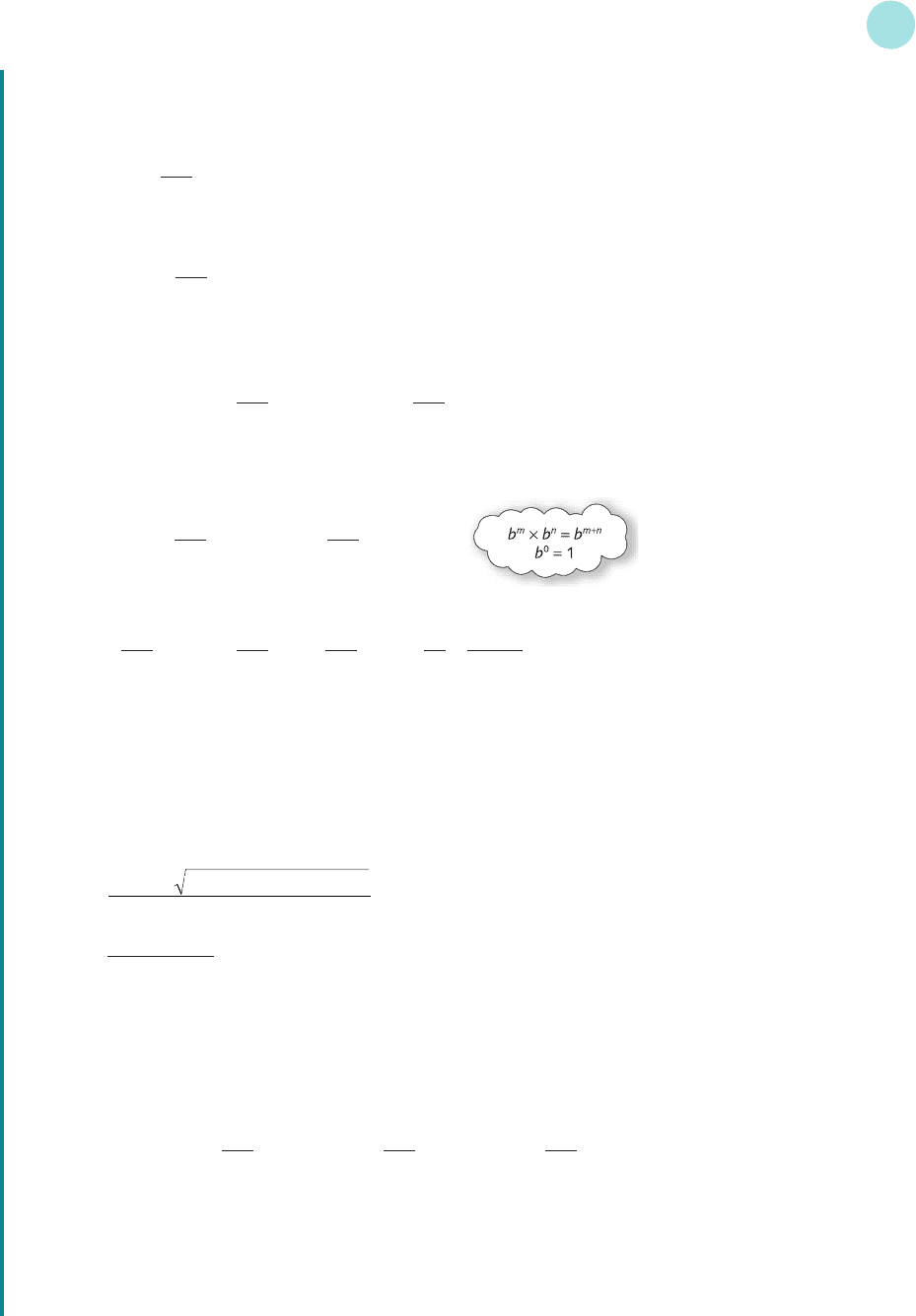
In Section 3.2 the following two formulas were used to solve compound interest problems

S = P 1 +

(1)

S = Pe

rt/100

(2)

The ﬁrst of these can be applied to any type of compounding in which the interest is added on

to the investment at the end of discrete time intervals. The second formula is used when the

interest is added on continuously. Both formulas involve the variables

P = principal

S = future value

r = interest rate

t = time

In the case of discrete compounding, the letter t represents the number of time periods. (In

Section 3.2 this was denoted by n.) For continuous compounding, t is measured in years. Given

100

Objectives

At the end of this section you should be able to:

 Calculate present values under discrete and continuous compounding.

 Use net present values to appraise investment projects.

 Calculate the internal rate of return.

 Calculate the present value of an annuity.

 Use discounting to compare investment projects.

 Calculate the present value of government securities.

MFE_C03d.qxd 16/12/2005 11:03 Page 220

any three of these variables it is possible to work out the value of the remaining variable.

Various examples were considered in Section 3.2. Of particular interest is the case where S, r

and t are given, and P is the unknown to be determined. In this situation we know the future

value, and we want to work backwards to calculate the original principal. This process is called

discounting and the principal, P, is called the present value. The rate of interest is sometimes

referred to as the discount rate. Equations (1) and (2) are easily rearranged to produce explicit

formulas for the present value under discrete and continuous compounding:

P = = S 1 +

−t

P = = Se

−rt/100

rt/100

100

(1 + r/100)

3.4 • Investment appraisal

221

Example

Find the present value of $1000 in 4 years’ time if the discount rate is 10% compounded

(a) semi-annually

(b) continuously

Solution

(a) The discount formula for discrete compounding is

P = S 1 +

−t

If compounding occurs semi-annually then r = 5 since the interest rate per 6 months is 10/2 = 5, and

t = 8 since there are eight 6-month periods in 4 years. We are given that the future value is $1000, so

P = $1000(1.05)

−8

= $676.84

(b) The discount formula for continuous compounding is

P = Se

−rt/ 100

In this formula, r is the annual discount rate, which is 10, and t is measured in years, so is 4. Hence the

present value is

P = $1000e

−0.4

= $670.32

Notice that the present value in part (b) is smaller than that in part (a). This is to be expected because con-

tinuous compounding always produces a higher yield. Consequently, we need to invest a smaller amount

under continuous compounding to produce the future value of $1000 after 4 years.

100

Practice Problem

1 Find the present value of $100 000 in 10 years’ time if the discount rate is 6% compounded

(a) annually (b) continuously

MFE_C03d.qxd 16/12/2005 11:03 Page 221

Present values are a useful way of appraising investment projects. Suppose that you are

invited to invest $600 today in a business venture that is certain to produce a return of $1000

in 5 years’ time. If the discount rate is 10% compounded semi-annually then part (a) of the

previous example shows that the present value of this return is $676.84. This exceeds the initial

outlay of $600, so the venture is regarded as proﬁtable. We quantify this proﬁt by calculating

the difference between the present value of the revenue and the present value of the costs,

which is known as the net present value (NPV). In this example, the net present value is

$676.84 − $600 = $76.84

Quite generally, a project is considered worthwhile when the NPV is positive. Moreover, if a

decision is to be made between two different projects then the one with the higher NPV is the

preferred choice.

An alternative way of assessing individual projects is based on the internal rate of return

(IRR). This is the annual rate which, when applied to the initial outlay, yields the same return

as the project after the same number of years. The investment is considered worthwhile pro-

vided the IRR exceeds the market rate. Obviously, in practice, other factors such as risk need to

be considered before a decision is made.

The following example illustrates both NPV and IRR methods and shows how a value of the

IRR itself can be calculated.

Mathematics of Finance

222

Example

A project requiring an initial outlay of $15 000 is guaranteed to produce a return of $20 000 in 3 years’ time.

Use the

(a) net present value

(b) internal rate of return

methods to decide whether this investment is worthwhile if the prevailing market rate is 5% compounded

annually. Would your decision be affected if the interest rate were 12%?

Solution

(a) The present value of $20 000 in 3 years’ time, based on a discount rate of 5%, is found by setting

S = 20 000, t = 3 and r = 5 in the formula

P = S 1 +

−t

This gives

P = $20 000(1.05)

−3

= $17 276.75

The NPV is therefore

$17 276.75 − $15 000 = $2276.75

The project is to be recommended because this value is positive.

(b) To calculate the IRR we use the formula

S = P 1 +

We are given S = 20 000, P = 15 000 and t = 3, so we need to solve

20 000 = 15 000 1 +

100

MFE_C03d.qxd 16/12/2005 11:03 Page 222

for r. An obvious ﬁrst step is to divide both sides of this equation by 15 000 to get

= 1 +

We can extract r by taking cube roots of both sides of

1 +

to get

1 +=

1/ 3

= 1.1

Hence

= 1.1 − 1 = 0.1

and so the IRR is 10%. The project is therefore to be recommended because this value exceeds the mar-

ket rate of 5%.

For the last part of the problem we are invited to consider whether our advice would be different if the

market rate were 12%. Using the NPV method, we need to repeat the calculations, replacing 5 by 12. The

corresponding net present value is then

$20 000(1.12)

−3

− $15 000 =−$764.40

This time the NPV is negative, so the project leads to an effective loss and is not to be recommended. The

same conclusion can be reached more easily using the IRR method. We have already seen that the internal

rate of return is 10% and can deduce immediately that you would be better off investing the $15 000 at the

market rate of 12%, since this gives the higher yield.

100

3.4 • Investment appraisal

223

Practice Problem

2 An investment project requires an initial outlay of $8000 and will produce a return of $17 000 at the

end of 5 years. Use the

(a) net present value

(b) internal rate of return

methods to decide whether this is worthwhile if the capital could be invested elsewhere at 15% com-

pounded annually.

Advice

This problem illustrates the use of two different methods for investment appraisal. It may

appear at first sight that the method based on the IRR is the preferred approach, particu-

larly if you wish to consider more than one interest rate. However, this is not usually the

case. The IRR method can give wholly misleading advice when comparing two or more

projects, and you must be careful when interpreting the results of this method. The fol-

lowing example highlights the difficulty.

MFE_C03d.qxd 16/12/2005 11:03 Page 223

Mathematics of Finance

224

Example

Suppose that it is possible to invest in only one of two different projects. Project A requires an initial outlay of

$1000 and yields $1200 in 4 years’ time. Project B requires an outlay of $30 000 and yields $35 000 after 4 years.

Which of these projects would you choose to invest in when the market rate is 3% compounded annually?

Solution

Let us ﬁrst solve this problem using net present values.

For Project A

NPV = $1200(1.03)

−4

− $1000 = $66.18

For Project B

NPV = $35 000(1.03)

−4

− $30 000 = $1097.05

Both projects are viable as they produce positive net present values. Moreover, the second project is preferred,

since it has the higher value. You can see that this recommendation is correct by considering how you might

invest $30 000. If you opt for Project A then the best you can do is to invest $1000 of this amount to give a

return of $1200 in 4 years’ time. The remaining $29 000 could be invested at the market rate of 3% to yield

$29 000(1.03)

= $32 639.76

The total return is then

$1200 + $32 639.76 = $33 839.76

On the other hand, if you opt for Project B then the whole of the $30 000 can be invested to yield $35 000.

In other words, in 4 years’ time you would be

$35 000 − $33 639.76 = $1160.24

better off by choosing Project B, which conﬁrms the advice given by the NPV method.

However, this is contrary to the advice given by the IRR method. For Project A, the internal rate of return,

, satisﬁes

1200 = 1000 1 +

Dividing by 1000 gives

1 +

= 1.2

and if we take fourth roots we get

1 +=(1.2)

1/ 4

= 1.047

so r

= 4.7%.

For Project B the internal rate of return, r

, satisﬁes

35 000 = 30 000 1 +

This can be solved as before to get r

= 3.9%.

Project A gives the higher internal rate of return even though, as we have seen, Project B is the preferred

choice.

100

MFE_C03d.qxd 16/12/2005 11:03 Page 224

The results of this example show that the IRR method is an unreliable way of comparing

investment opportunities when there are signiﬁcant differences between the amounts involved.

This is because the IRR method compares percentages, and obviously a large percentage of a

small sum could give a smaller proﬁt than a small percentage of a larger sum.

3.4 • Investment appraisal

225

Practice Problem

3 A firm needs to choose between two projects, A and B. Project A involves an initial outlay of $13 500

and yields $18 000 in 2 years’ time. Project B requires an outlay of $9000 and yields $13 000 after

2 years. Which of these projects would you advise the firm to invest in if the annual market rate of

interest is 7%?

So far in this section we have calculated the present value of a single future value. We now

consider the case of a sequence of payments over time. The simplest cash ﬂow of this type is an

annuity, which is a sequence of regular equal payments. It can be thought of as the opposite of

a sinking fund. This time a lump sum is invested and, subsequently, equal amounts of money

are withdrawn at ﬁxed time intervals. Provided that the payments themselves exceed the

amount of interest gained during the time interval between payments, the fund will decrease

and eventually become zero. At this point the payments cease. In practice, we are interested in

the value of the original lump sum needed to secure a regular income over a known period of

time. This can be done by summing the present values of the individual payments.

Example

Find the present value of an annuity that yields an income of $10 000 at the end of each year for 10 years,

assuming that the interest rate is 7% compounded annually.

What would the present value be if the annuity yields this income in perpetuity?

Solution

The ﬁrst payment of $10 000 is made at the end of the ﬁrst year. Its present value is calculated using the

formula

P = S 1 +

−t

with S = 10 000, r = 7 and t = 1, so

P = $10 000(1.07)

−1

= $9345.79

This means that if we want to take out $10 000 from the fund in 1 year’s time then we need to invest

$9345.79 today. The second payment of $10 000 is made at the end of the second year, so its present value

$10 000(1.07)

−2

= $8734.39

This is the amount of money that needs to be invested now to cover the second payment from the fund. In

general, the present value of $10 000 in t years’ time is

10 000(1.07)

−t

100



MFE_C03d.qxd 16/12/2005 11:03 Page 225

so the total present value is

10 000(1.07)

−1

+ 10 000(1.07)

−2

+ ...+ 10 000(1.07)

−10

This is a geometric series, so we may use the formula

In this case, a = 10 000(1.07)

−1

, r = 1.07

−1

and n = 10, so the present value of the annuity is

$10 000(1.07)

−1

= $70 235.82

This represents the amount of money that needs to be invested now so that a regular annual income of

$10 000 can be withdrawn from the fund for the next 10 years.

If the income stream is to continue for ever then we need to investigate what happens to the formula

as n gets bigger and bigger. In this case r = 1.07

−1

< 1, so as n increases, r

decreases and tends towards zero.

This behaviour can be seen clearly from the table:

n 1 10 100

1.07

−n

0.9346 0.5083 0.0012

Setting r

= 0 in the formula for the sum of geometric series shows that if the series goes on for ever then

eventually the sum approaches

so that the present value of the annuity in perpetuity is

= $142 857.14

10 000(1.07)

−1

1 − 1.07

−1

1 − r

− 1

r − 1

1.07

−10

− 1

1.07

−1

− 1

r − 1

Mathematics of Finance

226

Practice Problem

4 Find the present value of an annuity that yields an income of $2000 at the end of each month for

10 years, assuming that the interest rate is 6% compounded monthly.

The argument used in the previous example can be used to calculate the net present value.

For instance, suppose that a business requires an initial investment of $60 000, which is guar-

anteed to return a regular payment of $10 000 at the end of each year for the next 10 years. If

the discount rate is 7% compounded annually then the previous example shows that the pre-

sent value is $70 235.82. The net present value of the investment is therefore

$70 235.82 − $60 000 = $10 235.82

A similar procedure can be used when the payments are irregular, although it is no longer

possible to use the formula for the sum of a geometric progression. Instead the present value

of each individual payment is calculated and the values are then summed longhand.

MFE_C03d.qxd 16/12/2005 11:03 Page 226

3.4 • Investment appraisal

227

Table 3.19

Revenue ($)

End of year Project A Project B

1 6 000 10 000

2 3 000 6 000

3 10 000 9 000

4 8 000 1 000

Total 27 000 26 000

Table 3.20

Discounted revenue ($)

End of year Project A Project B

1 5 405.41 9 000.01

2 2 434.87 4 869.73

3 7 311.91 6 580.72

4 5 269.85 658.73

Total 20 422.04 21 109.19

Example

A small business has a choice of investing $20 000 in one of two projects. The revenue ﬂows from the two

projects during the next 4 years are listed in Table 3.19. If the interest rate is 11% compounded annually,

which of these two projects would you advise the company to invest in?



Solution

If we simply add together all of the individual receipts, it appears that Project A is to be preferred, since the

total revenue generated from Project A is $1000 greater than that from Project B. However, this naïve

approach fails to take into account the time distribution.

From Table 3.19 we see that both projects yield a single receipt of $10 000. For Project A this occurs at

the end of year 3, whereas for Project B this occurs at the end of year 1. This $10 000 is worth more in Project

B because it occurs earlier in the revenue stream and, once received, could be invested for longer at the pre-

vailing rate of interest. To compare these projects we need to discount the revenue stream to the present

value. The present values obtained depend on the discount rate. Table 3.20 shows the present values based

on the given rate of 11% compounded annually. These values are calculated using the formula

P = S(1.11)

−t

For example, the present value of the $10 000 revenue in Project A is given by

$10 000(1.11)

−3

= $7311.91

The net present values for Project A and Project B are given by

$20 422.67 − $20 000 = $422.67

MFE_C03d.qxd 16/12/2005 11:03 Page 227

It is sometimes useful to ﬁnd the internal rate of return of a project yielding a sequence of payments over

time. However, as the following example demonstrates, this can be difﬁcult to calculate, particularly when

there are more than two payments.

Mathematics of Finance

228

Practice Problem

5 A firm has a choice of spending $10 000 today on one of two projects. The revenue obtained from

these projects is listed in Table 3.21. Assuming that the discount rate is 15% compounded annually,

which of these two projects would you advise the company to invest in?

and

$21 109.19 − $20 000 = $1109.19

respectively. Consequently, if it is possible to invest in only one of these projects, the preferred choice is

Project B.

Table 3.21

Revenue ($)

End of year Project 1 Project 2

1 2000 1000

2 2000 1000

3 3000 2000

4 3000 6000

5 3000 4000

Example

(a) Calculate the IRR of a project which requires an initial outlay of $20 000 and produces a return of $8000

at the end of year 1 and $15 000 at the end of year 2.

(b) Calculate the IRR of a project which requires an initial outlay of $5000 and produces returns of $1000,

$2000 and $3000 at the end of years 1, 2 and 3, respectively.

Solution

(a) In the case of a single payment, the IRR is the annual rate of interest, r, which, when applied to the

initial outlay, P, yields a known future payment, S. If this payment is made after t years then

S = P 1 +

or, equivalently

P = S 1 +

−t

100

MFE_C03d.qxd 16/12/2005 11:03 Page 228

Note that the right-hand side of this last equation is just the present value of S. Consequently, the IRR

can be thought of as the rate of interest at which the present value of S equals the initial outlay P.

The present value of $8000 in 1 year’s time is

8000 1 +

−1

where r is the annual rate of interest. Similarly, the present value of $15 000 in 2 years’ time is

15 000 1 +

−2

If r is to be the IRR then the sum of these present values must equal the initial investment of $20 000.

In other words, the IRR is the value of r that satisﬁes the equation

20 000 = 8000 1 +

−1

+ 15 000 1 +

−2

The simplest way of solving this equation is to multiply both sides by (1 + r/100)

to remove all

negative indices. This gives

20 000 1 +

= 8000 1 ++15 000

Now

1 +

= 1 + 1 +=1 ++

so if we multiply out the brackets, we obtain

20 000 + 400r + 2r

= 8000 + 80r + 15 000

Collecting like terms gives

+ 320r − 3000 = 0

This is a quadratic in r, so can be solved using the formula described in Section 2.1 to get

r =

= 8.9% or −168.9%

We can obviously ignore the negative solution, so can conclude that the IRR is 8.9%.

(b) If an initial outlay of $5000 yields $1000, $2000 and $3000 at the end of years 1, 2 and 3, respectively,

then the internal rate of return, r, satisﬁes the equation

5000 = 1000 1 +

−1

+ 2000 1 +

−2

+ 3000 1 +

−3

A sensible thing to do here might be to multiply through by (1 + (r/100))

−3

. However, this produces an

equation involving r

(and lower powers of r), which is no easier to solve than the original. Indeed, a

moment’s thought should convince you that in general, when dealing with a sequence of payments over

n years, the IRR will satisfy an equation involving r

(and lower powers of r). Under these circumstances

100

−320 ± 355.5

−± − −320 320 4 2 3000

(( ) ( )( ))

()

10 000

100

3.4 • Investment appraisal

229



MFE_C03d.qxd 16/12/2005 11:03 Page 229