Hirsch M., Smale S., Devaney R., Differential Equations, Dynamical Systems, and an Introduction to Chaos
Подождите немного. Документ загружается.


376 Chapter 16 Homoclinic Phenomena
F
0
(R)
F
1
(R)
(a) (b)
b
a
R
F
0
(b)
F
1
(b)
F
1
(a)
F
0
(a)
Figure 16.12 The images F
λ
(R) for (a) λ = 0 and
(b) λ =1.
We now introduce a parameter into the double scroll system. When = 0
the system will be the double scroll system considered in the previous section.
When = 0 we change the system by simply translating ζ
+
∩ C (and the
corresponding transit map) in the z direction by . More precisely, we assume
that the system remains unchanged in the cylindrical region r ≤ 1, |z|≤1,
but we change the transit map defined on the upper disk D
+
by adding (0, )
to the image. That is, the new Poincaré map is given on C
+
by
+
(θ, z) =
1
2
√
z sin
θ + log(
√
z)
1
2
√
z cos
θ + log(
√
z)
+
and
−
is defined similarly using the skew-symmetry of the system. We further
assume that is chosen small enough (|| < 1/2) so that
±
(C) ⊂ C.
When > 0, ζ
+
intersects C in the upper cylindrical region C
+
and then,
after passing close to the origin, winds around itself before reintersecting C
a second time. When < 0, ζ
+
now meets C in C
−
and then takes a very
different route back to C, this time winding around ζ
−
.
Recall that
+
0
has infinitely many fixed points in C
±
. This changes
dramatically when = 0.
Proposition. The maps
±
each have only finitely many fixed points in C
±
when = 0.
Proof: To find fixed points of
+
, we must solve
θ =
√
z
0
2
sin
θ + log(
√
z)
z =
√
z
0
2
cos
θ + log(
√
z)
+

16.4 Homoclinic Bifurcations 377
where > 0. As in Section 16.1, we must therefore have
z
4
= θ
2
+ (z − )
2
so that
θ =±
1
2
z − 4(z − )
2
.
In particular, we must have
z − 4(z − )
2
≥ 0
or, equivalently,
4(z − )
2
z
≤ 1.
This inequality holds provided z lies in the interval I
defined by
1
8
+ −
1
8
√
1 + 16 ≤ z ≤
1
8
+ +
1
8
√
1 + 16.
This puts a further restriction on for
+
to have fixed points, namely,
> −1/16. Note that, when > −1/16, we have
1
8
+ −
1
8
√
1 + 16 > 0
so that I
has length
√
1 + 16/4 and this interval lies to the right of 0.
To determine the z-values of the fixed points, we must now solve
cos
±
1
2
z − 4(z − )
2
+ log(
√
z)
=
2(z − )
√
z
or
cos
2
±
1
2
z − 4(z − )
2
+ log(
√
z)
=
4(z − )
2
z
.
With a little calculus, one may check that the function
g (z) =
4(z − )
2
z

378 Chapter 16 Homoclinic Phenomena
has a single minimum 0 at z = and two maxima equal to 1 at the endpoints
of I
. Meanwhile the graph of
h(z) = cos
2
±
1
2
z − 4(z − )
2
+ log(
√
z)
oscillates between ±1 only finitely many times in I
. Hence h(z) = g (z)
at only finitely many z-values in I
. These points are the fixed points
for
±
.
Note that, as → 0, the interval I
tends to [0, 1/4] and so the number of
oscillations of h in I
increases without bound. Therefore we have
Corollary. Given N ∈
Z, there exists
N
such that if 0 < <
N
, then
+
has at least N fixed points in C
+
.
When > 0, the unstable curve misses the stable surface in its first pass
through C. Indeed, ζ
+
crosses C
+
at θ = 0, z = . This does not mean that
there are no homoclinic orbits when = 0. In fact, we have the following
proposition:
Proposition. There are infinitely many values of for which ζ
±
are
homoclinic solutions that pass twice through C.
Proof: To show this, we need to find values of for which
+
(0, ) lies on
the stable surface of the origin. Thus we must solve
0 =
√
2
cos
0 − log(
√
)
+
or
−2
√
= cos
−log(
√
)
.
But, as in Section 16.1, the graph of cos(−log
√
) meets that of −2
√
infinitely often. This completes the proof.
For each of the -values for which ζ
±
is a homoclinic solution, we again have
infinitely many fixed points (for
±
◦
±
) as well as a very different structure
for the attractor. Clearly, a lot is happening as changes. We invite the reader
who has lasted this long with this book to go and figure out everything that is
happening here. Good luck! And have fun!
16.5 Exploration: The Chua Circuit 379
16.5 Exploration: The Chua Circuit
In this exploration, we investigate a nonlinear three-dimensional system of
differential equations related to an electrical circuit known as Chua’s circuit.
These were the first examples of circuit equations to exhibit chaotic behavior.
Indeed, for certain values of the parameters these equations exhibit behavior
similar to the double scroll attractor in Section 16.3. The original Chua circuit
equations possess a piecewise linear nonlinearity. Here we investigate a varia-
tion of these equations in which the nonlinearity is given by a cubic function.
For more details on the Chua circuit, refer to [11] and [25].
The nonlinear Chua circuit system is given by
x
= a(y − φ(x))
y
= x − y + z
z
=−bz
where a and b are parameters and the function φ is given by
φ(x) =
1
16
x
3
−
1
6
x.
Actually, the coefficients of this polynomial are usually regarded as parameters,
but we will fix them for the sake of definiteness in this exploration. When
a = 10. 91865 ...and b = 14, this system appears to have a pair of symmetric
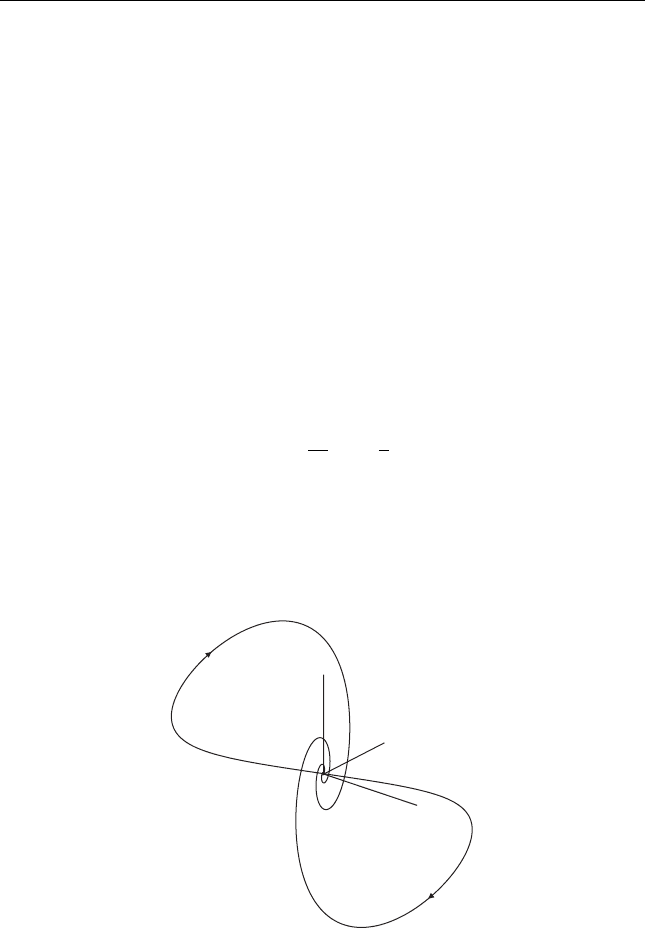
homoclinic orbits as illustrated in Figure 16.13. The goal of this exploration
x
y
z
Figure 16.13 A pair of homoclinic
orbits in the nonlinear Chua system
at parameter values a =
10.91865... and b = 14.

380 Chapter 16 Homoclinic Phenomena
is to investigate how this system evolves as the parameter a changes. As a
consequence, we will also fix the parameter b at 14 and then let a vary. We
caution the explorer that proving any of the chaotic or bifurcation behavior
observed below is nearly impossible; virtually anything that you can do in this
regard would qualify as an interesting research result.
1. As always, begin by finding the equilibrium points.
2. Determine the types of these equilibria, perhaps by using a computer
algebra system.
3. This system possesses a symmetry; describe this symmetry and tell what
it implies for solutions.
4. Let a vary from 6 to 14. Describe any bifurcations you observe as a varies.
Be sure to choose pairs of symmetrically located initial conditions in this
and other experiments in order to see the full effect of the bifurcations.
Pay particular attention to solutions that begin near the origin.
5. Are there values of a for which there appears to be an attractor for this
system? What appears to be happening in this case? Can you construct a
model?
6. Describe the bifurcation that occurs near the following a-values:
(a) a = 6. 58
(b) a = 7. 3
(c) a = 8. 78
(d) a = 10. 77
EXERCISES
1. Prove that
d[(s), (t)]=
∞
i=−∞
|s
i
− t
i
|
2
|i|
is a distance function on
2
, where
2
is the set of doubly infinite
sequences of 0’s and 1’s as described in Section 16.2.
2. Prove that the shift σ is a homeomorphism of
2
.
3. Prove that S : →
2
gives a conjugacy between σ and F.
4. Construct a dense orbit for σ .
5. Prove that periodic points are dense for σ .
6. Let s
∗
∈
2
. Prove that W
s
(s
∗
) consists of precisely those sequences
whose entries agree with those of s
∗
to the right of some entry of s
∗
.
7. Let (0) = (...00. 000 ...) ∈
2
. A sequence s ∈
2
is called homoclinic
to (0)ifs ∈ W
s
(0) ∩ W
u
(0). Describe the entries of a sequence that is
Exercises 381
homoclinic to (0). Prove that sequences that are homoclinic to (0) are
dense in
2
.
8. Let (1) = (...11. 111 ...) ∈
2
. A sequence s is a heteroclinic sequence if
s ∈ W
s
(0)∩W
u
(1). Describe the entries of such a heteroclinic sequence.
Prove that such sequences are dense in
2
.
9. Generalize the definitions of homoclinic and heteroclinic points to
arbitrary periodic points for σ and reprove Exercises 7 and 8 in this
case.
10. Prove that the set of homoclinic points to a given periodic point is
countable.
11. Let
1
2
denote the set of one-sided sequences of 0’s and 1’s. Define
:
1
2
→
2
by
(s
0
s
1
s
2
...) = (...s
5
s
3
s
1
· s
0
s
2
s
4
...).
Prove that is a homeomorphism.
12. Let X
∗
denote the fixed point of F in H
0
for the horseshoe map. Prove
that the closure of W
u
(X
∗
) contains all points in as well as points on
their unstable curves.
13. Let R :
2
→
2
be defined by
R(...s
−2
s
−1
. s
0
s
1
s
2
...) = (...s
2
s
1
s
0
. s
−1
s
−2
...).
Prove that R ◦ R = id and that σ ◦ R = R ◦ σ
−1
. Conclude that
σ = U ◦ R where U is a map that satisfies U ◦ U = id. Maps that
are their own inverses are called involutions. They represent very simple
types of dynamical systems. Hence the shift may be decomposed into a
composition of two such maps.
14. Let s be a sequence that is fixed by R, where R is as defined in the previous
exercise. Suppose that σ
n
(s) is also fixed by R. Prove that s is a periodic
point of σ of period 2n.
15. Rework the previous exercise, assuming that σ
n
(s) is fixed by U , where
U is given as in Exercise 13. What is the period of s?
16. For the Lorenz system in Chapter 14, investigate numerically the bifur-
cation that takes place for r between 13. 92 and 13. 96, with σ = 10 and
b = 8/3.
This Page Intentionally Left Blank

17
Existence and
Uniqueness Revisited
In this chapter we return to the material presented in Chapter 7, this time filling
in all of the technical details and proofs that were omitted earlier. As a result,
this chapter is more difficult than the preceding ones; it is, however, central
to the rigorous study of ordinary differential equations. To comprehend thor-
oughly many of the proofs in this section, the reader should be familiar with
such topics from real analysis as uniform continuity, uniform convergence of
functions, and compact sets.
17.1 The Existence and Uniqueness
Theorem
Consider the autonomous system of differential equations
X
= F(X)
where F :
R
n
→ R
n
. In previous chapters, we have usually assumed that F
was C
∞
; here we will relax this condition and assume that F is only C
1
. Recall
that this means that F is continuously differentiable. That is, F and its first
partial derivatives exist and are continuous functions on
R
n
. For the first few
383
384 Chapter 17 Existence and Uniqueness Revisited
sections of this chapter, we will deal only with autonomous equations; later
we will assume that F depends on t as well as X.
As we know, a solution of this system is a differentiable function X : J →
R
n
defined on some interval J ⊂ R such that for all t ∈ J
X
(t) = F(X (t )).
Geometrically, X(t) is a curve in
R
n
whose tangent vector X
(t) equals
F(X (t )); as in previous chapters, we think of this vector as being based at
X(t ), so that the map F :
R
n
→ R
n
defines a vector field on R
n
.Aninitial
condition or initial value for a solution X : J →
R
n
is a specification of the
form X(t
0
) = X
0
where t
0
∈ J and X
0
∈ R
n
. For simplicity, we usually take
t
0
= 0.
A nonlinear differential equation may have several solutions that satisfy
a given initial condition. For example, consider the first-order nonlinear
differential equation
x
= 3x
2/3
.
In Chapter 7 we saw that the identically zero function u
0
: R → R given by
u
0
(t) ≡ 0 is a solution satisfying the initial condition u(0) = 0. But u
1
(t) = t
3
is also a solution satisfying this initial condition, and, in addition, for any τ > 0,
the function given by
u
τ
(t) =
0ift ≤ τ
(t − τ )
3
if t>τ
is also a solution satisfying the initial condition u
τ
(0) = 0.
Besides uniqueness, there is also the question of existence of solutions. When
we dealt with linear systems, we were able to compute solutions explicitly. For
nonlinear systems, this is often not possible, as we have seen. Moreover, certain
initial conditions may not give rise to any solutions. For example, as we saw
in Chapter 7, the differential equation
x
=
1ifx<0
−1ifx ≥ 0
has no solution that satisfies x(0) = 0.
Thus it is clear that, to ensure existence and uniqueness of solutions, extra
conditions must be imposed on the function F. The assumption that F is
continuously differentiable turns out to be sufficient, as we shall see. In the
first example above, F is not differentiable at the problematic point x = 0,
while in the second example, F is not continuous at x = 0.

17.2 Proof of Existence and Uniqueness 385
The following is the fundamental local theorem of ordinary differential
equations.
The Existence and Uniqueness Theorem. Consider the initial value
problem
X
= F(X), X (0) = X
0
where X
0
∈ R
n
. Suppose that F : R
n
→ R
n
is C
1
. Then there exists a unique
solution of this initial value problem. More precisely, there exists a > 0 and a
unique solution
X : (−a, a) →
R
n
of this differential equation satisfying the initial condition
X(0) = X
0
.
We will prove this theorem in the next section.
17.2 Proof of Existence and Uniqueness
We need to recall some multivariable calculus. Let F : R
n
→ R
n
.In
coordinates (x
1
, ..., x
n
)onR
n
, we write
F(X ) = (f
1
(x
1
, ..., x
n
), ..., f
n
(x
1
, ..., x
n
)).
Let DF
X
be the derivative of F at the point X ∈ R
n
. We may view this derivative
in two slightly different ways. From one point of view, DF
X
is a linear map
defined for each point X ∈
R
n
; this linear map assigns to each vector U ∈ R
n
the vector
DF
X
(U ) = lim
h→0
F(X + hU ) − F(X )
h
,
where h ∈
R. Equivalently, from the matrix point of view, DF
X
is the n × n
Jacobian matrix
DF
X
=
∂f
i
∂x
j
where each derivative is evaluated at (x
1
, ..., x
n
). Thus the derivative may be
viewed as a function that associates different linear maps or matrices to each
point in
R
n
. That is, DF : R
n
→ L(R
n
).
